Exact teleparallel gravity of binary black holes

Abstract

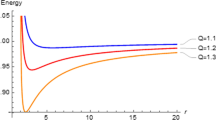
An exact solution of two singularities in the teleparallel equivalent to general relativity theory has been obtained. A holographic visualization of the binary black holes (BBHs) space-time, due to the non vanishing torsion scalar field, has been given. The acceleration tensor of BBHs space-time has been calculated. The results identify the repulsive gravity zones of the BBHs field. The total conserved quantities of the BBHs has been evaluated. Possible gravitational radiation emission by the system has been calculated without assuming a weak field initial data.

Appendix: Notation

As is known, the exterior product is indicated by ∧, however the interior product of a vector $\xi$ and a p-form $\varPsi$ is indicated by $\xi \rfloor\varPsi$. The vector basis dual to the frame 1-forms $\vartheta^{\alpha}$ is indicated by $e_{\alpha}$ and they fulfil $e_{\alpha}\rfloor\vartheta^{\beta}={\delta}_{\alpha}^{\beta}$. By using local coordinates $x^{i}$, we get $\vartheta^{\alpha}=h^{\alpha}_{i} dx^{i}$ and $e_{\alpha}=h^{i}_{\alpha}\partial_{i}$ with $h^{\alpha}_{i}$ and $h^{i}_{\alpha}$ are the covariant and contravariant components of the tetrad field. The volume 4-form is defined by $\eta\stackrel{\mathrm{def.}}{=} \vartheta^{\hat{0}}\wedge\vartheta^{\hat{1}}\wedge \vartheta^{\hat{2}}\wedge\vartheta^{\hat{3}}$. Additionally, by using the interior product we define

$$\eta_{\alpha}\stackrel {\mathrm{def.}}{=} e_{\alpha}\rfloor\eta= \frac{1}{3!} \epsilon_{\alpha\beta\gamma\delta} \vartheta^{\beta}\wedge \vartheta^{\gamma}\wedge\vartheta^{\delta}, $$

with $\epsilon_{\alpha\beta\gamma\delta}$ is completely antisymmetric tensor and $\epsilon_{0123}=1$

$$\begin{aligned} &{\eta_{\alpha\beta} := e_{\beta}\rfloor\eta_{\alpha}= \frac{1}{2!}\epsilon_{\alpha\beta\gamma\delta} \vartheta^{\gamma}\wedge \vartheta^{\delta},} \end{aligned}$$

(62)

$$\begin{aligned} &{\eta_{\alpha\beta\gamma} := e_{\gamma}\rfloor\eta_{\alpha\beta}= \frac{1}{1!} \epsilon_{\alpha\beta \gamma\delta} \vartheta^{\delta},} \end{aligned}$$

(63)

that are the bases for 3-, 2- and 1-forms respectively. Finally,

$$\eta_{\alpha\beta\mu\nu} \stackrel{\mathrm{def.}}{=} e_{\nu}\rfloor \eta_{\alpha\beta\mu}= e_{\nu}\rfloor e_{\mu}\rfloor e_{\beta}\rfloor e_{\alpha}\rfloor \eta, $$

is the Levi-Civita tensor density. The $\eta$-forms fulfil the following useful identities:

$$\begin{aligned} \begin{aligned} {}&\vartheta^{\beta}\wedge \eta_{\alpha}:= \delta^{\beta}_{\alpha}\eta, \\ &\vartheta^{\beta}\wedge\eta_{\mu\nu} := \delta^{\beta}_{\nu}\eta_{\mu}-\delta^{\beta}_{\mu}\eta_{\nu}, \\ &\vartheta^{\beta}\wedge\eta_{\alpha\mu\nu} := \delta^{\beta}_{\alpha}\eta_{\mu\nu}+\delta^{\beta}_{\mu}\eta_{\nu\alpha}+ \delta^{\beta}_{\nu}\eta_{ \alpha\mu}, \\ &\vartheta^{\beta}\wedge\eta_{\alpha\gamma\mu\nu} := \delta^{\beta}_{\nu}\eta_{\alpha\gamma \mu}-\delta^{\beta}_{\mu}\eta_{\alpha\gamma\nu }+\delta^{\beta}_{\gamma}\eta_{ \alpha\mu\nu}- \delta^{\beta}_{\alpha}\eta_{ \gamma\mu\nu}. \end{aligned} \end{aligned}$$

(64)

The line element $ds^{2} := g_{\alpha\beta} \vartheta^{\alpha}\bigotimes\vartheta^{\beta}$ is fulfil by the space-time metric $g_{\alpha\beta}$.

One can consider teleparallel geometry as a gauge theory of translation (Hehl et al. 1995; Hayashi 1977; Hayashi and Shirafuji 1979; Obukhov et al. 2006; Nashed 2010a). In such geometry the coframe $\vartheta^{\alpha}$ plays the role of the gauge translational potential of the gravitational field. General relativity can be reconstructed as the teleparallel theory. From geometric viewpoint, teleparallel gravity can be regarded as a particular case of the metric-affine gravity whose coframe 1-form $\vartheta^{\alpha}$ and Lorentz connection are due to distant parallelism constraint ${R_{\alpha}}^{\beta}=0$ (Nashed 2010a; Obukhov and Pereira 2004; Obukhov et al. 2006). In such geometry the torsion 2-form

$$\begin{aligned} {\mathcal{T}}^{\alpha} =&D\vartheta^{\alpha}=d \vartheta^{\alpha}+{\varGamma_{\beta}}^{\alpha}\wedge \vartheta^{\beta} \\ =&\frac{1}{2}{\mathcal{T}_{\mu\nu}}^{\alpha}\vartheta^{\mu}\wedge\vartheta^{\nu}=\frac{1}{2}{ \mathcal{T}_{i j}}^{\alpha}dx^{i} \wedge dx^{j}, \end{aligned}$$

(65)

occurs as the gravitational gauge field strength, ${\varGamma_{\alpha}}^{\beta}$ is the Weitzenböck connection 1-form, $d$ being the exterior derivative and finally $D$ is the exterior covariant derivative. The torsion $\mathcal{T}^{\alpha}$ can be divided into three irreducible parts: the tensor part, the trace, and the axial trace, provided by (Hayashi 1977; Hayashi and Shirafuji 1979; Blagojevic and Vasilic 1988; Kawai 2000; Kawai et al. 2000; Lucas et al. 2009)

$$ {^{{( 1)}}{\mathcal{T}}^{\alpha}} := \mathcal{T}^{\alpha}-{^{{( 2)}}{\mathcal{T}}^{\alpha}}-{^{{( 3)}}{ \mathcal{T}}^{\alpha}}, $$

(66)

with

$$ {^{{( 2)}}{\mathcal{T}}^{\alpha}}:=\frac{1}{3} \vartheta^{\alpha}\wedge{\mathcal{T}}, $$

(67)

where $\mathcal{T}= (e_{\beta}\rfloor{\mathcal{T}}^{\beta})$ and $e_{\alpha}\rfloor {\mathcal{T}}={\mathcal{T}_{\mu\alpha}}^{\mu}$ vectors of torsion trace, and

$$ {^{{( 3)}}{\mathcal{T}}^{\alpha}} :=\frac{1}{3} e^{\alpha}\rfloor {\mathcal{P}}, $$

(68)

with $\mathcal{P}= (\vartheta^{\beta}\wedge{\mathcal{T}}_{\beta})$ and $e_{\alpha}\rfloor P=\mathcal{T}^{\mu\nu\lambda}\eta_{\mu\nu \lambda \alpha}$ the axial torsion trace.