Abstract
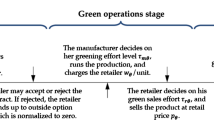
This paper examines the optimal channel structure of a green supply chain consisting of one manufacturer and one retailer. The manufacturer, who is the Stackelberg leader, is responsible for green technology costs. Consumers prefer green products and therefore are green aware. We study four channel structures: a manufacturer’s dual-channel supply chain, a retailer’s dual-channel supply chain, a manufacturer-online and retailer-offline (hybrid I) structure, and a manufacturer-offline and retailer-online (hybrid II) structure. For each structure, we analytically investigate the impact of consumers’ green awareness and proportion of online and offline consumers on the level of green technology, profits, and retail prices. We also examine the effect on the optimal solutions of the manufacturer and retailer when they share the green cost. The results show that the manufacturer’s dual-channel supply chain performs the best in improving the greenness of products and its own profits. Concerning hybrid dual-channel supply chains, the manufacturer will always choose the channel with the majority of consumers to directly sell products through. The retailer, in most cases, also prefers to operate two channels simultaneously. In addition, regardless of the type of channel structure involved, consumers’ green awareness encourages the manufacturer to improve the greenness of its products; however, the proportion of online consumers has a positive effect on the greenness of products in the retailer-offline and manufacturer-online cases but a negative effect in the retailer-online and manufacturer-offline cases.



Similar content being viewed by others
Notes
For more information, please refer to https://www.mi.com/about/history.
For more information, please refer to https://www.gree.com/nyxst.
For more information, please refer to https://www.gree.com/Article/view/18927.
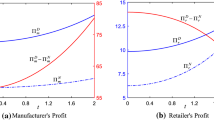
We further investigate the reason to find that with an increase in the proportion, the online sales profit gradually increases from less than that of the offline sales profit to greater than that of the offline profit. Thus, it resembles Lemma 1.
Equality may hold in one of the inequality signs.
If \(\overline{{\rho_{0} }} < 0\), then \(t^{M} > t^{H} > t^{HH} > t^{R}\).
Due to the complexity of the solutions, it is infeasible to analyze the relations between the threshold values. Thus, we resort to numerical examples to validate the current conclusions. However, unfortunately, they are parameter dependent.
To make sure the solutions are meaningful, the values that result in negative outcomes are omitted and replaced.
References
Ai, X. Z., Chen, J., & Ma, J. H. (2012). Contracting with demand uncertainty under supply chain competition. Annals of Operations Research, 201(1), 17–38.
Amacher, G. S., Koskela, E., & Ollikainen, M. (2004). Environmental quality competition and eco-labeling. Journal of Environmental Economics and Management., 47, 284–306.
Chan, C. K., Man, N., & Campbell, J. F. (2020). Supply chain coordination with reverse logistics: A vendor/recycler–-buyer synchronized cycles model. Omega. https://doi.org/10.1016/j.omega.2019.07.006
Chiang, W. Y. K., Chhajed, D., & Hess, J. D. (2003). Direct marketing, indirect profits: A strategic analysis of dual-channel supply chain design. Management Science, 49(1), 1–20.
Chitra, K. (2007). In search of the green consumers: A perceptual study. Journal of Services Research, 7(1), 173–191.
Choi, S. C. (1991). Price competition in a channel structure with a common retailer. Marketing Science, 10(4), 271–279.
Ghosh, D., & Shah, J. (2012). A comparative analysis of greening policies across supply chain structures. International Journal of Production Economics, 135(2), 568–583.
Henriques, I., & Sadorsky, P. (1999). The relationship between environmental commitment and managerial perception of stakeholder importance. The Academy of Management Journal., 42(1), 89–99.
Heydari, J., Govindan, K., & Basiri, Z. (2021). Balancing price and green quality in presence of consumer environmental awareness: A green supply chain coordination approach. International Journal of Production Research, 59(7), 1957–1975.
Hsiao, L., & Chen, Y. J. (2014). Strategic motive for introducing internet channels in a supply chain. Production and Operations Management, 23(1), 36–47.
Klasse, R. D., & McLaughlin, C. P. (1996). The impact of environmental management on firm performance. Management Science, 42(8), 1199–1214.
Kök, A. G., Shang, K., & Yücel, S. (2018). Impact of electricity pricing policies on renewable energy investments and carbon emissions. Management Science., 64(1), 131–148.
Kumar, S., & Putnam, V. (2008). Cradle to cradle: Reverse logistics strategies and opportunities across three industry sectors. International Journal of Production Economics, 115(2), 305–315.
Lei, Q., He, J., Ma, C., & Jin, Z. L. (2020). The impact of consumer behavior on preannounced pricing for a dual-channel supply chain. International Transactions in Operational Research, 27(6), 2949–2975.
Li, J., Liang, J., Shi, V., & Zhu, J. (2021). The benefit of manufacturer encroachment considering consumer’s environmental awareness and product competition. Annals of Operations Research,. https://doi.org/10.1007/s10479-021-04185-y
Lin, Y. T., Sun, H. Y., & Wang, S. Q. (2020). Designing Sustainable Products Under Coproduction Technology. Manufacturing & Service Operations Management, 22(6), 1181–1198.
Liu, Y., Huang, C., Song, Q. L. G., & Xiong, Y. (2018). Carbon emissions reduction and transfer in supply chains under a cap-and-trade system with emission-sensitive demand. System Science & Control Engineering, 6(2), 37–44.
Liu, Z. L., Anderson, T. D., & Cruz, J. M. (2012). Consumer environmental awareness and competition in two-stage supply chains. International Journal of Production Research, 218, 602–613.
Lu, Q. H., & Liu, N. (2015). Effects of e-commerce channel entry in a two-echelon supply chain: A comparative analysis of single- and dual-channel distribution systems. International Journal of Production Economics, 165, 100–111.
Peter, C., Verhoef, P. K., & InmanJ, J. (2015). From multi-channel retailing to omni-channel retailing: Introduction to the special issue on multi-channel retailing. Journal of Retailing, 91(2), 174–181.
Ranjan, A., & Jha, J. K. (2019). Pricing and coordination strategies of a dual-channel supply chain considering green quality and sales effort. Journal of Cleaner Production, 218, 409–424.
Sundarakani, B., Souza, B., Goh, M., Wagner, S. M., & Manikandan, M. (2010). Modeling carbon footprints across the supply chain. International Journal of Production Economics, 128, 43–50.
Swami, S., & Saha, J. (2013). Channel co-ordination in green supply chain management. The Journal of the Operational Research Society, 64(3), 336–351.
Tao, F., Lai, K., Wang, Y. Y., & Fan, T. J. (2020). Determinant on RFID technology investment for dominant retailer subject to inventory misplacement. International Transactions in Operational Research, 27(2), 1058–1079.
Tsay, A., & Agrawal, N. (2000). Channel dynamics under price and service competition. Manufacturing and Service Operations Management, 2(4), 372–391.
Wang, J. C., Yang, L., Wang, Y. Y., & Wang, Z. H. (2018). Optimal pricing contracts and level of information asymmetry in a supply chain. International Transactions in Operational Research, 25(5), 1583–1610.
Wang, R., Zhou, X., & Li, B. (2021). Pricing strategy of dual-channel supply chain with a risk-averse retailer considering consumers’ channel preferences. Annals of Operations Research. https://doi.org/10.1007/s10479-021-04326-3
Wang, W., Li, G., & Cheng, T. C. E. (2016). Channel selection in a supply chain with a multi-channel retailer: The role of channel operating costs. International Journal of Production Economics, 173, 54–65.
Wang, X., Cho, S. H., & Scheller-Wolf, A. (2021). Green technology development and adoption: Competition, regulation, and uncertainty-a global game approach. Management Science, 67(1), 201–219.
Wang, Y. Y., Fan, R. J., Shen, L., & Zhou, J. M. (2020). Decisions and coordination of green e-commerce supply chain considering green manufacturer’s fairness concerns. International Journal of Production Research, 58(24), 7471–7489.
Wen, D. P., Xiao, T. J., & Dastani, M. (2021). Channel choice for an independent remanufacturer considering environmentally responsible consumers. International Journal of Production Economics,. https://doi.org/10.1016/j.ijpe.2020.107941
Xie, J. P., Liang, L., Liu, L. H., & Ieromonachou, P. (2017). Coordination contracts of dual-channel with cooperation advertising in closed-loop supply chains. International Journal of Production Economics, 183, 528–538.
Xu, L., Wang, C., & Jhao, J. (2018). Decision and co-ordination in the dual-channel supply chain considering cap-and-trade regulation. Journal of Cleaner Production, 197, 551–561.
Yang, L., Ji, J. N., Wang, M. Z., & Wang, Z. Z. (2018). The Manufacturer’s Joint Decisions of Channel Selections and Carbon Emission Reductions under the Cap-and-Trade Regulation. Journal of Cleaner Production, 193, 506–523.
Yu, Y. G., Han, X. Y., & Hu, G. P. (2016). Optimal production for manufacturers considering consumer environmental awareness and green subsidies. International Journal of Production Economics, 182, 397–408.
Zhang, T., Feng, X. H., & Wang, N. N. (2020). Manufacturer encroachment and product assortment under vertical differentiation. European Journal of Operational Research, 293(1), 120–132.
Zhang, Y. M., & Hezarkhani, B. (2020). Competition in dual-channel supply chains: The manufacturers’ channel selection. European Journal of Operational Research, 291(1), 244–262.
Funding
Major Research Plan, 71872064, Feng Tao.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix
1.1 To save space, this section can be provided in a separate file if the paper is accepted.
For the simplicity of calculation, we define some useful expressions as follows:
\({\text{T = }}\gamma^{2} \left( {2\rho - 1 + s\left( {1 + \beta } \right)} \right)\), \({\text{L}} = 1 - \beta^{2}\), \(K = \rho + \beta - \rho \beta\),
\(G = \gamma^{2} \left( {1 - 2\rho + 3s\left( {1 + \beta } \right)} \right)\), \(F = \rho b - \rho + 1\).
Part 1: Proof of Proposition and Lemma
2.1 Proof of Proposition1
This paper uses the Stackelberg model. The manufacturer is the leader. In the manufacturer’s dual-channel model, there is no retailer, and the manufacturer’s profit is the profit of the entire supply chain.
The manufacturer’s profit function is:
The first order conditions are as follows:
Thus, equating the first order conditions to 0 and solving the equations simultaneously, we get:
Substituting the values in the expressions for the demand of each channel and supply chain profit function gives us the other results.
For \(t^{M} > 0\), \(1 - s\left( {1 - \beta } \right) > 0\) to make sure the solution is meaningful, there is an implicit condition that \({1} - {\upgamma }^{2} - {\upbeta } > 0\).
Additionally, we have.
\(\frac{{\partial^{2} \prod_{MM} }}{{\partial p_{d}^{2} }} = - 2 < 0\), \(\left[ {\begin{array}{*{20}c} {\frac{{\partial^{2} \prod_{MM} }}{{\partial p_{d}^{2} }}} & {\frac{{\partial^{2} \prod_{MM} }}{{\partial p_{d} \partial p_{n} }}} \\ {\frac{{\partial^{2} \prod_{MM} }}{{\partial p_{n} \partial p_{d} }}} & {\frac{{\partial^{2} \prod_{MM} }}{{\partial p_{n}^{2} }}} \\ \end{array} } \right] = 4 - 4\beta^{2} > 0\) and.
\(\left[ {\begin{array}{*{20}c} {\frac{{\partial^{2} \prod_{MM} }}{{\left( {\partial p_{d}^{M} } \right)^{2} }}} & {\frac{{\partial^{2} \prod_{MM} }}{{\partial p_{d} \partial p_{n} }}} & {\frac{{\partial^{2} \prod_{MM} }}{{\partial p_{d} \partial t}}} \\ {\frac{{\partial^{2} \prod_{MM} }}{{\partial p_{n} \partial p_{d} }}} & {\frac{{\partial^{2} \prod_{MM} }}{{\partial p_{n}^{2} }}} & {\frac{{\partial^{2} \prod_{MM} }}{{\partial p_{n} \partial t}}} \\ {\frac{{\partial^{2} \prod_{MM} }}{{\partial t\partial p_{d} }}} & {\frac{{\partial^{2} \prod_{MM} }}{{\partial t\partial p_{n} }}} & {\frac{{\partial^{2} \prod_{MM} }}{{\partial t^{2} }}} \\ \end{array} } \right] = 4\left( {\beta^{2} + \gamma^{2} + \beta \gamma^{2} - 1} \right)\).
Since \({1} - {\upgamma }^{2} - {\upbeta } > 0\), we know \(\beta^{2} + \gamma^{2} + \beta \gamma^{2} - 1 < \beta^{2} + 1 - \beta + \beta \left( {1 - \beta } \right) - 1 = 0\).
Thus, the third-order principal formula is less than zero, and the Hessian matrix is a negative definite matrix.
Proof of Lemma 1
First consider the impact of \(\rho\) on prices, green technology levels and profit. We take the derivative of the decision variable with respect to the parameter \(\rho\), and get the following formula:
-
(1)
\(\frac{{\partial p_{d}^{M} }}{\partial \rho } = \frac{1}{{2\left( {\beta + 1} \right)}}\), we know \(\beta + 1 > 0\), therefore \(\frac{{\partial p_{d}^{M} }}{\partial \rho } > 0\).
-
(2)
\(\frac{{\partial p_{n}^{M} }}{\partial \rho } = - \frac{1}{{2\left( {\beta + 1} \right)}}\), we know \(\beta + 1 > 0\), therefore \(\frac{{\partial p_{n}^{M} }}{\partial \rho } < 0\).
-
(3)
\( \frac{{\partial t^{M} }}{\partial \rho } = 0 \)
-
(4)
\(\frac{{\partial \Pi_{{{\text{MM}}}}^{ * } }}{\partial \rho } = \frac{{\left( {2\rho - 1} \right)}}{{2\left( {\beta + 1} \right)}} + \frac{s}{2}\), when \(\rho > \frac{{1 - s\left( {1 + \beta } \right)}}{2}\), then \(\frac{{\partial \Pi_{{{\text{MM}}}}^{ * } }}{\partial \rho } > 0\); otherwise \(\frac{{\partial \Pi_{{{\text{MM}}}}^{ * } }}{\partial \rho } < 0\).
Then consider the impact of \(\gamma\) on prices, green technology levels and profit. We take the derivative of the decision variable with respect to the parameter \(\gamma\), and get the following formula:
-
(1)
The results of the derivation of prices online and offline are the same \({ }\frac{{\partial p_{d}^{M} }}{\partial \gamma } = \frac{{\partial p_{n}^{M} }}{\partial \gamma } = \frac{{\gamma \left( {1 - s\left( {1 - \beta } \right)} \right)}}{{2\left( {{1} - {\upgamma }^{2} - {\upbeta }} \right)^{2} }}\). Since \(1 - s\left( {1 - \beta } \right) > 0\), then \(\frac{{\partial p_{d}^{M} }}{\partial \gamma } = \frac{{\partial p_{n}^{M} }}{\partial \gamma } > 0\).
-
(2)
\(\frac{{\partial t^{M} }}{\partial \gamma } = \frac{{\left( {\gamma^{2} - \beta + 1} \right)\left( {1 - s\left( {1 - \beta } \right)} \right)}}{{2\left( {{1} - {\upgamma }^{2} - {\upbeta }} \right)^{2} }}\), since \(\gamma^{2} - \beta + 1 > 0\),\(1 - s\left( {1 - \beta } \right) > 0\), then \(\frac{{\partial t^{M} }}{\partial \gamma } > 0\).
-
(3)
\(\frac{{\partial \Pi_{MM}^{ * } }}{\partial \gamma } = \frac{{\gamma \left( {1 + s\left( {\beta - 1} \right)} \right)^{2} }}{{4\left( {\beta + \gamma^{2} - 1} \right)^{2} }}\) Because the numerator and denominator are quadratic, \(\frac{{\partial \Pi_{MM}^{ * } }}{\partial \gamma } > 0\).
Proof of Proposition2
In the retailer’s dual-channel supply chain, the profit functions of manufacturers and retailers are:
Because it is a Stackelberg game led by the manufacturer, the retailer makes the decision first. The first order conditions of retailer are:
Hessian matrix is:\(H = \left[ {\begin{array}{*{20}c} { - 2} & {2\beta } \\ {2\beta } & { - 2} \\ \end{array} } \right] = 4 - 4\beta > 0\).
Equating the first order condition to 0 we get
We substitute the value of \( p_{d}\),\(p_{n}\) into the above equation and derive \(\prod_{MR}\).
The first order condition of \(\prod_{MR}\)
Equating the first order conditions to 0 and solving the equations simultaneously, we get \(w^{R} = \frac{{1 - \left( {1 - \beta } \right)s}}{{2\left( {2 - \gamma^{2} - 2\beta } \right)}}\)
Substituting the above value into the values of \(p_{d}\) and \(p_{n}\) we get
Substituting the values in the expressions for the demand of each channel and supply chain profit function gives us the other results.
For \(t^{R} > 0\)\(,\) \(1 - s\left( {1 - \beta } \right) > 0\), to make sure the solution is meaningful, there is an implicit condition that \(2 - \gamma^{2} - 2\beta > 0\).
Hessian matrix is:\(H = \left[ {\begin{array}{*{20}c} { - 2\left( {1 - \beta } \right)} & \gamma \\ \gamma & { - 1} \\ \end{array} } \right] = 2 - \gamma^{2} - 2\beta > 0\).
Proof of Lemma 2
First consider the impact of ρ on prices, green technology levels and profit.
-
(1)
\(\frac{{\partial p_{d}^{R} }}{\partial \rho } = \frac{1}{{2\left( {\beta + 1} \right)}}\) We know \(\beta + 1 > 0\), therefore \(\frac{{\partial p_{d}^{R} }}{\partial \rho } > 0\).
-
(2)
\(\frac{{\partial p_{n}^{R} }}{\partial \rho } = - \frac{1}{{2\left( {\beta + 1} \right)}}\) We know \(\beta + 1 > 0\), therefore \(\frac{{\partial p_{n}^{R} }}{\partial \rho } < 0\).
-
(3)
\(\frac{{\partial t^{R} }}{\partial \rho } = 0\)
-
(4)
\(\frac{{\partial w^{R} }}{\partial \rho } = 0\)
-
(5)
\(\frac{{\partial \Pi_{{{\text{MR}}}}^{ * } }}{\partial \rho } = 0\)
-
(6)
\(\frac{{\partial \Pi_{{{\text{RR}}}}^{ * } }}{\partial \rho } = \frac{{\left( {s\left( {1 + \beta } \right) + \left( {2\rho - 1} \right)} \right)}}{{2\left( {\beta + 1} \right)}}\). \(\rho > \frac{{1 - s\left( {1 + \beta } \right)}}{2}\), \(\frac{{\partial \Pi_{{{\text{RR}}}}^{ * } }}{\partial \rho } > 0\); otherwise, \(\frac{{\partial \Pi_{{{\text{RR}}}}^{ * } }}{\partial \rho } < 0\).
Then consider the impact of \(\gamma\) on prices, green technology levels and profit.
We know \({ }1 - s\left( {1 + \beta } \right) > 0\), and then we can get the following results:
-
(1)
\(\frac{{\partial p_{d}^{R} }}{\partial \gamma } = \frac{{3\gamma \left( {1 - s\left( {1 + \beta } \right)} \right)}}{{2\left( {2 - \gamma^{2} - 2\beta } \right)^{2} }} > 0\)
-
(2)
\(\frac{{\partial p_{n}^{R} }}{\partial \gamma } = \frac{{3\gamma \left( {1 - s\left( {1 + \beta } \right)} \right)}}{{2\left( {2 - \gamma^{2} - 2\beta } \right)^{2} }} > 0\)
-
(3)
\(\frac{{\partial t^{R} }}{\partial \gamma } = \frac{{\left( {\gamma^{2} - 2\beta + 2} \right)\left( {1 - s\left( {1 + \beta } \right)} \right)}}{{2\left( {2 - \gamma^{2} - 2\beta } \right)^{2} }} > 0\)
-
(4)
\(\frac{{\partial w^{R} }}{\partial \gamma } = \frac{{\gamma \left( {1 - s\left( {1 + \beta } \right)} \right)}}{{\left( {2 - \gamma^{2} - 2\beta } \right)^{2} }} > 0\)
-
(5)
\(\frac{{\partial \Pi_{{{\text{MR}}}}^{ * } }}{\partial \gamma } = \frac{{\gamma \left( {1 - s\left( {1 + \beta } \right)} \right)^{2} }}{{2\left( {2 - \gamma^{2} - 2\beta } \right)^{2} }} > 0\)
-
(6)
\(\frac{{\partial \Pi_{{{\text{RR}}}}^{ * } }}{\partial \gamma } = \frac{{\gamma \left( {1 - \beta } \right)\left( {1 - s\left( {1 + \beta } \right)} \right)^{2} }}{{2\left( {2 - \gamma^{2} - 2\beta } \right)^{3} }}\) For \(1 - \beta > 0\),\(2 - \gamma^{2} - 2\beta > 0\) then \(\frac{{\partial \Pi_{{{\text{RR}}}}^{ * } }}{\partial \gamma } > 0\).
Proof of Proposition 3
The profit functions for the retailer and manufacturer are:
First take the derivative of the retailer’s expression, the first order condition is
Thus the retailer's profit function is strictly concave in \(p_{n}\).Equating the first order condition to 0 we get:
We substitute the value of \({ }p_{n}\) into the above equation and derive \(\prod_{MH}\).
The first order conditions of \(\prod_{MH}\) are:
\(H = \left[ {\begin{array}{*{20}c} {2\left( {\beta^{2} - 2} \right)} & \beta & {\frac{1}{2}\gamma \left( {\beta + 2} \right)} \\ \beta & { - 1} & {\frac{1}{2}\gamma } \\ {\frac{1}{2}\gamma \left( {\beta + 2} \right)} & {\frac{1}{2}\gamma } & { - 1} \\ \end{array} } \right]\), Hessian matrix negative definite.
Equating the first order conditions to 0 and solving the equations simultaneously, we get
Substituting the values in the expressions for the demand of each channel and supply chain profit function gives us the other results.
For \(t^{H} > 0\), to make sure the solution is meaningful, there is an implicit condition that \(4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta > 0\).
Proof of Lemma 3
First consider the impact of ρ on prices, green technology levels and profit.
We know \(4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta > 0\) (see proof of proposition 3), and then we can get.
-
(1)
\(\frac{{\partial p_{d}^{H} }}{\partial \rho } = \frac{{\left( {2 - \gamma^{2} - 2\beta } \right)}}{{\left( {\beta + 1} \right)\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)}} > 0\)
-
(2)
\(\frac{{\partial p_{n}^{H} }}{\partial \rho } = \frac{{a\left( {\beta^{2} + 2\beta \gamma^{2} + 2\beta + 3\gamma^{2} - 3} \right)}}{{\left( {\beta + 1} \right)\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)}}\) Since \({\upgamma }^{2} + {\upbeta } - 1 < 0\), then \({\upgamma }^{2} < 1 - {\upbeta }\). Bring \({\upgamma }^{2} < 1 - {\upbeta }\) into \(\beta^{2} + 2\beta \gamma^{2} + 2\beta + 3\gamma^{2} - 3\) to get \(\beta^{2} + 2\beta \gamma^{2} + 2\beta + 3\gamma^{2} - 3 < - \beta^{2} + \beta < 0\) and so \(\beta^{2} + 2\beta \gamma^{2} + 2\beta + 3\gamma^{2} - 3 < 0\) Therefore \(\frac{{\partial p_{n}^{H} }}{\partial \rho } < 0\).
-
(3)
\(\frac{{\partial t^{H} }}{\partial \rho } = \frac{{\gamma \left( {1 - \beta } \right)}}{{4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta }} > 0\)
-
(4)
\(\frac{{\partial {\text{w}}^{H} }}{\partial \rho } = - \frac{{2 - \gamma^{2} - 2\beta }}{{\left( {\beta + 1} \right)\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)}} < 0\)
Then consider the impact of \(\gamma\) on prices, green technology levels and profit.
\(\frac{{\partial p_{d}^{H} }}{\partial \gamma } = \frac{{4\gamma \left( {\left( {1 + \rho + \beta - \beta \rho } \right) - s\left( {1 - \beta } \right)} \right)}}{{\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)^{2} }}\)
\(1 + \rho + \beta - \beta \rho > 1\) and \(s\left( {1 - \beta } \right) < 1\), so the conclusion will always be \(\frac{{\partial p_{d}^{H} }}{\partial \gamma } > 0\).
\(\frac{{\partial p_{n}^{H} }}{\partial \gamma } = \frac{{2\gamma \left( {3 - \beta } \right)\left( {\left( {1 + \rho + \beta - \beta \rho } \right) - s\left( {1 - \beta } \right)} \right)}}{{\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)^{2} }} > 0\)
Proof of Proposition 4
The profit expressions for manufacturers and retailers are:
First, take the derivative of the retailer’s profit, the first order condition is
Thus, the retailer's profit function is strictly concave in \(p_{d}\). Equating the first order condition to 0 we get
We substitute the value of \(p_{n}\) into the above equation and derive \(\prod_{MHH}\).
The first order condition of \(\prod_{MHH}\)
\(H = \left[ {\begin{array}{*{20}c} { - 4 + \beta^{3} } & \beta & {\frac{1}{2}\gamma \left( {\beta + 2} \right)} \\ \beta & { - 1} & {\frac{1}{2}\gamma } \\ {\frac{1}{2}\gamma \left( {\beta + 2} \right)} & {\frac{1}{2}\gamma } & { - 1} \\ \end{array} } \right]\), Hessian matrix negative definite.
Equating the first order conditions to 0 and solving the equations simultaneously, we get
Substituting the values in the expressions for the demand of each channel and supply chain profit function gives us the other results.
For \(t^{HH} > 0\), to make sure the solution is meaningful, there is an implicit condition that \(4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta > 0\).
Proof of Lemma 4
First consider the impact of ρ on prices, green technology levels and profit.
We know \(4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta > 0\),\(1 - \beta > 0\), \(2 - \gamma^{2} - 2\beta > 0\), then we can get.
-
(1)
\(\frac{{\partial p_{d}^{HH} }}{\partial \rho } = \frac{{ - \left( {\beta^{2} + 2\beta \gamma^{2} + 2\beta + 3\gamma^{2} - 3} \right)}}{{\left( {\beta + 1} \right)\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)}} > 0\), From Proposition 1 we can get \(\gamma^{2} + \beta - 1 < 0\), then \(\gamma^{2} < 1 - \beta\), Bring this formula into \(\beta^{2} + 2\beta \gamma^{2} + 2\beta + 3\gamma^{2} - 3\), then we can get \(\beta^{2} + 2\beta \gamma^{2} + 2\beta + 3\gamma^{2} - 3 < - \beta^{2} - \beta\). Since \(- \beta^{2} - \beta < 0\), then \(\beta^{2} + 2\beta \gamma^{2} + 2\beta + 3\gamma^{2} - 3 < 0\).
-
(2)
Apparently \(\frac{{\partial p_{n}^{HH} }}{\partial \rho } = \frac{{ - \left( {2 - \gamma^{2} - 2\beta } \right)}}{{\left( {\beta + 1} \right)\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)}} < 0\).
-
(3)
Apparently \(\frac{{\partial t^{HH} }}{\partial \rho } = - \frac{{\gamma \left( {1 - \beta } \right)}}{{4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta }} < 0\)
-
(4)
\( \frac{{\partial w^{HH} }}{\partial \rho } = \frac{{\left( {2 - \gamma^{2} - 2\beta } \right)}}{{\left( {\beta + 1} \right)\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)}} > 0 \)
Then consider the impact of \(\gamma\) on prices, green technology levels and profit.
Since \(\left( {2 - \rho + \rho^{2} } \right) - s\left( {2 - \rho + \rho^{2} } \right) > 0\),
then \(\left( {2 - \rho + \rho^{2} } \right) - s\left( {2 - \rho - \rho \beta } \right) > \left( {2 - \rho + \rho^{2} } \right) - s\left( {2 - \rho + \rho^{2} } \right) > 0\).
-
(1)
\( \frac{{\partial p_{d}^{HH} }}{\partial \gamma } = \frac{{2\gamma \left( {3 - \beta } \right)\left( {\left( {2 - \rho + \rho^{2} } \right) - s\left( {2 - \rho - \rho \beta } \right)} \right)}}{{\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)^{2} }} > 0 \)
-
(2)
\( \frac{{\partial p_{d}^{HH} }}{\partial \gamma } = \frac{{4\gamma \left( {\left( {2 - \rho + \rho^{2} } \right) - s\left( {2 - \rho - \rho \beta } \right)} \right)}}{{\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)^{2} }} > 0 \)
-
(3)
\(\frac{{\partial t^{HH} }}{\partial \gamma } = \frac{{\left( {\left( {\beta + 3} \right)\gamma^{2} + 4\left( {1 - \beta } \right)} \right)\left( {\left( {2 - \rho + \rho^{2} } \right) - s\left( {2 - \rho - \rho \beta } \right)} \right)}}{{\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)^{2} }}\),
Since \(\left( {\beta + 3} \right)\gamma^{2} + 4\left( {1 - \beta } \right) > 0\), then \(\frac{{\partial t^{H} }}{\partial \gamma } > 0\).
-
(4)
\(\frac{{\partial w^{HH} }}{\partial \gamma } = \frac{{4\gamma \left( {\left( {2 - \rho + \rho^{2} } \right) - s\left( {2 - \rho - \rho \beta } \right)} \right)}}{{\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)^{2} }} > 0\).
Part 2: Proof of Corollary
10.1 Proof of Corollary 1
10.1.1 Product green technology level
-
(1)
\(t^{M} - t^{R} = \frac{{\gamma \left( {1 - \beta } \right)\left( {1 - s\left( {1 - \beta } \right)} \right)}}{{2\left( {2 - \gamma^{2} - 2\beta } \right)\left( {1 - \gamma^{2} - \beta } \right)}}\), since \(1 - s\left( {1 - \beta } \right) > 0\),\(1 - \beta > 0\), then \(t^{M} > t^{R}\).
-
(2)
\(t^{H} - t^{HH} = \frac{{\gamma \left( {1 - \beta } \right)\left( {2\rho - 1 + s\left( {1 + \beta } \right)} \right)}}{{\left( {4 - 4\beta - \beta \gamma^{2} - 3\gamma^{2} } \right)}}\). When \(\rho > \frac{{1 - s\left( {1 + \beta } \right)}}{2}\), then \(t^{H} > t^{HH}\); otherwise, \(t^{H} < t^{HH}\), \(\overline{{\rho_{0} }} = \frac{{1 - s\left( {1 + \beta } \right)}}{2}\).
-
(3)
\(t^{H} - t^{R} = \frac{{2\rho \left( {2 - \gamma^{2} - 2\beta } \right) + \beta \left( {4 - \gamma^{2} s} \right) + \gamma^{2} \left( {1 - s} \right)}}{{\left( {4 - 4\beta - \beta \gamma^{2} - 3\gamma^{2} } \right)\left( {2 - \gamma^{2} - 2\beta } \right)}} > 0\), \(t^{H} > t^{R}\).
\(t^{M} - t^{HH} = \frac{{2\rho \left( {1 - \beta - \gamma^{2} } \right) + 2\left( {1 - \beta } \right)\beta s + \gamma^{2} \left( {1 - \left( {1 + \beta } \right)s} \right)}}{{\left( {1 - \beta - \gamma^{2} } \right)}} > 0\), \(t^{HH} > t^{M}\).
\(t^{H} - t^{M} = \frac{{2\rho \left( {1 - \beta - \gamma^{2} } \right) + \left( { - 2 + 2\beta + \gamma^{2} } \right) - \left( {2 - \gamma^{2} - \beta \left( {2 + \gamma^{2} } \right)} \right)s}}{{\left( {1 - \beta - \gamma^{2} } \right)\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)}}\),
\(\overline{{\rho_{1} }} = \frac{{\left( {2 - 2\beta - \gamma^{2} } \right) - \left( {2 - \gamma^{2} - \beta \left( {2 + \gamma^{2} } \right)} \right)s}}{{2a\left( {1 - \beta - \gamma^{2} } \right)}}\) if \(\rho \ge \overline{{\rho_{1} }}\), then \(t^{H} \ge t^{M}\);otherwise \(t^{H} < t^{M}\).
$$ t^{R} - t^{HH} = \frac{{2\left( {2 - 2\beta - \gamma^{2} } \right) + \left( {4 - \gamma^{2} } \right) + \left( {1 + \beta } \right)\gamma^{2} s - 4s\left( {1 - \beta^{2} } \right)}}{{\left( {2 - 2\beta - \gamma^{2} } \right)\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)}} $$\(\overline{{\rho_{2} }} = \frac{{\left( {4 - \gamma^{2} } \right) + \left( {1 + \beta } \right)\gamma^{2} s - 4s\left( {1 - \beta^{2} } \right)}}{{2a\left( {2 - 2\beta - \gamma^{2} } \right)}}\) if \(\rho \ge \overline{{\rho_{2} }}\), then \(t^{R} \ge t^{HH}\); otherwise \(t^{R} < t^{HH}\).
Proof of Corollary 2
Comparison of different channel selection on manufacturer's profit.
We know \(1 - \gamma^{2} - \beta > 0\), \(4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta > 0\).In the next three formulas, we will use these two inequalities.
Since \(1 + s\left( {1 - \beta } \right) > 0\), if \(\rho > \frac{{1 - s\left( {1 + \beta } \right)}}{2}\),\(\prod_{{{\text{MH}}}}^{ * } > \prod_{{{\text{MHH}}}}^{ * }\); if \(\rho < \frac{{1 - s\left( {1 + \beta } \right)}}{2}\),\(\prod_{{{\text{MH}}}}^{ * } < \prod_{{{\text{MHH}}}}^{ * }\).\(\overline{{\rho_{0} }} = \frac{{1 - s\left( {1 + \beta } \right)}}{2}\).
Retailer
-
(1)
\( \prod_{{{\text{RH}}}}^{ * } - \prod_{{{\text{RHH}}}}^{ * } = - \frac{{\left( {1 - \beta } \right)\left( {1 - \gamma^{2} - \beta } \right)\left( {\left( {2\rho - 1} \right) + s\left( {1 + \beta } \right)} \right)\left( {1 + s\left( {1 - \beta } \right)} \right)}}{{2\left( {4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta } \right)^{2} }} \)
when \(\rho < \overline{{\rho_{0} }}\), then \(\prod_{{{\text{RH}}}}^{ * } > \prod_{{{\text{RHH}}}}^{ * }\); when \(\rho > \overline{{\rho_{0} }}\), then \(\prod_{{{\text{RHH}}}}^{ * } > \prod_{{{\text{RH}}}}^{ * }\)
-
(2)
\( \prod_{{{\text{RR}}}}^{ * } - \prod_{{{\text{RH}}}}^{ * } = \frac{{A\rho^{2} + B\rho + C}}{{8\left( {\beta + 1} \right)\left( {\gamma^{2} + 2\beta - 2} \right)^{2} \left( {4\beta + \beta \gamma^{2} + 3\gamma^{2} - 4} \right)^{2} }} \)
-
(a)
If A > 0 and \(\Delta < 0\), then \(\prod_{{{\text{RR}}}}^{ * } > \prod_{{{\text{RH}}}}^{ * }\);
-
(b)
If A > 0 and \(\Delta > 0\), then when \(0 < \rho < \rho_{1}^{*}\), \(\prod_{{{\text{RR}}}}^{ * } > \prod_{{{\text{RH}}}}^{ * }\); when \(\rho_{1}^{*} < \rho < \rho_{2}^{*}\), \(\prod_{{{\text{RR}}}}^{ * } < \prod_{{{\text{RH}}}}^{ * }\); when \(\rho_{2}^{*} < \rho < 1\), \(\prod_{{{\text{RR}}}}^{ * } > \prod_{{{\text{RH}}}}^{ * }\).
-
(c)
If A < 0 and \(\Delta < 0\), then \(\prod_{{{\text{RR}}}}^{ * } < \prod_{{{\text{RH}}}}^{ * }\);
-
(d)
If A < 0 and \(\Delta > 0\),then when \(0 < \rho < \rho_{1}^{*}\), \(\prod_{{{\text{RR}}}}^{ * } < \prod_{{{\text{RH}}}}^{ * }\); when \(\rho_{1}^{*} < \rho < \rho_{2}^{*}\), \(\prod_{{{\text{RR}}}}^{ * } > \prod_{{{\text{RH}}}}^{ * }\); when \(\rho_{1}^{*} < \rho < 1\), \(\prod_{{{\text{RR}}}}^{ * } < \prod_{{{\text{RH}}}}^{ * }\).
Note: \(\Delta = B^{2} - 4AC\), \(\rho_{0}^{*} = \frac{{1 - s\left( {1 + \beta } \right)}}{2}\), \(\rho_{1}^{*} = \frac{{ - B - \sqrt {B^{2} - 4AC} }}{2A}\),\(\rho_{2}^{*} = \frac{{ - B + \sqrt {B^{2} - 4AC} }}{2A}\)
$$ A = 4\left( { - 2 + 2\beta + \gamma^{2} } \right)^{2} \left( {2\left( { - 7 + \beta } \right)\left( {1 - \beta } \right)^{2} + 4\left( {1 - \beta } \right)\left( {5 + \beta } \right)\gamma^{2} - \left( {7 + \beta \left( {4 + \beta } \right)} \right)\gamma^{4} } \right) $$$$ B = - 4\left( { - 2 + 2\beta + \gamma^{2} } \right)^{2} \left( \begin{gathered} \left( {12 - 18\gamma^{2} + 7\gamma^{4} } \right)\left( { - 1 + s} \right) + \beta^{3} \left( {4 + \left( {12 + 6\gamma^{2} + \gamma^{4} } \right)s} \right) \hfill \\ + \beta^{2} \left( { - 4\left( {5 + 3s} \right) + \gamma^{4} \left( { - 1 + 5s} \right) + 2\gamma^{2} \left( { - 1 + 9s} \right)} \right) \hfill \\ + \beta \left( {28 - 12s - 2\gamma^{2} \left( {8 + 3s} \right) + \gamma^{4} \left( { - 4 + 11s} \right)} \right) \hfill \\ \end{gathered} \right) $$$$ \begin{gathered} C = 16\beta \left( {12 + \beta \left( { - 5 + 2\beta } \right)\left( {4 + \left( { - 2 + \beta } \right)\beta } \right)} \right) + 8\beta \left( { - 42 + \beta \left( {44 + \beta \left( { - 22 + 5\beta } \right)} \right)} \right)\gamma^{2} \\ - \left( { - 1 + \beta } \right)\left( { - 109 + \beta \left( {77 + 3\left( { - 1 + \beta } \right)\beta } \right)} \right)\gamma^{4} + 4\left( { - 1 + \beta } \right)\left( {11 + \beta \left( {4 + \beta } \right)} \right)\gamma^{6} + \left( {7 + \beta \left( {4 + \beta } \right)} \right)\gamma^{8} \\ - 2\left( \begin{gathered} 48\beta \left( { - 3 + \beta \left( {2 + \left( { - 2 + \beta } \right)\left( { - 1 + \beta } \right)\beta } \right)} \right) + 24\left( { - 1 + \beta } \right)^{3} \left( {1 + \beta } \right)\left( {5 + \beta } \right)\gamma^{2} \\ + \left( {1 - \beta } \right)^{2} \left( {1 + \beta } \right)\left( {109 + \beta \left( {38 + 5\beta } \right)} \right)\gamma^{4} + 4\left( {\beta - 1} \right)\left( {1 + \beta } \right)\left( {11 + \beta \left( {5 + \beta } \right)} \right)\gamma^{6} \\ + \left( {1 + \beta } \right)\left( {7 + \beta \left( {4 + \beta } \right)} \right)\gamma^{8} )s \\ \end{gathered} \right) \\ - \left( \begin{gathered} 48\left( {1 - \beta } \right)^{4} \left( {1 + \beta } \right)^{2} + 24\left( { - 1 + \beta } \right)^{3} \left( {1 + \beta } \right)^{2} \left( {5 + \beta } \right)\gamma^{2} + \left( { - 1 + \beta^{2} } \right)^{2} \left( {109 + \beta \left( {44 + 3\beta } \right)} \right)\gamma^{4} \hfill \\ + 4\left( { - 1 + \beta } \right)\left( {1 + \beta } \right)^{2} \left( {11 + \beta \left( {6 + \beta } \right)} \right)\gamma^{6} + \left( {7 + 2\beta \left( {9 + \beta \left( {8 + 3\beta \left( {1 + 8\beta } \right)} \right)} \right)} \right)\gamma^{8} )s^{2} \hfill \\ \end{gathered} \right) \\ + 24\left( {5\gamma^{2} + 4s} \right) \\ \end{gathered} $$ -
(3)
\( \prod_{{{\text{RR}}}}^{ * } - \prod_{{{\text{RHH}}}}^{ * } = \frac{{A_{1} \rho^{2} + B_{1} \rho + C_{1} }}{{8\left( {\beta + 1} \right)\left( {\gamma^{2} + 2\beta - 2} \right)^{2} \left( {4\beta + \beta \gamma^{2} + 3\gamma^{2} - 4} \right)^{2} }} \)
-
(a)
If A1 > 0 and \(\Delta_{1} < 0\), then \(\prod_{{{\text{RR}}}}^{ * } > \prod_{{{\text{RHH}}}}^{ * }\);
-
(b)
If A1 > 0 and \(\Delta_{1} > 0\), then when \(0 < \rho < \rho_{3}^{*}\), \(\prod_{{{\text{RR}}}}^{ * } > \prod_{{{\text{RHH}}}}^{ * }\); when \(\rho_{3}^{*} < \rho < \rho_{4}^{*}\), \(\prod_{{{\text{RR}}}}^{ * } < \prod_{{{\text{RHH}}}}^{ * }\); when \(\rho_{4}^{*} < \rho < 1\), \(\prod_{{{\text{RR}}}}^{ * } > \prod_{{{\text{RHH}}}}^{ * }\).
-
(c)
If A1 < 0 and \(\Delta < 0\), then \(\prod_{{{\text{RR}}}}^{ * } < \prod_{{{\text{RHH}}}}^{ * }\);
-
(d)
If A1 < 0 and \(\Delta > 0\),then when \(0 < \rho < \rho_{3}^{*}\), \(\prod_{{{\text{RR}}}}^{ * } < \prod_{{{\text{RHH}}}}^{ * }\); when \(\rho_{3}^{*} < \rho < \rho_{4}^{*}\), \(\prod_{{{\text{RR}}}}^{ * } > \prod_{{{\text{RHH}}}}^{ * }\); when \(\rho_{4}^{*} < \rho < 1\), \(\prod_{{{\text{RR}}}}^{ * } < \prod_{{{\text{RHH}}}}^{ * }\).
Note: \(\Delta_{1} = B_{1}^{2} - 4A_{1} C_{1}\), \(\rho_{3}^{*} = \frac{{ - B_{1} - \sqrt {B_{1}^{2} - 4A_{1} C_{1} } }}{{2A_{1} }}\),\(\rho_{4}^{*} = \frac{{ - B_{1} + \sqrt {B_{1}^{2} - 4A_{1} C_{1} } }}{{2A_{1} }}\).
\(A_{1} = - 4\left( \begin{gathered} 6 - 8\beta \left( {29 + \beta \left( { - 46 + \beta \left( {34 + \left( { - 11 + \beta } \right)\beta } \right)} \right)} \right) + 8\left( { - 1 + \beta } \right)^{3} \left( {17 + \beta } \right)\gamma^{2} \hfill \\ + 2\left( { - 1 + \beta } \right)^{2} \left( {61 + \beta \left( {15 + 2\beta } \right)} \right)\gamma^{4} + 4\left( { - 1 + \beta } \right)\left( {12 + \beta \left( {5 + \beta } \right)} \right)\gamma^{6} \hfill\\ + \left( {7 + \beta \left( {4 + \beta } \right)} \right)\gamma^{8} \hfill \\ \end{gathered} \right)\) \(B_{1} = 4\left( { - 2 + 2\beta + \gamma^{2} } \right)^{2} \left( \begin{gathered} - \left( { - 2 + \gamma^{2} } \right)\left( { - 8 + 7\gamma^{2} } \right)\left( { - 1 + s} \right) + 4\beta^{4} s\hfill\\ - \beta^{3} \left( {20 + 2\gamma^{2} + \gamma^{4} } \right)s \hfill \\ + \beta^{2} \left( {\gamma^{2} \left( {6 - 22s} \right) + \gamma^{4} \left( {1 - 5s} \right) + 4\left( {4 + 3s} \right)} \right) \hfill\\ + \beta \left( \begin{gathered} \gamma^{4} \left( {4 - 11s} \right) \hfill \\ + 2\gamma^{2} \left( {8 + s} \right) + 4\left( { - 8 + 5s} \right) \hfill \\ \end{gathered} \right) \hfill \\ \end{gathered} \right)\) \(\begin{gathered} C_{1} = - 16\beta \left( { - 18 + \beta \left( {24 + \beta \left( { - 14 + 3\beta } \right)} \right)} \right) - 8\beta \left( {58 + \beta \left( { - 44 + 3\beta \left( {2 + \beta } \right)} \right)} \right)\gamma^{2} \hfill \\ + \left( {1 - \beta } \right)\left( { - 149 + \beta \left( {77 + \beta \left( {37 + 3\beta } \right)} \right)} \right)\gamma^{4} + 4\left( {1 - \beta } \right)\left( {13 + \beta \left( {6 + \beta } \right)} \right)\gamma^{6}\hfill \\ + \left( {7 + \beta \left( {4 + \beta } \right)} \right)\gamma^{8} - \left( {1 + \beta } \right)\left( {7 + \beta \left( {4 + \beta } \right)} \right)\gamma^{8} s\left( {2 + s + \beta s} \right) \hfill \\ + 16s\left( \begin{gathered} 10 - 10\beta \left( { - 3 + \beta \left( {2 + \left( { - 2 + \beta } \right)\left( { - 1 + \beta } \right)\beta } \right)} \right) \hfill \\ \left( { - 1 + \beta } \right)^{4} \left( {1 + \beta } \right)^{2} \left( { - 5 + 2\beta } \right)s \hfill \\ \end{gathered} \right) \hfill \\ + 4\left( {1 - \beta^{2} } \right)\gamma^{6} s\left( {26 + 2\beta \left( {5 + \beta } \right) + \left( {1 + \beta } \right)\left( {13 + \beta \left( {4 + \beta } \right)} \right)s} \right) \hfill \\ - 8\gamma^{2} \left( { - 23 + \left( { - 1 + \beta } \right)^{3} \left( {1 + \beta } \right)s\left( {23\left( {2 + s} \right) + \beta \left( {6 + \left( {18 - 5\beta } \right)s} \right)} \right)} \right) \hfill \\ - \left( { - 1 + \beta } \right)^{2} \left( {1 + \beta } \right)\gamma^{4} s\left( {149\left( {2 + s} \right) + \beta \left( {76 + 153s + \beta \left( {10 + \left( {7 + 3\beta } \right)s} \right)} \right)} \right) \hfill \\ \end{gathered}\)
-
(a)
Proof of Corollary 3
-
(1)
(1) \(t_{e}^{M} - t_{e}^{R} = \frac{{\gamma \left( {2\varphi - 1} \right)\left( {1 - \beta } \right)\left( {1 + \left( {1 - \beta } \right)} \right)}}{{2\left( {2\varphi - \gamma^{2} - 2\beta \varphi } \right)\left( {{1} - {\upgamma }^{2} - {\upbeta }} \right)}}\) If \(\varphi < \frac{1}{2}\), \(t_{e}^{M} < t_{e}^{R}\); If \(\varphi > \frac{1}{2}\),\(t_{e}^{M} > t_{e}^{R}\).
-
(2)
(2) \(t_{e}^{H} - t_{e}^{HH} = - \frac{{\gamma \left( {1 - \beta } \right)\left( {\left( {1 - 2\rho } \right) - s\left( {1 + \beta } \right)} \right)}}{{4 - 3\gamma^{2} - \beta \gamma^{2} - 4\beta }}\), if \(\rho < \overline{{\rho_{0} }}\), \(t_{e}^{HH} > t_{e}^{H}\); if \(\rho > \overline{{\rho_{0} }}\), \(t_{e}^{H} < t_{e}^{HH}\).
Rights and permissions
About this article
Cite this article
Tao, F., Zhou, Y., Bian, J. et al. Optimal channel structure for a green supply chain with consumer green-awareness demand. Ann Oper Res 324, 601–628 (2023). https://doi.org/10.1007/s10479-022-04665-9
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10479-022-04665-9