Abstract
The increased environmental awareness of consumers has led supply chains (SC) to green their operations. To extract a higher portion from the expanded demand due to greening activities, SC parties may hide key information regarding their green activities. This paper investigates the channel coordination problem in a green SC consisting of a manufacturer who sells a green product through a retailer. Both parties may involve in greening operations to expand an environmental-aware market; however, the retailer is privy to the information about his green sales effort. The analysis of the first-best outcome characterizes the conditions for (i) hold-up problem under which the retailer benefits from free ride on the manufacturer's greening operations effort, (ii) commitment strategy from the retailer to cover for the market expansion due to the manufacturer’s underinvestment in greening operations, and (iii) synergy in greening efforts. We then solve for the optimal incentive contracts under asymmetric information. Our analysis suggests that the manufacturer can include her greening effort in the contract to work as an incentive-fee; the higher level of greening effort by the manufacturer incentivizes the retailer to increase his green sales effort. We also show that the wholesale price term works as a screening tool to avoid the low efficient retailer from mimicking the high efficient one. Finally, we show that information asymmetry reduces the social welfare in a green market; it leads to a higher market price and a lower greening effort level.





Similar content being viewed by others
Notes
Refer to https://learn.g2.com/green-marketing.
Similar to Swami & Shah (2013), the market demand is negatively related to the retail price but positively related to the greening efforts of both the retailer and the manufacturer.
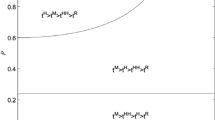
If the retailer claims his type as \(H\) then he has to pick contract \(H:\left( {w_{H} ,\tau_{mH} } \right)\) and if he claims his type as \(L \) then he has to pick contract \(L:\left( {w_{L} ,\tau_{mL} } \right)\). However, the retailer can make a false claim and therefore it is necessary to consider appropriate feature in the model to prevent the retailer to make false claims.
Previous works have considered social welfare as the function of the consumer welfare and the environmental impacts (Choi and Luo, 2019). Hence, any activity that can enhance these two factors contributes to the increase of the social welfare.
References
Akan, M., Ata, B., & Lariviere, M. A. (2011). Asymmetric information and economies of scale in service contracting. Manufacturing and Service Operations Management, 13(1), 58–72.
Babich, V., Li, H., Ritchken, P., & Wang, Y. (2012). Contracting with asymmetric demand information in supply chains. European Journal of Operational Research, 217(2), 333–341.
Basiri, Z., & Heydari, J. (2017). A mathematical model for green supply chain coordination with substitutable products. Journal of Cleaner Production, 145, 232–249.
Burnetas, A., Gilbert, S. M., & Smith, C. (2005). Quantity discounts in single period supply contracts with asymmetric demand information. IIE Transactions, 39(5), 465–479.
Cachon, G. P. (2003). Supply Chain Management: Design, Coordination and Operation. International Journal of Production Economics, 11, 227–339.
Cachon, G. P., & Zhang, F. (2006). Procuring fast delivery: Sole sourcing with information asymmetry. Management Science, 52(6), 881–896.
Cakanyıldırım, M., Feng, Q., Gan, X., & Sethi, S. P. (2012). Contracting and coordination under asymmetric production cost information. Production and Operations Management, 21(2), 345–360.
Cao, E., Ma, Y., Wan, C., & Lai, M. (2013). Contracting with asymmetric cost information in a dual-channel supply chain. Operations Research Letters, 41(4), 410–414.
Chen, W., & Li, L. (2020). Incentive contracts for green building production with asymmetric information. International Journal of Production Research. https://doi.org/10.1080/00207543.2020.1727047
Chiu, C., Choi, T. M., Hao, G., & Li, X. (2015). Innovative menu of contracts for coordinating a supply chain with multiple mean-variance retailers. European Journal of Operational Research, 246, 815–826.
Choi, T. M. (2013). Local sourcing and fashion quick response system: The impacts of carbon footprint tax. Transportation Research Part e: Logistics and Transportation Review, 55(1), 43–54.
Choi, T. M. (2019). Data quality challenges for sustainable fashion supply chain operations in emerging markets: Roles of blockchain, government sponsors and environment taxes. Transportation Research Part e: Logistics and Transportation Review, 133, 135–152.
Corbett, C. J., & de Groote, X. (2000). A supplier’s optimal quantity discount policy under asymmetric information. Management Science, 46(3), 444–450.
Corbett, C. J., Zhou, D., & Tang, C. S. (2004). Designing Supply Contracts : Contract type and information asymmetry. Management Science, 50(4), 550–559.
Fang, X., Ru, J., & Wang, Y. (2014). Optimal procurement design of an assembly supply chain with information asymmetry. Production and Operations Management, 23(12), 2075–2088.
Feng, Q., Lai, G., & Lu, L. X. (2015). Dynamic bargaining in a supply chain with asymmetric demand information. Management Science, 61(2), 301–315.
Gan, X., Sethi, S. P., & Yan, H. (2004). Coordination of supply chains with risk-averse agents. Production and Operations Management, 13(2), 135–149.
Gan, X., Sethi, S. P., & Yan, H. (2009). Channel coordination with a risk-neutral supplier and a downside-risk-averse retailer. Production and Operations Management, 14(1), 80–89.
Giovanni, P. D. (2016). Closed-loop supply chain coordination through incentives with asymmetric information. Annals of Operations Research, 253(1), 133–167.
Gümüş, M. (2014). With or without forecast sharing: Competition and credibility under information asymmetry. Production and Operations Management, 23(10), 1732–1747.
Guo, S., Shen, B., Choi, T. M., & Jung, S. (2017). A review on supply chain contracts in reverse logistics: Supply chain structures and channel leaderships. Journal of Cleaner Production, 144, 387–402.
Ha, A. Y. (2001). Supplier-buyer contracting: Asymmetric cost information and cutoff level policy for buyer participation. Naval Research Logistics, 48(1), 41–64.
Heydari, J., Chaharsooghi, S. K., & Alipour, L. (2009). Animation supply chain modelling and diagnosis: A case study in animation industry of Iran. International Journal of Business Performance and Supply Chain Modelling, 1(4), 319–332.
Hong, Z., & Guo, X. (2019). Green product supply chain contracts considering environmental responsibilities. Omega, 83, 155–166.
Hosseini, M. M. (2020). A decision support contract for cost-quality trade-off in projects under information asymmetry. International Journal of Business and Management, 15, 4.
Hosseini-Motlagh, S. M., Nouri-Harzvili, M., Choi, T. M., & Ebrahimi, S. (2019). Reverse supply chain systems optimization with dual channel and demand disruptions: Sustainability, CSR investment and pricing coordination. Information Sciences, 503, 606–634.
Kautish, P. (2016). Volkswagen AG: Defeat device or device defeat? IMT Case Journal, 7, 19–30.
Kerkkamp, R. B. O., Heuvel, W. V. D., & Wagelmans, A. P. M. (2018). Two-echelon supply chain coordination under information asymmetry with multiple types. Omega, 76, 137–159.
Ketokivi, M., & Choi, T. (2014). Renaissance of case research as a scientific method. Journal of Operations Management, 32(5), 232–240.
Kim, D., & Kim, S. (2017). Sustainable supply chain based on news articles and sustainability reports: Text mining with Leximancer and DICTION. Sustainability, 9(6), 1008.
Kostamis, D., & Duenyas, I. (2011). Purchasing under asymmetric demand and cost information: When is more private information better? Operations Research, 59(4), 914–928.
Laffont, J.-J., & Martimort, D. (2009). The theory of incentives: The principal-agent model. Princeton University Press.
Li, G., Li, L., Choi, T. M., & Sethi, S. P. (2020a). Green supply chain management in Chinese firms: Innovative measures and the moderating role of quick response technology. Journal of Operations Management, 66(7–8), 958–988.
Li, G., Lim, M. K., & Wang, Z. (2020b). Stakeholders, green manufacturing, and practice performance: Empirical evidence from Chinese fashion businesses. Annals of Operations Research, 290(1), 961–982.
Li, G., Liu, M., Bian, Y., & Sethi, S. P. (2020c). Guarding against Disruption Risk by contracting under information asymmetry. Decision Sciences, 51(6), 1521–1559.
Li, G., Zheng, H., Sethi, S. P., & Guan, X. (2020d). Inducing downstream information sharing via manufacturer information acquisition and retailer subsidy. Decision Sciences, 51(3), 691–719.
Li, H., & Cao, E. (2021). Competitive crowdfunding under asymmetric quality information. Annals of Operations Research. https://doi.org/10.1007/s10479-021-03939-y
Li, Y., Xu, X., Zhao, X., Yeung, J. H. Y., & Ye, F. (2012). Supply chain coordination with controllable lead time and asymmetric information. European Journal of Operational Research, 217(1), 108–119.
Liu, A., Luo, S., Mou, J., & Qiu, H. (2021). The antagonism and cohesion of the upstream supply chain under information asymmetry. Annals of Operations Research. https://doi.org/10.1007/s10479-020-03881-5
Liu, H., Sun, S., Lei, M., Leong, G. K., & Deng, H. (2016). Research on cost information sharing and channel choice in a dual-channel supply Chain. Mathematical Problems in Engineering, 2016, 4368326.
Liu, Y., Li, J., Quan, B., & Yang, J. (2019). Decision analysis and coordination of two-stage supply chain considering cost information asymmetry of corporate social responsibility. Journal of Cleaner Production, 228, 1073–1087.
Ma, P., Shang, J., & Wang, H. (2017). Enhancing corporate social responsibility: Contract design under information asymmetry. Omega, 67, 19–30.
Ma, X., Wang, S., Islam, S. M. N., & Liu, X. (2018). Coordinating a three-echelon fresh agricultural products supply chain considering freshness-keeping effort with asymmetric information. Applied Mathematical Modelling, 67, 337–356.
Mavlanova, T., Benbunan-Fich, R., & Koufaris, M. (2012). Signaling theory and information asymmetry in online commerce. Information and Management, 49(5), 240–247.
Mobini, Z., van den Heuvel, W., & Wagelmans, A. (2019). Designing multi-period supply contracts in a two-echelon supply chain with asymmetric information. European Journal of Operational Research, 277(2), 542–560.
Myerson, R. B. (1982). Optimal coordination mechanisms in generalized principal–agent problems. Journal of Mathematical Economics, 10(1), 67–81.
Nazerzadeh, H., & Perakis, G. (2016). Technical note: Nonlinear pricing competition with private capacity information. Operations Research, 64(2), 329–340.
Nikoofal, M. E., & Gümüş, M. (2020). Value of audit for supply chains with hidden action and information. European Journal of Operational Research, 285(3), 902–915.
Rahbar, E., & Wahid, N. A. (2011). Investigation of green marketing tools’ effect on consumers’ purchase behavior. Business Strategy Series, 12, 73–83.
Raza, S. A., & Govindaluri, S. M. (2019). Greening and price differentiation coordination in a supply chain with partial demand information and cannibalization. Journal of Cleaner Production, 229, 706–726.
Sane-Zerang, E., Razmi, J., & Taleizadeh, A. A. (2019). Coordination in a closed-loop supply chain under asymmetric and symmetric information with sales effort-dependent demand. Journal of Business Economics, 90, 303–334.
Savaskan, R. C., & Van Wassenhove, L. N. (2006). Reverse channel design: The case of competing retailers. Management Science, 52(1), 1–14.
Shen, B., Choi, T. M., & Minner, S. (2018). A review on supply chain contracting with information considerations: Information updating and information asymmetry. International Journal of Production Research, 47(21), 6145–6158.
Shen, B., Choi, T. M., & Minner, S. (2019). A review on supply chain contracting with information considerations: Information updating and information asymmetry. International Journal of Production Research, 57(15–16), 4898–4936.
Swami, S., & Shah, J. (2013). Channel coordination in green supply chain management. Journal of the Operational Research Society, 64(3), 336–351.
Vosooghidizaji, M., Taghipour, A., & Canel-Depitre, B. (2019). Supply chain coordination under information asymmetry: A review. International Journal of Production Research, 58(6), 1805–1834.
Wang, K., Zhao, R., & Chen, H. (2018). Optimal credit period and green consumption policies with cash-credit payments under asymmetric information. Journal of Cleaner Production, 205, 706–720.
Wang, Q., & He, L. (2018). Incentive strategies for low-carbon supply chains with asymmetric information of carbon reduction efficiency. International Journal of Environmental Research and Public Health, 15(12), 2736.
Yang, D., Chen, Z., & Nie, P. (2016). Output subsidy of renewable energy power industry under asymmetric information. Energy, 117, 291–299.
Yang, D., Xiao, T., Choi, T. M., & Cheng, T. (2018a). Optimal reservation pricing strategy for a fashion supply chain with forecast update and asymmetric cost information. International Journal of Production Research, 56(5), 1960–1981.
Yang, R., Tang, W., Dai, R., & Zhang, J. (2018b). Contract design in reverse recycling supply chain with waste cooking oil under asymmetric cost information. Journal of Cleaner Production, 201, 61–77.
Yoon, J., Talluri, S., & Rosales, C. (2019). Procurement decisions and information sharing under multi-tier disruption risk in a supply chain. International Journal of Production Research, 58(7), 3263–3283.
Yuan, B., Gu, B., Guo, J., Xia, L., & Xu, C. (2018). The optimal decisions for a sustainable supply chain with carbon information asymmetry under. Sustainability, 10(4), 1002.
Zhang, T., Choi, T. M., & Zhu, X. (2018). Optimal green product’s pricing and level of sustainability in supply chains: Effects of information and coordination. Annals of Operations Research. https://doi.org/10.1007/s10479-018-3084-8
Zhao, Y., Choi, T. M., Cheng, T. C. E., & Wang, S. (2014). Mean-risk analysis of wholesale price contracts with stochastic price-dependent demand. Annals of Operations Research., 257(1–2), 491–518.
Acknowledgements
The authors sincerely thank the Editors for their encouragements and two anonymous referees for all their invaluable comments and suggestions that have helped us significantly improve the paper.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix
Appendix
1.1 Proof of Proposition 1
The total channel profit under centralized setting would be:
Due to the concavity of the objective function (see Swami & Shah, 2013), the first order conditions result in the following optimal solutions:
1.2 Proof of Lemma 1
The profit of the retailer is:
Due to the concavity of the above objective function, the first best conditions for \( \tau_{r}^{{\text{d}}}\) and \(p^{{\text{d}}}\) yield:
Proof of Proposition 2. Applying backward induction, we first solve the retailer’s profit considering the manufacturer’s decisions are known. i.e.:
So we have:
Now we solve the manufacturer’s optimal response function. Plugging the found solutions into \(\Pi_{m}\), we have:
Applying the first-best conditions for \(\Pi_{m}\), we obtain the optimal values of \(w\) and \(\tau_{m}\):
Inserting the extracted equilibriums into equations (A.10) and (A.11) we get:
Proof of Corollary 1. To study the impact of \(\beta_{r}\) and \(\beta_{m}\) on the manufacturer’s greening effort, we need to find \(\partial \tau_{m\theta } /\partial \beta_{r}\) and \(\partial \tau_{m\theta } /\partial \beta_{m}\), respectively;
Note that, from Eq. (A.17), we have \(\partial \tau_{m\theta } /\partial \beta_{r} < 0\). However, from Eq. (A.18), one can verify that \(\partial \tau_{m\theta } /\partial \beta_{m} \ge 0\) if \(\alpha_{r}^{2} \ge 4\beta_{r}\), or \(\alpha_{r} \ge 2\sqrt {\beta_{r} }\).
Similarly, we need to find \(\partial \tau_{r\theta } /\partial \beta_{m}\) and \(\partial \tau_{r\theta } /\partial \beta_{r}\), respectively;
Note that, therefore, from Eq. (A.19), we have \(\partial \tau_{m\theta } /\partial \beta_{r} < 0\). However, from Eq. (A.20), one can verify that \(\partial \tau_{r\theta } /\partial \beta_{r} \ge 0\) if \(\alpha_{m}^{2} \ge 8\beta_{m}\), or \(\alpha_{m} \ge 2\sqrt {2\beta_{m} }\).
Proof of Proposition 3. The problem can be written as follows:
s.t.
For the sake of simplicity and based on \(\left( {p_{HH}^{*} } \right) = argmax\Pi_{rHH}\) and \(\left( {p_{LL}^{*} } \right) = argmax\Pi_{rLL}\), we have:
Thus, computing the first order derivative over market price and setting it equal to zero, we derive:
Similarly, we define:
So, we have:
Plugging \(p_{HH}\), \(p_{HL}\), \(p_{LL}\), \(p_{LH}\) into \(\Pi_{rHH}\), \(\Pi_{rHL}\), \(\Pi_{rLL}\), \(\Pi_{rLH}\), \(\Pi_{mH}\) and \(\Pi_{mL}\), we derive the retailer and the manufacturer’s profit functions:
Let \(\lambda 1\), \(\lambda 2\), \(\lambda 3\), \(\lambda 4\) denote the Lagrange multiplier of the participation constraints and the incentive compatibility constraints and \(l\) represent the Lagrange function, we have:
We first set all the Lagrange multipliers to zero and derive the optimal solutions from first-order partial derivatives of the first fourth equations of the KKT condition (obtain optimal solutions). Then we check the other KKT conditions to see whether the obtained solution is feasible. Thus, we have:
The KKT condition will be:
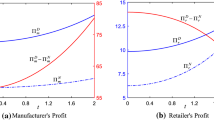
As mentioned earlier, because of the complexity of the model, closed-forms are derived where we fix some parameters to specific values. Hence, the parameters that we listed in the numerical illustration section are fixed firstly and then, we checked the KKT condition. In doing so, the values in Table
8 are obtained:
Since we set all the Lagrange multipliers to zero, all the constraints must be positive or zero (\(M_{f} \ge 0\)). However, the first constraint is negative, so the results are infeasible. For the second round, we add the first constraint to the Lagrange function. Thus we have:
Applying KKT conditions we can obtain values illustrated in Table
9.
The obtained results apply in KKT conditions; thus, they are both optimal and feasible. In fact, with this method, since the complexity of the proposed model makes it hard or impossible to check all the KKT conditions, we search for the feasible solution in the optimal area.
Finally, from the KKT conditions with given Lagrange multipliers (\(\lambda_{1} = 0.017,\lambda_{2} = \lambda_{3} = \lambda_{4} = 0\)), we can obtain the closed-form expressions:
By setting the first order derivatives of Lagrange function \(l\) and using the abbreviation Table 3, the optimal solutions can be achieved as:
Finally, by plugging the found solutions into Eq. (A.27) and Eq. (A.28), we can obtain
Proof of Lemma 2. Given \(\left( {w_{\theta } ,\tau_{m\theta } } \right)\), the profit of the \(H\)-type retailer would be:
In a similar way, given \(\left( {w_{\theta } ,\tau_{m\theta } } \right)\), the profit of the \(L\)-type retailer would be:
The retailer is rational, so he exerts high green sales effort if it leads to more profit. By comparing his profit under both \(H\) and \(L\) conditions, we obtain:
Rights and permissions
About this article
Cite this article
Ranjbar, A., Heydari, J., Madani Hosseini, M. et al. Green channel coordination under asymmetric information. Ann Oper Res 329, 1049–1082 (2023). https://doi.org/10.1007/s10479-021-04284-w
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10479-021-04284-w