To share or not to share? When information sharing meets remanufacturing

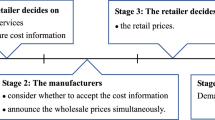
Abstract

Traditional research shows that a retailer suffers a loss due to information sharing with a manufacturer in a supply chain. However, when the manufacturer produces both new and remanufactured products, the retailer’s trade-offs change. This paper investigates whether the retailer can share demand information with the manufacturer if they can add remanufactured products to the product line. We reveal that the retailer can benefit from information sharing when the manufacturer may remanufacture. On the one hand, if the retailer shares information, the manufacturer utilizes information to strategically adjust wholesale prices, resulting in a reduction in the retailer’s profit. On the other hand, if the retailer does not share the information, the manufacturer abandons remanufacturing, leading to a lower profit for the retailer. Therefore, when the former loss is less than the latter, the retailer is willing to share information with the manufacturer. Furthermore, we find that remanufacturing and information sharing can achieve a triple-win situation for the retailer, the manufacturer and the environment.

Appendices

Appendix A: Proofs

For ease of exposition, we define

$$ \begin{aligned} \pi _{M}^{{NR1}} = & \frac{{\left( {3 - 2{\mkern 1mu} c} \right)^{2} }}{{32}} - F,\pi _{R}^{{NR1}} = \frac{{12c^{2} - 36c + {\text{372}}}}{{192}}, \\ \pi _{M}^{{NR2}} = & \frac{{4\left( {c - x - 2} \right)\left( {b - 1} \right)\varepsilon + \left( {4cx - 4x + 1} \right)b + 4c^{2} + 4x^{2} - 8c + 2}}{{8\left( {b + 3} \right)\left( {1 - b} \right)}} - F\pi _{R}^{{NR2}} \\ = & \frac{{8\varepsilon \left( {c - x - 2\varepsilon } \right)\left( {b - 1} \right) + \left( {2x - 1} \right)\left( {2c - 1} \right)\left( {b + 1} \right) + \left( {2c - 1} \right)^{2} + \left( {2x - 1} \right)^{2} }}{{16\left( {b + 3} \right)\left( {1 - b} \right)}} \\ \pi _{M}^{{IR1}} = & \frac{{3c^{2} - 9c + 7}}{{24}} - F,\pi _{R}^{{IR1}} = \frac{{3c^{2} - 9c + {\text{7}}}}{{48}}, \\ \pi _{M}^{{IR2}} = & \frac{{\varepsilon \left( {1 + \varepsilon {\text{ + }}x - c} \right)\left( {1 - b} \right) + \left( {1 - c} \right)\left( {1 - x + bx + c} \right) + x^{2} }}{{2\left( {b + 3} \right)\left( {1 - b} \right)}} - F \\ \pi _{R}^{{IR2}} = & \frac{{\varepsilon \left( {1 + \varepsilon {\text{ + }}x - c} \right)\left( {1 - b} \right) + \left( {1 - c} \right)\left( {1 - x + bx + c} \right) + x^{2} }}{{4\left( {b + 3} \right)\left( {1 - b} \right)}}, \\ \tau _{0} = & \frac{{9b - 12x + 3 + 2\sqrt {6 - 3b^{2} - 3b} }}{{6\left( {1 + b} \right)}}, \quad \tau _{1} = \frac{{3b - 4x + 1}}{{2\left( {b + 1} \right)}}. \\ \end{aligned} $$

Proof of Proposition 1 First, we solve the equilibrium solution of remanufacturing or no remanufacturing without information sharing.

No remanufacturing Given that R does not share demand information, if M does not remanufacture, the profit function of R is

$$ \pi _{R}^{{NN}} = \left( {1 + \varepsilon - q_{n}^{{NN}} - w_{n}^{{NN}} } \right)q_{n}^{{NN}} . $$

Given the wholesale price $w_{n}$, R’s optimal order quantity is

$$ q_{n}^{{NN}} (w_{n}^{{NN}} ) = \frac{1}{2}\left( {1 + \varepsilon - w_{n}^{{NN}} } \right). $$

Without demand information, M uses expected value ${\text{E}}\left[ \varepsilon \right]$ to anticipate R’s optimal order quantity. Thus, the problem of M under no information sharing and no remanufacturing is

$$ E\left[ {\pi _{M}^{{NN}} } \right] = \left( {w_{n}^{{NN}} - c} \right)\left( {\frac{{1 + {\text{E}}\left[ \varepsilon \right] - w_{n}^{{NN}} }}{2}} \right). $$

As $\varepsilon \sim U\left[ {0,1} \right]$, we have ${\text{E}}\left[ \varepsilon \right] = {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}$. The optimal wholesale price of the new product is

$$ w_{n}^{{NN}} = \frac{1}{4}\left( {3 + 2c} \right). $$

By substituting the optimal wholesale price into R’s order quantity response function, we write the optimal ex post order quantity as

$$q_{n}^{{NN}} = \frac{1}{8}\left( {1 + 4\varepsilon - 2c} \right).$$

Let $c \le {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}$ to guarantee $q_{n}^{{NN}} \ge 0$. The ex post optimal profits are $\pi _{M}^{{NN}} = 0$ and $\pi _{R}^{{NN}} = 0$, and the ex ante profits of M and R are

$$ \Pi _{M}^{{NN}} = \int_{0}^{1} {\frac{{\left( {3 - 2c} \right)\left( {1 + 4\varepsilon - 2c} \right)}}{{32}}d\varepsilon = } \frac{{\left( {3 - 2{\mkern 1mu} c} \right)^{2} }}{{32}}, $$

$$ \Pi _{R}^{{NN}} = \int_{0}^{1} {\frac{{\left( {1 + 4\varepsilon - 2c} \right)^{2} }}{{64}}d\varepsilon = } \frac{{12c^{2} - 36c + {\text{372}}}}{{192}}. $$

Remanufacturing Given that R does not share demand information, if M remanufactures, the profit function of R is

$$ \pi _{R}^{{NR}} = \left( {1 + \varepsilon - q_{n}^{{NR}} - bq_{r}^{{NR}} - w_{n}^{{NR}} } \right)q_{n}^{{NR}} + \left( {x + \varepsilon - q_{r}^{{NR}} - q_{n}^{{NR}} - w_{r}^{{NR}} } \right)q_{r}^{{NR}} . $$

Given the wholesale price $w_{n}$ of the new product and the wholesale price $w_{r}$ of the remanufactured product, R’s optimal order quantities of each product are

$$ q_{n}^{{NR}} (w_{n}^{{NR}} ,w_{r}^{{NR}} ) = \frac{{\left( {1 - b} \right)\varepsilon - \left( {1 + b} \right)\left( {x - w_{r}^{{NR}} } \right) + 2\left( {1 - w_{n}^{{NR}} } \right)}}{{\left( {3 + b} \right)\left( {1 - b} \right)}}, $$

$$ q_{r}^{{NR}} (w_{n}^{{NR}} ,\quad w_{r}^{{NR}} ) = \frac{{\left( {1 - b} \right)\varepsilon - \left( {1 + b} \right)\left( {1 - w_{n}^{{NR}} } \right) + 2\left( {x - w_{r}^{{NR}} } \right)}}{{\left( {3 + b} \right)\left( {1 - b} \right)}}. $$

Without demand information, M uses expected demand value ${\text{E(}}\varepsilon )$ to anticipate R’s optimal order quantities of each product. Hence, the problem of M can be written as

$$ \begin{aligned} E\left[ {\pi _{M}^{{NR}} } \right] = \left( {w_{n}^{{NR}} - c} \right)\left( {\frac{{\left( {1 - b} \right){\text{E}}\left[ \varepsilon \right] - \left( {1 + b} \right)\left( {x - w_{r}^{{NR}} } \right) + 2\left( {1 - w_{n}^{{NR}} } \right)}}{{\left( {3 + b} \right)\left( {1 - b} \right)}}} \right) \hfill \\ \quad + w_{r}^{{NR}} \left( {\frac{{\left( {1 - b} \right){\text{E}}\left[ \varepsilon \right] - \left( {1 + b} \right)\left( {1 - w_{n}^{{NR}} } \right) - 2\left( {x - w_{r}^{{NR}} } \right)}}{{\left( {3 + b} \right)\left( {1 - b} \right)}}} \right) - F. \hfill \\ \end{aligned} $$

The optimal wholesale prices of the new product and remanufactured product are

$$ w_{n}^{{NR}} = \frac{{3 + 2c}}{4},w_{r}^{{NR}} = \frac{{1 + 2x}}{4}. $$

By substituting the wholesale prices into R’s order quantity response functions, we obtain the optimal ex post optimal order quantities of each product as

$$q_{n}^{{NR}} = \frac{{\left( {2x + 4{\mkern 1mu} \varepsilon - 1} \right)\left( {1 - b} \right) + 4x\left( {1 - x - c} \right)}}{{4\left( {3 + b} \right)\left( {1 - b} \right)}},$$

and

$$ q_{r}^{{NR}} = \left\{ \begin{gathered} 0,{\text{ }}if{\text{ 0}} \le \varepsilon \le \varepsilon _{0} , \hfill \\ \frac{{\left( {4{\mkern 1mu} \varepsilon - 1} \right)\left( {1 - b} \right) + 4x - 2\left( {1 + b} \right)\left( {1 - c} \right)}}{{4\left( {3 + b} \right)\left( {1 - b} \right)}}, \quad if \quad \varepsilon _{0} < \varepsilon \le 1, \hfill \\ \end{gathered} \right. $$

where $\varepsilon _{0} = {{\left( {4x - \left( {1 - b} \right) - 2\left( {1 - c} \right)\left( {1 + b} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {4x - \left( {1 - b} \right) - 2\left( {1 - c} \right)\left( {1 + b} \right)} \right)} {\left( {4\left( {1 - b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {4\left( {1 - b} \right)} \right)}}$. Let $c \le 1 - x$ to guarantee $q_{n}^{{NR}} \ge q_{r}^{{NR}}$.

Thus, if $\varepsilon \le \varepsilon _{0} ,q_{r}^{{NR}} = 0.$ The ex post optimal profits of M and R are $\pi _{M}^{{NR}} = \pi _{M}^{{NR1}}$ and $\pi _{R}^{{NR}} = \pi _{R}^{{NR1}}$. If $\varepsilon > \varepsilon _{0} ,q_{r}^{{NR}} > 0.$ The ex post optimal profits of M and R are $\pi _{M}^{{NR}} = \pi _{M}^{{NR2}}$ and $\pi _{R}^{{NR}} = \pi _{R}^{{NR2}}$.

Next, we calculate the ex ante profits of M and R. If $c \le {{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}},\varepsilon _{0} > 1,q_{r}^{{NR}} = 0$ always holds in the feasible region, and the ex ante profits of M and R are

$$ \Pi _{M}^{{NR{\text{1}}}} = \int_{0}^{1} {\pi _{M}^{{NR1}} d\varepsilon } = \frac{{\left( {3 - 2{\mkern 1mu} c} \right)^{2} }}{{32}} - F, $$

$$ \Pi _{R}^{{NR{\text{1}}}} = \int_{0}^{1} {\pi _{R}^{{NR1}} d\varepsilon = } \frac{{12c^{2} - 36c + {\text{372}}}}{{192}}. $$

If ${{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}} < c \le {{\left( {b - 4x + 3} \right)} \mathord{\left/ {\vphantom {{\left( {b - 4x + 3} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}},q_{r}^{{NR}} > 0$ when $\varepsilon > \varepsilon _{0}$. The corresponding ex ante profits of M and R are

$$ \begin{gathered} \Pi _{M}^{{NR{\text{2}}}} = \int_{0}^{{\varepsilon _{{\text{0}}} }} {\pi _{M}^{{NR1}} d\varepsilon } {\text{ + }}\int_{{\varepsilon _{{\text{0}}} }}^{{\text{1}}} {\pi _{M}^{{NR{\text{2}}}} d\varepsilon } \hfill \\ \quad = \frac{{\kappa _{0} ^{2} \left( { - 2bc + 2\kappa _{0} + 7b - 2c - 4x - 3} \right)}}{{256\left( {3 + b} \right)\left( {1 - b} \right)^{2} }} + \frac{{8\left( {2c - 3} \right)^{2} }}{{256\left( {3 + b} \right)\left( {1 - b} \right)^{2} }} - F, \hfill \\ \end{gathered} $$

$$ \begin{gathered} \Pi _{R}^{{NR{\text{2}}}} = \int_{0}^{{\varepsilon _{0} }} {\pi _{R}^{{NR1}} d\varepsilon } + \int_{{\varepsilon _{0} }}^{1} {\pi _{R}^{{NR2}} d\varepsilon } \hfill \\ {\text{ }} = \frac{{12x\kappa _{0} \left( {\kappa _{0} - 4x} \right) + 8\left( {b + 1} \right)^{3} c^{3} + 12\left( {13b^{3} - 5b^{2} - 23b} \right)c^{2} }}{{768\left( {1 - b} \right)^{2} \left( {3 + b} \right)}} \hfill \\ \quad + \frac{{64x^{3} - 6\left( {71 - 111b + 9b^{2} - b^{3} } \right)c - b^{3} + 199b^{2} - 635b + 373}}{{768\left( {1 - b} \right)^{2} \left( {3 + b} \right)}}, \hfill \\ \end{gathered} $$

where $\kappa _{0} = 2bc - 5b + 2c + 4x + 1$. If $c > {{\left( {b - 4x + 3} \right)} \mathord{\left/ {\vphantom {{\left( {b - 4x + 3} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}}$, the corresponding ex ante profits of the manufacturer and the retailer are

$$ \Pi _{M}^{{NR{\text{3}}}} = \int_{0}^{1} {\pi _{M}^{{NR2}} d\varepsilon } = \frac{{4x\left( {1 + x} \right) + 1 + \left( {3 - 2c} \right)\left( {2\left( {1 - xb - c - x} \right) - b} \right)}}{{8\left( {3 + b} \right)\left( {1 - b} \right)}} - F, $$

$$ \Pi _{R}^{{NR{\text{3}}}} = \int_{0}^{1} {\pi _{R}^{{NR2}} d\varepsilon } = \frac{{6x\left( {2c - 1} \right)\left( {1 + b} \right) + 12x\left( {x - b} \right) + 12\left( {1 - c} \right)^{2} + \left( {13 - 6c} \right)\left( {1 - b} \right)}}{{48\left( {b + 3} \right)\left( {1 - b} \right)}}. $$

As a result, we have

$$ \Pi _{i}^{{NR}} \left( {i = M,R} \right) = \left\{ \begin{gathered} \Pi _{i}^{{NR{\text{1}}}} ,{\text{ }}if{\text{ }}c \le \frac{{5b - 4x - 1}}{{2\left( {1 + b} \right)}}, \hfill \\ \Pi _{i}^{{NR{\text{2}}}} ,{\text{ }}if{\text{ }}\frac{{5b - 4x - 1}}{{2\left( {1 + b} \right)}} < c \le \min \left\{ {\frac{{b - 4x + 3}}{{2\left( {1 + b} \right)}},\frac{1}{2}} \right\}, \hfill \\ \Pi _{i}^{{NR{\text{3}}}} ,{\text{ }}if{\text{ }}\min \left\{ {\frac{{b - 4x + 3}}{{2\left( {1 + b} \right)}},\frac{1}{2}} \right\} < c \le \bar{c}. \hfill \\ \end{gathered} \right. $$

We analyze M’s remanufacturing strategy by comparing the optimal profits under remanufacturing and no remanufacturing.

• 1. If $c \le {{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}}$, we have

  $$\Pi _{M}^{{NR}} - \Pi _{M}^{{NN}} = - F.$$

  Let $F_{1}^{N} = 0$. $\Pi _{M}^{{NR}} \le \Pi _{M}^{{NN}}$ if $F \ge F_{1}^{N}$. Thus, M never remanufactures in this case.

• 2. If ${{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}} < c \le {{\left( {b - 4x + 3} \right)} \mathord{\left/ {\vphantom {{\left( {b - 4x + 3} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}}$, we have

  $$ \Pi _{M}^{{NR}} - \Pi _{M}^{{NN}} = \frac{{\left( {2bc - 3b + 2c + 4x - 1} \right)\left( {2bc - 5b + 2c + 4x + 1} \right)^{2} }}{{256\left( {b + 3} \right)\left( {1 - b} \right)^{2} }} - F. $$

  Let

  $$ F_{2}^{N} = \frac{{\left( {2bc - 3b + 2c + 4x - 1} \right)\left( {2bc - 5b + 2c + 4x + 1} \right)^{2} }}{{256\left( {b + 3} \right)\left( {1 - b} \right)^{2} }}. $$

Then, $\Pi _{M}^{{NR}} > \Pi _{M}^{{NN}}$ and $F < F_{2}^{N}$, and M remanufactures; otherwise, $\Pi _{M}^{{NR}} \le \Pi _{M}^{{NN}}$, and M never remanufactures.

(3) If $c > {{\left( {b - 4x + 3} \right)} \mathord{\left/ {\vphantom {{\left( {b - 4x + 3} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}}$, we have

$$ \Pi _{M}^{{NR}} - \Pi _{M}^{{NN}} = \frac{{\left( {2bc - 3b + 2c + 4x - 1} \right)^{2} }}{{32\left( {b + 3} \right)\left( {1 - b} \right)}} - F. $$

Let.

$$ F_{3}^{N} = \frac{{\left( {2bc - 3b + 2c + 4x - 1} \right)^{2} }}{{32\left( {b + 3} \right)\left( {1 - b} \right)}}. $$

Then, $\Pi _{M}^{{NR}} > \Pi _{M}^{{NN}}$ and $F < F_{3}^{N}$, and M remanufactures; otherwise, $\Pi _{M}^{{NR}} \le \Pi _{M}^{{NN}}$, and M never remanufactures. Define

$$ \Omega _{N} = \left\{ \begin{gathered} F_{0}^{N} ,{\text{ }}if{\text{ }}c \le \frac{{5b - 4x - 1}}{{2\left( {1 + b} \right)}}, \hfill \\ F_{1}^{N} ,{\text{ }}if{\text{ }}\frac{{5b - 4x - 1}}{{2\left( {1 + b} \right)}} < c \le \min \left\{ {\frac{1}{2},\frac{{b - 4x + 3}}{{2\left( {1 + b} \right)}}} \right\}, \hfill \\ F_{2}^{N} ,{\text{ }}if{\text{ }}\frac{{b - 4x + 3}}{{2\left( {1 + b} \right)}} < c \le \bar{c}. \hfill \\ \end{gathered} \right. $$

Thus, M remanufactures if and only if $F \le \Omega _{N}$.

Proof of Proposition 2 We solve the equilibrium of remanufacturing and no remanufacturing with information sharing.

No remanufacturing Given that R shares demand information, if M does not remanufacture, the profit function of R is

$$ \pi _{R}^{{IN}} = \left( {1 + \varepsilon - q_{n}^{{IN}} - w_{n}^{{IN}} } \right)q_{n}^{{IN}} . $$

Given wholesale price $w_{n}$, R’s optimal order quantity is

$$ q_{n}^{{IN}} (w_{n}^{{IN}} ) = \frac{1}{2}\left( {1 + \varepsilon - w_{n}^{{IN}} } \right). $$

With demand information, the problem of M for the case with no remanufacturing is

$$ \mathop {\max }\limits_{{w_{n}^{{IN}} }} \left( {w_{n}^{{IN}} - c} \right)\left( {\frac{{1 + \varepsilon - w_{n}^{{IN}} }}{2}} \right). $$

The optimal wholesale price of the new product is

$$ w_{n}^{{IN}} = \frac{1}{2}\left( {1 + \varepsilon + c} \right). $$

By substituting the optimal wholesale price into R’s order quantity response function, we write the optimal ex post optimal order quantity as

$$ q_{n}^{{IN}} = \frac{1}{4}\left( {1 + \varepsilon - c} \right). $$

Obviously, $q_{n}^{{IN}} > 0$, and the ex post optimal profits of M and R are

$$\pi _{M}^{{IN}} = \frac{{\left( {1 + \varepsilon - c} \right)^{2} }}{8}$$

and

$$\pi _{R}^{{IN}} = \frac{{\left( {1 + \varepsilon - c} \right)^{2} }}{{16}}$$

The ex ante profits of M and R are

$\Pi _{M}^{{IN}} = \int_{0}^{1} {\frac{{\left( {1 + \varepsilon - c} \right)^{2} }}{8}d\varepsilon = } \frac{{3c^{2} - 9c + 7}}{{24}}$ and $\Pi _{R}^{{IN}} = \int_{0}^{1} {\frac{{\left( {1 + \varepsilon - c} \right)^{2} }}{{16}}d\varepsilon = } \frac{{3c^{2} - 9c + {\text{7}}}}{{48}}.$

Remanufacturing Given that R shares demand information, if M remanufactures, the profit function of R is

$$ \pi _{R}^{{IR}} = \left( {1 + \varepsilon - q_{n}^{{IR}} - bq_{r}^{{IR}} - w_{n}^{{IR}} } \right)q_{n}^{{IR}} + \left( {x + \varepsilon - q_{r}^{{IR}} - q_{n}^{{IR}} - w_{r}^{{IR}} } \right)q_{r}^{{IR}} . $$

Given the wholesale price $w_{n}$ of the new product and the wholesale price $w_{r}$ of the remanufactured product, R’ optimal order quantities are

$$ q_{n}^{{IR}} (w_{n}^{{IR}} ,w_{r}^{{IR}} ) = \frac{{\left( {1 - b} \right)\varepsilon - \left( {1 + b} \right)\left( {x - w_{r}^{{IR}} } \right) + 2\left( {1 - w_{n}^{{IR}} } \right)}}{{\left( {3 + b} \right)\left( {1 - b} \right)}}, $$

$$ q_{r}^{{NR}} (w_{n}^{{NR}} ,w_{r}^{{NR}} ) = \frac{{\left( {1 - b} \right)\varepsilon - \left( {1 + b} \right)\left( {1 - w_{n}^{{NR}} } \right) + 2\left( {x - w_{r}^{{NR}} } \right)}}{{\left( {3 + b} \right)\left( {1 - b} \right)}}. $$

With demand information, the problem of M can be written as

$$ \begin{gathered} \mathop {\max }\limits_{{w_{n}^{{IR}} ,w_{r}^{{IR}} }} \left( {w_{n}^{{IR}} - c} \right)\left( {\frac{{\left( {1 - b} \right)\varepsilon - \left( {1 + b} \right)\left( {x - w_{r}^{{IR}} } \right) + 2\left( {1 - w_{n}^{{IR}} } \right)}}{{\left( {3 + b} \right)\left( {1 - b} \right)}}} \right) \hfill \\ {\text{ }} + w_{r}^{{IR}} \left( {\frac{{\left( {1 - b} \right)\varepsilon - \left( {1 + b} \right)\left( {1 - w_{n}^{{IR}} } \right) - 2\left( {x - w_{r}^{{IR}} } \right)}}{{\left( {3 + b} \right)\left( {1 - b} \right)}}} \right) - F. \hfill \\ \end{gathered} $$

The optimal wholesale price of the new product and the remanufactured product is as follows.

$$ w_{n}^{{IR}} = \frac{1}{2}\left( {1 + \varepsilon + c} \right),w_{r}^{{IR}} = \frac{1}{2}\left( {1 + \varepsilon } \right). $$

By substituting the optimal wholesale prices into R’s order quantity response functions, we write the optimal ex post optimal order quantities as

$$q_{n}^{{IR}} = \frac{{2\left( {1 - c} \right) + \varepsilon \left( {1 - b} \right) - bx}}{{2\left( {b + 3} \right)\left( {1 - b} \right)}}, $$

and

$$ q_{r}^{{IR}} = \left\{ \begin{gathered} 0,{\text{ , }}if{\text{ 0}} \le \varepsilon \le \varepsilon _{1} , \hfill \\ \frac{{\varepsilon \left( {1 - b} \right) + 2x - \left( {1 - c} \right)\left( {1 + b} \right)}}{{2\left( {3 + b} \right)\left( {1 - b} \right)}},{\text{ }}if{\text{ }}\varepsilon _{1} {\text{ < }}\varepsilon \le 1, \hfill \\ \end{gathered} \right. $$

where $\varepsilon _{1} = {{\left( {4x - \left( {1 - b} \right) - 2\left( {1 - c} \right)\left( {1 + b} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {4x - \left( {1 - b} \right) - 2\left( {1 - c} \right)\left( {1 + b} \right)} \right)} {\left( {4\left( {1 - b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {4\left( {1 - b} \right)} \right)}}$. If $\varepsilon \le \varepsilon _{1}$, the ex post optimal profits of M and R are $\pi _{M}^{{IR}} = \pi _{M}^{{IR1}}$ and $\pi _{R}^{{IR}} = \pi _{R}^{{IR1}}$, respectively. If $\varepsilon > \varepsilon _{1}$, the ex post optimal profits of M and R are $\pi _{M}^{{IR}} = \pi _{M}^{{IR2}}$ and $\pi _{R}^{{IR}} = \pi _{R}^{{IR2}}$, respectively.

Next, we solve the ex ante profits of M and R. If $c \le {{\left( {2\left( {b - x} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {2\left( {b - x} \right)} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}$, $\varepsilon _{1} > 1$, which indicates that $q_{r}^{{IR}} = 0$ always holds and the ex ante profits of M and R are

$$ \Pi _{M}^{{IR1}} = \int_{0}^{1} {\pi _{M}^{{IR1}} d\varepsilon = } \frac{{3c^{2} - 9c + 7}}{{24}} - F, \quad \Pi _{R}^{{IR1}} = \int_{0}^{1} {\pi _{R}^{{IR1}} d\varepsilon = } \frac{{3c^{2} - 9c + {\text{7}}}}{{48}}. $$

If ${{\left( {2\left( {b - x} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {2\left( {b - x} \right)} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} < c \le {{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}$, $q_{n}^{{IR}} = 0$ only when $\varepsilon \le \varepsilon _{1}$. The corresponding ex ante profits of M and R are

$$ \begin{aligned} \Pi _{M}^{{IR2}} &= \int_{0}^{{\varepsilon _{1} }} {\pi _{M}^{{IR1}} d\varepsilon } + \int_{{\varepsilon _{1} }}^{1} {\pi _{M}^{{IR2}} d\varepsilon } \hfill \\ & = \frac{{\left( {1 + b} \right)^{3} c^{3} + 6x\kappa _{0} \left( {\kappa _{0} - 2x} \right) - 3\left( {b^{3} + 3b^{2} + 7b - 3} \right)c^{2} }}{{24\left( {3 + b} \right)\left( {1 - b} \right)^{2} }} \hfill \\ & \quad + \frac{{3\left( {b^{3} + b^{2} + 15b - 9} \right)c - b^{3} + 7b^{2} - 35b + 21}}{{24\left( {3 + b} \right)\left( {1 - b} \right)^{2} }} - F, \hfill \\ \end{aligned} $$

$$\begin{aligned} \Pi _{R}^{{IR2}} & = \int_{0}^{{\varepsilon _{1} }} {\pi _{R}^{{IR1}} d\varepsilon } + \int_{{\varepsilon _{1} }}^{1} {\pi _{R}^{{IR2}} d\varepsilon } \hfill \\ & = \frac{{\left( {1 + b} \right)^{3} c^{3} + 6x\kappa _{1} \left( {\kappa _{1} - 2x} \right) - 3\left( {b^{3} + 3b^{2} + 7b - 3} \right)c^{2} }}{{48\left( {3 + b} \right)\left( {1 - b} \right)^{2} }} \hfill \\ & \quad +\frac{{3\left( {b^{3} + b^{2} + 15b - 9} \right)c - b^{3} + 7b^{2} - 35b + 21}}{{48\left( {3 + b} \right)\left( {1 - b} \right)^{2} }}, \hfill \\ \end{aligned}$$

where $\kappa _{1} = bc - 2b + c + 2x$. If ${{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} < c \le \bar{c}$, the corresponding ex ante profits of M and R are

$$ \Pi _{M}^{{IR3}} = \int_{0}^{1} {\pi _{M}^{{IR2}} d\varepsilon } = \frac{{6\left( {1 - c} \right)\left( {1 - c - x - xb} \right) + \left( {5 - 3c - 3x} \right)\left( {1 - b} \right) + 6x^{2} }}{{24\left( {b + 3} \right)\left( {1 - b} \right)}} - F, $$

$$ \Pi _{R}^{{IR3}} = \int_{0}^{1} {\pi _{R}^{{IR2}} d\varepsilon } = \frac{{6\left( {1 - c} \right)\left( {1 - c - x - xb} \right) + \left( {5 - 3c - 3x} \right)\left( {1 - b} \right) + 6x^{2} }}{{24\left( {b + 3} \right)\left( {1 - b} \right)}}. $$

As a result, we have

$$ \Pi _{i}^{{IR3}} \left( {i = M,R} \right) = \left\{ \begin{gathered} \Pi _{i}^{{IR1}} ,{\text{ }}if{\text{ }}c \le \frac{{2\left( {b - x} \right)}}{{1 + b}}, \hfill \\ \Pi _{i}^{{IR2}} ,{\text{ }}if{\text{ }}\frac{{2\left( {b - x} \right)}}{{1 + b}} < c \le \frac{{b - 2x + 1}}{{1 + b}}, \hfill \\ \Pi _{i}^{{IR3}} ,{\text{ }}if{\text{ }}\frac{{b - 2x + 1}}{{1 + b}} < c \le \bar{c}. \hfill \\ \end{gathered} \right. $$

Similar to that shown by the proof of Proposition 8, we find that M remanufactures if and only if $F \le \Omega _{I}$.

Proof of Proposition 3 As $\Omega _{N} \le \Omega _{I}$, we analyze R’s optimal information sharing decision for the scenarios of $F \le \Omega _{N}$, $F > \Omega _{I}$ and $\Omega _{N} < F \le \Omega _{I}$.

Scenario 1 When $F \le \Omega _{N}$, regardless of whether R shares demand information, M will always remanufacture. In anticipating M’s decision, R makes the information sharing decision by comparing her optimal ex ante profits from information sharing and no information sharing.

• (1.1) If $c \le {{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}}$, $\Pi _{R}^{{IR}} - \Pi _{R}^{{NR}} = - {1 \mathord{\left/ {\vphantom {1 {64}}} \right. \kern-\nulldelimiterspace} {64}}$. Obviously, $\Pi _{R}^{{IR}} < \Pi _{R}^{{NR}}$. Thus, R does not share information in this case.

• (1.2) If ${{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}} < c \le {{\left( {2\left( {b - x} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {2\left( {b - x} \right)} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}$, we assume that $f_{1} \left( c \right) = \Pi _{R}^{{IR}} - \Pi _{R}^{{NR}}$. As $f^{\prime}_{1} \left( c \right) < 0$ and $f_{1} \left( {c = {{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}}} \right) < 0$, $\Pi _{R}^{{IR}} < \Pi _{R}^{{NR}}$ and R do not share information in this case.

• (1.3) If ${{\left( {2\left( {b - x} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {2\left( {b - x} \right)} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} < c \le {{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}$, we have $f^{\prime}_{1} \left( c \right) < 0$ and $f_{1} \left( {c = {{\left( {2\left( {b - x} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {2\left( {b - x} \right)} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}} \right) < 0$. Thus, $\Pi _{R}^{{IR}} < \Pi _{R}^{{NR}}$, and R does not share information in this case.

• (1.4) If ${{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} \le c < {{\left( {b - 4x + 3} \right)} \mathord{\left/ {\vphantom {{\left( {b - 4x + 3} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}}$, we have

  $$ \Pi _{R}^{{IR}} - \Pi _{R}^{{NR}} = - \frac{{\left( {1 + b} \right)\left( {2bc - b + 2c + 4x - 3} \right)^{2} }}{{128\left( {3 + b} \right)\left( {1 - b} \right)^{2} }} $$

  Obviously, and R do not share information in this case.

• (1.5) If, $(b - 4x + 3)/(2(1 + b)) \le c < \bar{c}$ we have

  $$ \Pi _{R}^{{IR}} - \Pi _{R}^{{NR}} = \frac{{2bx + b + 4c + 2x - 5}}{{8\left( {b + 3} \right)\left( {1 - b} \right)}}. $$

Thus, $\Pi _{R}^{{IR}} < \Pi _{R}^{{NR}}$ and R do not share information in this case. Consequently, R never shares information when $F \le \Omega _{N}$.

Scenario 2 When $F > \Omega _{I}$, similar to that shown by the proof of, we can obtain $\Pi _{R}^{{IR}} < \Pi _{R}^{{NR}}$. Thus, R never shares information when.

Scenario 3 When, M’s remanufacturing strategy depends on R’s information sharing decision. Specifically, M remanufactures if R shares demand information, while M does not remanufacture if R does not share information. In anticipating M’s reaction, R makes her demand information sharing decision. Next, we determine R’s best information sharing strategy by comparing her equilibrium profits with and without information sharing.

• 3.1 If $c \le {{\left( {2\left( {b - x} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {2\left( {b - x} \right)} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}$, we have $\Pi _{R}^{{IR}} = \Pi _{R}^{{NN}}$ and R does not share demand information.

• 3.2 If ${{\left( {2\left( {b - x} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {2\left( {b - x} \right)} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} < c \le {{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}$, we have

  $$ \begin{gathered} \Pi _{R}^{{IR}} - \Pi _{R}^{{NN}} = \frac{{4\left( {1 + b} \right)^{3} c^{3} - 35b^{3} + 32x^{3} - 3b^{2} + 15b - 9}}{{192\left( {3 + b} \right)\left( {1 - b} \right)^{2} }} \hfill \\ {\text{ }} - \frac{{24\left( {b - x} \right)\left( {bc - 2b + c} \right)\left( {bc + c + 2x} \right)}}{{192\left( {3 + b} \right)\left( {1 - b} \right)^{2} }}. \hfill \\ \end{gathered} $$

  When $f_{2} \left( c \right) = \Pi _{R}^{{IR}} - \Pi _{R}^{{NN}}$, we have $f_{1} \left( {c = {{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}} \right) > 0$ and $f_{2} \left( c \right) > 0$ when ${{\left( {2\left( {b - x} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {2\left( {b - x} \right)} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} < c \le {{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}$. Thus, we have $\Pi _{R}^{{IR}} < \Pi _{R}^{{NN}}$, and R does not share demand information in this case.

• 3.3 If ${{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} < c \le \bar{c}$, we have

  $$ \begin{gathered} \Pi _{R}^{{IR}} - \Pi _{R}^{{NR}} = \frac{{12c\left( {1 + b} \right)\left( {bc - 3b + c + 4x - 1} \right)}}{{192\left( {b + 3} \right)\left( {1 - b} \right)}} \hfill \\ {\text{ }} + \frac{{31b^{2} + 22b - 24x\left( {3b - 2x - 1} \right) - 5}}{{192\left( {b + 3} \right)\left( {1 - b} \right)}}. \hfill \\ \end{gathered} $$

By solving $\Pi _{R}^{{IR}} - \Pi _{R}^{{NR}} = 0$ for $c$, we have

$$c = \tau _{0} = \frac{{9b - 12x + 3 + 2\sqrt {6 - 3b^{2} - 3b} }}{{6\left( {1 + b} \right)}},{\text{ }}c = \tau ^{\prime}_{0} = \frac{{9b - 12x + 3 - 2\sqrt {6 - 3b^{2} - 3b} }}{{6\left( {b + 1} \right)}}$$

We have $\Pi _{R}^{{IR}} \le \Pi _{R}^{{NN}}$ when ${{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} < c \le \tau _{0}$ and $\Pi _{R}^{{IR}} > \Pi _{R}^{{NN}}$ when $\tau _{0} < c < \bar{c}$.

By combining all of the results of scenario 3, R shares information only when $\Omega _{1} < F \le \Omega _{2}$ and $\tau _{0} < c < \bar{c}$.

Proof of Proposition 5 We calculate the ex ante total environmental impact in the equilibrium of each case as follows:

• Case 1 In equilibrium NN, the ex ante expected environmental impact is

  $$E^{{NN}} = \int_{0}^{1} {q_{n}^{{NN}} } d\varepsilon = \frac{3}{8} - \frac{c}{4}$$

• Case 2 In equilibrium NR, if $c \le {{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}}$, we have

  $$E^{{NR}} = \int_{0}^{1} {q_{n}^{{NR}} } d\varepsilon = \frac{3}{8} - \frac{c}{4}.$$

  If ${{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}} < c \le {{\left( {b - 4x + 3} \right)} \mathord{\left/ {\vphantom {{\left( {b - 4x + 3} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}}$, we have

  $$E^{{NR}} = \int_{0}^{1} {q_{n}^{{NR}} } d\varepsilon + \delta \left( {\int_{0}^{{\varepsilon _{0} }} 0 d\varepsilon + \int_{{\varepsilon _{0} }}^{1} {q_{r}^{{NR}} } d\varepsilon } \right) = \frac{{\kappa _{0} ^{2} \left( {2b\delta + 2b + 6\delta + 3} \right) + 8\left( {1 - b} \right)^{2} \left( {3 - 2c} \right)}}{{64\left( {1 - b} \right)^{2} }}.$$

  If ${{\left( {b - 4x + 3} \right)} \mathord{\left/ {\vphantom {{\left( {b - 4x + 3} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}} < c \le \bar{c}$, we have

  $$E^{{NR}} = \int_{0}^{1} {q_{n}^{{NR}} } d\varepsilon + \delta \int_{0}^{1} {q_{r}^{{NR}} } d\varepsilon = \frac{{\left( {\kappa _{0} + 2b - 2} \right)\delta + 5 - b - 4c - 2x\left( {1 + b} \right)}}{{4\left( {3 + b} \right)\left( {1 - b} \right)}}.$$

• Case 3 In equilibrium IN, we have

  $$E^{{IN}} = \int_{0}^{1} {q_{n}^{{IN}} } d\varepsilon = \frac{3}{8} - \frac{c}{4}$$

• Case 4 In equilibrium IR: If $c \le {{\left( {2\left( {b - x} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {2\left( {b - x} \right)} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}$, we have

  $$E^{{IR}} = \int_{0}^{1} {q_{n}^{{IR}} } d\varepsilon = \frac{3}{8} - \frac{c}{4}.$$

  If ${{\left( {2\left( {b - x} \right)} \right)} \mathord{\left/ {\vphantom {{\left( {2\left( {b - x} \right)} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} < c \le {{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}$, we have

  $$E^{{IR}} = \int_{0}^{1} {q_{n}^{{IR}} } d\varepsilon + \delta \left( {\int_{0}^{{\varepsilon _{1} }} 0 d\varepsilon + \int_{{\varepsilon _{1} }}^{1} {q_{r}^{{IR}} } d\varepsilon } \right) = \frac{{\left( {3 + b + \kappa _{{\text{0}}} - 4x - 4c} \right)}}{{8\left( {1 - b} \right)}}$$

  If ${{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} < c \le \bar{c}$, we have

  $$E^{{IR}} = \int_{0}^{1} {q_{n}^{{IR}} } d\varepsilon + \delta \int_{0}^{1} {q_{r}^{{IR}} } d\varepsilon = \frac{{\left( {2\kappa _{{\text{0}}} + b - 1} \right)\delta + 5 - b - 4c - 2x\left( {1 + b} \right)}}{{4\left( {b + 3} \right)\left( {1 - b} \right)}}.$$

Next, we compare the environmental impact of each equilibrium to benchmark NN.

Scenario 1 $F \le \Omega _{1}$:

• If $c \le {{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}}$, we have $E^{{IR}} = E^{{NN}}$.

• If ${{\left( {5b - 4x - 1} \right)} \mathord{\left/ {\vphantom {{\left( {5b - 4x - 1} \right)} {\left( {2\left( {1 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {2\left( {1 + b} \right)} \right)}} < c \le {{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}}\delta \le {{\left( {1 + b} \right)} \mathord{\left/ {\vphantom {{\left( {1 + b} \right)} 2}} \right. \kern-\nulldelimiterspace} 2}$, we have $E^{{IR}} - E^{{NN}} = \frac{{\left( {2\delta - \left( {1 + b} \right)} \right)\left( {2bc - 5b + 2c + 3} \right)^{2} }}{{64\left( {3 + b} \right)\left( {1 - b} \right)^{2} }}$

  Obviously, $E^{{IR}} \le E^{{NN}}$ when $\delta \le {{\left( {1 + b} \right)} \mathord{\left/ {\vphantom {{\left( {1 + b} \right)} 2}} \right. \kern-\nulldelimiterspace} 2}$ and $E^{{IR}} > E^{{NN}}$ when $\delta > {{ = \left( {1 + b} \right)} \mathord{\left/ {\vphantom {{ = \left( {1 + b} \right)} 2}} \right. \kern-\nulldelimiterspace} 2}$.

• If ${{\left( {b - 2x + 1} \right)} \mathord{\left/ {\vphantom {{\left( {b - 2x + 1} \right)} {\left( {1 + b} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {1 + b} \right)}} < c \le \bar{c}$, we have

  $$E^{{NR}} - E^{{NN}} = \frac{{\left( {2\delta - \left( {1 + b} \right)} \right)\left( {2bc - 3b + 2c + 1} \right)}}{{8\left( {b + 3} \right)\left( {1 - b} \right)}}$$

Hence, $E^{{IR}} \le E^{{NN}}$ when $\delta \le {{\left( {1 + b} \right)} \mathord{\left/ {\vphantom {{\left( {1 + b} \right)} 2}} \right. \kern-\nulldelimiterspace} 2}$ and $E^{{IR}} > E^{{NN}}$ when $\delta > {{ = \left( {1 + b} \right)} \mathord{\left/ {\vphantom {{ = \left( {1 + b} \right)} 2}} \right. \kern-\nulldelimiterspace} 2}$.

Similarly, the proofs for when $\Omega _{1} < F \le \Omega _{2}$ and $F > \Omega _{2}$ are similar to the proof for when $F \le \Omega _{1}$. We do not include the proofs to save space.

Proof of Proposition 6 By relaxing the constraint of $c < \min \left\{ {{1 \mathord{\left/ {\vphantom {1 {2,1 - x}}} \right. \kern-\nulldelimiterspace} {2,1 - x}}} \right\}$, we have

$$ \tilde{q}_{n}^{{NN}} = \left\{ \begin{gathered} 0,{\text{ }}if{\text{ }}\varepsilon \le \frac{{2c - 1}}{4}, \hfill \\ \frac{1}{8}\left( {1 + 4\varepsilon - 2c} \right){\text{ }}if{\text{ }}\varepsilon > \frac{{2c - 1}}{4}{\text{. }} \hfill \\ \end{gathered} \right. $$

When $c \le {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}$, the ex ante profits of M and R are

$$ \tilde{\Pi }_{M}^{{NN}} = \int_{0}^{1} {\frac{{\left( {3 - 2c} \right)\left( {1 + 4\varepsilon - 2c} \right)}}{{32}}d\varepsilon = } \frac{{\left( {3 - 2{\mkern 1mu} c} \right)^{2} }}{{32}}, $$

$$\tilde{\Pi }_{R}^{{NN}} = \int_{0}^{1} {\frac{{\left( {1 + 4\varepsilon - 2c} \right)^{2} }}{{64}}d\varepsilon = } \frac{{12c^{2} - 36c + {\text{372}}}}{{192}}.$$

When $c > {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}$, the ex ante profits of M and R are

$$ \tilde{\Pi }_{M}^{{NN}} = \int_{0}^{{{{\left( {2c - 1} \right)} \mathord{\left/ {\vphantom {{\left( {2c - 1} \right)} 4}} \right. \kern-\nulldelimiterspace} 4}}} {0d\varepsilon } + \int_{{{{\left( {2c - 1} \right)} \mathord{\left/ {\vphantom {{\left( {2c - 1} \right)} 4}} \right. \kern-\nulldelimiterspace} 4}}}^{1} {\frac{{\left( {3 - 2c} \right)\left( {1 + 4\varepsilon - 2c} \right)}}{{32}}d\varepsilon } = \frac{{\left( {3 - 2{\mkern 1mu} c} \right)\left( {5 - 2{\mkern 1mu} c} \right)^{2} }}{{256}}, $$

$$ \tilde{\Pi }_{R}^{{NN}} = \int_{0}^{{{{\left( {2c - 1} \right)} \mathord{\left/ {\vphantom {{\left( {2c - 1} \right)} 4}} \right. \kern-\nulldelimiterspace} 4}}} {0d\varepsilon } + \int_{{{{\left( {2c - 1} \right)} \mathord{\left/ {\vphantom {{\left( {2c - 1} \right)} 4}} \right. \kern-\nulldelimiterspace} 4}}}^{1} {\frac{{\left( {1 + 4\varepsilon - 2c} \right)^{2} }}{{64}}d\varepsilon = } \frac{{\left( {5 - 2{\mkern 1mu} c} \right)^{3} }}{{768}}. $$

Next, we consider the cases of NR and IR with constraint $q_{r} = q_{n}$. Similar to proofs of the previous problem, we obtain the optimal production quantities for the case of NR as

$$ \tilde{q}_{n}^{{NR}} = \tilde{q}_{r}^{{NR}} = \left\{ \begin{gathered} 0,{\text{ }}if{\text{ }}\varepsilon \le \frac{{c - x}}{4}, \hfill \\ \frac{{x + 4\varepsilon - c}}{{4\left( {3 + b} \right)}},{\text{ }}if{\text{ }}\varepsilon > \frac{{c - x}}{4}. \hfill \\ \end{gathered} \right. $$

The ex ante profits of M and R are written as

$$ \tilde{\Pi }_{M}^{{NR}} = \left\{ \begin{gathered} \frac{{\left( { - x - 2 + c} \right)^{2} }}{{8\left( {b + 3} \right)}} - F,{\text{ }}if{\text{ }}c \le x, \hfill \\ \frac{{\left( {2 + x - c} \right)\left( {4 + x - c} \right)^{2} }}{{64\left( {3 + b} \right)}} - F,{\text{ }}if{\text{ }}c > x, \hfill \\ \end{gathered} \right. $$

$$ \tilde{\Pi }_{M}^{{NR}} = \left\{ \begin{gathered} \frac{{\left( {4 + x - c} \right)^{2} \left( {{\text{1 + }}\delta } \right)}}{{32\left( {3 + b} \right)}},{\text{ }}if{\text{ }}c \le x, \hfill \\ \frac{{3\left( {c - x} \right)\left( {4 + c - x} \right) + 16}}{{48\left( {3 + b} \right)}},{\text{ }}if{\text{ }}c > x. \hfill \\ \end{gathered} \right. $$

Unlike in the case with no remanufacturing, M remanufactures when $\tilde{\Pi }_{M}^{{NR}} < \tilde{\Pi }_{M}^{{NN}}$. Let $F = \Phi _{N}$ be $\tilde{q}_{r} = \tilde{q}_{n}$ the unique solution of $g_{1} (F) = 0$ where $g_{1} (F) = \tilde{\Pi }_{M}^{{NR}} - \tilde{\Pi }_{M}^{{NN}}$. Thus, M remanufactures when $0 < F \le \Phi _{N}$. With information, if M remanufactures under the constraint of, the optimal production quantities are $\tilde{q}_{n}^{{IR}} = \tilde{q}_{r}^{{IR}} = {{\left( {1 + x + 2\varepsilon - c} \right)} \mathord{\left/ {\vphantom {{\left( {1 + x + 2\varepsilon - c} \right)} {\left( {4\left( {3 + b} \right)} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {4\left( {3 + b} \right)} \right)}}$. The expect profits of M and R are

$$ \tilde{\Pi }_{M}^{{IR}} = \frac{{3\left( {x - c} \right)\left( {4 + x - c} \right) + 13}}{{24\left( {b + 3} \right)}} - F,\tilde{\Pi }_{R}^{{IR}} = \frac{{3\left( {x - c} \right)\left( {4 + x - c} \right) + 13}}{{48\left( {b + 3} \right)}}. $$

Let $F = \Phi _{I}$ be the unique solution of $g_{2} (F) = 0$, where $g_{2} (F) = \tilde{\Pi }_{M}^{{IR}} - \tilde{\Pi }_{M}^{{IN}}$. M remanufactures when $0 < F \le \Phi _{I}$. As $\Phi _{N} < \Phi _{I}$, M always remanufactures when $F \le \Phi _{N}$ and never remanufactures when $F > \Phi _{I}$ regardless of whether R shares her information. When $\max \left\{ {\Phi _{N} ,0} \right\} < F \le \Phi _{I}$, M remanufactures if R shares demand information and does not without demand information. In anticipating M’s reaction, R makes her information sharing decision by comparing her optimal profits from cases $IR$ and $NN$. First, if $c \le {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}$, then

$$ \tilde{\Pi }_{R}^{{IR}} - \tilde{\Pi }_{R}^{{NN}} = - \frac{{12\left( {2 + b} \right)c^{2} - 12\left( {3b - 2x + 5} \right)c - 12x^{2} + 31b - 48x + 41}}{{192\left( {3 + b} \right)}}. $$

By solving $\tilde{\Pi }_{R}^{{IR}} - \tilde{\Pi }_{R}^{{NN}} = 0$ for c, we obtain the feasible solution as

$$ l_{1}^{\prime } = \frac{{15 + 9b - 6x - \sqrt {36x\left( {x + 1} \right)\left( {b + 3} \right) - 12b^{2} - 39b - 21} }}{{12\left( {2 + b} \right)}}. $$

If $c > {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}$, then

$$ \tilde{\Pi }_{R}^{{IR}} - \tilde{\Pi }_{R}^{{NN}} = \frac{{8\left( {b + 3} \right)c^{3} - 12\left( {5b + 11} \right)c^{2} + 6\left( {25b - 16x + 43} \right)c + 48x\left( {x + 4} \right) - 125b - 167}}{{768\left( {b + 3} \right)}}. $$

Let $f_{3} \left( c \right) = \tilde{\Pi }_{R}^{{IR}} - \tilde{\Pi }_{R}^{{NN}}$ and $l^{\prime\prime}_{1}$ be the unique solution of $f_{3} \left( c \right) = 0$ for the feasible region. Assume that

$$ l_{1} = \left\{ \begin{gathered} l^{\prime}_{1} ,{\text{ }}if{\text{ }}c \le \frac{1}{2}, \hfill \\ l^{\prime\prime}_{1} ,{\text{ }}if{\text{ }}c > \frac{1}{2}. \hfill \\ \end{gathered} \right. $$

We have $\tilde{\Pi }_{R}^{{IR}} > \tilde{\Pi }_{R}^{{NN}}$ if $c > l_{1}$ and $\tilde{\Pi }_{R}^{{IR}} \le \tilde{\Pi }_{R}^{{NN}}$ if $c \le l_{1}$. Hence, R shares demand information with M when $\max \left\{ {\Phi _{N} ,0} \right\} < F \le \Phi _{I}$ and $c > l_{1}$.

Appendix B: Additional Analysis

In the main body of the paper, we do not consider constraint $q_{r} \le q_{n}$. In Appendix B, we incorsporate the constraint into our model. Thus, the model uses nonlinear programming. We use KKT conditions to solve the nonlinear programming problem. Due to the complexity of nonlinear programming, we conduct a numerical study to present the results and demonstrate the validity of our main results.

In the following, we show the retailer’s information sharing decision in Fig. 

Fig. 7

Retailer’s information sharing decision ($x = {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}$)

7. Then, we examine the economic impacts of remanufacturing and information sharing on the manufacturer and retailer in Fig. 

Fig. 8

Economic impact of remanufacturing and information sharing ($x = {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}$)

8. Finally, we explore the environmental impact of remanufacturing and information sharing on the environment.

Figure 7 shows that the retailer’s decision does not change when taking constraint $q_{r} \le q_{n}$ into consideration. We find that the retailer shares her information with the manufacturer if and only if the fixed remanufacturing cost is moderate and the remanufacturing cost savings are high.

As shown in Figure 8, when the manufacturer chooses to remanufacture, this always creates win-win outcomes. When the manufacturer abandons remanufacturing, no impact occurs. The results are same as those outlined in Proposition 4.

As shown in Figure

Fig. 9

Environmental impact ($x = {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2},b = {1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}$)

9, intuitively, when the manufacturer does not remanufacture, there is no impact on the environment. When the manufacturer embraces remanufacturing, the environment may improve or worsen depending on the environmental saving parameter. The results are quite similar to those outlined in Proposition 10.

Appendix C: Two-Period Model

In the main model, we explore the retailer’s information strategy adopted within a single period. Next, we introduce a two-period model to examine whether the retailer’s information strategy obtained from our main model still holds. In the model, the manufacturer produces only new products in the first period, but he may make new products and remanufactured products in the second period. The new product price in the first period is, $p_{1} \left( {q_{1} } \right) = 1 + \varepsilon - q_{1}$, and the prices of new and remanufactured products in the second period are $p_{n} \left( {q_{n} ,q_{r} } \right) = 1 + \varepsilon - q_{n} - bq_{r}$ and $p_{r} \left( {q_{n} ,q_{r} } \right) = x + \varepsilon - q_{r} - q_{n}$, respectively. We assume that the production cost of the new product is not too high, i.e., $c \le \left\{ {{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2},{{\left( {9 - 8{\mkern 1mu} x - b^{2} } \right)} \mathord{\left/ {\vphantom {{\left( {9 - 8{\mkern 1mu} x - b^{2} } \right)} {2{\mkern 1mu} \left( {5 - b^{2} } \right)}}} \right. \kern-\nulldelimiterspace} {2{\mkern 1mu} \left( {5 - b^{2} } \right)}}} \right\}$, so that the quantity of the new product is not equal to zero and the quantity of the remanufactured product is less than that of the new product, e.g., $q_{r} \le q_{1}$. Under the case with $NR$, the profit functions of the manufacturer and retailer are

$$ E\left[ {\pi _{M}^{{NR}} } \right] = \left( {w_{1}^{{NR}} - c} \right)E\left[ {q_{1}^{{NR}} } \right] + \left( {w_{n}^{{NR}} - c} \right)E\left[ {q_{n}^{{NR}} } \right] + w_{r}^{{NR}} E\left[ {q_{r}^{{NR}} } \right] - F, $$

(25)

$$ \pi _{R}^{{NR}} = \left( {p_{{\text{1}}}^{{NR}} - w_{{\text{1}}}^{{NR}} } \right)q_{{\text{1}}}^{{NR}} {\text{ + }}\left( {p_{n}^{{NR}} - w_{n}^{{NR}} } \right)q_{n}^{{NR}} + \left( {p_{r}^{{NR}} - w_{r}^{{NR}} } \right)q_{r}^{{NR}} , $$

(26)

where subscript ${\text{1}}$ denotes the first period, and subscripts $n$ and $r$ denote new and remanufactured products, respectively. For the case of $IR$, the corresponding profit functions are

$$ \pi _{M}^{{IR}} = \left( {w_{{\text{1}}}^{{IR}} - c} \right)q_{{\text{1}}}^{{IR}} + \left( {w_{n}^{{IR}} - c} \right)q_{n}^{{IR}} + w_{r}^{{IR}} q_{r}^{{IR}} - F $$

(27)

$$ \pi _{R}^{{IR}} = \left( {p_{{\text{1}}}^{{IR}} - w_{{\text{1}}}^{{IR}} } \right)q_{{\text{1}}}^{{IR}} + \left( {p_{n}^{{IR}} - w_{n}^{{IR}} } \right)q_{n}^{{IR}} + \left( {p_{r}^{{IR}} - w_{r}^{{IR}} } \right)q_{r}^{{IR}} . $$

(28)

In Fig. 10, we show the retailer’s information sharing decision made over of two periods.

Fig. 10

Retailer’s information sharing decision in a two-period setting

We find that the retailer shares her information with the manufacturer if and only if the fixed remanufacturing cost is moderate and the remanufacturing cost savings are high, which is consistent with the retailer’s information strategy obtained from the single-period model.