n-Fold obstinate filters in BL-algebras

Abstract

In this paper, we introduced the notion of n-fold obstinate filter in BL-algebras and we stated and proved some theorems, which determine the relationship between this notion and other types of n-fold filters in a BL-algebra. We proved that if F is a 1-fold obstinate filter, then A/F is a Boolean algebra. Several characterizations of n-fold fantastic filters are given, and we show that A is a n-fold fantastic BL-algebra if A is a MV-algebra (n ≥ 1) and A is a 1-fold positive implicative BL-algebra if A is a Boolean algebra. Finally, we construct some algorithms for studying the structure of the finite BL-algebras and n-fold filters in finite BL-algebras.

Appendix

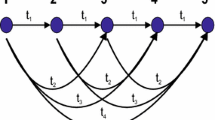
1.1 Diagrams

In diagram (a), we show that the relations between n-fold obstinate filters and other n-fold filters in BL-algebras, also in diagram (b), we show that the relations between structure of BL-algebras and other algebraic structures, where I = Implicative, P.I. = Positive implicative, F = Fantastic.

1.2 Algorithms

In the following, we construct some algorithms for studying finite BL-algebras and (n-fold obstinate) filters in finite BL-algebras.

Algorithm for finite BL-algebras

Input (A: set; $\wedge,\,\vee,\,\ast,\,\rightarrow$: binary operations; 0, 1: constants)

Output (“A is a BL-algebra or not”)

Begin

IfA = Ø then

go to (1.);

EndIf

If (A, ∧, ∨, 0, 1) is not a bounded lattice then

go to (1.);

EndIf

If$(A,\ast,1)$ is not an abelian monoid then

go to (1.);

EndIf

Stop:=false;

i := 1;

While$i\leq \mid A\mid$and not(stop) do

j := 1

While$j\leq \mid A\mid$and not(stop) do

If $x_{i}\wedge y_{j}\neq x_{i}\ast (x_{i}\rightarrow y_{j})$ then

Stop:=true;

EndIf

If $(x_{i}\rightarrow y_{j})\vee (y_{j}\rightarrow x_{i})\neq 1$ then

Stop:=true;

EndIf

k := 1;

While$k\leq \mid A\mid$and not(stop) do

If $(x_{i}\ast y_{j}\leq z_{k})$ and

$x_{i}>(y_{j}\rightarrow z_{k})$ then

Stop:=true;

EndIf

EndWhile

EndWhile

EndWhile

If Stop then

(1.) Output (“A is not a BL-algebra”)

Else

Output (“A is a BL-algebra”)

EndIf

End

Algorithm for filters in finite BL-algebras

Input (A: BL-algebra, F: subset of A);

Output (“F is a filter or not”)

Begin

IfF = Ø then

go to (1.);

EndIf

If $1 \not\in F$ then

go to (1.);

EndIf

Stop:=false;

i := 1;

While$i\leq \mid A\mid$and not(stop) do

j := 1

While$j\leq \mid A\mid$and not(stop)do

If $(x_{i}\in F)$ and $(x_{i}\rightarrow y_{j}\in F)$

then

If $y_{j}\not\in F$ then

Stop:=true;

EndIf

EndIf

EndWhile

EndWhile

If Stop then

(1.) Output (“F is not a filter”)

Else

Output (“F is a filter”)

EndIf

End

Algorithm for n-fold obstinate filters in finite BL-algebras

Input (A: BL-algebra, F: filter of A,  x: element of A,  n: natural number);

Output (“F is a n-fold obstinate filter or not”)

Begin

IfF = Ø then

go to (1.)

EndIf

Stop:=false;

i := 1;

While$i\leq \mid A\mid$and not(stop) do

j := 1

While$j\leq \mid A\mid$and not(stop) do

If $(x_{i}\not\in F)$ and $(y_{j}\not\in F)$ then

If $(x^{n}_{i}\rightarrow y_{j})\not\in F$ or $(y^{n}_{j}\rightarrow x_{i})\not\in F$ then

Stop:=true;

EndIf

EndIf

EndWhile

EndWhile

If Stop then

(1.) Output (“F is not a n-fold obstinate filter”)

Else

Output (“F is a n-fold obstinate filter”)

EndIf

End