Abstract
We reconsider the class of weighted Kalai-Smorodinsky solutions of Dubra (Econ Lett 73:131–136, 2001), and using methods of Imai (Econometrica 51:389–401, 1983), extend their characterization to the domain of multilateral bargaining problems. Aside from standard axioms in the literature, this result involves a new property that weakens the axiom Bilateral Consistency (Lensberg, J Econ Theory 45:330–341, 1988), by making the notion of consistency dependent on how ideal values in a reduced problem change relative to the original problem.











Similar content being viewed by others
Notes
Lensberg calls this axiom Bilateral Stability.
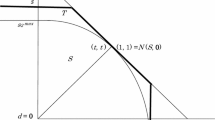
This claim is more carefully motivated in Sect. 2.3.1.
Vector inequalities: \(\geqq \), \(\ge \), \(>\).
Inclusion is denoted \(\subseteq \), and strict inclusion \(\subset \).
For \(N\in \mathcal {N}\) and \(S\in \Sigma ^{N}\), the Nash solution (Nash 1950) is defined as the unique maximizer of \(\prod _{i\in N}x_{i}\) on \(S\cap \mathbb {R}^{N}_{+}\). A proportional solution is defined as \(\beta ^{*}w\) where w is some vector in \(\mathbb {R}^{N}_{++}\), and \(\beta ^{*}:=\max \{\beta \mid \beta w\in S\}\). The Kalai-Smorodinsky solution (Kalai and Smorodinsky 1975) is defined as \(K(S):=\beta ^{*}u(S)\), where \(\beta ^{*}:=\max \{\beta \mid \beta u(S)\in S\}\). The Raiffa solution is defined as the (possibly infinite) sum \(\frac{1}{|N|}u(S)+\frac{1}{|N|}u(S-\frac{1}{|N|}u(S))+\frac{1}{|N|}u(S-\frac{1}{|N|}u(S)-\frac{1}{|N|}u(S-\frac{1}{|N|}u(S)))+\ldots \).
See also Luce and Raiffa (1957, p. 133).
Peters et al. refer to reduced problems as reduced games.
Given \(N\in \mathcal {N}\) and \(V\subset \mathbb {R}^{N}\), \(cch\, V\) denotes the convex comprehensive hull of (the points in) V. It is defined as the intersection of all convex and comprehensive sets in \(\mathbb {R}^{N}\) that contain (the points in) V.
See the solution D, defined in Sect. 4.1.
This proof is included in the Appendix.
For all \(Q,N\in \mathcal {N}\) with \(Q\subset N\) and \(|Q|=2\), and for all \(S\in \Sigma ^{N}\).
It is not known in general whether Imai’s (1983) lexicographic Kalai-Smorodinsky solution – and by extension, the weighted generalizations considered in this paper—can be characterized on this smaller domain.
References
Anbarci N, Sun C (2013) Asymmetric Nash bargaining solutions: a simple Nash program. Econ. Lett. 120:211–214
Bossert W (1993) An alternative solution to bargaining problems with claims. Math. Soc. Sci. 25:205–220
Britz V, Herings JJ, Predtetchinski A (2010) Non-cooperative support for the asymmetric Nash bargaining solution. J. Econ. Theory 145:1951–1967
Chang C, Hwang Y (1999) A characterization of the leximin solution of the bargaining problem. Math. Methods Oper. Res. 49:395–400
Chang C, Liang M (1998) A characterization of the lexicographic Kalai-Smorodinsky solution for \(n=3\). Math. Soc. Sci. 35:307–319
Chen M (2000) Individual monotonicity and the leximin solution. Econ. Theory 15:353–365
Chun Y, Peters H (1989a) The lexicographic egalitarian solution. Cahiers du C.E.R.O 30:149–156
Chun Y, Peters H (1989b) Lexicographic monotone path solutions. OR Spektrum 11:43–47
Chun Y, Peters H (1991) The lexicographic equal-loss solution. Math. Soc. Sci. 22:151–161
Chun Y, Thomson W (1992) Bargaining problems with claims. Math. Soc. Sci. 24:19–33
del Carmen MGM (1995) Efficient solutions for bargaining problems with claims. Math. Soc. Sci. 30:57–69
Driesen B (2012) Proportional concessions and the leximin solution. Econ. Lett. 114:288–291
Dubra J (2001) An asymmetric Kalai-Smorodinsky solution. Econ. Lett. 73:131–136
Harsanyi J (1959) A bargaining model for the cooperative \(n\)-person game. In: Tucker AW, Luce RD (eds) Contributions to the theory of games IV. Princeton University Press, Princeton
Imai H (1983) Individual monotonicity and lexicographic maxmin solution. Econometrica 51:389–401
Kalai E (1977a) Nonsymmetric Nash solutions and replications of 2-person bargaining. Int. J. Game Theory 6:129–133
Kalai E (1977b) Proportional solutions to bargaining situations: interpersonal utility comparisons. Econometrica 45:1623–1630
Kalai E, Smorodinsky M (1975) Other solutions to Nash’s bargaining problem. Econometrica 43:513–518
Laruelle A, Valenciano F (2008) Non-cooperative foundations of bargaining power in committees and the Shapley–Shubik index. Games Econ. Behav. 63:341–353
Lensberg T (1988) Stability and the Nash solution. J. Econ. Theory 45:330–341
Luce D, Raiffa H (1957) Games and decisions: introduction and critical survey. Wiley, New York
Nash J (1950) The bargaining problem. Econometrica 18:155–162
Nieto J (1992) The lexicographic egalitarian solution on economic environments. Soc. Choice Welf. 9:203–212
Peters H, Tijs S, Zarzuelo J (1994) A reduced-game property for the Kalai-Smorodinsky and egalitarian bargaining solutions. Math. Soc. Sci. 27:11–18
Raiffa H (1953) Arbitration schemes for generalized two-person games. In: Kuhn H, Tucker A (eds) Contributions to the Theory of Games II, vol 28., Annals of Mathematics StudiesPrinceton University Press, Princeton, pp 361–387
Roth A (1977) Independence of irrelevant alternatives, and solutions to Nash’s bargaining problem. J. Econ. Theory 16:247–251
Roth A (1979) An impossibility result concerning \(n\)-person bargaining games. Int. J. Game Theory 8:129–132
Thomson W, Lensberg T (1989) Axiomatic theory of bargaining with a variable number of agents. Cambridge University Press, Cambridge
Acknowledgments
I thank Hans Peters, Michele Lombardi and Naoki Yoshihara for useful comments and suggestions. The usual caveat applies.
Author information
Authors and Affiliations
Corresponding author
Appendix
Appendix
This Appendix elaborates on several results of Imai (1983). In particular, Appendix 1 corresponds with Imai’s Lemmas 6 and 7, and part of his Proposition 1 (pp. 396–397). Appendix 2 is a visual illustration of Imai’s auxiliary problems. Appendix 3 is a modification of Imai’s Lemma 5, and part of his Proposition 2 (pp. 396, 398). Appendix 4 repeats the final part of that same Proposition 2.
1.1 Appendix 1: Solutions \(\varphi \in \mathcal {L}\) satisfy IM
Consider some solution \(L^{w}\in \mathcal {L}\) with \(w\in \mathbb {R}^{\mathbb {N}}_{++}\). Let \(N\in \mathcal {N}\), and let S and T be problems in \(\Sigma ^{N}\) with \(T\subseteq S\) and \(T_{-i}=S_{-i}\) for some \(i\in N\). Since \(L^{w}\) satisfies SI, we may assume without loss of generality that \(u(S)=w^{-1}_{N}\), where \(w_{N}\) is the restriction of w to the agents in N. Then
Define \(u:=u(S)u(T)^{-1}\), and observe that
By Equations (5) and (6) it is sufficient to show that \(\xi _{i}(S)\geqq \frac{1}{u_{i}}\xi _{i}(uT)\). This is done in two steps. First it is established that \(\xi _{i}(S)\geqq \xi _{i}(T)\), and subsequently, that \(\xi _{i}(T) \geqq \frac{1}{u_{i}}\xi _{i}(uT)\).
Lemma 6.1
Let \(y\in T\) with \(i\in Q(T,y)\). Then \(Q(T,y)=Q(S,y)\).
Proof
Suppose there is some \(i'\in Q(S,y)\setminus Q(T,y)\). Define \(x:=x(S,y)\) and \(x':=x(T,y)\). Since \(S_{-i}=T_{-i}\) and \(x\in S\), there is a \(z\in T\) such that \(z_{j}=x_{j}\) for all \(j\in N\setminus i\). By convexity of T it follows that \(\lambda x' +(1-\lambda ) z \in T\) for all \(\lambda \in [0,1]\). We have \(x,x'\geqq y\), \(z_{j}=x_{j}\) for all \(j\in N\setminus i\), and \(x_{i}'>y_{i}\) (since \(i\in Q(T,y)\)). Hence, there exists a \(\lambda \in (0,1)\) such that \(z^{*}:=\lambda x' +(1-\lambda ) z\geqq y\) and \(z^{*}\in T\). Since \(i'\in Q(S,y)\setminus Q(T,y)\), \(x_{i'}>y_{i'}=x_{i'}'\). Then \(z_{i'}^{*}=\lambda x_{i'}'+(1-\lambda )x_{i'}>y_{i'}\). This implies \(i'\in Q(T,y)\), a contradiction. \(\square \)
In essence, as long as the ith coordinate can be further increased in problem T, it can be further increased in S as well. It follows that \(\xi _{i}(S)\geqq \xi _{i}(T)\).
To show that \(\xi _{i}(T)\geqq \frac{1}{u_{i}}\xi _{i}(uT)\), define the following:
The aim is to show that \(x_{i}\geqq x_{i}'\).
Observation 6.2
\(x\succ _{N} x'\) and \(y'\succ _{N} y\) (or \(x=x'\) and \(y=y'\)).
Proof
Observe that \(\xi (T)\in T\), implying \(u\xi (T)\in uT\). Since \(\xi (uT)\succ _{N} z\) for all \(z\in uT\) with \(z\ne \xi (uT)\), \(\xi (uT)\succ _{N} u\xi (T)\) (or \(\xi (uT) = u\xi (T)\)). In other words, \(y'\succ _{N}y\) (or \(y'=y\)). Similarly, observe that \(\xi (uT)\in uT\), and thus \(u^{-1}\xi (uT)\in T\). Since \(\xi (T)\succ _{N} z\) for all \(z\in T\) with \(z\ne \xi (T)\), this implies \(\xi (T)\succ _{N} u^{-1}\xi (uT)\) (or \(\xi (T)= u^{-1}\xi (uT)\)). In other words, \(x\succ _{N}x'\) (or \(x=x'\)). Since \(x=x'\) if and only if \(y=y'\), the observation follows. \(\square \)
Lemma 6.3
\(x_{i}\geqq x_{i}'\).
Proof
Clearly, if \(x=x'\) and \(y=y'\), then the inequality holds trivially, and we are done. Hence, assume \(x\succ _{N} x'\) and \(y'\succ _{N} y\). Since \(y'\succ _{N} y\), there is an index \(m\leqq |N|\) such that the first \(m-1\) elements of \(\mu (y)\) and \(\mu (y')\) are equal, and \(\mu _{m}(y')>\mu _{m}(y)\). Assume first that \(x_{i}'\geqq \mu _{m}(y')\). Since \(x'\) and \(y'\) only differ in the i-th coordinate, \(x_{i}'\geqq \mu _{m}(y')\) implies that the first m elements of \(\mu (x')\) and \(\mu (y')\) coincide. Then the first \(m-1\) elements of \(\mu (x')\) coincide with the first \(m-1\) elements of \(\mu (y)\). Observe that x and y only differ in the i-th coordinate and \(y_{i}>x_{i}\), so there are two possibilities:
-
1.
there is some \(k\leqq m-1\) such that the first \(k-1\) elements of \(\mu (y)\) and \(\mu (x)\) coincide, and \(\mu _{k}(y)>\mu _{k}(x)\). Then the first \(k-1\) elements of \(\mu (x')\) and \(\mu (x)\) coincide and \(\mu _{k}(x')>\mu _{k}(x)\). Then \(x'\succ _{N} x\), a contradiction.
-
2.
the first m elements of \(\mu (y)\) and \(\mu (x)\) coincide. Since the first \(m-1\) elements of \(\mu (x')\) and \(\mu (y)\) coincide, this implies that the first \(m-1\) elements of \(\mu (x)\) and \(\mu (x')\) coincide. However, the m-th element of \(\mu (x')\) coincides with the m-th element of \(\mu (y')\). Since \(\mu _{m}(y')>\mu _{m}(y)\) this implies \(\mu _{m}(x')>\mu _{m}(y)\). Since \(\mu _{m}(x)=\mu _{m}(y)\) this implies \(\mu _{m}(x')>\mu _{m}(x)\), and thus \(x'\succ _{N} x\). This is a contradiction.
It follows from the above that \(x_{i}'<\mu _{m}(y')\). Hence, \(x_{i}'=\mu _{m'}(x')\) for some \(m'\in \{1,\ldots ,m-1\}\), and take the lowest \(m'\) in case of ties. Similarly, \(x_{i}=\mu _{k}(x)\) for some \(k\in \{1,\ldots ,n\}\), and take the highest possible k in case of ties. There are two possibilities: \(k\geqq m'\) or \(k<m'\).
-
1.
If \(k<m'\), then \(\mu _{k}(x')=\mu _{k}(y')\) (they only differ in the i-th coordinate; since \(\mu _{m'}(x')=x_{i}\), \(k<m'\) implies that \(\mu _{k'}(x')=\mu _{k'}(y')\) for all \(k'=1,\ldots ,k\)). Since the first \(m-1\) elements of \(\mu (y)\) and \(\mu (y')\) coincide, and \(k<m'\leqq m-1\), the first k elements of \(\mu (y)\) and \(\mu (y')\) coincide. Since x and y only differ in the i-th coordinate and \(x_{i}=\mu _{k}(x)\), the first \(k-1\) elements of \(\mu (x)\) and \(\mu (y)\) coincide. This in turn implies that the first \(k-1\) elements of \(\mu (x)\) and \(\mu (x')\) coincide. Since \(y_{i}>x_{i}\) and by the choice of k (it was chosen such that \(\mu _{k+1}(x)>\mu _{k}(x)\)), \(\mu _{k}(y)>\mu _{k}(x)\). Since the first k elements of \(\mu (y)\) coincide with the first k elements of \(\mu (x')\), this implies \(\mu _{k}(x')>\mu _{k}(x)\). Hence, \(x'\succ _{N} x\), a contradiction.
-
2.
Let \(k\geqq m'\). Observe that the first \(m' -1\) elements of \(\mu (x')\) and \(\mu (y')\) coincide (this is so because they only differ in the i-th coordinate and \(\mu _{m'}(x')=x_{i}'\)). From before, we know that the first \(m-1\) elements of \(\mu (y)\) and \(\mu (y')\) coincide, which implies that their first \(m'\) elements coincide as well. Hence, the first \(m'-1\) elements of \(\mu (x')\) coincide with the first \(m'-1\) elements of \(\mu (y)\). Since x and y only differ in the i-th coordinate and \(x_{i}=\mu _{k}(x)\), the first \(m'\) elements of \(\mu (x)\) coincide with the first \(m'\) elements of \(\mu (y)\). Hence, the first \(m'-1\) elements of \(\mu (x)\) and \(\mu (x')\) coincide. If \(\mu _{m'}(x')>\mu _{m'}(x)\), then \(x'\succ _{N} x\), and we obtain a contradiction. Hence, \(\mu _{m'}(x')\leqq \mu _{m'}(x)\). But then \(x_{i}'=\mu _{m'}(x')\leqq \mu _{m'}(x)\leqq \mu _{k}(x)=x_{i}\), as desired.\(\square \)
1.2 Appendix 2: The Auxiliary Problems of Proposition 3.4
This section presents a worked out example of the auxiliary problems used in the proof of Proposition 3.4, for some weights vector w. Suppose that the problem and the solution outcome of a problem S are as in Fig. 12. Then \(L^{w}(S)\) is reached in two iterations, i.e., \(L^{w}(S)=x^{2}\).
Recall that \(\underline{S}^{0}=H(e(N),1)\). This problem is depicted in Fig. 13a. From \(\underline{S}^{0}\) one can construct the problem \(\overline{S}^{1}\): it is given by \(H(e(N),\alpha ^{1})\cap (e(N)-\mathbb {R}^{N}_{+})\). Thus, the half-space that determines \(\underline{S}^{0}\) slides upwards, and is intersected by a set that limits utilities to 1 (Fig. 13b).
The problem \(S^{1}\) is the intersection of S with the half-space \(H(e(N),\alpha ^{1})\) (Fig. 14a). Similarly, \(S'^{1}\) is the intersection of the problem S and the problem \(\overline{S}^{1}\) (Fig. 14a). Note that these two problems coincide. For the first iteration this is always the case.
To determine the auxiliary problems for the second iteration, one must determine \(\underline{S}^{1}\). It is equal to \(\overline{S}^{1}\), intersected by \(H(p^{2},p^{2}\cdot x^{2})\), a half-space that leaves the utilities of agent 3 free, but restricts those of agents 1 and 2 in a \(\{1,2\}\)-symmetric fashion (Fig. 15a). The problem \(\overline{S}^{2}\) is given by \(H(e(N),\alpha ^{2})\), intersected by that same half-space \(H(p^{2},p^{2}\cdot x^{2})\), and the set \((e(N)-\mathbb {R}^{N}_{+})\) that limits the utilities of all agents to 1 (Fig. 15b).
The problem \(S'^{2}\) is the intersection of \(\overline{S}^{2}\), and the original problem S (Fig. 16a). The problem \(S^{2}\) is given by the intersection of the original problem S and the half-space \(H(e(N),\alpha ^{2})\) (Fig. 16b).
1.3 Appendix 3: Proof of Claim 1
In order to show that \(u(\overline{S}^{j})=u(\underline{S}^{j})=u(S^{j})=u(S'^{j})=e(N)\) for all \(j=1,\ldots , k\), it is sufficient to show that \(e^{i}\) is in \(\overline{S}^{j}, \underline{S}^{j}, S^{j}\) and \(S'^{j}\) for each j and i. This follows from four observations.
-
(a)
\(e^{i}\in H(p^{j},\ p^{j}\cdot x^{j})\) for each i and j;
-
(b)
\(e^{i}\in H(e(N),\alpha ^{j})\) for each i and j;
-
(c)
\(e^{i}\in S\) for all i;
-
(d)
\(e^{i}\in (e(N)-\mathbb {R}^{N}_{+})\) for all i.
Note that (a), (b) and (d) together imply \(e^{i}\in \overline{S}^{j}\) for each i and j. Then by (c), \(e^{i}\in S'^{j}\) for each i and j; for \(j<k\), it is implied by (a) that each \(e^{i}\) is in \(\underline{S}^{j}\). Finally, (b) and (c) together imply \(e^{i}\in S^{j}\) for each i and j.
Observation (d) is trivial. Observation (c) follows from comprehensiveness of S and the assumption that \(u(S)=e(N)\). We now show (a) and (b). Denote \(N\setminus Q^{2}\) by Q, \(Q^{2}\) by \(Q'\), and for \(i\in Q\), denote \(Q'\cup \{i\}\) by \(Q_{i}\). Let \(\bar{w}:=(\bar{w}_{i})_{i\in N}\) where \(\bar{w}_{i}:=w_{i}/\sum _{i'\in Q}w_{i'}\) for all \(i\in N\). Note that \(\sum _{i\in Q}\bar{w}_{i}=1\).
By the supporting hyperplane theorem and the definition of \(x^{1}\), there is a \(p\in \mathbb {R}^{N}_{+}\) with \(p_{i}=0\) for all \(i\in Q'\), such that \(p\cdot z\leqq p\cdot x^{1}\) for all \(z\in S\). By observation (c), \(p\cdot e^{i}\leqq p\cdot x^{1}\) for all \(i\in Q\). Since \(p_{i}=0\) for all \(i\in Q'\), this implies \(p\cdot e(Q_{i})=p\cdot e^{i}\leqq p\cdot x^{1}\) for all \(i\in Q\). It follows that \(p\cdot \sum _{i\in Q}\bar{w}_{i}e(Q_{i})\leqq p\cdot x^{1}\). Note that \(\sum _{i\in Q}\bar{w}_{i}e(Q_{i})=\bar{w}+(e(Q')-\bar{w}e(Q'))\). Since \(p_{i}=0\) for all \(i\in Q'\), we obtain \(p\cdot \bar{w}\leqq p\cdot x^{1}\). Note that \(x^{1}=\alpha ^{1}w_{N}=[\alpha ^{1}\sum _{i\in Q}w_{i}]\bar{w}\). Then \(p\cdot \bar{w}\leqq p\cdot x^{1}\) is equivalent to \(\alpha ^{1}\sum _{i\in Q}w_{i} \geqq 1\). Hence, \(x^{1}=\alpha ^{1}w_{N}\geqq \frac{w_{N}}{\sum _{i\in Q}w_{i}}=\bar{w}\).
Since \(p^{1}\cdot e^{i}=0\) for all \(i\in N\), each \(e^{i}\) is trivially in \(H(p^{1},\ p^{1}\cdot x^{1})\). Consider some \(j\in \{2,\ldots ,k\}\). Note that \(p_{i}^{j}=0\) for all \(i\in Q^{j}\). Hence,
The inequality follows from the observation that \(N\setminus Q^{2}\) is a subset of \(N\setminus Q^{j}\). By the above, \(x^{j}\geqq x^{1}\geqq \bar{w}\). Hence, \(p^{j}\cdot x^{j}\geqq p^{j}\cdot x^{1}\geqq p^{j}\cdot \bar{w}\geqq 1\). Since \(p^{j}\cdot e^{i}\leqq 1\) for all \(i\in N\), we obtain \(p^{j}\cdot x^{j}\geqq p^{j}\cdot e^{i}\) for all \(i\in N\). This establishes observation (a).
Since \(x^{1}\geqq \bar{w}\) and \(\sum _{i\in N}\bar{w}_{i} \geqq \sum _{i\in Q}\bar{w}_{i}=1\), \(e(N)\cdot e^{i}=1\leqq e(N)\cdot \bar{w}\leqq e(N) \cdot x^{1}= \alpha ^{1}\) for all \(i\in N\). Hence, \(e^{i}\in H(e(N),\alpha ^{1})\) for all \(i\in N\). Since \(H(e(N),\alpha ^{1})\subset H(e(N),\alpha ^{j})\) for each \(1<j\leqq k\), observation (b) follows. \(\square \)
1.4 Appendix 4: Proof of Proposition 3.4 (continued)
The proof of Proposition 3.4 is concluded by the following three claims.
Claim 7
\(\varphi (\overline{S}^{1})=\varphi (\underline{S}^{1})=\varphi (S^{1})=\varphi (S'^{1})=x^{1}\).
Proof
Since \(\varphi (\underline{S}^{0})=x^{0}\), \(\varphi (\overline{S}^{1})=x^{1}\) by Claim 6. Since \(x^{1}\in \overline{S}^{1}\) and \(x^{1}\in S\), \(x^{1}\in S'^{1}\). Then \(x^{1}=\varphi (\overline{S}^{1})\in S'^{1}\subseteq \overline{S}^{1}\), and by Claim 1, \(u(S'^{1})=u(\overline{S}^{1})\). Thus by IIIA, \(\varphi (S'^{1})=\varphi (\overline{S}^{1})=x^{1}\). For all \(i\in N\setminus Q^{2}\), \(x^{1}_{i}=x^{2}_{i}\), implying \(p^{2}\cdot x^{1}=p^{2}\cdot x^{2}\). Hence, \(x^{1}\in H(p^{2}, p^{2}\cdot x^{2})\). Then \(x^{1}=\varphi (\overline{S}^{1})\in \underline{S}^{1}\subseteq \overline{S}^{1}\); by Claim 1, \(u(\underline{S}^{1})=u(\overline{S}^{1})\). Then by IIIA, \(\varphi (\underline{S}^{1})=\varphi (\overline{S}^{1})=x^{1}\). To see that \(\varphi (S^{1})=x^{1}\), observe first that \(S'^{1}=S^{1}\cap (e(N)-\mathbb {R}^{N}_{+})\). Since \(\varphi (S^{1})\leqq u(S^{1})=e(N)\), \(\varphi (S^{1})\in (e(N)-\mathbb {R}^{N}_{+})\). Hence, \(\varphi (S^{1})\in S'^{1}\subseteq S^{1}\), and by Claim 1, \(u(S'^{1})=u(S^{1})\). Thus, \(\varphi (S^{1})=\varphi (S'^{1})=x^{1}\) by IIIA. \(\square \)
Claim 8
\(\varphi (\overline{S}^{j})=\varphi (\underline{S}^{j})=\varphi (S^{j})=\varphi (S'^{j})=x^{j}\) for each \(j=1,\ldots ,k\) (or \(j=1,\ldots ,k-1\) for \(\varphi (\underline{S}^{j})\)).
Proof
Consider \(j\in \{2,\ldots ,k\}\), and assume \(\varphi (\overline{S}^{j-1})=\varphi (\underline{S}^{j-1})=\varphi (S^{j-1})=\varphi (S'^{j-1})=x^{j-1}\). By Claim 6, \(\varphi (\overline{S}^{j})=x^{j}\). Then \(\varphi (S'^{j})=x^{j}\) follows as in Claim 7. Furthermore, if \(j<k\), then also \(\varphi (\underline{S}^{j})=x^{j}\) follows as in Claim 7. What is left to show is that \(\varphi (S^{j})=x^{j}\). To this end, it is first argued that \(\varphi (S^{j})\) is an element of \(\overline{S}^{j}\).
-
1.
Since \(S^{j}\subseteq H(e(N),\alpha ^{j})\), \(\varphi (S^{j})\in H(e(N),\alpha ^{j})\).
-
2.
Since \(\varphi (S^{j})\leqq u(S^{j})=e(N)\), \(\varphi (S^{j})\in (e(N)-\mathbb {R}^{N}_{+})\).
-
3.
By part (ii) of Lemma 3.5, \(S_{-i}^{j-1}=S_{-i}^{j}\) for all \(i\in N\). Furthermore, \(S^{j-1}\subseteq S^{j}\). Thus by an |N|-fold application of IM, \(\varphi (S^{j})\geqq \varphi (S^{j-1})=x^{j-1}\). As in Claim 3, this implies \(\varphi _{i}(S^{j})=x_{i}^{j}\) for all \(i\in N\setminus Q^{j}\). From this it follows that for all \(j'\in \{1,\ldots ,j\}\), \(\varphi (S^{j})\in H(p^{j'},p^{j'}\cdot x^{j'})\). Thus, \(\varphi (S^{j})\in \bigcap _{j'=1}^{j}H(p^{j'},p^{j'}\cdot x^{j'})\).
It follows that \(\varphi (S^{j})\in \overline{S}^{j}\). Since \(S^{j}\subseteq S\), we further have \(\varphi (S^{j})\in S\). Thus, \(\varphi (S^{j})\in S'^{j}\subseteq S^{j}\), and by Claim 1, \(u(S^{j})=u(S'^{j})\). Then by IIIA, \(\varphi (S^{j})=\varphi (S'^{j})=x^{j}\). \(\square \)
Claim 9
\(\varphi (S)=x^{k}\).
Proof
Since \(S^{k}=S\cap H(e(N),\alpha ^{k})\), \(\varphi (S)\geqq \varphi (S^{k})\) by an |N|-fold application of IM and part (ii) of Lemma 3.5. Then by Claim 8, \(\varphi (S)\geqq x^{k}\). The claim follows from the observation that \(x^{k}\in P(S)\). \(\square \)
Rights and permissions
About this article
Cite this article
Driesen, B. Bargaining, conditional consistency, and weighted lexicographic Kalai-Smorodinsky Solutions. Soc Choice Welf 46, 777–809 (2016). https://doi.org/10.1007/s00355-015-0936-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00355-015-0936-x