Skip to main content
SpringerLink
Log in

Direct vector control strategy of 2-phase induction motor drives based on the conventional rotating transformation matrix and EKF

  • Original Paper
  • Published:
Electrical Engineering Aims and scope Submit manuscript

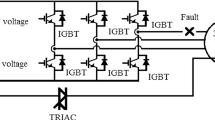
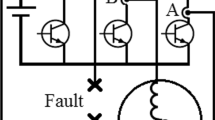
Abstract

This research presents a novel mathematical model of 2-phase induction Motor (M2) or star-connected 3-phase induction Motor (M3) under the Open-Phase Fault (FOP). In addition, a Direct Vector Control (DVC) strategy based on the Extended Kalman Filter (EKF) is proposed for M2 drives. It is shown that using the conventional rotating Transformation Matrix (TM), the mathematical model of the M2 can be written as forward and backward quantities. Based on this modeling, a developed DVC strategy according to forcing the backward quantities equal to zero is achieved for M2 drives. In the proposed DVC, an EKF is developed to estimate the rotor flux amplitude and angle. The proposed approach with minor modifications can be employed for both M3 and M2 drives. The experimental results on a star-connected M3 test-bed with a DSP/TMS320F28335 indicate that the proposed EKF-based DVC (DVCEKF) method can precisely and effectively control the faulty M3 drive during different conditions. The results also show that the proposed DVCEKF system has a better performance in comparison to conventional control systems during the FOP.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17

Similar content being viewed by others

Data availability and materials

The data that support the findings of this study are available on request from the corresponding author.

Abbreviations

\(\alpha \beta ,dq\) :

Stationary reference and rotating reference

\(\left[ {TM^{h} } \right]\) :

\(abc \to \alpha \beta\) Transformation during normal mode

\(\left[ {TM^{f} } \right]\) :

\(ab \to \alpha \beta\) Transformation during the fault mode

\(\left[ {TM^{e} } \right]\) :

Conventional rotating TM

\(\left[ {TM_{f}^{e} } \right]\) :

Forward rotating TM

\(\left[ {TM_{b}^{e} } \right]\) :

Backward rotating TM

\(\begin{gathered} V_{\alpha s} ,V_{\beta s} ,V_{\alpha r} ,V_{\beta r} \hfill \\ V_{ds} ,V_{qs} ,V_{dr} ,V_{qr} \hfill \\ \end{gathered}\) :

Stator and rotor \(\alpha \beta\) and \(dq\) voltages

\(\begin{gathered} I_{\alpha s} ,I_{\beta s} ,I_{\alpha r} ,I_{\beta r} \hfill \\ I_{ds} ,I_{qs} ,I_{dr} ,I_{qr} \hfill \\ \end{gathered}\) :

Stator and rotor \(\alpha \beta\) and \(dq\) currents

\(I_{as} ,I_{bs} ,I_{cs}\) :

Stator line currents

\(\Psi_{\alpha r} ,\Psi_{\beta r} ,\Psi_{dr} ,\Psi_{qr}\) :

Rotor \(\alpha \beta\) and \(dq\) fluxes

\(N_{p}\) :

Number of pole pairs

\(\left| {\Psi_{r} } \right|,\delta_{e}\) :

Rotor flux amplitude and angle

\(R_{s} ,R_{r}\) :

Stator and rotor resistances

\(L_{ds} ,L_{qs} ,L_{r} ,L_{d} ,L_{q}\) :

Stator and rotor self and mutual inductances

\(L_{ls} ,L_{ms}\) :

Stator leakage and magnetizing inductances

\(\Omega_{r}\) :

Rotor electrical angular speed

\(T_{e} ,T_{l}\) :

Electromagnetic torque and external load torque

\(J,F_{r}\) :

Moment of inertia and viscous friction coefficient

\(p\) :

Differential operator

\(X,Y,U\) :

State, input, and output vectors

\(W,V\) :

System and measurement noises

\(f,H\) :

Functions of states and outputs

\(A,B,C\) :

System, input, and measurement matrices

\(T_{s}\) :

Sampling time

\(P,Q,R\) :

Covariance matrices of the state estimation error, system noise, and measurement noise

\(K\) :

Kalman filter gain

Superscripts “*”, “^”:

Reference and estimated values

References

  1. Shi P, Wang X, Meng X, He M, Mao Y, Wang Z (2022) Adaptive fault-tolerant control for open-circuit faults in dual three-phase PMSM drives. IEEE Trans Power Electron 38(3):3676–3688

    Article  Google Scholar 

  2. Aguilera F, de la Barrera PM, De Angelo CH (2022) Speed and current sensor fault-tolerant induction motor drive for electric vehicles based on virtual sensors. Electr Eng 104(5):3157–3171

    Article  Google Scholar 

  3. Gholipour A, Ghanbari M, Alibeiki E, Jannati M (2021) Speed sensorless fault-tolerant control of induction motor drives against current sensor fault. Electr Eng 103(3):1493–1513

    Article  Google Scholar 

  4. Geng Y, Lai Z, Li Y, Wang D, Chen R, Zheng P (2019) Sensorless fault-tolerant control strategy of six-phase induction machine based on harmonic suppression and sliding mode observer. IEEE Access 7:110086–110102

    Article  Google Scholar 

  5. Tousizadeh M, Che HS, Selvaraj J, Rahim NA, Ooi BT (2018) Performance comparison of fault-tolerant three-phase induction motor drives considering current and voltage limits. IEEE Trans Industr Electron 66(4):2639–2648

    Article  Google Scholar 

  6. Tousizadeh M, Che HS, Selvaraj J, Rahim NA, Ooi BT (2018) Fault-tolerant field-oriented control of three-phase induction motor based on unified feedforward method. IEEE Trans Power Electron 34(8):7172–7183

    Article  Google Scholar 

  7. Liu TH, Fu JR, Lipo TA (1993) A strategy for improving reliability of field-oriented controlled induction motor drives. IEEE Trans Ind Appl 29(5):910–918

    Article  Google Scholar 

  8. Kastha D, Bose BK (1994) Fault mode single-phase operation of a variable frequency induction motor drive and improvement of pulsating torque characteristics. IEEE Trans Industr Electron 41(4):426–433

    Article  Google Scholar 

  9. Jannati M, Idris NRN, Salam Z (2012) A new method for modeling and vector control of unbalanced induction motors. In: 2012 IEEE Energy conversion congress and exposition (ECCE) (pp. 3625–3632). IEEE

  10. Jannati M, Monadi A, Idris NRN, Abdul Aziz MJ (2017) Experimental evaluation of FOC of 3-phase IM under open-phase fault. Int J Electron 104(10):1675–1688

    Article  Google Scholar 

  11. Jannati M, Idris NRN (2017) Vector control of unbalanced 3-phase IM using forward and backward components. Turk J Electr Eng Comput Sci 25(2):1358–1374

    Article  Google Scholar 

  12. Abbasi H, Ghanbari M, Ebrahimi R, Jannati M (2020) IRFOC of induction motor drives under open-phase fault using balanced and unbalanced transformation matrices. IEEE Trans Ind Electron 68(10):9160–9173

    Article  Google Scholar 

  13. Sayed-Ahmed A, Mirafzal B, Demerdash NA (2010) Fault-tolerant technique for Δ-connected AC-motor drives. IEEE Trans Energy Convers 26(2):646–653

    Article  Google Scholar 

  14. Sayed-Ahmed A, Demerdash NA (2011) Fault-tolerant operation of delta-connected scalar-and vector-controlled AC motor drives. IEEE Trans Power Electron 27(6):3041–3049

    Article  Google Scholar 

  15. Tabasian R, Ghanbari M, Esmaeli A, Jannati M (2021) A novel direct field-oriented control strategy for fault-tolerant control of induction machine drives based on EKF. IET Electr Power Appl 15(8):979–997

    Article  Google Scholar 

  16. Nikpayam M, Ghanbari M, Esmaeli A, Jannati M (2020) Fault-tolerant control of Y-connected three-phase induction motor drives without speed measurement. Measurement 149:1–14

    Article  Google Scholar 

  17. Tabasian R, Ghanbari M, Esmaeli A, Jannati M (2022) A novel speed control strategy for 3-phase induction motor drives with star-connected under single-phase open-circuit fault using modified RFOC strategy. ISA Trans 125:492–513

    Article  Google Scholar 

  18. Wang W, Zhang J, Cheng M (2016) Common model predictive control for permanent-magnet synchronous machine drives considering single-phase open-circuit fault. IEEE Trans Power Electron 32(7):5862–5872

    Article  Google Scholar 

  19. Gaeta A, Scelba G, Consoli A (2012) Modeling and control of three-phase PMSMs under open-phase fault. IEEE Trans Ind Appl 49(1):74–83

    Article  Google Scholar 

  20. Zhou X, Sun J, Li H, Lu M, Zeng F (2019) PMSM open-phase fault-tolerant control strategy based on four-leg inverter. IEEE Trans Power Electron 35(3):2799–2808

    Article  Google Scholar 

  21. Zhou Y, Lin X, Cheng M (2015) A fault-tolerant direct torque control for six-phase permanent magnet synchronous motor with arbitrary two opened phases based on modified variables. IEEE Trans Energy Convers 31(2):549–556

    Article  Google Scholar 

  22. Zhou H, Zhou C, Tao W, Wang J, Liu G (2019) Virtual-stator-flux-based direct torque control of five-phase fault-tolerant permanent-magnet motor with open-circuit fault. IEEE Trans Power Electron 35(5):5007–5017

    Article  Google Scholar 

  23. de Souza TS, Bastos RR, Cardoso Filho BJ (2020) Synchronous-frame modeling and dq current control of an unbalanced nine-phase induction motor due to open phases. IEEE Trans Ind Appl 56(2):2097–2106

    Article  Google Scholar 

  24. Wang H, Zheng X, Yuan X, Wu X (2022) Enhanced natural fault-tolerant model predictive current control in nine-phase motor drives under open-phase faults. IEEE Trans Energy Convers 37(4):2449–2460

    Article  Google Scholar 

  25. Jin L, Mao Y, Wang X, Shi P, Lu L, Wang Z (2022) Optimization-based maximum-torque fault-tolerant control of dual three-phase PMSM drives under open-phase fault. IEEE Trans Power Electron 38(3):3653–3663

    Article  Google Scholar 

  26. Bermudez M, Gonzalez-Prieto I, Barrero F, Guzman H, Kestelyn X, Duran MJ (2017) An experimental assessment of open-phase fault-tolerant virtual-vector-based direct torque control in five-phase induction motor drives. IEEE Trans Power Electron 33(3):2774–2784

    Article  Google Scholar 

  27. Yepes AG, Abdel-Majeed MS, Che HS, Abdel-Khalik AS, Ahmed S, Doval-Gandoy J (2022) DC-signal injection for stator-resistance estimation in symmetrical six-phase induction motors under open-phase fault. IEEE Trans Ind Electron 70(6):5444–5453

    Article  Google Scholar 

  28. Sun X, Li T, Tian X, Zhu J (2021) Fault-tolerant operation of a six-phase permanent magnet synchronous hub motor based on model predictive current control with virtual voltage vectors. IEEE Trans Energy Convers 37(1):337–346

    Article  Google Scholar 

  29. Sun X, Li T, Zhu Z, Lei G, Guo Y, Zhu J (2021) Speed sensorless model predictive current control based on finite position set for PMSHM drives. IEEE Trans Transport Electrif 7(4):2743–2752

    Article  Google Scholar 

  30. Sun X, Li T, Yao M, Lei G, Guo Y, Zhu J (2021) Improved finite-control-set model predictive control with virtual vectors for PMSHM drives. IEEE Trans Energy Convers 37(3):1885–1894

    Google Scholar 

  31. Li T, Sun X, Lei G, Yang Z, Guo Y, Zhu J (2022) Finite-control-set model predictive control of permanent magnet synchronous motor drive systems—an overview. IEEE/CAA J Autom Sinica 9:2087–2105

    Article  Google Scholar 

  32. Hajary A, Kianinezhad R, Seifossadat SG, Mortazavi SS, Saffarian A (2019) Detection and localization of open-phase fault in three-phase induction motor drives using second order rotational park transformation. IEEE Trans Power Electron 34(11):11241–11252

    Article  Google Scholar 

  33. Demir R (2023) Robust stator flux and load torque estimations for induction motor drives with EKF-based observer. Electr Eng 105(1):551–562

    Article  Google Scholar 

  34. Welchko BA, Lipo TA, Jahns TM, Schulz SE (2004) Fault tolerant three-phase AC motor drive topologies: a comparison of features, cost, and limitations. IEEE Trans Power Electron 19(4):1108–1116

    Article  Google Scholar 

  35. Attaianese C, Monaco MD, Tomasso G (2021) Maximum Torque Per Watt (MTPW) field-oriented control of induction motor. Electr Eng 103:2611–2623

    Article  Google Scholar 

  36. Sen PC (2007) Principles of electric machines and power electronics. John Wiley & Sons, New York

    Google Scholar 

  37. Vas P (1998) Sensorless vector and direct torque control. Oxford Univ Press, Oxford

    Google Scholar 

  38. Pasqualotto D, Rigon S, Zigliotto M (2022) Sensorless speed control of synchronous reluctance motor drives based on extended Kalman filter and neural magnetic model. IEEE Trans Industr Electron 70(2):1321–1330

    Article  Google Scholar 

  39. Yin Z, Li G, Zhang Y, Liu J, Sun X, Zhong Y (2016) A speed and flux observer of induction motor based on extended Kalman filter and Markov chain. IEEE Trans Power Electron 32(9):7096–7117

    Article  Google Scholar 

  40. Alsofyani IM, Idris NRN (2015) Simple flux regulation for improving state estimation at very low and zero speed of a speed sensorless direct torque control of an induction motor. IEEE Trans Power Electron 31(4):3027–3035

    Article  Google Scholar 

Download references

Funding

None.

Author information

Authors and Affiliations

  1. Department of Electrical Engineering, Gorgan Branch, Islamic Azad University, Gorgan, Iran

    Amir Mohamad Taghinezhad Vaskeh Mahaleh, Mahmood Ghanbari & Reza Ebrahimi

Authors
  1. Amir Mohamad Taghinezhad Vaskeh Mahaleh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mahmood Ghanbari
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Reza Ebrahimi
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

Conceptualization: AMTVM, MG, RE, Data curation: AMTVM, Formal analysis: AMTVM, Investigation: AMTVM, MG, RE, Methodology: AMTVM, MG, Project administration: AMTVM, MG, RE, Software: AMTVM, MG, RE, Validation: AMTVM, MG, RE, Visualization: AMTVM, MG, Writing: AMTVM, MG.

Corresponding author

Correspondence to Mahmood Ghanbari.

Ethics declarations

Conflict of interest

The authors declare that there is no conflict of interest.

Ethical approval

This declaration is not applicable.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix A

Using the conventional rotating TM, equations of the M2 can be written as (A1)-(A3):

Stator voltage equations:

$$ \begin{aligned} \left[ \begin{gathered} V_{ds} \hfill \\ V_{qs} \hfill \\ \end{gathered} \right] & = \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {R_{s} + L_{ds} p} & 0 \\ 0 & {R_{s} + L_{qs} p} \\ \end{array} } \right]\left[ {TM^{e} } \right]^{{ - 1}} \left[ \begin{gathered} I_{ds} \hfill \\ I_{qs} \hfill \\ \end{gathered} \right] \\ & \quad + \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {L_{d} p} & 0 \\ 0 & {L_{q} p} \\ \end{array} } \right]\left[ {TM^{e} } \right]^{{ - 1}} \left[ \begin{gathered} I_{dr} \hfill \\ I_{qr} \hfill \\ \end{gathered} \right] \\ & = \left[ {TM^{e} } \right]\left( {\left[ {\begin{array}{*{20}c} {R_{s} } & 0 \\ 0 & {R_{s} } \\ \end{array} } \right] + \left[ {\begin{array}{*{20}c} {L_{ds} p} & 0 \\ 0 & {L_{qs} p} \\ \end{array} } \right]} \right)\left[ {TM^{e} } \right]^{{ - 1}} \left[ \begin{gathered} I_{ds} \hfill \\ I_{qs} \hfill \\ \end{gathered} \right] \\ & \quad + \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {L_{d} } & 0 \\ 0 & {L_{q} } \\ \end{array} } \right]\left( {\left( {p\left[ {TM^{e} } \right]^{{ - 1}} } \right)\left[ \begin{gathered} I_{dr} \hfill \\ I_{qr} \hfill \\ \end{gathered} \right] + \left[ {TM^{e} } \right]^{{ - 1}} \left[ \begin{gathered} pI_{dr} \hfill \\ pI_{qr} \hfill \\ \end{gathered} \right]} \right) \\ & = \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {R_{s} } & 0 \\ 0 & {R_{s} } \\ \end{array} } \right]\left[ {TM^{e} } \right]^{{ - 1}} \left[ \begin{gathered} I_{ds} \hfill \\ I_{qs} \hfill \\ \end{gathered} \right] \\ & \quad + \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {L_{ds} } & 0 \\ 0 & {L_{qs} } \\ \end{array} } \right]\left( {\left( {p\left[ {TM^{e} } \right]^{{ - 1}} \left[ \begin{gathered} I_{ds} \hfill \\ I_{qs} \hfill \\ \end{gathered} \right]} \right) + \left[ {TM^{e} } \right]^{{ - 1}} \left[ \begin{gathered} pI_{ds} \hfill \\ pI_{qs} \hfill \\ \end{gathered} \right]} \right) \\ & \quad + \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {L_{d} } & 0 \\ 0 & {L_{q} } \\ \end{array} } \right]\left( {\left( {p\left[ {TM^{e} } \right]^{{ - 1}} } \right)\left[ \begin{gathered} I_{dr} \hfill \\ I_{qr} \hfill \\ \end{gathered} \right] + \left[ {TM^{e} } \right]^{{ - 1}} \left[ \begin{gathered} pI_{dr} \hfill \\ pI_{qr} \hfill \\ \end{gathered} \right]} \right) \\ & = \underbrace {{\left[ {\begin{array}{*{20}c} {R_{s} + \left( {\frac{{L_{ds} + L_{qs} }}{2}} \right)p} & { - \Omega_{e} \left( {\frac{{L_{ds} + L_{qs} }}{2}} \right)} \\ {\Omega_{e} \left( {\frac{{L_{ds} + L_{qs} }}{2}} \right)} & {R_{s} + \left( {\frac{{L_{ds} + L_{qs} }}{2}} \right)p} \\ \end{array} } \right]\left[ \begin{gathered} I_{ds1} \hfill \\ I_{qs1} \hfill \\ \end{gathered} \right] + \left[ {\begin{array}{*{20}c} {\left( {\frac{{L_{d} + L_{q} }}{2}} \right)p} & { - \Omega_{e} \left( {\frac{{L_{d} + L_{q} }}{2}} \right)} \\ {\Omega_{e} \left( {\frac{{L_{d} + L_{q} }}{2}} \right)} & {\left( {\frac{{L_{d} + L_{q} }}{2}} \right)p} \\ \end{array} } \right]\left[ \begin{gathered} I_{dr1} \hfill \\ I_{qr1} \hfill \\ \end{gathered} \right]}}_{{\left[ \begin{subarray}{l} V_{ds1} \\ V_{qs1} \end{subarray} \right]}} \\ & \quad + \underbrace {{\left[ {\begin{array}{*{20}c} {\left( {\frac{{L_{ds} - L_{qs} }}{2}} \right)p} & {\Omega_{e} \left( {\frac{{L_{ds} - L_{qs} }}{2}} \right)} \\ {\Omega_{e} \left( {\frac{{L_{ds} - L_{qs} }}{2}} \right)} & { - \left( {\frac{{L_{ds} - L_{qs} }}{2}} \right)p} \\ \end{array} } \right]\left[ \begin{gathered} I_{ds2} \hfill \\ I_{qs2} \hfill \\ \end{gathered} \right] + \left[ {\begin{array}{*{20}c} {\left( {\frac{{L_{d} - L_{q} }}{2}} \right)p} & {\Omega_{e} \left( {\frac{{L_{d} - L_{q} }}{2}} \right)} \\ {\Omega_{e} \left( {\frac{{L_{d} - L_{q} }}{2}} \right)} & { - \left( {\frac{{L_{d} - L_{q} }}{2}} \right)p} \\ \end{array} } \right]\left[ \begin{gathered} I_{dr2} \hfill \\ I_{qr2} \hfill \\ \end{gathered} \right]}}_{{\left[ \begin{subarray}{l} V_{ds2} \\ V_{qs2} \end{subarray} \right]}} \\ \end{aligned} $$
(29)

Rotor voltage equations:

$$ \begin{aligned} \left[ \begin{gathered} V_{\alpha r} \hfill \\ V_{\beta r} \hfill \\ \end{gathered} \right] & = \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {L_{d} p} & {\Omega_{r} L_{q} } \\ { - \Omega_{r} L_{d} } & {L_{q} p} \\ \end{array} } \right]\left[ {TM^{e} } \right]^{ - 1} \left[ \begin{gathered} I_{ds} \hfill \\ I_{qs} \\ \end{gathered} \right] + \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {R_{r} + L_{r} p} & {\Omega_{r} L_{r} } \\ { - \Omega_{r} L_{r} } & {R_{r} + L_{r} p} \\ \end{array} } \right]\left[ {TM^{e} } \right]^{ - 1} \left[ \begin{gathered} I_{dr} \hfill \\ I_{qr} \hfill \\ \end{gathered} \right] \\ & = \left[ {TM^{e} } \right]\left( {\left[ {\begin{array}{*{20}c} {L_{d} p} & 0 \\ 0 & {L_{q} p} \\ \end{array} } \right] + \left[ {\begin{array}{*{20}c} 0 & {\Omega_{r} L_{q} } \\ { - \Omega_{r} L_{d} } & 0 \\ \end{array} } \right]} \right)\left[ {TM^{e} } \right]^{ - 1} \left[ \begin{gathered} I_{ds} \hfill \\ I_{qs} \hfill \\ \end{gathered} \right] + \left[ {TM^{e} } \right]\left( {\left[ {\begin{array}{*{20}c} {R_{r} } & {\Omega_{r} L_{r} } \\ { - \Omega_{r} L_{r} } & {R_{r} } \\ \end{array} } \right] + \left[ {\begin{array}{*{20}c} {L_{r} p} & 0 \\ 0 & {L_{r} p} \\ \end{array} } \right]} \right)\left[ {TM^{e} } \right]^{ - 1} \left[ \begin{gathered} I_{dr} \hfill \\ I_{qr} \hfill \\ \end{gathered} \right] \\ & = \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {L_{d} } & 0 \\ 0 & {L_{q} } \\ \end{array} } \right]\left( {\left( {p\left[ {TM^{e} } \right]^{ - 1} } \right)\left[ \begin{gathered} I_{ds} \hfill \\ I_{qs} \hfill \\ \end{gathered} \right] + \left[ {TM^{e} } \right]^{ - 1} \left[ \begin{gathered} pI_{ds} \hfill \\ pI_{qs} \hfill \\ \end{gathered} \right]} \right) + \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} 0 & {\Omega_{r} L_{q} } \\ { - \Omega_{r} L_{d} } & 0 \\ \end{array} } \right]\left[ {TM^{e} } \right]^{ - 1} \left[ \begin{gathered} I_{ds} \hfill \\ I_{qs} \hfill \\ \end{gathered} \right] + \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {R_{r} } & {\Omega_{r} L_{r} } \\ { - \Omega_{r} L_{r} } & {R_{r} } \\ \end{array} } \right]\left[ {TM^{e} } \right]^{ - 1} \left[ \begin{gathered} I_{dr} \hfill \\ I_{qr} \hfill \\ \end{gathered} \right] \\ & \quad + \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {L_{r} } & 0 \\ 0 & {L_{r} } \\ \end{array} } \right]\left( {\left( {p\left[ {TM^{e} } \right]^{ - 1} } \right)\left[ \begin{gathered} I_{dr} \hfill \\ I_{qr} \hfill \\ \end{gathered} \right] + \left[ {TM^{e} } \right]^{ - 1} \left[ \begin{gathered} pI_{dr} \hfill \\ pI_{qr} \hfill \\ \end{gathered} \right]} \right) \\ & = \underbrace {{\left[ {\begin{array}{*{20}c} {\left( {\frac{{L_{d} + L_{q} }}{2}} \right)p} & { - \left( {\Omega_{e} - \Omega_{r} } \right)\left( {\frac{{L_{d} + L_{q} }}{2}} \right)} \\ {\left( {\Omega_{e} - \Omega_{r} } \right)\left( {\frac{{L_{d} + L_{q} }}{2}} \right)} & {\left( {\frac{{L_{d} + L_{q} }}{2}} \right)p} \\ \end{array} } \right]\left[ \begin{gathered} I_{ds1} \hfill \\ I_{qs1} \hfill \\ \end{gathered} \right] + \left[ {\begin{array}{*{20}c} {R_{r} + L_{r} p} & { - \left( {\Omega_{e} - \Omega_{r} } \right)L_{r} } \\ {\left( {\Omega_{e} - \Omega_{r} } \right)L_{r} } & {R_{r} + L_{r} p} \\ \end{array} } \right]\left[ \begin{gathered} I_{dr1} \hfill \\ I_{qr1} \hfill \\ \end{gathered} \right]}}_{{\left[ \begin{subarray}{l} V_{dr1} \\ V_{qr1} \end{subarray} \right]}} \\ & \quad + \underbrace {{\left[ {\begin{array}{*{20}c} {\left( {\frac{{L_{d} - L_{q} }}{2}} \right)p} & {\left( {\Omega_{e} - \Omega_{r} } \right)\left( {\frac{{L_{d} - L_{q} }}{2}} \right)} \\ {\left( {\Omega_{e} - \Omega_{r} } \right)\left( {\frac{{L_{d} - L_{q} }}{2}} \right)} & { - \left( {\frac{{L_{d} - L_{q} }}{2}} \right)p} \\ \end{array} } \right]\left[ \begin{gathered} I_{ds2} \hfill \\ I_{qs2} \hfill \\ \end{gathered} \right]}}_{{\left[ \begin{subarray}{l} V_{dr2} \\ V_{qr2} \end{subarray} \right]}} \\ \end{aligned} $$
(30)

Rotor flux equations:

$$ \begin{aligned} \left[ {\begin{array}{*{20}c} {\Psi_{dr} } \\ {\Psi_{qr} } \\ \end{array} } \right] & = \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {L_{d} } & 0 \\ 0 & {L_{q} } \\ \end{array} } \right]\left[ {TM^{e} } \right]^{{ - 1}} \left[ \begin{gathered} I_{ds} \hfill \\ I_{qs} \hfill \\ \end{gathered} \right] \\ & \quad + \left[ {TM^{e} } \right]\left[ {\begin{array}{*{20}c} {L_{r} } & 0 \\ 0 & {L_{r} } \\ \end{array} } \right]\left[ {TM^{e} } \right]^{ - 1} \left[ \begin{gathered} I_{dr} \hfill \\ I_{qr} \hfill \\ \end{gathered} \right] \\ & = \underbrace {{\left[ {\begin{array}{*{20}c} {\left( {\frac{{L_{d} + L_{q} }}{2}} \right)} & 0 \\ 0 & {\left( {\frac{{L_{d} + L_{q} }}{2}} \right)} \\ \end{array} } \right]\left[ \begin{gathered} I_{ds1} \hfill \\ I_{qs1} \hfill \\ \end{gathered} \right] + \left[ {\begin{array}{*{20}c} {L_{r} } & 0 \\ 0 & {L_{r} } \\ \end{array} } \right]\left[ \begin{gathered} I_{dr1} \hfill \\ I_{qr1} \hfill \\ \end{gathered} \right]}}_{{\left[ {\begin{array}{*{20}c} {\Psi_{dr1} } \\ {\Psi_{qr1} } \\ \end{array} } \right]}} \\ & \quad + \underbrace {{\left[ {\begin{array}{*{20}c} {\left( {\frac{{L_{d} - L_{q} }}{2}} \right)} & 0 \\ 0 & { - \left( {\frac{{L_{d} - L_{q} }}{2}} \right)} \\ \end{array} } \right]\left[ \begin{gathered} I_{ds2} \hfill \\ I_{qs2} \hfill \\ \end{gathered} \right]}}_{{\left[ {\begin{array}{*{20}c} {\Psi_{dr2} } \\ {\Psi_{qr2} } \\ \end{array} } \right]}} \\ \end{aligned} $$
(31)

Appendix B

The recursive EKF equations are as (32–36):

$$ \hat{X}_{k + 1/k} = A_{k} \hat{X}_{k/k} + B_{k} U_{k} $$
(32)
$$ P_{k + 1/k} = F_{k} P_{k/k} F_{k} + Q $$
(33)
$$ K_{k + 1} = HP_{k + 1/k} \left( {HP_{k + 1/k} H^{T} + R} \right)^{ - 1} $$
(34)
$$ \hat{X}_{k + 1/k + 1} = \hat{X}_{k + 1/k} + K_{k + 1} \left( {Y_{k} - HX_{k + 1/k} } \right) $$
(35)
$$ P_{k + 1/k + 1} = \left( {I - K_{k + 1} H} \right)P_{k + 1/k} $$
(36)

Appendix C

The EKF formulation in Fig. 8 is according to (32–36) and the healthy motor equations, where \(I_{\alpha s} ,I_{\beta s} ,\Psi_{\alpha r} ,\Psi_{\beta r} ,\Omega_{r}\) are considered as state variables. Based on the healthy motor equations the following equations can be written:

$$ \begin{aligned} \mathop {\left[ {\begin{array}{*{20}c} {pI_{\alpha s} } \\ {pI_{\beta s} } \\ {p\Psi_{\alpha r} } \\ {p\Psi_{\beta r} } \\ {p\Omega_{r} } \\ \end{array} } \right]}\limits^{pX} & = \mathop {\left[ {\begin{array}{*{20}c} { - \frac{{L_{r} }}{{L_{s} L_{r} - L^{2} }}\left( {R_{s} + \frac{{R_{r} L^{2} }}{{L_{r}^{2} }}} \right)} & 0 & {\frac{{R_{r} L}}{{L_{s} L_{r}^{2} - L^{2} L_{r} }}} & {\frac{{\Omega_{r} L}}{{L_{s} L_{r} - L^{2} }}} & 0 \\ 0 & { - \frac{{L_{r} }}{{L_{s} L_{r} - L^{2} }}\left( {R_{s} + \frac{{R_{r} L^{2} }}{{L_{r}^{2} }}} \right)} & { - \frac{{\Omega_{r} L}}{{L_{s} L_{r} - L^{2} }}} & {\frac{{R_{r} L}}{{L_{s} L_{r}^{2} - L^{2} L_{r} }}} & 0 \\ {\frac{{R_{r} L}}{{L_{r} }}} & 0 & { - \frac{{R_{r} }}{{L_{r} }}} & { - \Omega_{r} } & 0 \\ 0 & {\frac{{R_{r} L}}{{L_{r} }}} & {\Omega_{r} } & { - \frac{{R_{r} }}{{L_{r} }}} & 0 \\ 0 & 0 & 0 & 0 & 0 \\ \end{array} } \right]}\limits^{A} \mathop {\left[ {\begin{array}{*{20}c} {I_{\alpha s} } \\ {I_{\beta s} } \\ {\Psi_{\alpha r} } \\ {\Psi_{\beta r} } \\ {\Omega_{r} } \\ \end{array} } \right]}\limits^{X} \\ & \quad + \mathop {\left[ {\begin{array}{*{20}c} {\frac{{L_{r} }}{{L_{s} L_{r} - L^{2} }}} & 0 \\ 0 & {\frac{{L_{r} }}{{L_{s} L_{r} - L^{2} }}} \\ 0 & 0 \\ 0 & 0 \\ 0 & 0 \\ \end{array} } \right]}\limits^{B} \mathop {\left[ {\begin{array}{*{20}c} {V_{\alpha s} } \\ {V_{\beta s} } \\ \end{array} } \right]}\limits^{U} + W\left( t \right) \\ \end{aligned} $$
(37)

In this case, the discrete Jacobian and measurement matrices should be achieved in each sampling time as (38) and (39):

$$ F\left( k \right) = \left[ {\begin{array}{*{20}c} {1 - \frac{{L_{r} }}{{L_{s} L_{r} - L^{2} }}\left( {R_{s} + \frac{{R_{r} L^{2} }}{{L_{r}^{2} }}} \right)T_{s} } & 0 & {\frac{{R_{r} L}}{{L_{s} L_{r}^{2} - L^{2} L_{r} }}T_{s} } & {\frac{{\Omega_{r} L}}{{L_{s} L_{r} - L^{2} }}T_{s} } & 0 \\ 0 & {1 - \frac{{L_{r} }}{{L_{s} L_{r} - L^{2} }}\left( {R_{s} + \frac{{R_{r} L^{2} }}{{L_{r}^{2} }}} \right)T_{s} } & { - \frac{{\Omega_{r} L}}{{L_{s} L_{r} - L^{2} }}T_{s} } & {\frac{{R_{r} L}}{{L_{s} L_{r}^{2} - L^{2} L_{r} }}T_{s} } & 0 \\ {\frac{{R_{r} L}}{{L_{r} }}T_{s} } & 0 & {1 - \frac{{R_{r} }}{{L_{r} }}T_{s} } & { - \Omega_{r} T_{s} } & 0 \\ 0 & {\frac{{R_{r} L}}{{L_{r} }}T_{s} } & {\Omega_{r} T_{s} } & {1 - \frac{{R_{r} }}{{L_{r} }}T_{s} } & 0 \\ 0 & 0 & 0 & 0 & 1 \\ \end{array} } \right] $$
(38)
$$ H\left( k \right) = \left[ {\begin{array}{*{20}c} 1 & 0 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 & 0 \\ \end{array} } \right] $$
(39)

Based on the above equations, the rotor flux amplitude and angle of the healthy motor can be achieved as (27) and (28).

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Taghinezhad Vaskeh Mahaleh, A.M., Ghanbari, M. & Ebrahimi, R. Direct vector control strategy of 2-phase induction motor drives based on the conventional rotating transformation matrix and EKF. Electr Eng (2023). https://doi.org/10.1007/s00202-023-02063-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00202-023-02063-3

Keywords

Search

Navigation