On the impact of spatial heterogeneity and drift rate in a three-patch two-species Lotka–Volterra competition model over a stream

Abstract

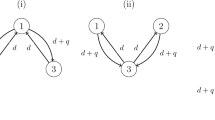
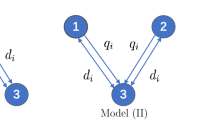
In this paper, we study a three-patch two-species Lotka–Volterra competition patch model over a stream network. The individuals are subject to both random and directed movements, and the two species are assumed to be identical except for the movement rates. The environment is heterogeneous, and the carrying capacity is lager in upstream locations. We treat one species as a resident species and investigate whether the other species can invade or not. Our results show that the spatial heterogeneity of environment and the magnitude of the drift rates have a large impact on the competition outcomes of the stream species.

Appendix

In the Appendix, we study the relations of ${{\overline{q}}}$, ${\underline{q}}$ and $q_0$. For convenience, we recall the definition of ${{\overline{q}}}$, ${\underline{q}}$ and $q_0$:

$$\begin{aligned}&{{\overline{q}}}=\max \left\{ \displaystyle \frac{r_1}{k_1}(k_1-k_2),\displaystyle \frac{r_3}{k_3}(k_2-k_3)\right\} ,\end{aligned}$$

(6.9a)

$$\begin{aligned}&{\underline{q}}=\min \left\{ \displaystyle \frac{r_1}{k_1}(k_1-k_2),\displaystyle \frac{r_3}{k_3}(k_2-k_3)\right\} ,\end{aligned}$$

(6.9b)

$$\begin{aligned}&q_0=\max \left\{ r_1\left( 1-\frac{u_1^*}{k_1}\right) , r_2\left( 1-\frac{u_2^*}{k_2}\right) \right\} . \end{aligned}$$

(6.9c)

Lemma 6.4

Suppose that $(\textbf{H})$ holds, $\varvec{r}\gg \varvec{0}$, and $d_1,q_1>0$. Then, the following statements hold:

• (i) If $q_1<{\underline{q}}$, then $q_0>q_1$;

• (ii) If $q_1>{{\overline{q}}}$, then $q_0<q_1$;

• (iii) If $q_1>{\underline{q}}$, then $q_0>{\underline{q}}$;

• (iv) If $q_1<{{\overline{q}}}$, then $q_0<{{\overline{q}}}$.

Proof

By (5.10) and (5.11) and Lemma 5.1 (i), we have

$$\begin{aligned}&{{\tilde{f}}}_1=d_1u_{2}^*-(d_1+q_1)u_1^*=-r_1u_1^*\left( 1-\frac{u_1^*}{k_1}\right) <0,\end{aligned}$$

(6.10a)

$$\begin{aligned}&{{\tilde{f}}}_2=d_1u_3^*-(d_2+q_2)u_2^*=r_3\left( 1-\frac{u_3^*}{k_3}\right) <0,\end{aligned}$$

(6.10b)

$$\begin{aligned}&{{\tilde{f}}}_2-{{\tilde{f}}}_1=-r_2u_2^*\left( 1-\frac{u_2^*}{k_2}\right) , \end{aligned}$$

(6.10c)

which will be used in the proof below.

(i) By Lemma 5.1 (iv), we have $u^*_1>u^*_2>u^*_3$. This, together with (6.9c) and (6.10a), implies that

$$\begin{aligned} q_0\ge r_1\left( 1-\frac{u_1^*}{k_1}\right) =\frac{(d_1+q_1)u^*_1-d_1u^*_2}{u^*_1}=d_1\left( 1-\frac{u^*_2}{u^*_1}\right) +q_1>q_1. \end{aligned}$$

(6.11)

(ii) By Lemma 5.1 (iii), we have $u^*_1<u^*_2<u^*_3$. Then, by (6.10a) again, we obtain

$$\begin{aligned} r_1\left( 1-\frac{u_1^*}{k_1}\right) =d_1\left( 1-\frac{u^*_2}{u^*_1}\right) +q_1<q_1. \end{aligned}$$

(6.12)

By (6.10c), we obtain that

$$\begin{aligned} \begin{aligned} r_2\left( 1-\frac{u_2^*}{k_2}\right) =&\displaystyle \frac{\left( (d_1+q_1)u^*_2-d_1u_3^*\right) +{{\tilde{f}}}_1}{u^*_2}\\ =&d_1\left( 1-\frac{u^*_3}{u^*_2}\right) +\frac{{{\tilde{f}}}_1}{u^*_2}+q_1<q_1, \end{aligned} \end{aligned}$$

(6.13)

where we have used (6.10c) and $u_2^*<u_3^*$ in the last step. It follows from (6.9c), (6.12) and (6.13) that $q_0<q_1$.

(iii) We divide the proof into three cases:

$$\begin{aligned} (\textrm{A1})\;\;u_1^*<k_2, \;\;(\textrm{A2})\;\; u^*_1\ge u^*_2,\;\;(\textrm{A3})\;\; k_2\le u_1^*<u_2^*. \end{aligned}$$

For case (A1), we see from (6.9b) and (6.9c) that

$$\begin{aligned} q_0\ge r_1\left( 1-\frac{u_1^*}{k_1}\right) >\displaystyle \frac{r_1}{k_1}(k_1-k_2)\ge {\underline{q}}. \end{aligned}$$

For case (A2), we see from (6.9c) and (6.10a) that

$$\begin{aligned} q_0\ge r_1\left( 1-\frac{u_1^*}{k_1}\right) =d_1\left( 1-\frac{u^*_2}{u^*_1}\right) +q_1\ge q_1>{\underline{q}}. \end{aligned}$$

Now we consider (A3). Suppose to the contrary that $q_0\le {\underline{q}}$. This, combined with (6.9b) and (6.9c), yields

$$\begin{aligned} r_1\left( 1-\frac{u_1^*}{k_1}\right) \le q_0\le {\underline{q}}\le \frac{r_3}{k_3}\left( k_2-k_3\right) . \end{aligned}$$

(6.14)

Noticing that $u_2^*>k_2$, we see from (6.10c) that

$$\begin{aligned} {{\tilde{f}}}_2-{{\tilde{f}}}_1=d_1(u^*_3-u^*_2)-(d_1+q_1)(u^*_2-u^*_1)=-r_2u^*_2\left( 1-\displaystyle \frac{u^*_2}{k_2}\right) >0. \end{aligned}$$

(6.15)

Since $u^*_1< u^*_2$, we see from (6.15) that $u^*_2< u^*_3$. Then, we have

$$\begin{aligned} 0>{{\tilde{f}}}_2>{{\tilde{f}}}_1\;\;\text {and}\;\;k_2\le u^*_1< u^*_2< u^*_3, \end{aligned}$$

(6.16)

which yields

$$\begin{aligned} -\displaystyle \frac{{{\tilde{f}}}_2}{u^*_3}<-\displaystyle \frac{{{\tilde{f}}}_1}{u^*_1}. \end{aligned}$$

(6.17)

This, together with (6.16), (6.10a) and (6.10b), implies that

$$\begin{aligned} r_1\left( 1-\frac{u^*_1}{k_1}\right) =-\displaystyle \frac{{{\tilde{f}}}_1}{u^*_1}>-\displaystyle \frac{{{\tilde{f}}}_2}{u^*_3}=\displaystyle \frac{r_3}{k_3}\left( u^*_3-k_3\right) >\displaystyle \frac{r_3}{k_3}\left( k_2-k_3\right) , \end{aligned}$$

which contradicts (6.14). Therefore, $q_0>{\underline{q}}$ for case (A3).

(iv) We first show that

$$\begin{aligned} r_1\left( 1-\frac{u_1^*}{k_1}\right) <{{\overline{q}}}, \end{aligned}$$

(6.18)

and the proof is divided into three cases:

$$\begin{aligned} (\textrm{B1})\;\;u^*_1> k_2,\;\;(\textrm{B2})\;\;u_1^*\le u_2^*,\;\;(\textrm{B3})\;\; k_2\ge u^*_1> u^*_2. \end{aligned}$$

For case (B1), we have

$$\begin{aligned} r_1\left( 1-\frac{u_1^*}{k_1}\right) <\frac{r_1}{k_1}(k_1-k_2)\le {{\overline{q}}}. \end{aligned}$$

For case (B2), we see from (6.10a) that

$$\begin{aligned} r_1\left( 1-\frac{u_1^*}{k_1}\right) =d_1\left( 1-\frac{u^*_2}{u^*_1}\right) +q_1\le q_1<{{\overline{q}}}. \end{aligned}$$

(6.19)

For case (B3), using similar arguments as the above case (A3), we have

$$\begin{aligned} 0>{{\tilde{f}}}_1>{{\tilde{f}}}_2\;\;\text {and}\;\;k_2\ge u^*_1> u^*_2> u^*_3. \end{aligned}$$

This, combined with (6.10a) and (6.10b), implies that

$$\begin{aligned} r_1\left( 1-\frac{u_1^*}{k_1}\right) =-\frac{{{\tilde{f}}}_1}{u^*_1}<-\frac{{{\tilde{f}}}_2}{u^*_1}<-\frac{{{\tilde{f}}}_2}{u^*_3} =r_3\left( \frac{u_3^*}{k_3}-1\right) <\frac{r_3}{k_3}(k_2-k_3)\le {{\overline{q}}}. \end{aligned}$$

Then, we show that

$$\begin{aligned} r_2\left( 1-\frac{u_2^*}{k_2}\right) <{{\overline{q}}}, \end{aligned}$$

(6.20)

and the proof is also divided into three cases:

$$\begin{aligned} (\textrm{C1})\;\;u^*_2\le u_3^*,\;\;(\textrm{C2})\;\;u_2^*> u_3^*\ge k_2,\;\;(\textrm{C3})\;\; u_2^*> u_3^*\;\;\text {and}\;\;k_2>u_3^*. \end{aligned}$$

For case (C1), we see from (6.13) that

$$\begin{aligned} r_2\left( 1-\frac{u_2^*}{k_2}\right)<q_1<{{\overline{q}}}. \end{aligned}$$

For case (C2), we have

$$\begin{aligned} r_2\left( 1-\frac{u_2^*}{k_2}\right)<0<{{\overline{q}}}. \end{aligned}$$

For case (C3), we see from (6.10) that

$$\begin{aligned} r_2\left( 1-\frac{u_2^*}{k_2}\right) =\frac{{{\tilde{f}}}_1-{{\tilde{f}}}_2}{u^*_2}<-\frac{{{\tilde{f}}}_2}{u^*_2}<-\frac{{{\tilde{f}}}_2}{u^*_3} =\frac{r_3}{k_3}\left( u^*_3-k_3\right) <\frac{r_3}{k_3}\left( k_2-k_3\right) \le {{\overline{q}}}. \end{aligned}$$

By (6.18) and (6.20), we see that (iv) holds. $\square$

Remark 6.5

By ${\underline{q}}\le {{\overline{q}}}$ and Lemma 6.4, we see that if $q_1< {\underline{q}}$, then $q_1<q_0<{{\overline{q}}}$; if $q_1> {{\overline{q}}}$, then ${\underline{q}}<q_0<q_1$; and if ${\underline{q}}<q_1<{{\overline{q}}}$, then ${\underline{q}}<q_0<{{\overline{q}}}$.