Skip to main content
SpringerLink
Log in

The spectrum of the Laplacian in a domain bounded by a flexible polyhedron in \(\mathbb R^d\) does not always remain unaltered during the flex

  • Published:
Journal of Geometry Aims and scope Submit manuscript

Abstract

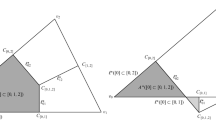
Being motivated by the theory of flexible polyhedra, we study the Dirichlet and Neumann eigenvalues for the Laplace operator in special bounded domains of Euclidean d-space. The boundary of such a domain is an embedded simplicial complex which allows a continuous deformation (a flex), under which each simplex of the complex moves as a solid body and the change in the spatial shape of the domain is achieved through a change of the dihedral angles only. The main result of this article is that both the Dirichlet and Neumann spectra of the Laplace operator in such a domain do not necessarily remain unaltered during the flex of its boundary.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Notes

  1. We use uppercase letters to indicate specially choosen \((d-2)\)-dimensional faces, i.e., faces which will appear again in subsequent constructions; and we use lowercase letters to indicate “arbitrary” \((d-2)\)-dimensional faces.

References

  1. Alexander, R.: Lipschitzian mappings and total mean curvature of polyhedral surfaces. I. Trans. Am. Math. Soc. 288, 661–678 (1985). https://doi.org/10.2307/1999957

    Article  MathSciNet  MATH  Google Scholar 

  2. Alexandrov, V.: Flexible polyhedra in Minkowski 3-space. Manuscr. Math. 111(3), 341–356 (2003). https://doi.org/10.1007/s00229-003-0375-3

    Article  MathSciNet  MATH  Google Scholar 

  3. Alexandrov, V.: The Dehn invariants of the Bricard octahedra. J. Geom. 99(1–2), 1–13 (2010). https://doi.org/10.1007/s00022-011-0061-7

    Article  MathSciNet  MATH  Google Scholar 

  4. Arendt, W., Nittka, R., Peter, W., Steiner, F.: Weyl’s law: spectral properties of the Laplacian in mathematics and physics. In: W. Arendt (ed.) et al. Mathematical Analysis of Evolution, Information, and Complexity, pp. 1–71. Wiley-VCH, Weinheim (2009)

  5. Connelly, R.: A counterexample to the rigidity conjecture for polyhedra. Publ. Math. Inst. Hautes Étud. Sci. 47, 333–338 (1977). https://doi.org/10.1007/BF02684342

    Article  MathSciNet  MATH  Google Scholar 

  6. Connelly, R.: Conjectures and open questions in rigidity. In: Proceedings of International Congress on Mathematics, Helsinki 1978, vol. 1, pp. 407–414 (1980)

  7. Connelly, R., Sabitov, I., Walz, A.: The Bellows conjecture. Beitr. Algebra Geom. 38(1), 1–10 (1997)

    MathSciNet  MATH  Google Scholar 

  8. Demaine, E., O’Rourke, J.: Geometric Folding Algorithms. Linkages, Origami, Polyhedra. Cambridge University Press, Cambridge (2007)

    MATH  Google Scholar 

  9. Fedosov, B.V.: Asymptotic formulas for the eigenvalues of the Laplacian in the case of a polygonal region. Sov. Math. Dokl. 4, 1092–1096 (1963)

    MATH  Google Scholar 

  10. Fedosov, B.V.: Asymptotic formulas for the eigenvalues of the Laplace operator in the case of a polyhedron. Sov. Math. Dokl. 5, 988–990 (1964)

    MATH  Google Scholar 

  11. Gaifullin, A.A.: Flexible cross-polytopes in spaces of constant curvature. Proc. Steklov Inst. Math. 286, 77–113 (2014). https://doi.org/10.1134/S0081543814060066

    Article  MathSciNet  MATH  Google Scholar 

  12. Gaifullin, A.A.: Generalization of Sabitov’s theorem to polyhedra of arbitrary dimensions. Discrete Comput. Geom. 52(2), 195–220 (2014). https://doi.org/10.1007/s00454-014-9609-2

    Article  MathSciNet  MATH  Google Scholar 

  13. Gaifullin, A.A.: Sabitov polynomials for volumes of polyhedra in four dimensions. Adv. Math. 252, 586–611 (2014). https://doi.org/10.1016/j.aim.2013.11.005

    Article  MathSciNet  MATH  Google Scholar 

  14. Gaifullin, A.A.: Embedded flexible spherical cross-polytopes with nonconstant volumes. Proc. Steklov Inst. Math. 288, 56–80 (2015). https://doi.org/10.1134/S0081543815010058

    Article  MathSciNet  MATH  Google Scholar 

  15. Gaifullin, A.A.: The analytic continuation of volume and the Bellows conjecture in Lobachevsky spaces. Sb. Math. 206(11), 1564–1609 (2015). https://doi.org/10.1070/SM2015v206n11ABEH004505

    Article  MathSciNet  MATH  Google Scholar 

  16. Gaifullin, A.A.: The bellows conjecture for small flexible polyhedra in non-Euclidean spaces. Mosc. Math. J. 17(2), 269–290 (2017)

    MathSciNet  MATH  Google Scholar 

  17. Gaifullin, A.A.: Flexible polyhedra and their volumes. In: European Congress of Mathematics. In: Proceedings of the 7th ECM (7ECM) Congress, Berlin, Germany, July 18–22, 2016, pp. 63–83. European Mathematical Society (EMS), Zürich (2018)

  18. Gaifullin, A.A., Ignashchenko, L.S.: Dehn invariant and scissors congruence of flexible polyhedra. Proc. Steklov Inst. Math. 302, 130–145 (2018). https://doi.org/10.1134/S0081543818060068

    Article  MathSciNet  MATH  Google Scholar 

  19. Gittins, K., Larson, S.: Asymptotic behaviour of cuboids optimising Laplacian eigenvalues. Integral Equ. Oper. Theory 89(4), 607–629 (2017). https://doi.org/10.1007/s00020-017-2407-5

    Article  MathSciNet  MATH  Google Scholar 

  20. Ivrii, V.: 100 years of Weyl’s law. Bull. Math. Sci. 6(3), 379–452 (2016). https://doi.org/10.1007/s13373-016-0089-y

    Article  MathSciNet  MATH  Google Scholar 

  21. Kuiper, N.: Sphères polyedriques flexibles dans \(E^3\), d’apres Robert Connelly. Seminaire Bourbaki, Vol. 1977/78, Expose No.514, Lect. Notes Math. 710, 147–168 (1979)

  22. Maksimov, I.: Nonflexible polyhedra with a small number of vertices. J. Math. Sci. 149(1), 956–970 (2008). https://doi.org/10.1007/s10958-008-0037-9

    Article  MathSciNet  MATH  Google Scholar 

  23. Mazzeo, R., Rowlett, J.: A heat trace anomaly on polygons. Math. Proc. Camb. Philos. Soc. 159(2), 303–319 (2015). https://doi.org/10.1017/S0305004115000365

    Article  MathSciNet  MATH  Google Scholar 

  24. Netrusov, Yu, Safarov, Yu: Weyl asymptotic formula for the Laplacian on domains with rough boundaries. Commun. Math. Phys. 253(2), 481–509 (2005). https://doi.org/10.1007/s00220-004-1158-8

    Article  MathSciNet  MATH  Google Scholar 

  25. Sabitov, IKh: On the problem of invariance of the volume of a flexible polyhedron. Russ. Math. Surv. 50(2), 451–452 (1995). https://doi.org/10.1070/RM1995v050n02ABEH002095

    Article  MATH  Google Scholar 

  26. Sabitov, IKh: The volume of a polyhedron as a function of its metric. Fundam. Prikl. Mat. 2(4), 1235–1246 (1996)

    MathSciNet  MATH  Google Scholar 

  27. Sabitov, IKh: The volume as a metric invariant of polyhedra. Discrete Comput. Geom. 20(4), 405–425 (1998). https://doi.org/10.1007/PL00009393

    Article  MathSciNet  MATH  Google Scholar 

  28. Santaló, L.: Integral Geometry and Geometric Probability, vol. 1. Cambridge University Press, Cambridge (1976)

    MATH  Google Scholar 

  29. Schlenker, J.-M.: La conjecture des soufflets (d’après I. Sabitov). In: Séminaire Bourbaki. Volume 2002/2003. Exposés 909–923, pp. 77–95, ex. Paris: Société Mathématique de France (2004)

  30. Shtogrin, M.I.: On flexible polyhedral surfaces. Proc. Steklov Inst. Math. 288, 153–164 (2015). https://doi.org/10.1134/S0081543815010125

    Article  MathSciNet  MATH  Google Scholar 

  31. Smith, L.: The asymptotics of the heat equation for a boundary value problem. Invent. Math. 63, 467–493 (1981). https://doi.org/10.1007/BF01389065

    Article  MathSciNet  MATH  Google Scholar 

  32. Stachel, H.: Flexible cross-polytopes in the Euclidean 4-space. J. Geom. Graph. 4(2), 159–167 (2000)

    MathSciNet  MATH  Google Scholar 

  33. Stachel, H.: Flexible octahedra in the hyperbolic space. In: Non-Euclidean Geometries. János Bolyai Memorial Volume. Papers from the International Conference on Hyperbolic Geometry, Budapest, Hungary, July 6–12, 2002, pp. 209–225. Springer, New York (2006)

  34. van den Berg, M., Srisatkunarajah, S.: Heat equation for a region in\(\mathbb{R} ^2\)with a polygonal boundary. J. Lond. Math. Soc. II. Ser. 37(1), 119–127 (1988). https://doi.org/10.1112/jlms/s2-37.121.119

  35. Watson, S.: The trace function expansion for spherical polygons. N. Z. J. Math. 34(1), 81–95 (2005)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The author is grateful to Dr. Evgeniĭ P. Volokitin for assistance in preparation of the figures and to Prof. Alexey Yu. Kokotov for bringing his attention to [35].

Author information

Authors and Affiliations

  1. Sobolev Institute of Mathematics, Koptyug Ave., 4, Novosibirsk, Russia, 630090

    Victor Alexandrov

  2. Department of Physics, Novosibirsk State University, Pirogov Str., 2, Novosibirsk, Russia, 630090

    Victor Alexandrov

Authors
  1. Victor Alexandrov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Victor Alexandrov.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Alexandrov, V. The spectrum of the Laplacian in a domain bounded by a flexible polyhedron in \(\mathbb R^d\) does not always remain unaltered during the flex. J. Geom. 111, 32 (2020). https://doi.org/10.1007/s00022-020-00541-8

Download citation

  • Received:

  • Revised:

  • Published:

  • DOI: https://doi.org/10.1007/s00022-020-00541-8

Keywords

Mathematics Subject Classification

Search

Navigation