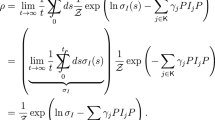Abstract
We study the evolution of probability measures under the action of stationary Markov processes by means of a non-equilibrium entropy defined in terms of a convex function ϕ. We prove that the convergence of the non-equilibrium entropy to zero for all measures of finite entropy is independent of ϕ for a wide class of convex functions, including ϕ0(t)=t log t. We also prove that this is equivalent to the convergence of all the densities of a finite norm to a uniform density, on the Orlicz spaces related to ϕ, which include the L p-spaces for p>1. By means of the quadratic function ϕ2(t)=t 2−1, we relate the non-equlibrium entropies defined by the past σ-algebras of a K-dynamical system with the non-equilibrium entropy of its associated irreversible Markov processes converging to equilibrium.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Neveu, J.: Bases Mathématiques du Calcul des Probabilités, Masson, Paris, 1970.
Neveu, J.: Martingales a temps discret, Masson, Paris, 1972.
Yosida, K.: Functional Analysis, Springer-Verlag, Berlin, 1968.
Brown, J.: Ergodic Theory and Topological Dynamics, Academic Press, New York, 1976.
Sinai, Y.: Introduction to Ergodic Theory, Princeton University Press, Princeton, 1977.
Walters, P.: An Introduction to Ergodic Theory, Springer-Verlag, New York, 1982.
Misra, B., Prigogine, I. and Courbage, M.: ‘From Deterministic Dynamics to Probabilistic Descriptions’, Physica 98A (1979), 1–26.
Courbage, M. and Misra, B.: ‘On the Equivalence Between Bernoulli Dynamical Systems and Stochastic Markov Processes’, Physica 104A (1980), 359–377.
Goldstein, S., Misra, B. and Courbage, M.: ‘On Intrinsic Randomness of Dynamical Systems’, J. Stat. Phys. 25, (1981), 111–126.
Courbage, M. and Prigogine, I.: ‘Intrinsic Randomness and Intrinsic Irreversibility in Classical Dynamical Systems’, Preprint ULB, 1982.
Goldstein, S. and Penrose, O.: ‘A Non-equilibrium Entropy for Dynamical Systems’, J. Stat. Phys. 24 (1981), 325–343.
Goldstein, S.: ‘Entropy Increase in Dynamical Systems’, Israel J. Math. 38, (1981), 241–256.
Martinez, S. and Tirapegui, E.: ‘Description of a Class of Markov Processes “Equivalent” to K-shifts’, to appear in J. Stat. Phys. 36 (1984).
Martinez, S. and Tirapegui, E.: ‘Markov Process and Entropy Associated to a Reversible Evolution’ Phys. Lett. 95A (1983), 143–145.
Martinez, S. and Tirapegui, E.: ‘Entropy Functionals Associated to Shifts Dynamical Systems’, Physica 122A (1983), 592–601.
Martinez, S. and Tirapegui, E.: ‘Irreversible Evolution of Dynamical Systems’, Proc. VII Sitges Conf. on Stat. Mechanics, Sept. 1982, Lecture Notes in Physics, Springer-Verlag, New York.
Author information
Authors and Affiliations
Additional information
Partially supported by DIB Universidad de Chile, E19468412.
Rights and permissions
About this article
Cite this article
Servet Martínez, A. Non-equilibrium entropy on stationary Markov processes. Acta Appl Math 3, 221–238 (1985). https://doi.org/10.1007/BF00047329
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF00047329