Design method of a modified layered aerobic waste landfill divided by coarse material

Abstract

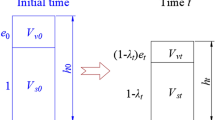
To overcome the weaknesses of traditional landfills, a modified aerobic landfill concept with intermediate covers of coarse material between waste layers functioning as facilities of drainage and aeration has been proposed recently. In this study, a one-dimensional coupled model, including aerobic biodegradation, oxygen diffusion, and advection, is proposed to describe oxygen distribution in this new type of landfill. Homotopy analysis method and perturbation method are applied to solve this model at passive aeration and active aeration, respectively. The model has six input variables, that is, oxygen diffusion coefficient, gas permeability, maximum oxygen consumption rate, layer thickness of waste, and injection pressure and extraction pressure. A combination of their typical values gives rise to over 700,000 scenarios which can be calculated by the proposed solution. The coupled effect of the above variables on oxygen migration is quantitatively investigated, followed by an estimation formula of the minimum oxygen concentration in waste layer. The maximum waste layer thickness is defined as a function of other variables for a given aeration target of oxygen volume concentration larger than 5%. A generalized design method of waste layer thickness, injection pressure, and extraction pressure is then developed for the newly proposed modified layered aerobic landfill, which can promote its popularization and application.

Appendices

Appendix 1. Derivation of the semi-analytical solution at passive aeration

Equation (15) can be rewritten as follows:

$$ \left\{\begin{array}{l}\overline{c}\frac{d^2\overline{c}}{d{\overline{z}}^2}+\frac{d^2\overline{c}}{d{\overline{z}}^2}-{A}_1\overline{c}=0\\ {}{\left.\overline{c}\right|}_{\overline{z}=0}=\overline{c_0}\kern0.5em {\left.\frac{d\overline{c}}{d\overline{z}}\right|}_{\overline{z}=1}=0\end{array}\right. $$

(35)

Let linear operator L and nonlinear operator N be as follows:

$$ {\displaystyle \begin{array}{l}L\left[\varphi \left(\overline{z};q\right)\right]=\frac{\partial^2\varphi \left(\overline{z};q\right)}{\partial {\overline{z}}^2}\\ {}N\left[\varphi \left(\overline{z};q\right)\right]=\varphi \left(\overline{z};q\right)\frac{\partial^2\varphi \left(\overline{z};q\right)}{\partial {\overline{z}}^2}+\frac{\partial^2\varphi \left(\overline{z};q\right)}{\partial {\overline{z}}^2}-{A}_1\varphi \left(\overline{z};q\right)\end{array}} $$

(36)

where q is embedding parameter.

The homotopy H for q∈[0,1] can be defined as follows:

$$ \left\{\begin{array}{l}H\left[\varphi \left(\overline{z};q\right),q\right]=\left(1-q\right)\cdot \varphi \left(\overline{z};q\right)\cdot L\left[\varphi \left(\overline{z};q\right)-\overline{c_0}\right]+ qN\left[\varphi \left(\overline{z};q\right)\right]=0\\ {}{\left.\varphi \left(\overline{z},q\right)\right|}_{\overline{z}=0}=\overline{c_0},\kern0.5em {\left.\frac{\partial \varphi \left(\overline{z},q\right)}{\partial \overline{z}}\right|}_{\overline{z}=1}=0\end{array}\right. $$

(37)

Obviously, Eq. (38) can be obtained from Eq. (37):

$$ \left\{\begin{array}{l}\varphi \left(\overline{z};0\right)=\overline{c_0}\\ {}\varphi \left(\overline{z};1\right)=\overline{c}\left(\overline{z}\right)\end{array}\right. $$

(38)

Thus, as q increases from 0 to 1, the φ varies from the boundary condition of Eq. (35) to the solution of Eq. (35). Expanding φ with Taylor series at q = 0 yields the following:

$$ \varphi \left(\overline{z};q\right)=\varphi \left(\overline{z};0\right)+\sum \limits_{m=1}^{+\infty}\left({\left.\frac{1}{m!}\frac{\partial \varphi \left(\overline{z};q\right)}{\partial q}\right|}_{q=0}{q}^m\right)=\overline{c_0}+\sum \limits_{m=1}^{+\infty}\overline{c_m}\left(\overline{z}\right){q}^m $$

(39)

where

$$ \overline{c_m}\left(\overline{z}\right)={\left.\frac{1}{m!}\frac{\partial \varphi \left(\overline{z};q\right)}{\partial q}\right|}_{q=0} $$

(40)

Substituting Eq. (38) into Eq. (39) yields the following:

$$ \overline{c}\left(\overline{z}\right)=\varphi \left(\overline{z};1\right)=\overline{c_0}+\sum \limits_{m=1}^{+\infty}\overline{c_m}\left(\overline{z}\right) $$

(41)

Substituting Eq. (39) into Eq. (37) yields the following:

$$ {\displaystyle \begin{array}{l}\left(1-q\right)\cdot \left[{c}_0+\sum \limits_{m=1}^{+\infty}\overline{c_m}{q}^m\right]\cdot \left[\sum \limits_{m=1}^{+\infty}\frac{{\mathrm{d}}^2\overline{c_m}}{\mathrm{d}{\overline{z}}^2}{q}^m\right]=\\ {}-q\left\{\left[{c}_0+\sum \limits_{m=1}^{+\infty}\overline{c_m}{q}^m\right]\cdot \left[\sum \limits_{m=1}^{+\infty}\frac{{\mathrm{d}}^2\overline{c_m}}{\mathrm{d}{\overline{z}}^2}{q}^m\right]+\left[\sum \limits_{m=1}^{+\infty}\frac{{\mathrm{d}}^2\overline{c_m}}{\mathrm{d}{\overline{z}}^2}{q}^m\right]-{A}_1\left[{c}_0+\sum \limits_{m=1}^{+\infty}\overline{c_m}{q}^m\right]\right\}\end{array}} $$

(42)

Rearranging q-terms with the same powers in Eq. (42) yields the following:

$$ {q}^1\kern0.5em {\displaystyle \begin{array}{cc}\overline{c_0}\frac{{\mathrm{d}}^2\overline{c_1}}{\mathrm{d}{\overline{z}}^2}+\overline{c_1}\frac{{\mathrm{d}}^2\overline{c_0}}{\mathrm{d}{\overline{z}}^2}+\frac{{\mathrm{d}}^2\overline{c_0}}{\mathrm{d}{\overline{z}}^2}-{A}_1\overline{c_0}=0& \overline{c_1}(0)=0,{\left.\frac{\mathrm{d}\overline{c_1}}{\mathrm{d}\overline{z}}\right|}_{\overline{z}=1}=0\end{array}} $$

(43a)

$$ {q}^2\kern0.5em {\displaystyle \begin{array}{cc}\overline{c_0}\frac{{\mathrm{d}}^2\overline{c_2}}{\mathrm{d}{\overline{z}}^2}+\overline{c_1}\frac{{\mathrm{d}}^2\overline{c_1}}{\mathrm{d}{\overline{z}}^2}+\overline{c_2}\frac{{\mathrm{d}}^2\overline{c_0}}{\mathrm{d}{\overline{z}}^2}+\frac{{\mathrm{d}}^2\overline{c_1}}{\mathrm{d}{\overline{z}}^2}-{A}_1\overline{c_1}=0& \overline{c_2}(0)=0,{\left.\frac{\mathrm{d}\overline{c_2}}{\mathrm{d}\overline{z}}\right|}_{\overline{z}=1}=0\end{array}} $$

(43b)

$$ {q}^m\kern0.5em {\displaystyle \begin{array}{cc}\sum \limits_{k=0}^m\overline{c_k}\frac{{\mathrm{d}}^2\overline{c_{m-k}}}{\mathrm{d}{\overline{z}}^2}+\frac{{\mathrm{d}}^2\overline{c_{m-1}}}{\mathrm{d}{\overline{z}}^2}-{A}_1\overline{c_{m-1}}=0& \overline{c_m}(0)=0,{\left.\frac{\mathrm{d}\overline{c}}{\mathrm{d}\overline{z}}\right|}_{\overline{z}=1}=0\end{array}} $$

(43c)

Equations (43a)~(43c) are all second-order linear ordinary differential equations and can be converted into the form of y″ = f (x). Thus, the solutions of Eqs. (43a)~(43c) can be obtained by integration:

$$ \overline{c_1}=\frac{A_1}{2}\left({\overline{z}}^2-2\overline{z}\right) $$

(44a)

$$ \overline{c_2}=-\frac{A_1}{2\overline{c_0}}\left({\overline{z}}^2-2\overline{z}\right) $$

(44b)

$$ \overline{c_3}=\frac{A_1}{2{\overline{c_0}}^2}\left[\frac{A_1}{12}{\overline{z}}^4-\frac{A_1}{3}{\overline{z}}^3+{\overline{z}}^2+\left(\frac{2{A}_1}{3}-2\right)\overline{z}\right] $$

(44c)

$$ \overline{c_4}=\left(\frac{A_1}{{\overline{c_0}}^3}-\frac{A_1^2}{{\overline{c_0}}^3}+\frac{2{A}_1^3}{15{\overline{c_0}}^3}\right)\overline{z}-\frac{A_1}{2{\overline{c_0}}^3}{\overline{z}}^2+\frac{A_1^2}{2{\overline{c_0}}^3}{\overline{z}}^3-\frac{2{A}_1^3+3{A}_1^2}{24{\overline{c_0}}^3}{\overline{z}}^4+\frac{A_1^3}{20{\overline{c_0}}^3}{\overline{z}}^5-\frac{A_1^3}{120{{\overline{c}}_0}^3}{\overline{z}}^6 $$

(44d)

…

Standing on Eq. (41) and Eqs. (44a)~(44d), the solution of Eq. (35) with five terms is obtained as follows:

$$ \overline{c}\left(\overline{z}\right)\approx \overline{c_0}+\overline{c_1}+\overline{c_2}+\overline{c_3}+\overline{c_4} $$

(45)

Compared with numerical solutions, the solution of Eq. (35) with five terms can achieve sufficient accuracy.

Appendix 2. Derivation of the semi-analytical solution at active aeration

Standing on Eq. (17), it is obvious that (1 + c*) ranges from − 1 < (1 + c*) ≤ 0. Thus, the nonlinear term 1 / (1 + c*) in Eq. (19) can be expanded with Taylor series at c* = 0:

$$ \frac{1}{c^{\ast }+1}=1-{c}^{\ast }+{c}^{\ast 2}+\cdots =\sum \limits_{n=0}^{\infty }{\left(-1\right)}^n{c}^{\ast n} $$

(46)

If the dimensionless perturbation parameter ε = 0, Eq. (19) can be simplified as follows:

$$ \left\{\begin{array}{l}\frac{d^2{c}^{\ast }}{d{z}^{\ast 2}}-\frac{d{c}^{\ast }}{d{z}^{\ast }}-{A}_2=0\\ {}{\left.{c}^{\ast}\right|}_{z^{\ast }=0}=0,\kern0.5em {\left.\frac{d{c}^{\ast }}{d{z}^{\ast }}\right|}_{z^{\ast }=\beta }=0\end{array}\right. $$

(47)

The general solution of the homogeneous differential equation corresponding to Eq. (47) can be obtained using the characteristic equation. And the special solution of Eq. (47) can be obtained using the method of variation of constants. The sum of these two solutions is the general solution of Eq. (47):

$$ {c}^{\ast }={c}_0^{\ast }={C}_1+{C}_2{e}^{z^{\ast }}-{A}_2{z}^{\ast } $$

(48)

where C₁ and C₂ are constants determined according to the boundary conditions.

Actually, ε is not equal to 0. Thus, the expression of c* should be modified. As ε is far less than 1, c* can be expended as follows:

$$ {c}^{\ast}\left({z}^{\ast}\right)={c}_0^{\ast }+\varepsilon {c}_1^{\ast }+{\varepsilon}^2{c}_2^{\ast }+\cdots $$

(49)

Substituting Eqs. (49) and (46) into Eq. (47) yields the following:

$$ {\displaystyle \begin{array}{l}\frac{d^2{c}_0^{\ast }}{d{z}^{\ast 2}}+\varepsilon \frac{d^2{c}_1^{\ast }}{d{z}^{\ast 2}}+{\varepsilon}^2\frac{d^2{c}_2^{\ast }}{d{z}^{\ast 2}}+\cdots -\frac{d{c}_0^{\ast }}{d{z}^{\ast }}-\varepsilon \frac{d{c}_1^{\ast }}{d{z}^{\ast }}-{\varepsilon}^2\frac{d{c}_2^{\ast }}{d{z}^{\ast }}-\cdots \\ {}-{A}_2+\varepsilon \left[1-\left({c}_0^{\ast }+\varepsilon {c}_1^{\ast }+\cdots \right)+{\left({c}_0^{\ast }+\varepsilon {c}_1^{\ast }+\cdots \right)}^2+\cdots \right]=0\end{array}} $$

(50)

And the boundary conditions are converted into the following:

$$ \left\{\begin{array}{l}{c}^{\ast }(0)={c}_0^{\ast }(0)+\varepsilon {c}_1^{\ast }(0)+{\varepsilon}^2{c}_2^{\ast }(0)+\cdots =0\\ {}{\left.\frac{d{c}^{\ast }}{d\overline{z}}\right|}_{\overline{z}=\beta }={\left.\frac{d{c}_0^{\ast }}{d\overline{z}}\right|}_{\overline{z}=\beta }+\varepsilon {\left.\frac{d{c}_1^{\ast }}{d\overline{z}}\right|}_{\overline{z}=\beta }+{\varepsilon}^2{\left.\frac{d{c}_2^{\ast }}{d\overline{z}}\right|}_{\overline{z}=\beta }+\cdots =0\end{array}\right. $$

(51)

Rearranging ε-terms with the same powers in Eq. (50) yields the following:

$$ {\varepsilon}^0\kern0.5em {\displaystyle \begin{array}{cc}\frac{d^2{c}_0^{\ast }}{d{z}^{\ast 2}}-\frac{d{c}_0^{\ast }}{d{z}^{\ast }}-{A}_2=0& {c}_0^{\ast }=0,{\left.\frac{d{c}_0^{\ast }}{d{z}^{\ast }}\right|}_{z^{\ast }=\beta }=0\end{array}} $$

(52a)

$$ {\varepsilon}^1\kern0.5em {\displaystyle \begin{array}{cc}\frac{d^2{c}_1^{\ast }}{d{z}^{\ast 2}}-\frac{d{c}_1^{\ast }}{d{z}^{\ast }}+1-{c}_0^{\ast }+{c}_0^{\ast 2}=0& {c}_1^{\ast }=0,{\left.\frac{d{c}_1^{\ast }}{d{z}^{\ast }}\right|}_{z^{\ast }=\beta }=0\end{array}} $$

(52b)

$$ {\varepsilon}^2\kern0.5em {\displaystyle \begin{array}{cc}\frac{d^2{c}_2^{\ast }}{d{z}^{\ast 2}}-\frac{d{c}_2^{\ast }}{d{z}^{\ast }}+1-{c}_1^{\ast }+2{c}_0^{\ast }{c}_1^{\ast }=0& {c}_1^{\ast }=0,{\left.\frac{d{c}_1^{\ast }}{d{z}^{\ast }}\right|}_{z^{\ast }=\beta }=0\end{array}} $$

(52c)

…

Substituting the boundary conditions of Eq. (52a) into Eq. (48) yields the general solution of Eq. (52a):

$$ {c}_0^{\ast }=-{A}_2\left({e}^{-\beta }-{e}^{z^{\ast }-\beta }+{z}^{\ast}\right) $$

(53)

The general solution of the homogeneous differential equation corresponding to Eq. (52b) is the following:

$$ {c}_{11}^{\ast }={B}_1+{B}_2{e}^{z^{\ast }} $$

(54)

where B₁ and B₂ are constants determined according to the boundary conditions.

The second-order nonhomogeneous linear ordinary differential equation (Eq. (52b)) can be solved using the method of variation of constants. The special solution of Eq. (52b) can be assumed as follows:

$$ {c}_{12}^{\ast }={C}_1\left({z}^{\ast}\right)+{C}_2\left({z}^{\ast}\right){e}^{z^{\ast }} $$

(55)

where C₁(z*) and C₂(z*) satisfy the following equations:

$$ \left(\begin{array}{cc}1& {e}^{z^{\ast }}\\ {}0& {e}^{z^{\ast }}\end{array}\right)\left(\begin{array}{c}{C_1}^{\prime}\left({z}^{\ast}\right)\\ {}{C_2}^{\prime}\left({z}^{\ast}\right)\end{array}\right)=\left(\begin{array}{c}0\\ {}-{c}_0^{\ast 2}+{c}_0^{\ast }-1\end{array}\right) $$

(56)

The expression of C₁(z*) and C₂(z*) are obtained from solving Eq. (56). Thus, the general solution of Eq. (52b) can be written as follows:

$$ {c}_1^{\ast }={c}_{11}^{\ast }+{c}_{12}^{\ast }={B}_1+{B}_2{e}^{z^{\ast }}+{C}_1\left({z}^{\ast}\right)+{C}_2\left({z}^{\ast}\right){e}^{z^{\ast }} $$

(57)

Substituting the boundary conditions of Eq. (52b) into Eq. (57) yields the general solution of Eq. (52b):

$$ {c}_1^{\ast }={\displaystyle \begin{array}{c}\left[1+\left(1+{e}^{-\beta}\right){A}_2+\left(2+2{e}^{-\beta }+{e}^{-2\beta}\right){z}^{\ast}\right]+\left[\frac{A}{2}+\left({e}^{-\beta }+1\right){A}_2^2\right]{z}^{\ast 2}+\frac{A_2^2}{3}{z}^{\ast 3}\\ {}-{e}^{z^{\ast }-\beta}\left[{A}_2\left(2+2\beta +{e}^{-\beta}\right)+{A}_2^2\left(-1+2\beta +2{\beta}^2+4{e}^{-\beta }+4{\beta e}^{-\beta }+{e}^{-2\beta}\right)+1\right]\\ {}\begin{array}{c}-\frac{A_2^2}{2}{e}^{2{z}^{\ast }-2\beta }+{z}^{\ast }{e}^{z^{\ast }-\beta}\left({A}_2-2{A}_2^2+2{A}_2^2{e}^{-\beta}\right)+{A}_2^2{z}^{\ast 2}{e}^{z^{\ast }-\beta }+{A}_2^2{e}^{-3\beta}\\ {}+{e}^{-\beta}\left(1+2{A}_2+2{A}_2\beta -{A}_2^2+2{A}_2^2\beta +2{A}_2^2{\beta}^2\right)+\left({A}_2+\frac{9}{2}{A}_2^2+4{A}_2^2\beta \right){e}^{-2\beta}\end{array}\end{array}} $$

(58)

Substituting Eqs. (53) and (58) into Eq. (49) yields the first-order perturbation solution of Eq. (18):

$$ {c}^{\ast}\left({z}^{\ast}\right)+\varepsilon {\displaystyle \begin{array}{c}\begin{array}{c}={c}_0^{\ast }+\varepsilon {c}_1^{\ast}\\ {}=-{A}_2\left({e}^{-\beta }-{e}^{z^{\ast }-\beta }+{z}^{\ast}\right)\end{array}\\ {}\left\{\begin{array}{c}\left[1+\left(1+{e}^{-\beta}\right){A}_2+\left(2+2{e}^{-\beta }+{e}^{-2\beta}\right){A}_2^2\right]{z}^{\ast }+\left[\frac{A}{2}+\left({e}^{-\beta }+1\right){A}_2^2\right]{z}^{\ast 2}+\frac{A_2^2}{3}{z}^{\ast 3}\\ {}-{e}^{z^{\ast }-\beta}\left[{A}_2\left(2+2\beta +{e}^{-\beta}\right)+{A}_2^2\left(-1+2\beta +2{\beta}^2+4{e}^{-\beta }+{e}^{-2\beta}\right)+1\right]\\ {}\begin{array}{c}-\frac{A_2^2}{2}{e}^{2{z}^{\ast }-2\beta }+{z}^{\ast }{e}^{z^{\ast }-\beta}\left({A}_2-2{A}_2^2{e}^{-\beta}\right)+{A}_2^2{z}^{\ast 2}{e}^{z^{\ast }-\beta }+{A}_2^2{e}^{-3\beta}\\ {}+{e}^{-\beta}\left(1+2{A}_2+2{A}_2\beta -{A}_2^2+2{A}_2^2\beta +2{A}_2^2{\beta}^2\right)+\left({A}_2+\frac{9}{2}{A}_2^2+4{A}_2^2\beta \right){e}^{-2\beta}\end{array}\end{array}\right.\end{array}} $$

(59)

Compared with numerical solutions, the first-order perturbation solution (Eq. (59)) can achieve sufficient accuracy.