Entropy Signatures of Topological Phase Transitions

Abstract

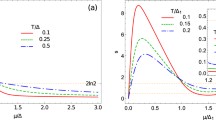
We review the behavior of the entropy per particle in various two-dimensional electronic systems. The entropy per particle is an important characteristic of any many-body system that tells how the entropy of the ensemble of electrons changes if one adds one more electron. Recently, it has been demonstrated how the entropy per particle of a two-dimensional electron gas can be extracted from the recharging current dynamics in a planar capacitor geometry. These experiments pave the way to the systematic studies of entropy in various crystal systems including novel two-dimensional crystals such as gapped graphene, germanene, and silicene. Theoretically, the entropy per particle is linked to the temperature derivative of the chemical potential of the electron gas by the Maxwell relation. Using this relation, we calculate the entropy per particle in the vicinity of topological transitions in various two-dimensional electronic systems. We show that the entropy experiences quantized steps at the points of Lifshitz transitions in a two-dimensional electron gas with a parabolic energy spectrum. In contrast, in doubled-gapped Dirac materials, the entropy per particle demonstrates characteristic spikes once the chemical potential passes through the band edges. The transition from a topological to trivial insulator phase in germanene is manifested by the disappearance of a strong zero-energy resonance in the entropy per particle dependence on the chemical potential. We conclude that studies of the entropy per particle shed light on multiple otherwise hidden peculiarities of the electronic band structure of novel two-dimensional crystals.

Appendices

APPENDIX A

1.1 Derivation of Eqs. (11) and (12)

Using Eqs. (10) and (9), we can cast the derivatives (∂n/∂μ)_T and (∂n/∂T)_μ in the form

$$\begin{gathered} {{\left( {\frac{{\partial n}}{{\partial \mu }}} \right)}_{T}} = \frac{{m{\text{*}}}}{{2\pi {{\hbar }^{2}}}} \\ \times \sum\limits_j^{} {\int\limits_{ - \infty }^\infty {\frac{{dz}}{{{{{\cosh }}^{2}}z}}\left[ {\frac{1}{2} + \frac{1}{\pi }\arctan (az + {{b}_{j}})} \right]} } , \\ \end{gathered} $$

((A.1))

$$\begin{gathered} {{\left( {\frac{{\partial n}}{{\partial T}}} \right)}_{\mu }} = \frac{{m{\text{*}}}}{{\pi {{\hbar }^{2}}}} \\ \times \sum\limits_j^{} {\int\limits_{ - \infty }^\infty {\frac{{zdz}}{{{{{\cosh }}^{2}}z}}\left[ {\frac{1}{2} + \frac{1}{\pi }\arctan (az + {{b}_{j}})} \right]} } , \\ \end{gathered} $$

((A.2))

where a ≡ 2T/γ, b_j ≡ (μ – E_j)/γ. Therefore, one is left with the integrals

$$\begin{gathered} I(a,b) = \int\limits_{ - \infty }^\infty {\frac{{dzz}}{{{{{\cosh }}^{2}}z}}\arctan (az + b),} \\ J(a,b) = \int\limits_{ - \infty }^\infty {\frac{{dz}}{{{{{\cosh }}^{2}}z}}\arctan (az + b),} \\ \end{gathered} $$

((A.3))

where a > 0.

As an example, let us consider the integral I(a, b), which is an even function in b. Clearly,

$$I(a,b \to \infty ) = 0.$$

((A.4))

It is worth evaluating the derivative

$$\frac{{\partial I(a,b)}}{{\partial b}} = \int\limits_{ - \infty }^\infty {\frac{{dx}}{{{{{\cosh }}^{2}}x}}\frac{x}{{{{{(ax + b)}}^{2}} + 1}}.} $$

((A.5))

Since

$$\tanh x = 2\sum\limits_{n = 0}^\infty {\frac{x}{{c_{n}^{2} + {{x}^{2}}}},\quad {{c}_{n}} = \pi (n + 1{\text{/}}2),} $$

((A.6))

by differentiation over x, one obtains

$$\begin{gathered} \frac{1}{{{{{\cosh }}^{2}}x}} = 2\sum\limits_{n = 0}^\infty {\left[ {\frac{{2c_{n}^{2}}}{{{{{(c_{n}^{2} + {{x}^{2}})}}^{2}}}} - \frac{1}{{c_{n}^{2} + {{x}^{2}}}}} \right],} \\ {{c}_{n}} = \pi (n + 1{\text{/}}2). \\ \end{gathered} $$

((A.7))

Therefore,

$$\begin{gathered} \frac{{\partial I(a,b)}}{{\partial b}} = 2\sum\limits_{n = 0}^\infty {\int\limits_{ - \infty }^\infty {\frac{{dxx}}{{{{{(ax + b)}}^{2}} + 1}}} } \\ \times \left[ {\frac{{2c_{n}^{2}}}{{{{{(c_{n}^{2} + {{x}^{2}})}}^{2}}}} - \frac{1}{{c_{n}^{2} + {{x}^{2}}}}} \right] \\ = 2\pi b\sum\limits_{n = 0}^\infty {\frac{{1 + {{b}^{2}} - {{a}^{2}}c_{n}^{2}}}{{{{{[{{b}^{2}} + {{{(1 + a{{c}_{n}})}}^{2}}]}}^{2}}}}} \\ = \frac{2}{{\pi {{a}^{2}}}}\operatorname{Im} \left[ {(1 - ib)\Psi '\left( {\frac{1}{2} + \frac{{1 - ib}}{{\pi a}}} \right)} \right]. \\ \end{gathered} $$

((A.8))

Integrating Eq. (A.8) over b, we get

$$\begin{gathered} I(a,b) = \frac{2}{a}\operatorname{Re} \left[ {(1 + ib)\Psi \left( {\frac{1}{2} + \frac{{1 + ib}}{{\pi a}}} \right)} \right. \\ \,\left. { - \pi a\ln \Gamma \left( {\frac{1}{2} + \frac{{1 + ib}}{{\pi a}}} \right)} \right] + C(a). \\ \end{gathered} $$

((A.9))

At b ≫ 1, we find that

$$\begin{gathered} I(a,b) \simeq \frac{2}{a}\operatorname{Re} \left[ {ib + \left( {1 - \frac{{\pi a}}{2}\ln {{{(2\pi )}}_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{}}}}}}}}}}}}}}}}}}}}}}}} \right.} \right. \\ \,\left. {\left. { - \frac{{i{{\pi }^{2}}{{a}^{2}}}}{{12b}} + O\left( {\frac{1}{{{{b}^{2}}}}} \right)} \right)} \right] + C(a). \\ \end{gathered} $$

((A.10))

Using the Eq. (A.4), one obtains

$$C(a) = \frac{2}{a}\left( { - 1 + \frac{{\pi a}}{2}\ln (2\pi )} \right).$$

((A.11))

The results of the aforementioned procedure and a similar procedure for J(a, b) read as

$$\begin{gathered} I(a,b) = \frac{2}{a}\operatorname{Re} \left[ {(1 + ib)\Psi \left( {\frac{1}{2} + \frac{{1 + ib}}{{\pi a}}} \right) - 1} \right. \\ \left. {\, - \pi a\ln \Gamma \left( {\frac{1}{2} + \frac{{1 + ib}}{{\pi a}}} \right) + \frac{{\pi a}}{2}\ln (2\pi )} \right], \\ \end{gathered} $$

((A.12))

$$J(a,b) = 2\operatorname{Im} \left[ {\Psi \left( {\frac{1}{2} + \frac{{1 + ib}}{{\pi a}}} \right)} \right].$$

((A.13))

Using the above auxiliary integrals, one easily obtains Eqs. (11) and (12). The limiting case γ → 0 follows from the properties of the Ψ-function:

$$\begin{gathered} \operatorname{Im} \left[ {\Psi \left( {\frac{1}{2} + ix} \right)} \right] = \frac{\pi }{2}\tanh (\pi x), \\ \Psi (z) \simeq \ln z + \mathcal{O}\left( {\frac{1}{z}} \right),\quad {\text{|}}z{\text{|}} \gg 1. \\ \end{gathered} $$

((A.14))

APPENDIX B

2.1 Gapped Dirac Materials: Details of Calculations

Relationship between the carrier density and carrier imbalance.In a relativistic theory, for example, in QED, the number of electrons or positrons is not conserved, while a conserving number operator is required in order to build the statistical density matrix [91]. In QED, the conserved quantity is the difference of the numbers of positively and negatively charged particles: electrons and positrons.

In the Dirac materials, the “relativistic” nature of carriers is encoded in the symmetric DOS function, D(ε) = D(–ε). Accordingly, it is convenient to operate with the difference between the densities of electrons and holes instead of the total density of electrons [92, 93]. The difference is given by

$$\begin{gathered} n(T,\,\,\mu ) = \int\limits_{ - \infty }^\infty {d\varepsilon D(\varepsilon )[{{f}_{{{\text{FD}}}}}(\varepsilon - \mu )\theta (\varepsilon )} \\ \, - [1 - {{f}_{{{\text{FD}}}}}(\varepsilon - \mu )]\theta ( - \varepsilon )] \\ = - \frac{1}{2}\int\limits_{ - \infty }^\infty {d\varepsilon D(\varepsilon )\tanh \frac{{\varepsilon - \mu }}{{2T}}.} \\ \end{gathered} $$

((B.1))

The last equation can be rewritten in the form of Eq. (3). One can verify that the carrier imbalance n(T, μ) and the total carrier density n_tot(T, μ) are related by the expression n(T, μ) = n_tot(T, μ) – n_hf, where n_hf is the density of particles for a half-filled band (in the lower Dirac cone), n_hf = $\int_{ - \infty }^\infty d$εD(ε)θ(–ε). Consequently, there is no difference whether the entropy per particle is defined via the total carrier density n_tot or the carrier imbalance n.

Expressions for ∂n/∂T and ∂n/∂μ. The first temperature derivative in Eq. (5) depends on whether the chemical potential μ hits the discontinuity of the DOS D(ε) given by Eq. (16). Differentiating Eq. (18) over the temperature, one obtains

$$\begin{gathered} \frac{{\partial n(T,\,\,\mu )}}{{\partial T}} = \frac{{{\text{sgn}}(\mu )}}{{4T}} \\ \times \int\limits_{ - \infty }^\infty {d\varepsilon D(\varepsilon )} \left[ {\frac{{\varepsilon - \,{\text{|}}\mu {\text{|}}}}{{2T}}\frac{1}{{{{{\cosh }}^{2}}\frac{{\varepsilon - \,{\text{|}}\mu {\text{|}}}}{{2T}}}} - \frac{{\varepsilon + \,{\text{|}}\mu {\text{|}}}}{{2T}}\frac{1}{{{{{\cosh }}^{2}}\frac{{\varepsilon + \,{\text{|}}\mu {\text{|}}}}{{2T}}}}} \right]. \\ \end{gathered} $$

Changing the variable ε = 2Tx ± |μ| in two terms and changing the limits of integration, one obtains

$$\begin{gathered} \frac{{\partial n(T,\,\,\mu )}}{{\partial T}} = {\text{sgn}}(\mu )\int\limits_0^\infty {dx[D({\text{|}}\mu {\text{|}} + 2Tx)} \\ \, - D({\text{|}}\mu {\text{|}} - 2Tx)]\frac{x}{{{{{\cosh }}^{2}}x}}. \\ \end{gathered} $$

((B.3))

If the DOS, D(E) has a continuous derivative at the point E = |μ|, where Δ_i < |μ| < Δ_{i+ 1}, one can expand D(|μ| + 2Tx) – D(|μ| – 2Tx) ≃ 4TxD'(|μ|). Then integrating over x, we arrive at Eq. (19):

$$\begin{gathered} \frac{{\partial n(T,\,\,\mu )}}{{\partial T}} \simeq 4T{\text{sgn}}(\mu )D'({\text{|}}\mu {\text{|}})\int\limits_0^\infty {\frac{{{{x}^{2}}dx}}{{{{{\cosh }}^{2}}x}}} \\ = {\text{sgn}}(\mu )D'({\text{|}}\mu {\text{|}})\frac{{{{\pi }^{2}}}}{3}T. \\ \end{gathered} $$

((B.4))

On the other hand, at the discontinuity points μ = ±Δ_J at T → 0, we arrive at Eq. (20).

The second derivative in Eq. (5) in the zero temperature limit is just the DOS. Indeed, we have

$$\begin{gathered} \frac{{\partial \mu (T,\,\,\mu )}}{{\partial \mu }} = \frac{1}{{8T}}\int\limits_{ - \infty }^\infty {d\varepsilon D(\varepsilon )} \\ \times \left[ {\frac{1}{{{{{\cosh }}^{2}}\frac{{\varepsilon + \mu }}{{2T}}}} + \frac{1}{{{{{\cosh }}^{2}}\frac{{\varepsilon - \mu }}{{2T}}}}} \right] \to D(\mu ), \\ T \to 0. \\ \end{gathered} $$

((B.5))

This is because (1/4T)cosh^–2(x/2T) → δ(x) for x → 0. Substituting the DOS given by Eq. (16) to Eq. (B.5), we arrive at Eq. (21).

The carrier imbalance for a gapped graphene is given by Eq. (23). The corresponding derivatives are given by Eqs. (24) and (25):

$$\begin{gathered} {{\left( {\frac{{\partial n}}{{\partial \mu }}} \right)}_{T}} = \frac{2}{{\pi {{\hbar }^{2}}{v}_{{\text{F}}}^{2}}}\left\{ {\frac{\Delta }{2}\left( {\tanh \frac{{\mu - \Delta }}{{2T}} - \tanh \frac{{\mu + \Delta }}{{2T}}} \right)} \right. \\ \,\left. { + T\left[ {\ln \left( {2\cosh \frac{{\mu - \Delta }}{{2T}}} \right) + \ln \left( {2\cosh \frac{{\mu + \Delta }}{{2T}}} \right)} \right]} \right\}, \\ \end{gathered} $$

((B.6))

$$\begin{gathered} {{\left( {\frac{{\partial n}}{{\partial T}}} \right)}_{\mu }} = \frac{2}{{\pi {{\hbar }^{2}}{v}_{{\text{F}}}^{2}}}\left\{ {2\Delta \ln \frac{{1 + \exp \left( {\frac{{\mu - \Delta }}{T}} \right)}}{{1 + \exp \left( { - \frac{{\mu + \Delta }}{T}} \right)}}} \right. \\ \, + 2T{\text{L}}{{{\text{i}}}_{2}}\left[ { - \exp \left( { - \frac{{\mu + \Delta }}{T}} \right)} \right] \\ \end{gathered} $$

$$\, - 2T{\text{L}}{{{\text{i}}}_{2}}\left[ { - \exp \left( {\frac{{\mu - \Delta }}{T}} \right)} \right]$$

((B.7))

$$\begin{gathered} \, - \mu \ln \left( {2\cosh \frac{{\mu - \Delta }}{{2T}}} \right) - \mu \ln \left( {2\cosh \frac{{\mu + \Delta }}{{2T}}} \right) \\ \left. {_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{_{{}}}}}}}}}}}}}}}}}}}}}}}}}}}}}}}}}}}}}}}} + \frac{\Delta }{T}\frac{{\mu \sinh (\Delta {\text{/}}T) + \Delta \sinh (\mu {\text{/}}T)}}{{\cosh (\Delta {\text{/}}T) + \cosh (\mu {\text{/}}T)}}} \right\}. \\ \end{gathered} $$

Equations (27)–(29) and (32) are obtained using the low-temperature expansions of the derivatives, Eqs. (24) and (25).

We also provide the corresponding expressions for the zero-gap graphene and 2DEG. In the case Δ = 0, Eq. (23) reduces to

$$n(T,\mu ) = \frac{{2{{T}^{2}}}}{{\pi {{\hbar }^{2}}{v}_{{\text{F}}}^{2}}}[{\text{L}}{{{\text{i}}}_{2}}( - {{e}^{{ - \mu /T}}}) - {\text{L}}{{{\text{i}}}_{2}}( - {{e}^{{\mu /T}}})].$$

((B.8))

Using Eq. (5), we obtain the general expression

$$\begin{gathered} {{\left( {\frac{{\partial \mu }}{{\partial T}}} \right)}_{\mu }} = \frac{\mu }{T} - \frac{1}{{\ln \left( {2\cosh \frac{\mu }{{2T}}} \right)}} \\ \, \times [{\text{L}}{{{\text{i}}}_{2}}( - {{e}^{{ - \mu /T}}}) - {\text{L}}{{{\text{i}}}_{2}}( - {{e}^{{\mu /T}}})]. \\ \end{gathered} $$

((B.9))

In the 2DEG in the presence of Zeeman splitting considered in the Supplementary material in [14], the carrier density reads

$$n(\mu ,T)\, = \,\frac{m}{{4\pi }}T[{\text{ln}}(1 + {{e}^{{(\mu + Z)/T}}}) + {\text{ln}}(1 + {{e}^{{(\mu - Z)/T}}})].$$

((B.10))

Here, Z is the Zeeman splitting energy and m is the carrier mass. One can show that the entropy per particle in this case also obeys the quantization rule

$$\begin{gathered} {{\left. {\frac{{\partial S}}{{\partial n}}} \right|}_{{\mu = - Z}}} = 2\ln 2, \\ {{\left. {\frac{{\partial S}}{{\partial n}}} \right|}_{{\mu = Z}}} = \frac{{2\ln 2}}{3},\quad T \to 0. \\ \end{gathered} $$

((B.11))