Hostname: page-component-76fb5796d-2lccl Total loading time: 0 Render date: 2024-04-26T07:34:02.349Z Has data issue: false hasContentIssue false
  • English
  • Français

Coprime subdegrees of twisted wreath permutation groups

Part of: Permutation groups Other groups of matrices

Published online by Cambridge University Press:  28 June 2019

Alexander Y. Chua ,
Michael Giudici  and
Luke Morgan
Alexander Y. Chua
Affiliation:
Department of Mathematics and Statistics, Centre for the Mathematics of Symmetry and Computation, The University of Western Australia, 35 Stirling Highway, Crawley, WA 6009, Australia (21506815@student.uwa.edu.au; michael.giudici@uwa.edu.au; luke.morgan@famnit.upr.si)
Michael Giudici
Affiliation:
Department of Mathematics and Statistics, Centre for the Mathematics of Symmetry and Computation, The University of Western Australia, 35 Stirling Highway, Crawley, WA 6009, Australia (21506815@student.uwa.edu.au; michael.giudici@uwa.edu.au; luke.morgan@famnit.upr.si)
Luke Morgan*
Affiliation:
Department of Mathematics and Statistics, Centre for the Mathematics of Symmetry and Computation, The University of Western Australia, 35 Stirling Highway, Crawley, WA 6009, Australia (21506815@student.uwa.edu.au; michael.giudici@uwa.edu.au; luke.morgan@famnit.upr.si)
*
*Corresponding author.
Get access Rights & Permissions [Opens in a new window]

Abstract

Dolfi, Guralnick, Praeger and Spiga asked whether there exist infinitely many primitive groups of twisted wreath type with non-trivial coprime subdegrees. Here, we settle this question in the affirmative. We construct infinite families of primitive twisted wreath permutation groups with non-trivial coprime subdegrees. In particular, we define a primitive twisted wreath group G(m, q) constructed from the non-abelian simple group PSL(2, q) and a primitive permutation group of diagonal type with socle PSL(2, q)m, and determine many subdegrees for this group. A consequence is that we determine all values of m and q for which G(m, q) has non-trivial coprime subdegrees. In the case where m = 2 and 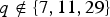$q\notin \{7,11,29\}$, we obtain a full classification of all pairs of non-trivial coprime subdegrees.

Keywords

primitive grouptwisted wreath groupsubdegrees

MSC classification

Primary: 20B15: Primitive groups
Secondary: 20H30: Other matrix groups over finite fields 20B05: General theory for finite groups
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 62 , Issue 4 , November 2019 , pp. 1137 - 1162
Copyright
Copyright © Edinburgh Mathematical Society 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Baddeley, R. W., Primitive permutation groups with a regular nonabelian normal subgroup, Proc. Lond. Math. Soc. (3) 67(3) (1993), 547–595.Google Scholar
2.Bosma, W., Cannon, J. and Playoust, C., The Magma algebra system. I. The user language, J. Symbolic Comput. 24(3–4) (1997), 235–265.Google Scholar
3.Cameron, P. J., Praeger, C. E., Saxl, J. and Seitz, G. M., On the Sims conjecture and distance transitive graphs, Bull. Lond. Math. Soc. 15(5) (1983), 499–506.Google Scholar
4.Dickson, L. E., Linear groups: with an exposition of the Galois field theory, with an introduction by Magnus, W. (Dover Publications, New York, 1958).Google Scholar
5.Dixon, J. D. and Mortimer, B., Permutation groups, Graduate Texts in Mathematics, Volume 163 (Springer-Verlag, New York, 1996).Google Scholar
6.Dolfi, S., Guralnick, R., Praeger, C. E. and Spiga, P., Coprime subdegrees for primitive permutation groups and completely reducible linear groups, Israel J. Math. 195(2) (2013), 745–772.Google Scholar
7.Dolfi, S., Guralnick, R., Praeger, C. E. and Spiga, P., On the maximal number of coprime subdegrees in finite primitive permutation groups, Israel J. Math. 216(1) (2016), 107–147.Google Scholar
8.Fawcett, J., Bases of primitive permutation groups. PhD thesis, University of Cambridge, 2013.Google Scholar
9.Fisman, E. and Arad, Z., A proof of Szep's conjecture on nonsimplicity of certain finite groups, J. Algebra 108(2) (1987), 340–354.Google Scholar
10.Giudici, M., Li, C. H., Praeger, C. E., Seress, Á. and Trofimov, V., On minimal subdegrees of finite primitive permutation groups, in Finite geometries, groups, and computation (eds Hulpke, A., Liebler, R., Penttila, T. and Seress, Á.), pp. 75–93 (Walter de Gruyter, Berlin, 2006).Google Scholar
11.Martin Isaacs, I., Finite group theory, Graduate Studies in Mathematics, Volume 92 (American Mathematical Society, Providence, RI, 2008).Google Scholar
12.Moore, E. H., The subgroups of the generalized finite modular group, The Decennial Publications, Volume 9 (University of Chicago Press, 1903).Google Scholar
13.Neumann, B. H., Twisted wreath products of groups, Arch. Math. (Basel) 14 (1963), 1–6.Google Scholar
14.Neumann, P. M., Finite permutation groups, edge-coloured graphs and matrices, in Proc. of the Summer School in Topics in Group Theory and Computation, University College, Galway, 1973 (ed. Curran, M. P. J.), pp. 82–118 (Academic Press, London, 1977).Google Scholar
15.Praeger, C. E., The inclusion problem for finite primitive permutation groups, Proc. Lond. Math. Soc. (3) 60(1) (1990), 68–88.Google Scholar