The Effect of Thermal Misbalance on Slow Magnetoacoustic Waves in a Partially Ionized Prominence-Like Plasma

Abstract

Solar prominences are partially ionized plasma structures embedded in the solar corona. Ground- and space-based observations have confirmed the presence of oscillatory motions in prominences, which have been interpreted in terms of standing or propagating MHD waves. Some of these observations suggest that slow magnetoacoustic waves could be responsible for these oscillations and have provided us with evidence about their damping/amplification with very small ratios between damping/amplifying times and periods, which have been difficult to explain from a theoretical point of view. Here we investigate the temporal behavior of non-adiabatic, slow, magnetoacoustic waves when a heating–cooling misbalance is present. The influence of optically thin losses and of a general heating term, in which density and temperature dependence can be modified, as well as the effect of partial ionization have been considered. Furthermore, a tentative example of how, using observational data, the observed ratio between damping/amplifying times and periods could be matched with those theoretically obtained is shown. In summary, different combinations of radiative losses, heating mechanisms, and typical wavenumbers, together with the effect of partial ionization, could provide a theoretical tool able to reproduce observational results on small-amplitude oscillations in prominences.

1 Introduction

The origin, formation, dynamics, and stability of solar prominences are some of the most intriguing topics in solar physics. Prominences are understood as cool and dense plasma structures in equilibrium under gravity and magnetic forces. Ground- and space-based observations have reported that prominences are highly dynamic (Berger et al., 2008) displaying small amplitude oscillations of local nature (Oliver and Ballester, 2002; Arregui, Oliver, and Ballester, 2012, 2018). The observed periods cover a wide range of values, and the detected Doppler velocities usually range from the noise level (about $0.1\text{ km}$ s⁻¹ in some cases) to 2 – 3 km s⁻¹. In order to explain these oscillations from a theoretical point of view, they have been usually interpreted in terms of magnetohydrodynamic (MHD) waves which has allowed the development of prominence seismology as a tool to infer the physical properties of these structures.

One of the most characteristic features of these oscillations is the presence of temporal damping (Landman, Edberg, and Laney, 1977; Tsubaki and Takeuchi, 1986; Wiehr, Balthasar, and Stellmacher, 1989; Molowny-Horas et al., 1999; Terradas et al., 2002; Lin, 2004; Berger et al., 2008; Ning et al., 2009; Ning, Cao, and Goode, 2009); however, there are also some observations showing temporal amplification. For instance, Molowny-Horas et al. (1999), using time series of H${\beta}$ filtergrams of a polar crown prominence, found six different spatial locations displaying oscillations with different periods and damping times with damping ratios in the range of 2 – 6, while they also found a location in which the oscillation was amplified with an amplification ratio of −5 (see Figure 1 and Table 3). Terradas et al. (2002) did a more detailed study of the same prominence and found regions displaying periodic motions. In one of these regions, propagating features were tracked and waves propagating in two opposite directions, with periods around 75 minutes, wavelengths of 70,000 km, and propagation speeds of 15 km s⁻¹, were identified. In general, the phase velocities determined in this study were in the range 10 – 20 km s⁻¹ which justifies to interpret these oscillations in terms of slow magnetoacoustic waves because for typical values of temperature, density, and magnetic field in quiescent prominences, the numerical value of the sound speed is of the order of $15\text{ km}$ s⁻¹. Furthermore, the sound speed is much smaller than the Alfvén speed with values of the order of $140\text{ km}$ s⁻¹. The temporal behavior of the Doppler velocity was described as,

$$ v = v_{\mathrm{0}} \cos \left (\frac{2 \pi}{P}t +\phi \right ) \mathrm{e}^{-t/\tau _{\mathrm{D}}} $$

(1)

with $v_{\mathrm{0}}$ the initial velocity amplitude, $P$ the period, $\phi$ the phase, and $\tau _{\mathrm{D}}$ the damping time when $\tau _{\mathrm{D}} > 0$ or the amplification time when $\tau _{\mathrm{D}} < 0$ and, in some prominence locations, the damping times were found to be between two and three times the period, while in other locations the fitted damping time was negative, suggesting the possibility of quasi-oscillatory motions growing with time. Regarding the nature of the waves, space observations enabled Berger et al. (2008) to identify several waves that were propagating upwards with speeds compatible with the sound speed at a temperature of 10,000 K, implying that these waves were of magnetoacoustic origin. Finally, Lin (2004), in a few adjacent slices of the 19 June 1998 filament, detected pronounced Doppler-velocity oscillations, with a dominant period of 26 minutes, which could only be observed for two or three periods after which they became strongly damped. However, in one of the slices, it was observed that before the beginning of the damping the amplitude had been growing with time. In summary, observational evidence about small-amplitude oscillations suggests that magnetoacoustic waves can be responsible for some of the reported observations and that, while temporal damping is a common feature of these observations, in some cases amplification of the oscillations or amplification followed by damping has also been observed.

In the coronal context and related with the study of damping/amplification of slow waves, thermal misbalance between heating and cooling has been considered by Nakariakov et al. (2017), Zavershinskii et al. (2019), Kolotkov, Nakariakov, and Zavershinskii (2019), Kolotkov, Duckenfield, and Nakariakov (2020), Belov, Molevich, and Zavershinskii (2020), Prasad, Srivastava, and Wang (2021a,b), Belov et al. (2021), Zavershinskii et al. (2021), Belov, Molevich, and Zavershinskii (2021), Prasad et al. (2022). This thermal misbalance is attributed to compressive waves that change the local thermal equilibrium and perturb the density, temperature, etc. of the background equilibrium, driving a local heating–cooling misbalance. At the same time, this effect establishes a feedback between the medium and the wave in which the wave loses or gains energy from the plasma that can lead to either wave damping or amplification (see Kolotkov, Zavershinskii, and Nakariakov (2021) for a recent review). This approach applied to magnetoacoustic waves in coronal structures has been considered by Nakariakov et al. (2017) when studying the evolution of slow waves in a cylindrical magnetic-flux tube, showing that a thermal misbalance strongly affects the wave behavior causing either damping or amplification, which means that in this last case the plasma acts as an active medium. Furthermore, they concluded that the consideration of thermal misbalance could explain the discrepancies between previous theoretical results and observations. Zavershinskii et al. (2019) studied the formation of slow-wave trains induced by heating–cooling misbalance in a coronal plasma. They took into account an optically thin radiative function, thermal conduction, and an unspecified heating term such as $H = h \rho ^{a} T^{b}$, with $a = 0$ and $b =1$, together with wavelengths long enough to make effects like thermal conduction and viscosity negligible. The results indicate that wave-induced thermal misbalance can produce damping or amplification and also dispersion of slow waves. Using a similar approach, but considering a variety of values for $a$ and $b$, and the infinite-magnetic-field approximation, Kolotkov, Nakariakov, and Zavershinskii (2019) studied the damping of standing slow waves in coronal loops. From the results obtained, a comparison was made with damping times and periods obtained from Solar-Ultraviolet Measurements of Emitted Radiation Experiment (SUMER) observations which helped to constrain the coronal heating function. Next, Kolotkov, Duckenfield, and Nakariakov (2020) assuming, again, thermal misbalance and an infinite magnetic field, used slow waves and different values for $a$ and $b$ to determine the conditions needed for the damping or amplification of slow and thermal waves in coronal-loop plasmas obtaining, at the same time, constraints on the solar-coronal heating function. Thermal misbalance has also been taken into account in the study of nonlinear shear Alfvén waves, and the effect is to modify the steepening and the amplitude of the wave under chromospheric or coronal-hole conditions (Belov, Molevich, and Zavershinskii, 2020). Related again with coronal loops, Prasad, Srivastava, and Wang (2021b) made a comparison between the effect of viscosity and thermal conduction on the damping of slow waves with and without thermal misbalance. The results suggest that it is possible to explain observations of oscillations obtained with SUMER when heating–cooling imbalance is taken into account together with thermal conduction and compressive viscosity. Next, Prasad, Srivastava, and Wang (2021a) and Prasad et al. (2022) have studied the influence of the same effects as before on the phase shifts of propagating and standing slow waves in solar coronal loops, respectively. Duckenfield, Kolotkov, and Nakariakov (2021) investigated the effect of magnetic field on the damping of slow waves in the solar corona when the heating term is characterized as $H = h \rho ^{a} T^{b} B^{c}$ in which $a$, $b$, and $c$ have been taken as free parameters. The results show that, basically, the change of the damping depends in a complex way on the plasma-$\beta$ and on the assumed coronal-heating function. However, it is also shown that for infinite magnetic field the behavior of the wave does not depend on how the heating function depends on the magnetic field. Belov et al. (2021) made a weakly nonlinear study, under thermal misbalance and coronal conditions, of the longitudinal motions generated by shear Alfvén waves, and it was shown that thermal misbalance induces the appearance of exponential in space and time bulk flows. Finally, Zavershinskii et al. (2021) have explored in detail the mixed properties of entropy and slow waves under heating–cooling misbalance in coronal conditions. Later, Belov, Molevich, and Zavershinskii (2021) studied how thermal misbalance affects slow magnetoacoustic waves inside a magnetic-flux tube under the second-order thin-flux-tube approximation. Using the parameters of an active-region-fan coronal loop, the phase speed, decrement and frequency dependence of the phase speed for the waves were determined and comparisons between seismological and spectrometric estimations of plasma parameters were done. Also, in coronal conditions, Claes and Keppens (2019) have studied the thermal stability of MHD modes in homogeneous plasma, revising Field’s original treatment. In order to compare with previous analytical results, they performed numerical simulations in which a heat-loss function with a constant heating term was included and they focused on slow and thermal waves. The obtained numerical results are in agreement with the theoretical ones, and of interest for prominence and coronal-rain formation. In the case of hydrodynamic waves and considering different heating mechanisms, Ibañez (1985) and Ibañez and Sanchez (1992) performed studies of spatial damping or amplification in solar-atmosphere physical conditions.

Regarding prominences, the temporal damping of prominence oscillations has been interpreted in terms of wave damping, although the different proposed mechanisms are still a matter of discussion. For instance, thermal mechanisms based on the joint action of radiation, thermal conduction, and heating have been put forward (Ibañez and Escalona, 1993; Terradas, Oliver, and Ballester, 2001; Carbonell, Oliver, and Ballester, 2004; Terradas et al., 2005; Soler, Oliver, and Ballester, 2007, 2008). In these mechanisms, different optically thin or thick radiative losses together with several heating mechanisms have been considered. The overall conclusion from these studies was that thermal mechanisms can account for the temporal damping of slow waves, although it has been difficult to match the strong damping found in observational data as well as to explain the cases of temporal amplification. In fact, one possible way to discriminate between the proposed mechanisms would be to compare the temporal scales that are produced by each of these mechanisms with those obtained from observations.

In the case of prominences, a first attempt to try to understand how the velocity amplitude of slow waves can be amplified, kept almost constant, or damped was made by Ballester et al. (2016), who made an study of how a non-stationary background influences the temporal behavior of slow waves. They considered an infinite homogeneous plasma with prominence physical properties together with an energy equation in which the radiative term was proportional to a time-dependent temperature and a constant external heating. Considering the initial background state, from the energy equation it is obtained that the temperature increases or decreases exponentially with time. Then, when initially the radiative losses are smaller than the heating, temperature increases; conversely, when the radiative losses are initially greater than the heating, temperature decreases with time. Finally, when the radiative losses become equal to the heating, the temperature becomes constant. The results point out that when the temperature increases the velocity perturbations are damped in time, but when the radiation becomes equal to the heating, the amplitude of the perturbations becomes constant. On the contrary, when temperature decreases with time, the velocity perturbations amplify. Furthermore, if we consider that during some time the temperature decreases while later it increases, the temporal behavior of velocity perturbations shows an initial increase of the amplitude followed by a decrease; therefore, a change in the temporal behavior of the prominence temperature produces a complete change of the temporal behavior of the velocity amplitude of slow waves. Then, if these sudden changes in the temperature happen in different regions of the prominence, different temporal behaviors of the velocity amplitude will be found in the different regions. In this approach, and for the sake of simplicity, two assumptions were considered: a constant external heating, independent of density and temperature, and a simplified radiative term. Despite these simplifications, the imbalance between prominence heating and cooling processes produces a temporal variation of the temperature, which at the same time modifies the temporal behavior of the amplitude of the velocity perturbations of the slow waves. This conclusion suggests that removing these simplifications by including terms describing thin or thick radiative losses as well as a heating term dependent on density and temperature could help us to obtain a more proper understanding of the temporal damping or amplification of slow waves.

Regarding prominence heating, in order to exist, prominences need mechanical equilibrium as well as a detailed energy balance between heating and radiative cooling. Energy balance studies suggest that incident radiation provides most of the heating of the prominence plasma (Gilbert, 2015); however, this radiative heating depends on the illumination from the surrounding atmosphere. Moreover, radiative-equilibrium prominence models, constructed from a balance between incident radiation and cooling (Anzer and Heinzel, 1999; Heinzel, Anzer, and Gunár, 2010; Heinzel and Anzer, 2012), as well as differential emission measures have pointed out that a further unknown heating is required in order to reproduce the observed temperatures in the prominence cores (Labrosse et al., 2010; Heinzel and Anzer, 2012; Heinzel, 2015) and to balance radiative losses (Parenti and Vial, 2007; Parenti, 2014; Soler et al., 2016; Melis, Soler, and Ballester, 2021).

In this work we investigate the temporal behavior of slow magnetoacoustic waves in an unbounded, partially ionized plasma, with physical properties akin to those of solar prominences, in which heating–cooling misbalance is present. The main aim will be to obtain the necessary conditions for the damping or amplification of the slow waves, as well as the influence of radiative losses on the derived conditions. To this end, and due to the uncertainty of prominence heating, we will consider a general heating term such as $H(\rho , T) = h_{0} \rho ^{a} T^{b}$ taking $a$ and $b$ as free parameters together with different radiative losses and thermal conduction. Finally, we will compare theoretical and observational results trying to match the observed ratios between damping/amplifying times and periods with those theoretically derived.

The structure of the article is as follows: In Section 2, the model, linearized equations for MHD waves, heat-loss functions, and the computation of the ionization degree are introduced; in Section 3, and in the case of parallel propagation, we obtain the dispersion relation for coupled thermal and slow waves, as well as approximate solutions which will help us to obtain qualitative information about the temporal behavior of these waves; in Section 4, the effect of partial ionization on the temporal behavior of slow and thermal waves is studied when different radiative losses and heating mechanisms are considered; next, a tentative example trying to reproduce observational data using our theoretical results is shown and, also, the case of oblique propagation is considered; finally, in Section 5, we summarize our results and draw our conclusions.

2 Model and Methods

2.1 Equilibrium Properties

As a background model, we consider a homogeneous unbounded, partially ionized hydrogen plasma threaded by a uniform magnetic field along the $x$-direction. The equilibrium magnitudes of the medium are given by

$$ p_{0} =\mathrm{const.}, \quad \rho _{0} = \mathrm{const.}, \quad T_{0} = \mathrm{const.}, \quad \boldsymbol{B}_{\mathbf{0}}= B_{0}^{\boldsymbol{x}}, \quad \boldsymbol{v}_{\mathbf{0}} = 0, $$

(2)

where $B_{0}$ is a constant, the effect of gravity has been ignored, and the number densities of neutrals, ions and electrons are $n_{\mathrm{n}}$, $n_{\mathrm{i}}$, $n_{\mathrm{e}}$, respectively, with $n_{\mathrm{e}}=n_{\mathrm{i}}$. Since we consider a medium with physical properties akin to those of a quiescent solar prominence, the plasma density, kept constant, is $\rho _{\mathrm{0}} = 4 \times 10^{-11}~\text{kg}$ m⁻³, and the strength of the magnetic field is $\vert \boldsymbol{B}_{\mathrm{0}} \vert = 10$ G, while the temperature is modified during our calculations which will modify, at the same time, other physical parameters. The relative densities of neutrals [$\xi _{\mathrm{n}}$] and ions [$\xi _{\mathrm{i}}$] are given by

$$ \xi _{\mathrm{n}} = \frac{\rho _{\mathrm{n}}}{\rho} \approx \frac{n_{\mathrm{n}}}{n_{\mathrm{i}} + n_{\mathrm{n}}}, \quad \xi _{ \mathrm{i}} = \frac{\rho _{\mathrm{i}}}{\rho} \approx \frac{n_{\mathrm{i}}}{n_{\mathrm{i}} + n_{\mathrm{n}}}, $$

(3)

where we have neglected the contribution of electrons. The degree of ionization of the plasma, represented by $i_{\mathrm{d}} =\xi _{\mathrm{i}}$, is characterized by the ionization fraction [$\tilde{\mu}$] defined as the mean atomic weight (the average mass per particle in units of $m_{\mathrm{p}}$), which is given by,

$$ \tilde{\mu} = \frac{1}{1+\xi _{\mathrm{i}}} = \frac{1}{1+i_{\mathrm{d}}} , $$

(4)

which gives us information about the plasma degree of ionization. Following Equation 4, for a fully ionized plasma $i_{\mathrm{d}} =1$, $\tilde{\mu} = 0.5$ while for a neutral plasma $i_{\mathrm{d}}= 0$, $\tilde{\mu} = 1$. Taking into account the above parameters, pressure $p_{0}$ is given by,

$$ p_{0} =\frac {\rho _{0} \mathrm{R} T_{0}}{\tilde{\mu}}= (1+i_{\mathrm{d}}) \rho _{0} \mathrm{R} T_{0} $$

(5)

with $\mathrm{R}$ the perfect gas constant. Then, plasma-$\beta$ is,

$$ \beta = \frac{2 \mu _{0} p_{0}}{B_{0}}= 1.5 \times 10^{-5} $$

(6)

2.2 Basic and Linearized Equations

The single-fluid approximation assumes a strong coupling between ions, electrons, and neutrals so that all the species effectively behave as one fluid. In this approximation, the basic magnetohydrodynamic (MHD) equations are written in terms of total quantities, while the effect of the interactions between the various species remains in the form of several nonideal terms. Single-fluid MHD equations for a partially ionized hydrogen plasma were derived by Forteza et al. (2007) (see also Ballester et al., 2018), and later were extended by including terms representing non-adiabatic processes in the energy equation (Forteza, Oliver, and Ballester, 2008; Carbonell, Oliver, and Ballester, 2009; Soler, Oliver, and Ballester, 2008; Soler, 2010; Barceló, Carbonell, and Ballester, 2011; Ballester et al., 2020). The single-fluid approximation is accurate to study MHD waves in partially ionized plasmas as long as the wave frequency remains lower than the ion–neutral collision frequency. The basic single-fluid equations used here are

$$\begin{aligned} \frac{{\mathrm{D}}\rho}{{\mathrm{D}}t} =& -\rho \nabla \cdot \boldsymbol{v}, \end{aligned}$$

(7)

$$\begin{aligned} \rho \frac{{\mathrm{D}}\boldsymbol{v}}{{\mathrm{D}}t} =& -\nabla p + \frac{1}{\mu} \left ( \nabla \times \boldsymbol{B} \right ) \times \boldsymbol{B}, \end{aligned}$$

(8)

$$\begin{aligned} \frac{\partial \boldsymbol{B}}{\partial t} =& \nabla \times \left ( { \mathbf{v}}\times \boldsymbol{B} \right ) - \nabla \times \left ( \eta \nabla \times \boldsymbol{B} \right ) \\ & + \nabla \times \left \{ \eta _{\mathrm{A}}\left [ \left ( \nabla \times \boldsymbol{B} \right ) \times \boldsymbol{B} \right ] \times \boldsymbol{B} \right \}, \end{aligned}$$

(9)

$$\begin{aligned} \frac{{\mathrm{D}}p}{{\mathrm{D}}t} =& - \gamma p \nabla \cdot \boldsymbol{v} + \left ( \gamma -1 \right ) \mathcal{L}, \end{aligned}$$

(10)

$$\begin{aligned} p =& \frac{\rho \mathrm{R} T}{\tilde{\mu}} \end{aligned}$$

(11)

where $\frac{{\mathrm{D}}}{{\mathrm{D}}t} = \frac{\partial}{\partial t} + \boldsymbol{v} \cdot \nabla$ denotes the material or total derivative, $\rho$ is the mass density, $p$ is the thermal pressure, $T$ is the temperature, $\boldsymbol{v}$ is the velocity vector, $\boldsymbol{B}$ is the magnetic field vector, $\gamma$ is the adiabatic exponent, $\mu$ the magnetic permeability, $\eta$ and $\eta _{\mathrm{A}}$ are the coefficients of Ohmic and ambipolar diffusion, respectively, ℒ represents the net effect of all the sources and sinks of energy such as radiation, heating, thermal conduction, Joule heating, etc., and $\mathrm{R}$ is the gas constant. Equations 7 – 11 are the continuity, momentum, induction, energy, and state equations, respectively.

The dispersion relation for linear MHD waves can be obtained by considering small perturbations from equilibrium of the form

$$\begin{aligned} &{} \boldsymbol{B}(t,\boldsymbol{r})=\boldsymbol{B}_{0}+\boldsymbol{B}_{1}(t,\boldsymbol{r}),\quad p(t,\boldsymbol{r})=p_{0}+p_{1}(t,\boldsymbol{r}), {} \\ &{} \rho (t,\boldsymbol{r})=\rho _{0}+\rho _{1}(t,\boldsymbol{r}), \quad T(t,\boldsymbol{r})=T_{0}+T_{1}(t,\boldsymbol{r}), \quad {} \\ &{} \boldsymbol{v}(t,\boldsymbol{r})= \boldsymbol{v}_{0}+\boldsymbol{v}_{1}(t,\boldsymbol{r}), {} \end{aligned}$$

and linearizing the single-fluid basic equations. Since the medium is unbounded, we perform a Fourier analysis in terms of plane waves and assume that perturbations behave as

$$ f_{1}(\boldsymbol{r},t)=f\mathrm{e}^{\mathrm{i}(\omega t-\boldsymbol{k} \cdot \boldsymbol{r})}, $$

(12)

with the wavevector $\boldsymbol{k}$ lying in the $xz$-plane, and introducing the propagation angle [$\theta$] between $\boldsymbol{k}$ and $\boldsymbol{B}_{0}$, the wavenumber components can be expressed as $k_{x}=k\cos \theta$ and $k_{z}=k\sin \theta$. Applying the above stated procedure to the single fluid MHD equations describing a partially ionized plasma in which non-adiabatic processes take place, the following scalar equations can be obtained,

$$ \omega \rho _{1} - \rho _{0}(k_{x}v_{1x}+k_{z}v_{1z})=0, $$

(13)

$$ \omega \rho _{0}v_{1x}-k_{x}p_{1}=0, $$

(14)

$$ \omega \rho _{0}v_{1y}+\frac{B_{0}}{\mu}k_{x}B_{1y}=0, $$

(15)

$$ \omega \rho _{0}v_{1z}-k_{z}p_{1}+\frac{B_{0}}{\mu}(k_{x}B_{1z}-k_{z}B_{1x})=0 ,$$

(16)

$$\begin{aligned} \mathrm{i}\omega \left (p_{1}-c_{\mathrm{s}}^{2}\rho _{1}\right )+( \gamma -1)\left (\kappa _{\parallel \mathrm{e}}k_{x} ^{2}+\kappa _{ \mathrm{n}} k^{2} +\rho _{0}L_{T}\right )T_{1}+ {} \\ +(\gamma -1)(L+\rho _{0}L_{\rho})\rho _{1}=0, \end{aligned}$$

(17)

$$\begin{aligned} B_{1x}\left (\mathrm{i}\omega +k_{x}^{2}\eta +k_{z}^{2}\eta _{ \mathrm{C}}\right )+(\eta -\eta _{\mathrm{C}})k_{x}k_{z}B_{1z}- {} \\ -B_{0}k_{z}(iv_{1z})=0, \end{aligned}$$

(18)

$$ B_{1y}\left (\mathrm{i}\omega +k_{x}^{2}\eta _{\mathrm{C}}+k_{z}^{2} \eta \right )+iB_{0}k_{x}v_{1y}=0, $$

(19)

$$\begin{aligned} B_{1z}\left (\mathrm{i}\omega +k_{x}^{2}\eta _{\mathrm{C}}+k_{z}^{2} \eta \right )+(\eta -\eta _{\mathrm{C}})k_{x}k_{z}B_{1x}+ {} \\ +B_{0}k_{x}(\mathrm{i}v_{1z})=0, \end{aligned}$$

(20)

$$ k_{x}B_{1x}+k_{z}B_{1z}=0, $$

(21)

$$ \frac{p_{1}}{p_{0}}-\frac{\rho _{1}}{\rho _{0}}-\frac{T_{1}}{T_{0}}=0, $$

(22)

where $L$ is the heat-loss function, which depends on the local plasma parameter, and $\eta _{\mathrm{C}}-\eta = \eta _{\mathrm{A}}\vert B_{0}\vert ^{2}$, $\eta _{\mathrm{C}}$ being Cowling’s diffusion coefficient. Usually, for solar applications, the heat-loss function $L$ represents the difference between an arbitrary heat input and a radiative-loss function such as

$$ L(\rho , T) =L_{r} (\rho , T) - H(\rho , T) $$

(23)

with $L_{r}(\rho , T)$ describing radiative losses while $H(\rho , T)$ describes the heat input, with the units of both quantities being W kg⁻¹. In the case of an equilibrium with uniform temperature, such as we will consider here, the heat-loss function is

$$ L(\rho _{0}, T_{0}) = 0 $$

(24)

and the factors $L_{\rho}$, $L_{T}$ in Equation 17 are,

$$ L_{\rho} = \left (\frac {\partial L}{\partial \rho}\right )_{T} $$

(25)

and

$$ L_{T} =\left (\frac {\partial L}{\partial T}\right )_{p} = \left ( \frac {\partial L}{\partial T}\right )_{\rho}- \frac{\rho _{0}}{T_{0}} L_{\rho} $$

(26)

with $T$, $p$, and $\rho$ held constant, respectively, at the equilibrium state. Furthermore, $\kappa _{\parallel \mathrm{e}}$ and $\kappa _{\mathrm{n}}$ represent the coefficients of parallel thermal conduction by electrons and isotropic thermal conduction by neutrals, respectively.

On the other hand, by modifying the prominence plasma temperature the value of the mean atomic weight [$\tilde{\mu}$] is modified as well as both Ohmic’s [$\eta$] and Cowling’s [$\eta _{\mathrm{C}}$] resistivities which in MKS units are given by (Soler, 2010),

$$\begin{aligned} &\eta = 5.2 \times 10^{7} T^{-1.5} (30.5 - 1.15 \log n_{\mathrm{e}} + 3.45 \log T), \end{aligned}$$

(27)

$$\begin{aligned} &\eta _{\mathrm{C}} = \eta + \frac{\xi _{\mathrm{n}}^{2} B_{\mathrm{0}}^{2}}{\mu _{\mathrm{0}} \alpha _{\mathrm{n}}}, \end{aligned}$$

(28)

where $n_{\mathrm{e}}$ is the electronic number density, and $\alpha _{\mathrm{n}}$ is the neutral-friction coefficient given by,

$$ \alpha _{\mathrm{n}} = 0.5 \xi _{\mathrm{n}} (1 - \xi _{\mathrm{n}}) \frac{\rho ^{2}}{m_{\mathrm{n}}}\sqrt{ \frac{16 \mathrm{k_{B}} T}{\pi m_{\mathrm{i}}}}\Sigma _{\mathrm{in}}, $$

(29)

with $\Sigma _{\mathrm{in}}$ being the ion–neutral collisional cross-section, and $\mathrm{k}_{\mathrm{B}}$ the Boltzmann constant

2.3 Heat-Loss Function

Processes of radiative heating and cooling are important for modeling the temperature distribution of atmospheric structures such as prominences. When an absorbed photon is destroyed by a collisional deexcitation, its energy is converted into thermal energy of the plasma (radiative heating). On the other hand, when the atom is excited by a collision and a photon emission follows, the energy lost by the colliding electron is converted to radiation energy of a photon and may escape from the plasma (radiative cooling) (Heinzel, 2015, 2019). For our study, two optically thin radiative-loss functions such as those from Hildner (1974) and CHIANTIv7 (Dere et al., 1997; Landi et al., 2012; Soler, Ballester, and Parenti, 2012) have been chosen. The general expression for these radiative functions is $L_{r}(\rho ,T)=\chi ^{*}\rho T^{\alpha}$ with $\chi ^{*}$ and $\alpha$ being piecewise functions depending on the temperature, while $H(\rho , T) = h_{\mathrm{0}}\rho ^{a} T^{b}$ represents an arbitrary heating function, which can be modified by taking different values for the exponents $a$ and $b$. Then, our heat-loss function is given by

$$ L(\rho , T) = \chi ^{*}\rho T^{\alpha} - h_{\mathrm{0}} \rho ^{a} T^{b}. $$

(30)

However, while the use of an optically thin radiative cooling seems to be a reasonable approach for coronal or almost coronal conditions, it may not be valid for prominence conditions because they are optically thick. In this case, the radiative losses from the internal part of the prominence are greatly reduced, and this is usually represented by changing the exponent $\alpha$ as well as $\chi ^{*}$ in the cooling function (Milne, Priest, and Roberts, 1979). Figure 2 displays the radiative losses, between 6000 and 9000 K, due to the three radiative functions considered, and we can observe that there are strong differences in the losses described by these functions. On the other hand, in the past, different heating mechanisms have been considered, and the values taken into account for exponents $a$ and $b$ have been (Rosner, Tucker, and Vaiana, 1978; Dahlburg and Mariska, 1988; Ibañez and Sanchez, 1992; Ibañez and Escalona, 1993)

• i) Constant heating per unit volume ($a = b = 0$).

• ii) Constant heating per unit mass ($a = 1$, $b = 0$).

• iii) Heating by coronal current disipation ($a = 1$, $b = 1$).

• iv) Heating by Alfvén mode/mode conversion ($a = b = 7/6$).

• v) Heating by Alfvén mode/anomalous conduction damping ($a = 1/2$, $b = -1/2$).

Finally, in the case of a partially ionized plasma, and because of its isotropy, a neutral contribution [$\kappa _{\mathrm{n}}$] must be added to parallel thermal conduction [$\kappa _{\parallel \mathrm{e}}$] which is dominated by electrons, and to perpendicular thermal conduction [$\kappa _{\perp \mathrm{i}}$] dominated by ions. Thus,

$$\begin{aligned} \kappa _{\parallel} = \kappa _{\parallel \mathrm{e}} + \kappa _{ \mathrm{n}}, \end{aligned}$$

(31)

$$\begin{aligned} \kappa _{\perp} = \kappa _{\perp \mathrm{i}} + \kappa _{\mathrm{n}}. \end{aligned}$$

(32)

In terms of plasma parameters, the expression for the parallel conductivity of electrons is

$$ \kappa _{\parallel \mathrm{e}} = 1.8 \times 10^{-10} \frac{\xi _{\mathrm{i}} T^{5/2}}{\ln \ \Lambda} \ \mathrm{\,W \,m^{-1} \,K^{-1}}, $$

(33)

the perpendicular conductivity due to ions is

$$ \kappa _{\perp \mathrm{i}} = 1.48 \times 10^{-42} \frac{\ln \ \Lambda \xi _{\mathrm{i}}^{3} \rho ^{2}}{m_{\mathrm{i}}^{2} \vert \boldsymbol{B}\vert ^{2} T^{1/2}} \ \mathrm{\,W \,m^{-1} \,K^{-1}}, $$

(34)

while the conductivity of neutrals is given by

$$ \kappa _{\mathrm{n}} = 2.44 \times 10^{-2} \xi _{\mathrm{n}} T^{1/2}~\text{W}\,\mbox{m}^{-1} \,\mbox{K}^{-1} $$

(35)

with $\ln \ \Lambda$ being the Coulomb logarithm.

2.4 Ionization Degree

The ionization degree of a prominence is mainly determined by radiation through ionization–recombination processes, however, the exact ionization degree of prominence plasma, which depends on physical conditions, is not well known. Following Patsourakos and Vial (2002), the ratio of electron density to neutral-hydrogen density seems to vary between 0.1 and 10, that is, from almost neutral to almost fully ionized plasma. On the other hand, the local thermodynamic equilibrium (LTE) assumption is not fully adequate for a prominence plasma which, in general, is far from LTE. The non-LTE ionization in prominences is dominated by the hydrogen Lyman and Balmer continuum photoionization where the continuum radiation fields strongly depend on the solar-disk radiation illuminating the prominence (radiative heating), which is quite different from LTE conditions (Heinzel, 2015, 2019).

In spite of this, although the assumption of LTE is not fully realistic for prominence conditions, for the sake of simplicity, we compute the mean atomic weight [$\tilde{\mu}$] by means of the Saha equation, which for a hydrogen plasma can be written as,

$$ \frac{n_{\mathrm{i}}^{2} }{n_{\mathrm{n}}} =\left ( \frac{2 \pi m_{\mathrm{e}} \mathrm{k_{B}} T_{0} }{\mathrm{h}^{2}} \right )^{3/2} \exp \left [-\frac{\chi}{\mathrm{k_{B}} T_{0} }\right ], $$

(36)

where $m_{\mathrm{e}}$ is the electron mass, $\mathrm{h}$ is Planck’s constant, and $\chi = 13.6$ eV is the hydrogen ionization potential. From Equation 36, we compute $\xi _{\mathrm{i}}$ and $\xi _{\mathrm{n}}$ as functions of density and temperature as

$$\begin{aligned} &\xi _{\mathrm{i}} = \frac{1}{2} M \left (\sqrt{1+\frac{4}{M}}-1\right ) , \end{aligned}$$

(37)

$$\begin{aligned} &\xi _{\mathrm{n}} = 1 - \frac{1}{2} M \left (\sqrt{1+\frac{4}{M}}-1\right ) , \end{aligned}$$

(38)

where

$$ M = 4 \times 10^{-6} \rho _{0}^{-1} T_{0} ^{3/2} \exp \left (-T^{*}/T_{0} \right ), $$

(39)

with

$$ T^{*} = 1.578 \times 10^{5}~K. $$

(40)

Then, using Equations 4 and 37, the mean atomic weight can be expressed as

$$ \tilde{\mu}= \frac{1}{1 + \frac{1}{2} M \left (\sqrt{1+\frac{4}{M }}-1\right )} $$

(41)

and, from Equation 4, the ionization degree is given by

$$ i_{\mathrm{d}} = \frac{1}{2} M \left (\sqrt{1+\frac{4}{M}}-1\right ) $$

(42)

while the sound speed [$c_{\mathrm{s}}$] is

$$ c_{\mathrm{s}} = \left (\frac{\gamma \mathrm{R} T_{0}}{\tilde{\mu}} \right )^{0.5} $$

(43)

Therefore, when prominence temperature, $T_{0}$, is modified, $\tilde{\mu}$ is also modified as well as the ionization degree [$i_{\mathrm{d}}$] and the sound speed [$c_{\mathrm{s}}$] which affects the frequencies of magnetoacoustic waves. Furthermore, once $\xi _{\mathrm{n}}$ has been computed, we could also compute Cowling’s resistivity from Equation 28, since $\eta$ is also modified due to the change in the electronic density [$n_{\mathrm{e}}$].

3 Magnetoacoustic Waves

From Equations 13 – 22 we can derive the dispersion relations governing the behavior of the different MHD waves. Since Equations 15 and 19 are decoupled from the rest, from them we can obtain a dispersion relation for Alfvén waves in a partially ionized plasma, which is

$$\begin{aligned} \omega ^{2} -\mathrm{i} \omega k^{2}( \eta _{\mathrm{C}} \cos ^{2} \theta + \eta \sin ^{2} \theta )-v_{\mathrm{a}}^{2} k^{2} \cos ^{2} \theta = 0, \end{aligned}$$

(44)

where $\theta$ is the angle between the wavenumber vector and the magnetic field, and $v_{\mathrm{a}}$ is the Alfvén speed. Furthermore, it is also important to remark that from Equation 44 we can conclude that the temporal damping of Alfvén waves is governed by Spitzer’s [$\eta$] and Cowling’s [$\eta _{\mathrm{C}}$] resistivities (Forteza, Oliver, and Ballester, 2008; Soler, 2010).

From the rest of the linearized equations, the dispersion relation for thermal and magnetoacoustic waves in a partially ionized plasma can be obtained,

$$\begin{aligned} (\omega ^{2} -k^{2} \Delta ^{2}) (\mathrm{i}k^{2} \eta _{\mathrm{C}} \omega -\omega ^{2})+k^{2} v_{\mathrm{a}}^{2}(\omega ^{2} -k_{x}^{2} \Delta ^{2}) =0, \end{aligned}$$

(45)

where $\Delta ^{2}$ is the non-adiabatic sound speed squared (Forteza, Oliver, and Ballester, 2008; Soler, 2010) defined as

$$\begin{aligned} \Delta ^{2} = \frac{c_{\mathrm{s}}^{2}}{\gamma}\Big[ \frac{(\gamma -1)(\bar{\kappa}_{\parallel \mathrm{e}}k^{2}_{x}+ \bar{\kappa}_{\mathrm{n}} k^{2}+\omega _{T}-\omega _{\rho}) +\mathrm{i} \gamma \omega}{(\gamma -1)(\bar{\kappa}_{\parallel \mathrm{e}}k^{2}_{x}+\bar{\kappa}_{\mathrm{n}} k^{2}+\omega _{T})+\mathrm{i} \omega} \Big], \end{aligned}$$

(46)

with

$$\begin{aligned} \bar{\kappa}_{\parallel \mathrm{e}} = &\frac{T_{0}}{p_{0}}\kappa _{ \parallel \mathrm{e}}, \quad \bar{\kappa}_{\mathrm{n}} = \frac{T_{0}}{p_{0}}\kappa _{\mathrm{n}}, \\ \omega _{\mathrm{\rho}} = & \frac{\rho _{0}}{p_{0}}\rho _{0} L_{\rho}, \quad \omega _{{T}} = \frac{\rho _{0}}{p_{0}} T_{0} L_{T}, \end{aligned}$$

and where in the adiabatic case $\Delta ^{2}= c_{\mathrm{s}}^{2}$.

The dispersion relation in Equation 45, with an additional term representing the diamagnetic-current coefficient, was solved by Forteza et al. (2007), showing that the effect of ion–neutral collisions is almost irrelevant for the damping of slow waves, while they could be a viable mechanism to damp fast waves. On the other hand, we can write Equations 45 and 46 in terms of characteristic times of the thermal misbalance introduced by Zavershinskii et al. (2019), Kolotkov, Nakariakov, and Zavershinskii (2019), Kolotkov, Duckenfield, and Nakariakov (2020), Prasad, Srivastava, and Wang (2021b) and Duckenfield, Kolotkov, and Nakariakov (2021), which are

$$\begin{aligned} \tau _{\mathrm{r1}} = \frac{\gamma c_{\mathrm{v}}}{\left (\frac {\partial L}{\partial T}\right )_{\rho}-\frac{\rho _{0}}{T_{0}} L_{\rho} }, \end{aligned}$$

(47)

$$\begin{aligned} \tau _{\mathrm{r2}} = \frac{c_{\mathrm{v}}}{\left (\frac {\partial L}{\partial T}\right )_{\rho}}, \end{aligned}$$

(48)

with $c_{\mathrm{v}}$ the specific heat given by

$$ c_{\mathrm{v}} = \frac{\mathrm{k_{B}}}{(\gamma - 1) \tilde{\mu}H }. $$

(49)

Now, developing Equations 47 and 48, we can write the characteristic times for thermal misbalance in terms of radiative losses and heating functions, obtaining

$$\begin{aligned} \tau _{\mathrm{r1}} = \frac{\gamma c_{\mathrm{v}} T_{0}}{(\alpha -1) L_{\mathrm{r}} (\rho _{0}, T_{0})+(a-b)H (\rho _{0}, T_{0})}, \end{aligned}$$

(50)

$$\begin{aligned} \tau _{\mathrm{r2}} = \frac{c_{\mathrm{v}} T_{0}}{\alpha L_{\mathrm{r}} (\rho _{0}, T_{0})-b H (\rho _{0}, T_{0})}. \end{aligned}$$

(51)

Now, using the characteristic times $\tau _{\mathrm{r1}}$ and $\tau _{\mathrm{r2}}$, we can write

$$\begin{aligned} \omega _{{T}} = \frac{1}{(\gamma - 1) \tau _{\mathrm{r2}}}, \end{aligned}$$

(52)

$$\begin{aligned} \omega _{\mathrm{\rho}} = \frac{1}{\gamma -1}\left ( \frac{1}{\tau _{\mathrm{r2}} }- \frac{\gamma}{\tau _{\mathrm{r1}}} \right ). \end{aligned}$$

(53)

Since, in principle, thermal conduction is also taken into account, we introduce

$$\begin{aligned} \tau _{\mathrm{ce}} = \frac{1}{(\gamma - 1) \bar{\kappa}_{\parallel \mathrm{e}} k_{\mathrm{x}}^{2}} \end{aligned}$$

(54)

$$\begin{aligned} \tau _{\mathrm{cn}} = \frac{1}{(\gamma - 1) \bar{\kappa}_{\mathrm{n}} k^{2}} \end{aligned}$$

(55)

corresponding to characteristic times of electronic thermal conduction parallel to the magnetic field and to isotropic thermal conduction by neutrals, respectively, while perpendicular thermal conduction by ions has been neglected. Furthermore, since we consider a partially ionized plasma, we have also included

$$\begin{aligned} \tau _{\mathrm{\eta _{\mathrm{C}}}} = \frac{1}{k^{2} \eta _{\mathrm{C}}}. \end{aligned}$$

(56)

Taking into account all these characteristic times, we can write Equation 46 as

$$\begin{aligned} \Delta ^{2} = \frac{c_{\mathrm{s}}^{2}}{\gamma} \frac{\left [\frac{1}{\tau _{\mathrm{ce}}} + \frac {1}{\tau _{\mathrm{cn}}}+\frac{\gamma}{\tau _{\mathrm{r1}}} +\mathrm{ i} \gamma \omega \right ]}{\left [\frac {1}{\tau _{\mathrm{ce}}}+ \frac {1}{\tau _{\mathrm{cn}}}+\frac{1}{\tau _{\mathrm{r2}}}+\mathrm{i} \omega \right ]}, \end{aligned}$$

(57)

which can be included in Equation 45. On the other hand, we must bear in mind that when partial ionization is considered the adiabatic exponent [$\gamma$] depends on the ionization degree, therefore, since it enters in the expression of parameters such as sound speed, characteristic times, etc, all these parameters will become ionization-degree dependent.

In the next section we will consider the propagation of slow waves parallel to the magnetic field.

3.1 Parallel Propagation

Considering only longitudinal propagation ($\theta = 0$), Equation 45 becomes

$$\begin{aligned} (\omega ^{2} -k_{{x}}^{2} \Delta ^{2}) \left ( \frac{\mathrm{i} \omega}{\tau _{\eta _{\mathrm{C}}}} -\omega ^{2}+k_{ {x}}^{2} v_{\mathrm{a}}^{2}\right )=0, \end{aligned}$$

(58)

then, setting the expression within the second bracket equal to zero, we obtain Equation 44 with $\theta = 0$, which is the dispersion relation corresponding to Alfvén waves propagating parallel to the magnetic field. Next, setting the expression within the first bracket equal to zero, we obtain

$$\begin{aligned} \omega ^{2} -k_{{x}}^{2} \Delta ^{2} = 0, \end{aligned}$$

(59)

which is the dispersion relation corresponding to coupled slow and thermal or entropy waves. Once Equation 59 has been expanded, we obtain

$$\begin{aligned} &\omega ^{3} -\mathrm{i} \omega ^{2}\left ( \frac{1}{\tau _{\mathrm{r2}}} + \frac{1}{\tau _{\mathrm{ce}}}+ \frac{1}{\tau _{\mathrm{cn}}} \right ) - c_{\mathrm{s}}^{2} k_{ {x}}^{2} \omega + \\ &+\mathrm{i} c_{\mathrm{s}}^{2} k_{{x}}^{2} \left ( \frac{1}{\tau _{\mathrm{r1}}} + \frac{1}{\gamma \tau _{\mathrm{ce}}}+ \frac{1}{\gamma \tau _{\mathrm{cn}}} \right ) =0. \end{aligned}$$

(60)

This dispersion relation is a third-order polynomial, which has a pair of roots representing slow waves which satisfy $(\omega _{1},\omega _{2})$, $\omega _{\mathrm{1}} = \omega _{\mathrm{r}} + \mathrm{i} \omega _{ \mathrm{i}}$ and $\omega _{\mathrm{2}} = - \omega _{\mathrm{r}} + \mathrm{i} \omega _{ \mathrm{i}}$, and a purely imaginary root that corresponds to the entropy mode (Carbonell, Oliver, and Ballester, 2004). In ideal MHD, the entropy mode is a solution of linearized MHD equations, it represents a nonpropagating perturbation of density, neither decaying or growing (Goedbloed and Poedts, 2004). When non-adiabatic mechanisms are considered, the entropy mode is called the thermal wave with $\omega = \mathrm{i} \omega _{\mathrm{i}}$. Then, the period of the waves is given by $T = \frac{2 \pi}{\omega _{\mathrm{r}}}$ while the damping time is $\tau _{\mathrm{D}} = \frac{1}{\omega _{\mathrm{i}}}$. Furthermore, we introduce a quality factor, $Q$, which we define as,

$$\begin{aligned} Q = \frac{1}{2} \frac{\vert \omega _{\mathrm{r}} \vert}{\omega _{\mathrm{i}}}, \end{aligned}$$

(61)

which can only be applied to slow waves, since the real part of the frequency of the thermal wave is always zero. The reason for this definition is that, since we are considering temporal damping ($\omega _{\mathrm{i}} > 0$) or amplification ($\omega _{\mathrm{i}} < 0$), then $Q > 0$ for damping while $Q < 0$ for amplification. In the case of the thermal wave, its temporal behavior is derived from the sign of the purely imaginary root.

Before proceeding to solve Equation 60, we can make a comparison between the characteristic times corresponding to thermal radiation,

$$ \tau _{\mathrm{rad}} = \frac{\gamma p_{\mathrm{0}}}{(\gamma - 1) \rho _{0}^{2} \chi ^{*} T_{0}^{\alpha}} $$

(62)

and conduction by electrons and neutrals along the magnetic field,

$$ \tau _{\mathrm{cond}} = \frac{c_{\mathrm{v}}}{\kappa k^{2}} $$

(63)

where $\kappa$ is the thermal-conduction coefficient corresponding electrons (Equation 33) and to neutrals (Equation 35), respectively. Since we consider parallel propagation, in Figure 3 we show a comparison between those characteristic time scales for an interval of parallel wavenumbers, $10^{-8}\text{ m}^{-1}$ $\le k_{{x}} \le 10^{-6}\text{ m}^{-1}$, derived from observations of prominence oscillations. Using the prominence-plasma parameters specified in Section 2.1 and $T_{0} = 6000$ K, Figure 3 shows that for the considered wavenumber interval the thermal radiation time is always much smaller than thermal conduction times. Also, it can be observed that the characteristic time for the neutrals thermal conduction is smaller than that of electrons, because at $T = 6000$ K the plasma is partially ionized, with a low ionization degree, which implies that the number of neutrals has increased and the number of free electrons has decreased. Furthermore, when the damping of prominence oscillations is considered, at small wavenumbers, radiation is the dominant mechanism, next, when the wavenumber is increased, thermal conduction becomes the dominant damping mechanism and, finally, for large wavenumbers the isothermal regime is attained. The end of the thermal-conduction dominance and the beginning of the isothermal regime can be also quantified following Porter, Klimchuk, and Sturrock (1994). For slow waves with $c_{\mathrm{s}}^{2}/v_{\mathrm{a}}^{2} \ll 1$ and assuming $\omega \approx c_{\mathrm{s}} k_{{x}}$, the critical $\omega$ at which the transition to the isothermal regime starts is given by

$$ \omega _{\mathrm{c}} = \frac{2 n_{\mathrm{0}}\mathrm{k_{B}} c_{\mathrm{s}}^{2}}{\kappa _{\parallel}\cos \theta}. $$

(64)

For $T = 6000$ K, ${\omega _{\mathrm{c}}} \approx 49\text{ rad}$ s⁻¹, corresponding to a period of 0.12 seconds. Therefore, the transition to the isothermal regime, in which temperature perturbations become zero, happens at a period much smaller than those observed in prominence oscillations (De Moortel and Hood, 2003; Carbonell et al., 2006).

On the other hand, we can make another different estimation of the importance of thermal conduction versus radiation, which is based on comparing thermal conduction and radiation times with the wave periods listed in Table 3. It is straightforward to observe that the logarithms of the wave periods shown in Table 3 are in the range 3 – 4, which are much smaller than the logarithms of thermal-conduction times shown in Figure 3. However, the logarithm of the radiation time for $T = 6000$ K is of order 4, which is quite close to the logarithms of the wave periods and, following what we have said in Section 1, this comparison of the temporal scales from radiation and observations is a potential way to discriminate between damping mechanisms, and it allows us to conclude, again, that thermal conduction can be neglected in our calculations.

Furthermore, the non-adiabatic sound speed in Equation 57 is also independent of $\tau _{\mathrm{\eta _{\mathrm{C}}}}$, therefore, taking into account all the above considerations, the dispersion relation to be solved is

$$\begin{aligned} &\omega ^{3} -\mathrm{i} \omega ^{2}\left ( \frac{1}{\tau _{\mathrm{r2}}} \right ) - c_{\mathrm{s}}^{2} k_{ {x}}^{2} \omega +\mathrm{i} c_{\mathrm{s}}^{2} k_{{x}}^{2} \left (\frac{1}{\tau _{\mathrm{r1}}} \right ) =0, \end{aligned}$$

(65)

and, in this case, the non-adiabatic sound speed reduces to,

$$\begin{aligned} \Delta ^{2} = \frac{c_{\mathrm{s}}^{2}}{\gamma} \frac{\left [\frac{\gamma}{\tau _{\mathrm{r1}}} + \mathrm{i} \gamma \omega \right ]}{\left [\frac{1}{\tau _{\mathrm{r2}}}+\mathrm{i} \omega \right ]}. \end{aligned}$$

(66)

Since we are interested in the temporal damping/amplification of oscillations, to solve Equation 65 we assume a standing wave, fix a real wavenumber [$k$] and seek a complex frequency $\omega = \omega _{\mathrm{r}} + \mathrm{i} \omega _{\mathrm{i}}$ where, following Equation 12, wave damping is characterized by $\omega _{\mathrm{i}} > 0$ while wave amplification corresponds to $\omega _{\mathrm{i}} < 0$. Furthermore, the imaginary root describing the behavior of the thermal wave is also obtained. In order to describe radiative losses, and such it is stated in Section 2.3, we consider two different optically thin functions expressed as $L_{r}(\rho , T) = \chi ^{*}\rho T^{\alpha}$, with $\chi ^{*} = 1.76 \times 10^{-13}$ and $\alpha = 7.4$ (Hildner, 1974), $\chi ^{*} = 2.02 \times 10^{-15}$ and $\alpha = 8.06$ (CHIANTIv7). Note that in all the radiative functions, the parameter $\alpha$ is positive. Regarding the heating function included in Equation 30, the constant $h_{\mathrm{0}}$ is obtained from the condition in Equation 24, while exponents $a$ and $b$ are considered as free parameters that can be zero, positive, or negative.

From Equation 65, analytical solutions for slow and thermal waves can be obtained by means of the symbolic language Mathematica, and these rather complicated solutions are shown in the Appendix. On the other hand, approximate analytical solutions would be useful to understand, in a qualitative way, the temporal behavior of the waves. Therefore, in the case of small wavenumbers, as those we are going to consider here, to obtain an analytical expression for the imaginary part of the slow wave frequency, we can substitute $\omega = \omega _{\mathrm{r}} + \mathrm{i} \omega _{\mathrm{i}}$ in Equation 65 and assuming that $\omega _{\mathrm{i}} \ll \omega _{\mathrm{r}}$, we neglect terms in $\omega _{\mathrm{i}}^{2}$ and $\omega _{\mathrm{i}}^{3}$ (Soler, 2010; Barceló, Carbonell, and Ballester, 2011). Following this approach, the real part is

$$\begin{aligned} \omega _{\mathrm{r}} = \sqrt{ \frac{\tau _{\mathrm{r2}}}{\tau _{\mathrm{r1}}}}c_{\mathrm{s}} k_{ {x}}, \end{aligned}$$

(67)

while the approximate imaginary part of the frequency is given by

$$\begin{aligned} \omega _{\mathrm{i}} = \frac{1}{2} \left ( \frac{\frac{1}{\tau _{\mathrm{r2}}} -\frac{1}{\tau _{\mathrm{r1}}}}{\tau _{\mathrm{r2}}^{-2}+c_{\mathrm{s}}^{2}k_{{x}}^{2}} \right )c_{\mathrm{s}}^{2}k_{{x}}^{2} \end{aligned}$$

(68)

and using Equation 67 we can write the imaginary part of the slow-wave frequency in terms of the real part,

$$\begin{aligned} \omega _{\mathrm{i}} = \frac{1}{2} \left ( \frac{\frac{1}{\tau _{\mathrm{r2}}} -\frac{1}{\tau _{\mathrm{r1}}}}{\tau _{\mathrm{r2}}^{-2}+\omega _{\mathrm{r}}^{2} \frac{\tau _{\mathrm{r2}}}{\tau _{r1}}} \right )c_{\mathrm{s}}^{2}k_{x}^{2}. \end{aligned}$$

(69)

Next, considering, again, small wavenumbers, from Equation 65 we can obtain an approximate analytical solution for the imaginary frequency of the thermal wave, which is

$$\begin{aligned} \omega _{\mathrm{ti}} = \frac{1}{\tau _{\mathrm{r2}}} \end{aligned}$$

(70)

Now, substituting Equations 67 and 68 in Equation 61, we obtain the following approximate expression for the slow-waves quality factor,

$$\begin{aligned} &Q = \frac{1}{2} \frac{\vert \omega _{r}\vert}{\omega _{\mathrm{i}}} = \frac{\vert \sqrt{\frac{\tau _{\mathrm{r2}}}{\tau _{\mathrm{r1}}}}\vert \left ( \tau _{\mathrm{r2}}^{-2} + c_{\mathrm{s}}^{2} k_{{x}}^{2}\right )}{\left (\frac{1}{\tau _{\mathrm{r2}}} - \frac{1}{\tau _{\mathrm{r1}}}\right )c_{\mathrm{s}} k_{{x}}} \end{aligned}$$

(71)

and from Equation 68 we can conclude that

$$ \frac{1}{\tau _{\mathrm{r2}}}- \frac{1}{\tau _{\mathrm{r1}}} \gtrless 0 $$

(72)

describes damping/amplification of slow waves, respectively, while from Equation 70 we can also conclude that

$$ \tau _{r2} \gtrless 0 $$

(73)

would describe damping/amplification of the thermal wave, respectively. From Equations 72 and 73 different inequalities involving parameters $a$, $b$, $\alpha$, and $\gamma$ can be derived but, as we have said before, the adiabatic exponent [$\gamma$] is not a constant but depends on the ionization degree. All these inequalities are summarized in Tables 1 and 2 (see also Zavershinskii et al. (2019), Kolotkov, Nakariakov, and Zavershinskii (2019), Kolotkov, Duckenfield, and Nakariakov (2020), Duckenfield, Kolotkov, and Nakariakov (2021), Zavershinskii et al. (2021)) for inequalities corresponding to coronal conditions in which thermal conduction time has been taken into account and the adiabatic exponent is $\gamma = 5/3$ since in coronal conditions plasma is fully ionized). Furthermore, using these inequalities, Kolotkov, Zavershinskii, and Nakariakov (2021) have derived thermal and acoustic Field’s lengths for the thermal and magnetoacooustic modes, respectively (Field, 1965).

Table 1 Conditions for damping/amplification of thermal and slow waves.

Table 2 Conditions for the sign of thermal-misbalance times.

On the other hand, from the dispersion relation for slow and thermal waves, described by the dispersion relation in Equation 59, the real and imaginary parts of the frequency of slow waves can also be written as

$$\begin{aligned} &\omega _{\mathrm{r}} = \Delta _{\mathrm{r}} k_{{x}}, \end{aligned}$$

(74)

$$\begin{aligned} &\omega _{\mathrm{i}} = \Delta _{\mathrm{i}} k_{{x}}, \end{aligned}$$

(75)

where $\Delta _{\mathrm{r}}$ and $\Delta _{\mathrm{i}}$ are the real and imaginary parts of the non-adiabatic sound speed $\Delta$.

Finally, since we want to use exponents $a$ and $b$ in the heating expression as free parameters, in principle, the number of possible combinations is infinite. Then, initially, we assume a numerical value for $b$ and seek values of $a$ that can give place to the different temporal behaviors for slow and thermal waves when Equation 65 is solved.

4 Effect of Partial Ionization

4.1 Qualitative Description

It is very common in research about prominences to consider prominence plasma as fully ionized, even at temperatures at which the plasma is partially ionized. When this approach is assumed, modifying the prominence temperature does not have any effect on the ionization degree, which is assumed to be constant and equal to unity, and on the numerical value of the adiabatic exponent, assumed to be equal to $5/3$. Therefore, a partially ionized prominence plasma behaves in the same way as any fully ionized plasma of the solar atmosphere. Here, by considering the prominence plasma as partially ionized, we would like to focus our research on the effect of partial ionization on the temporal behavior of slow and thermal waves. Therefore, and first of all, it is interesting to describe in a qualitative way what are the effects produced by a change of the ionization degree in the prominence-plasma parameters, as well as in the different equations that have been used. Since we are using an LTE approach, from the Saha equation we can modify the ionization degree in different ways: Keeping density constant and modifying prominence temperature, keeping temperature constant and modifying prominence density, or we could modify both quantities simultaneously. In our case, and as we said before, we have chosen to keep the density constant. Then, when we modify prominence temperature, from Equations 41 and 42 we can compute the new mean atomic weight and ionization degree which, at the same time, modifies the adiabatic exponent [$\gamma$]. However, not only the ionization is modified; the joint action of temperature, adiabatic exponent, and ionization-degree modifications contributes to modify the sound speed, which, from Equations 5 and 43, can be written as

$$ c_{\mathrm{s}} = \sqrt{ (1+i_{\mathrm{d}}) \gamma \mathrm{R} T_{0}} $$

(76)

with $i_{\mathrm{d}}$ the ionization degree and, at the same time, the sound speed $c_{\mathrm{s}}$ modifies the non-adiabatic sound speed in Equation 66. Furthermore, the adiabatic exponent [$\gamma$] enters in the expressions of the misbalance thermal times while radiative losses and the heating function are also modified by a change of prominence temperature; therefore, $\tau _{\mathrm{r1}}$ and $\tau _{\mathrm{r2}}$ will be also modified. These changes in the sound speed and in the thermal-misbalance times will modify the exact solutions corresponding to the frequencies of the slow and thermal waves shown in the Appendix. In particular, the adiabatic exponent [$\gamma$] will no longer be a constant anymore in the inequalities shown in Table 2 since in the case of a partially ionized hydrogen plasma, and assuming LTE conditions, it is given by (Hansen, Kawaler, and Trimble, 2004)

$$ \gamma = \frac{5 + \left (2.5 + \frac{\Psi}{\mathrm{k_{B}} T_{0}}\right )^{2} i_{\mathrm{d}} (1 - i_{\mathrm{d}})}{ 3 + \left [1.5 + \left (1.5 + \frac{\Psi}{\mathrm{k_{B}} T_{0}}\right )^{2} \right ] i_{\mathrm{d}} (1 - i_{\mathrm{d}}))}, $$

(77)

with $\Psi$ being the hydrogen-ionization potential. From this expression it can be seen that for a fully ionized or fully neutral hydrogen plasma $\gamma = 5/3$, while for temperatures around 6000 K the adiabatic exponent reaches a minimum value of about 1.1 (Ballester et al., 2021). Then, once the ionization degree is known, from Equation 77 the new value for $\gamma$ can be determined.

Summarizing, taking into account all the above modifications affecting different quantities and equations due to changes in the ionization degree, it is difficult to make a qualitative forecast of the expected changes in the temporal behavior of slow and thermal waves.

4.2 Effect of the Heating Function

For our study of the temporal behavior of slow and thermal waves, we are going to assume a heating mechanism, i.e. numerical values for $a$ and $b$, and we are going to modify prominence temperature. The considered prominence temperatures are in the range 9000 – 6000 K with the aim being to have different ionization degrees going from almost fully ionized to partially ionized plasma. Therefore, we consider the following temperatures: $T_{0} = 9000$ K, 8000 K, 7000 K, 6750 K, 6500 K, and 6000 K. Then, using the Saha equation (Equation 36), Equation 42, and Equation 77, we can compute the numerical values for $i_{\mathrm{d}}$ and $\gamma$, which for the different considered temperatures are 0.999 and 1.63, 0.995 and 1.42, 0.91 and 1.12, 0.82 and 1.09, 0.68 and 1.088, and 0.34 and 1.081, respectively. We will also consider a wavenumber $k_{{x}} = 10^{-8}\text{ m}^{-1}$ together with Hildner (1974) and CHIANTIv7 radiative-loss functions. Fixing the values of $a$ and $b$, exact solutions to Equation 65 have been computed using the above temperatures and parameters, which modify the numerical values of the thermal-misbalance times and sound speed [$c_{\mathrm{s}}$] in Equation 65.

Assuming $a=-1$ and $b=4$ and once the numerical values for $\tau _{\mathrm{r1}}$ and $\tau _{\mathrm{r2}}$ have been computed, from Table 1 we expect damping of slow waves. Figure 4 displays the temporal behavior of the non-dimensional perturbation of slow waves for both radiative-loss functions and as expected we observe damping of the slow waves, but at different rates. The first feature that we can observe is that all the curves are not in phase, which means that the period is different for the different temperatures and ionization degrees considered. This fact can be understood in the following way: when temperature and ionization degree decrease, sound speed [$c_{\mathrm{s}}$] also decreases, however, using our approximate solution (Equation 67), the behavior of the real part of the frequency also depends on the square root of the ratio between the characteristic misbalance times, which increases when temperature and ionization degree decrease. In our computations, since the wavenumber is considered constant, the interplay between these two factors produces a decrease of the real part of the frequency, which means that decreasing the ionization degree the period of the slow wave is increased. This behavior, computed using the exact frequencies, is shown in the left panel of Figure 5, which displays the behavior of the real part of the non-adiabatic sound speed with the ionization degree. Since $\omega _{\mathrm {r}} = \Delta _{\mathrm {r}} k_{x}$, the decrease of $\Delta _{\mathrm {r}}$ with the ionization degree means that $\omega _{\mathrm {r}}$ also decreases and, therefore, the period increases. From Figures 4 and 5 (left panel) we can observe that the shorter period corresponds to slow waves in almost fully ionized plasmas. On the other hand, the different damping rates, corresponding to different temperatures and ionization degrees, can be understood in terms of the quality factors shown in Figure 5 (right panel). In this figure, for both radiative-loss functions, all the quality factors are positive, which means that the imaginary part [$\omega _{\mathrm {i}}$] of the frequency is positive and, therefore, the oscillations are damped. Also, the different numerical values of the quality factors explain the different damping rates, and we can observe that for small ionization degrees the quality factor is positive but small, which means strong damping. In fact, for Hildner (1974) radiative-loss function the temporal damping is stronger than for CHIANTIv7 radiative-loss function.

Next we have considered a different heating mechanism with $a=2$ and $b=6$, and Figure 6 shows the temporal behavior of slow waves in this case. There is a strong difference with the previous case, since for Hildner (1974) radiative-loss function slow waves are amplified, while for CHIANTIv7 radiative-loss function they are damped which can be expected, again from Table 1. The most important difference between the two radiative functions considered is the numerical value of the parameter $\alpha$, which enters in all the inequalities shown in Table 2. We have computed the temporal behavior of the slow waves for the same temperatures and ionization degrees as before, and we find, again, that in both cases the period increases when the ionization degree decreases (Figure 6 and Figure 7 (left panel)). Also, different growing and damping rates are found, which, again, can be understood in terms of the quality factors shown in Figure 7 (right panel) where, again, we observe that small positive/negative quality factors correspond to strong damping/amplification of the slow waves. For the Hildner (1974) radiative-loss function, the quality factors are negative, which means that the imaginary part of the frequency is negative leading to amplification, while for the CHIANTIv7 radiative-loss function, the quality factors are positive, which means that the imaginary part of the frequency is positive leading to damping of the oscillations. If we take into account our approximate expression for the imaginary part of the frequency (Equation 68), the change of the imaginary part from negative to positive is controlled by the numerator $\frac{1}{\tau _{\mathrm{r2}} }-\frac{1}{\tau _{\mathrm{r1}} }$, which changes its sign when, as in this case, we consider two different radiative-loss functions.

Furthermore, one can consider another possibility, which is a change of the temporal behavior of the slow wave, from damping to amplification or vice versa, when a particular combination of $a$ and $b$ is considered. Assuming $a =2$, $b =5$, when a certain value of the ionization degree is attained the above-mentioned behavior is found which is due, again, to a change of sign in $\frac{1}{\tau _{\mathrm{r2}} }-\frac{1}{\tau _{\mathrm{r1}}}$ while the ionization degree is being modified. Figure 10 displays, for Hildner (1974) and CHIANTIv7 radiative-loss functions, the behavior of the imaginary part of the non-adiabatic sound speed versus the ionization degree. It can be seen that in the case of Hildner (1974) radiative-loss function, and for a particular value of the ionization degree, the imaginary part of the non-adiabatic sound speed changes from negative to positive, which means that the imaginary part of the frequency also changes its sign. As a consequence, the temporal behavior of the nondimensional velocity perturbations changes from amplification ($\omega _{\mathrm{i}} < 0$) to damping ($\omega _{\mathrm{i}} > 0$).

The above-considered cases show that, once a radiative function has been chosen and a parallel wavenumber has been fixed, by playing with the values of $a$ and $b$ it is possible to obtain damping as well as amplification of slow waves. In summary, in spite of the multiple modifications of parameters (sound speeds, thermal misbalance times, ionization degree, adiabatic exponent, etc.) produced by a modification of prominence temperature, the temporal behavior of slow waves follows the rules in Table 2.

On the other hand, for $a=-1$, $b = 4$ and the same two radiative-loss functions, the behavior of the imaginary frequencies of the thermal wave versus ionization degree is shown in Figure 8 (left panel), pointing out a decrease of these frequencies when the ionization degree decreases. This behavior means that the damping time for the thermal wave is smaller for almost fully ionized plasmas.

4.3 Effect of the Parallel Wavenumber

Another parameter that can be modified in our computations is the parallel wavenumber: $k_{{x}}$. Once a constant temperature has been assumed, which determines the ionization degree, a change of the parallel wavenumber does not affect the characteristic times of thermal misbalance. However, the exact solutions shown in the Appendix point out that slow and thermal waves are affected by $k_{{x}}$. For instance, the approximate expression for the imaginary frequency of the thermal wave is the inverse of $\tau _{\mathrm{r2}}$ (see Equation 70) independent of the wavenumber and suggesting that it is a constant; however, Figure 8 (right panel) shows how the exact imaginary frequency of the thermal wave changes with the parallel wavenumber. It can be seen that in some interval of wavenumbers the imaginary frequency of the thermal wave is significantly modified while for the rest of the wavenumber interval this frequency becomes almost constant.

On the other hand, to try to understand how the real and imaginary parts of the slow-wave frequency depend on the parallel wavenumber, we can take advantage of Equations 74 and 75, which become $\omega _{\mathrm{r}} = c_{\mathrm{s}} k_{{x}}$ and $\omega _{\mathrm{i}} = 0$ in the adiabatic case. Therefore, in this case, for a fixed temperature and ionization degree, the adiabatic sound speed is constant and the frequency grows linearly with the wavenumber. However, in our case, Figure 9 shows the behavior of the real (left panel) and imaginary (right panel) parts of the slow-wave frequency versus the parallel wavenumber. We can observe that the dependence of both frequencies on the parallel wavenumber is not linear, but seems to be parabolic. The reason for this behavior is that the real and imaginary parts of the non-adiabatic sound speed are not constants, but depend on the wave frequency. Of course, this behavior of real and imaginary parts of the frequency with the parallel wavenumber determines the behavior of the quality factor.

Finally, it is worth to point out that, in the coronal context, conditions for the stability of thermal and slow magnetoacoustic waves have been obtained (Kolotkov, Zavershinskii, and Nakariakov, 2021) and that thermal misbalance could produce that thermal and magnetoacoustic modes be stable or unstable (Kolotkov, Zavershinskii, and Nakariakov, 2021).

4.4 Optically Thick Losses

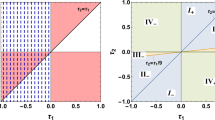
Up to now, we have only considered optically thin radiative losses; however, functions describing severely reduced radiative losses, as shown in Figure 2, can also be considered (Rosner, Tucker, and Vaiana, 1978; Milne, Priest, and Roberts, 1979). A similar analysis as for optically thin losses could be undertaken, but instead of repeating the same computations, we could use a different approach taking advantage of the inequalities shown in Tables 1 and 2. In this case, we fix a value for $a$ and once an optically thick function has been chosen, which provides us with the numerical value for $\alpha$; we could obtain the numerical value of $b$ that satisfies one of the inequalities $\frac{1}{\tau _{\mathrm{r2}}}-\frac{1}{\tau _{\mathrm{r1}}} > 0$ or $\frac{1}{\tau _{\mathrm{r2}}}-\frac{1}{\tau _{\mathrm{r1}}} < 0$ in Table 2.

For instance, for $T = 9000$ K and the Milne, Priest, and Roberts (1979) radiative function, $\alpha = 17.4$, then, fixing $a = 2$, and considering the case of $\frac{1}{\tau _{\mathrm{r2}}}-\frac{1}{\tau _{\mathrm{r1}}} < 0$, from $\alpha < \frac{a-1}{\gamma -1} + b$, which implies amplification of slow waves, we have $b > 15.9$, and from $\alpha < b-a+1$, which implies $\tau _{\mathrm{r2}} < 0$, giving place to the amplification of thermal perturbation, we also have $b > 18.4$. Taking $b =$ 17 we satisfy the first condition and, therefore, slow waves are amplified, while the thermal perturbation is damped. On the other hand, when $b = 19$, both conditions are satisfied and slow waves are amplified, while thermal perturbations are also amplified. However, the numerical values of $b$ are modified depending on the ionization degree, for instance, with $T = 6000$ K, $\gamma = 1.08$ and then $b > 4.9$, which is more consistent with previous values considered in the case of optically thin radiative losses. The same approach can be followed with the radiative function from Rosner, Tucker, and Vaiana (1978), in which $\alpha = 30$, although in this case the numerical values for $b$ are even greater than with the previous function, even for the case when $T = 6000$ K when $b$ must be greater than 17.5 to fulfill the requested inequality. In summary, considering optically thick radiative losses damping/amplification of thermal and slow waves can also be obtained but in both cases the high values of parameter $\alpha$ lead to high values of the parameter $b$ once a value for $a$ has been fixed. However, these high values of the parameter $b$ could be unrealistic, would place under question the linearization of the heat-loss function, and would suggest that, probably, optically thick losses from prominences would need a different parameterization.

4.5 Comparison with Observations

In order to compare our theoretical results with those coming from small-amplitude oscillations in prominences, and assuming that slow magnetoacoustic waves are responsible for the oscillations, we will perform a comparison with two observational results reported by Molowny-Horas et al. (1999) (see Figure 1 and Table 3). From the expressions for the quality factor (Equation 61) and the ratio between damping/amplifying times and periods [$\tau _{\textrm{D}}/T$], we can obtain a relationship which is

$$ Q = \pi \frac{\tau _{\textrm{D}}}{T}. $$

(78)

Then, the quality factors for the observational data in Molowny-Horas et al. (1999) have been computed and are shown in Table 3. In all of the cases except one, the quality factor indicates the presence of strong or intermediate damping, while in the remaining case, the quality factor indicates amplification. Now, we can try to compare observational and theoretical non-dimensional velocity perturbations corresponding to two different observational data (Locations 1 and 6 in Table 3) from Molowny-Horas et al. (1999). In both cases we have used the Hildner (1974) radiative functions and assumed values for $k_{{x}}$ and temperature, which determines the ionization degree through the Saha equation. Knowing the real and imaginary frequencies from observational data, we have used the approximate solutions given by Equations 67 and 68 to determine $\tau _{\mathrm{r1}}$ and $\tau _{\mathrm{r2}}$. Next, from Equations 50 and 51 we can compute approximate values for parameters $a$ and $b$. Since parameters $a$ and $b$ have been determined using approximate solutions of the dispersion relation, we use the exact solutions for the frequencies from the Appendix to slightly refine the values of $a$ and $b$ by performing an iterative process. The comparisons between observational and theoretical curves obtained following the procedure above described are shown in Figure 11. For Location 1 (Figure 11 left panel), the observational quality factor is 6.2, which means strong damping, while the theoretical one is 6.82. For Location 6 (Figure 11 right panel) the observational quality factor is −15.7, which means amplification, while the theoretical one is −13.35. In both cases, the agreement between observational and theoretical curves is reasonably good, even though that we have not computed the goodness of fit, since we only want to point out that it is possible to try to match observational with theoretical results following the described procedure. Finally, this procedure is not straightforward because, as we have said above, key parameters to be chosen are the wavenumber and temperature, which are important factors for the real and imaginary parts of the frequency and, if a proper matching can not be found, these parameters must be modified, and this choice could need several iterations as well as for the refinement of $a$ and $b$.

Figure 1

Doppler velocities (km s⁻¹) as a function of time for six different spatial locations in a prominence. The dots denote observational data, while the solid lines correspond to an analytical fit such as: $v = v_{\mathrm{0}} \cos (\frac{2 \pi}{P}t +\phi ) \mathrm{e}^{-t/ \tau _{\mathrm{D}}}$, with $v_{\mathrm{0}}$ the initial velocity amplitude, $P$ the period, $\tau _{\mathrm{D}}$ the damping time, and $\phi$ the phase. Adapted from Molowny-Horas et al. (1999).

Figure 2

Logarithm of the radiative losses versus temperature: CHIANTIv7 (blue line); Hildner (1974) (red line); Milne, Priest, and Roberts (1979) (green line). $\rho _{\mathrm{0}} = 4 \times 10^{-11}$ kg m⁻³.

Figure 3

Logarithms of characteristic time scales for radiation and thermal conduction for electrons and neutrals versus parallel wavenumber. Thermal-conduction time for electrons (blue line); Thermal-conduction time for neutrals (red line); Thermal-radiation time (black line). The rest of horizontal lines correspond to the periods of oscillations in Table 3. Hildner (1974) radiative-loss function. $T_{0} = 6000$ K, $\rho _{\mathrm{0}} = 4 \times 10^{-11}$ kg m⁻³.

Figure 4

Left panel: Comparison of the temporal behavior of the non-dimensional velocity perturbation of the slow waves for $T_{0} = 9000$ K, $\gamma = 1.63$ (red), $T_{0} = 8000$ K, $\gamma = 1.41$ (blue), $T_{0} = 7000$ K, $\gamma = 1.11$ (black), $T_{0} = 6750$ K, $\gamma = 1.09$ (brown), $T_{0} = 6500$ K, $\gamma = 1.088$ (green), $T_{0} = 6000$ K, $\gamma = 1.081$ (magenta). Hildner (1974) radiative-loss function. Right panel: Same comparison but for CHIANTIv7 radiative-loss function. $k_{x} = 10^{-8}$ m⁻¹, $b=4$, $a = -1$.

Figure 5

Slow waves. Left panel: Real part of the non-adiabatic sound speed versus ionization degree for Hildner (1974) (red dots) and CHIANTIv7 (blue squares) radiative-loss functions. Right panel: Quality factors versus ionization degree for Hildner (1974) (red dots) and CHIANTIv7 (blue squares) radiative-loss functions. $k_{x} = 10^{-8}$ m⁻¹, $b=4$, $a = -1$.

Figure 6

Left panel: Comparison of the temporal behavior of the non-dimensional velocity perturbation of the slow waves for $T_{0} = 9000$ K, $\gamma = 1.63$ (red), $T_{0} = 8000$ K, $\gamma = 1.41$ (blue), $T_{0} = 7000$ K, $\gamma = 1.11$ (black), $T_{0} = 6750$ K, $\gamma = 1.09$ (brown), $T_{0} = 6500$ K, $\gamma = 1.088$ (green), $T_{0} = 6000$ K, $\gamma = 1.081$ (magenta). Hildner (1974) radiative-loss function. Right panel: Same comparison, but for the CHIANTIv7 radiative-loss function. $k_{x} = 10^{-8}$ m⁻¹, $b=6$, $a = 2$.

Figure 7

Slow waves. Left panel: Real part of the non-adiabatic sound speed versus ionization degree for Hildner (1974) (red dots) and CHIANTIv7 (blue squares) radiative-loss functions. Right panel: Quality factors versus ionization degree for Hildner (1974) (red dots) and CHIANTIv7 (blue squares) radiative-loss functions. $k_{x} = 10^{-8}$ m⁻¹, $b=6$, $a = 2$.

Figure 8

Left panel: Imaginary part of the frequency of the thermal wave versus ionization degree for Hildner (1974) (red dots) and CHIANTIv7 (blue squares) radiative-loss functions. Right panel: Imaginary part of the frequency of the thermal wave versus parallel wavenumber. $T_{0} = 6000$ K. $a = -1$, $b=4$. Hildner (1974) radiative-loss function (red dots).

Figure 9

Left panel: Real part of the frequency of slow wave versus the parallel wavenumber. Right panel: Imaginary part of the frequency of slow wave versus the parallel wavenumber. $T_{0} = 6000$ K. $a = -1$, $b=4$. Hildner (1974) radiative-loss function (red dots).

Figure 10

Imaginary part of the non-adiabatic sound speed versus the ionization degree for Hildner (1974) (red dots) and CHIANTIv7 (blue squares) radiative-loss functions. $\rho _{\mathrm{0}} = 4 \times 10^{-11}~\text{kg}\,\mbox{m}^{-3}$, $k_{x} = 10^{-8}~\text{m}^{-1}$, $b=5$, $a = 2$.

Figure 11

Left panel: Temporal behavior of the non-dimensional velocity perturbation from Molowny-Horas et al. (1999) (Location 1, $T = 70$ minutes, $\tau _{\textrm{D}} = 140$ minutes) (blue); Temporal behavior of the theoretical non-dimensional velocity perturbation of the slow waves for $a = 0.93$, $b=7.4$, $k_{x} = 10^{-7}$ m⁻¹, $T_{0} = 9000$ K (red). Right panel: Temporal behavior of the non-dimensional velocity perturbation from Molowny-Horas et al. (1999) (Location 6, $T = 28$ minutes, $\tau _{\textrm{D}} = -140$ minutes) (blue); Temporal behavior of the theoretical non-dimensional velocity perturbation of the slow waves for $a = 1.07$, $b=7.4$, $k_{x} = 2 \times 10^{-7}$ m⁻¹ (red). $T_{0} = 9000$ K, Hildner (1974) radiative-loss function.

Table 3 Location, Period, Damping/Amplifying time, Damping/Amplifying time divided by Period, Quality factor $Q$. All times are given in minutes. Observational data from Molowny-Horas et al. (1999). SD: Strong damping; D: Damping; A: Amplification. The units of $\tau _{\mathrm{D}}$ and $T$ are minutes.

In other observational results (Terradas et al., 2002; Lin, 2004), the reported ratio between damping/amplifying times and periods is about two to three, which means that the quality factor is between 6.28 and 9.5, indicating strong damping or amplification in some cases, a behavior which, in principle, could also be reproduced following the above fitting procedure. These results point out that the consideration of a thermal mechanism with a heating dependent on powers of density and temperature can help us understand the damping or amplification of oscillations.

4.6 Oblique Propagation

When Equation 45 is expanded and, as before, thermal conduction is neglected compared to radiation, we obtain

$$\begin{aligned} &\omega ^{5} - \mathrm{i} \left ( \frac{1}{\tau _{\mathrm{\eta _{\mathrm{C}}}}} + \frac{1}{\tau _{\mathrm{r2}}} \right ) \omega ^{4}-\left (k^{2}(c_{ \mathrm{s}}^{2}+v_{\mathrm{a}}^{2})+ \frac{1}{\tau _{\mathrm{\eta _{\mathrm{C}}}}} \frac{1}{\tau _{\mathrm{r2}}} \right ) \omega ^{3} + \\ &+ \mathrm{i} k^{2}\left ( \frac{ c_{\mathrm{s}}^{2}}{\tau _{\mathrm{\eta _{\mathrm{C}}}}}+ \frac{ c_{\mathrm{s}}^{2}}{\tau _{\mathrm{r1}}} + \frac{v_{\mathrm{a}}^{2}}{\tau _{\mathrm{r2}}}\right ) \omega ^{2}+k^{2} c_{\mathrm{s}}^{2} \left (v_{\mathrm{a}}^{2} k_{{x}}^{2}+ \frac{1}{\tau _{\mathrm{\eta _{\mathrm{C}}}}} \frac{1}{\tau _{\mathrm{r1}}}\right ) \omega - \\ &-\mathrm{i} k^{2} k_{{x}}^{2} v_{\mathrm{a}}^{2} c_{ \mathrm{s}}^{2} \left (\frac{1}{\tau _{\mathrm{r1}}}\right )=0, \end{aligned}$$

(79)

describing coupled slow, thermal, and fast waves, with $k^{2} = k_{{x}}^{2} + k_{\mathrm{{\perp}}}^{2}$. The polynomial has one purely imaginary root corresponding to the thermal wave, while the rest of the roots consist of two pairs, one pair represents slow waves, whereas the other represents the fast waves. For each pair $(\omega _{\mathrm{1}},\omega _{\mathrm{2}})$, $\omega _{\mathrm{1}} = \omega _{\mathrm{r}} + \mathrm{i} \omega _{ \mathrm{i}}$ and $\omega _{\mathrm{2}} = - \omega _{\mathrm{r}} + \mathrm{i} \omega _{ \mathrm{i}}$ (Carbonell, Oliver, and Ballester, 2004).

In this case we have an additional ingredient with respect to the previous case, which is the presence of the characteristic time [$\tau _{ \mathrm{\eta _{\mathrm{C}}}}$] corresponding to Cowling’s resistivity. The contribution of Cowling’s resistivity characteristic time (Equation 56) must be considered in terms of wavenumber [$k$] and temperature [$T$] which determines the ionization degree. Decreasing temperature and increasing the wavenumber enhances Cowling’s resistivity and decreases the characteristic time (see Equation 56). In our case, taking into account typical prominence temperatures and wavenumbers from the considered interval, we can estimate the importance of terms $\frac{1}{\tau _{\mathrm{\eta _{\mathrm{C}}}}} \frac{1}{\tau _{\mathrm{r2}}}$ and $\frac{1}{\tau _{\mathrm{\eta _{\mathrm{C}}}}} \frac{1}{\tau _{\mathrm{r1}}}$. Figure 12 shows the behavior of the logarithms of $\frac{1}{\tau _{\mathrm{\eta _{\mathrm{C}}}}} \frac{1}{\tau _{\mathrm{r2}}}$, $\frac{1}{\tau _{\mathrm{\eta _{\mathrm{C}}}}} \frac{1}{\tau _{\mathrm{r1}}}$, and $k_{{x}}^{2} v_{\mathrm{a}}^{2}$ versus parameters $a$ and $b$ for $T = 6000$ K, where we have taken into account the correct value for $\gamma$. This figure points out that both terms are negligible, not only by themselves but also as compared with other terms in the coefficients of the dispersion relation (Equation 79) such as $k_{{x}}^{2}(c_{\mathrm{s}}^{2} + v_{\mathrm{a}}^{2})$. Therefore, if under these conditions of partial ionization we consider oblique propagation the only influence on the damping of slow and fast waves will come from the terms with $\frac{1}{\tau _{\mathrm{r1}}}$ and $\frac{1}{\tau _{\mathrm{r2}}}$. In order to have $\tau _{\mathrm{\eta _{\mathrm{C}}}} \ll \tau _{\mathrm{r1}}$ and $\tau _{\mathrm{\eta _{\mathrm{C}}}} \ll \tau _{\mathrm{r2}}$, we would need to consider low temperatures, unrealistic for prominence plasmas, which increases $\eta _{\mathrm{C}}$, as well as large wavenumbers (Soler, 2010).

Figure 12

Comparison of the logarithms of $\frac{1}{\tau _{\textrm{r}1} \tau _{\eta _{\mathrm{C}}}}$ (${\mathit{red}}$), of $\frac{1}{\tau _{\textrm{r}2} \tau _{\eta _{\mathrm{C}}}}$ (${\mathit{blue}}$) and of $k_{x}^{2} v_{a}^{2}$ (${\mathit{green}}$) versus $a$ and $b$. $T = 6000$ K. $k = 10^{-7}\text{ m}^{-1}$. Hildner (1974) radiative-loss function.

A different approach to understand the role played by Cowling’s resistivity is to consider the adiabatic case (Soler, 2010). Then the characteristic times, $\tau _{\textrm{r}1}$ and $\tau _{\textrm{r}2}$, become infinite, which means that the thermal wave is removed, and the dispersion relation is

$$\begin{aligned} &\omega ^{4} - \mathrm{i} \left ( \frac{1}{\tau _{\mathrm{\eta _{\mathrm{C}}}}} \right ) \omega ^{3}- \left (k^{2}(c_{\mathrm{s}}^{2}+v_{\mathrm{a}}^{2}) \right ) \omega ^{2} + \mathrm{i} k^{2}\left ( \frac{ c_{\mathrm{s}}^{2}}{\tau _{\mathrm{\eta _{\mathrm{C}}}}} \right ) \omega +k^{2} c_{\mathrm{s}}^{2} v_{\mathrm{a}}^{2} k_{ \mathrm{x}}^{2}=0, \end{aligned}$$

(80)

describing coupled slow and fast modes. Since for the same wavenumber the frequency of fast modes is greater than that of slow modes, we can assume that the higher-order terms in the frequency are related to the fast modes; then, the above dispersion relation becomes

$$\begin{aligned} (c_{\mathrm{s}}^{2}+v_{\mathrm{a}}^{2}) \omega ^{2} - \mathrm{i} \left ( \frac{ c_{\mathrm{s}}^{2}}{\tau _{\mathrm{\eta _{\mathrm{C}}}}} \right ) \omega - c_{\mathrm{s}}^{2} v_{\mathrm{a}}^{2} k_{x}^{2}=0, \end{aligned}$$

(81)

and the frequencies of slow modes are

$$\begin{aligned} \omega = \frac{\pm {\sqrt{4 c_{\mathrm{s}}^{2} k_{{x}}^{2} v_{\mathrm{a}}^{2} (c_{\mathrm{s}}^{2} + v_{\mathrm{a}}^{2})-\frac{c_{\mathrm{s}}^{4}}{\tau _{\mathrm{\eta _{\mathrm{C}}}}^{2}}}+\frac{\mathrm{i} c_{\mathrm{s}}^{2}}{\tau _{\mathrm{\eta _{\mathrm{C}}}}}}}{2(c_{\mathrm{s}}^{2} + v_{\mathrm{a}}^{2})} \end{aligned}$$

(82)

which in this approximation would describe the influence of Cowling’s resistivity on slow waves. Therefore, in this approximation, the real and imaginary parts of the slow-mode frequency could be modified by Cowling’s characteristic time $\tau _{\mathrm{\eta _{\mathrm{C}}}}$. However, using our prominence physical conditions, in the real part of the slow-wave frequency the term $\frac{c_{\mathrm{s}}^{4}}{\eta _{\mathrm{C}}^{2}}$ is much smaller than the other term inside the square root and, therefore, it can be neglected. Furthermore, in the imaginary part of the slow-wave frequency the ratio $\frac{c_{\mathrm{s}}^{2}}{\eta _{\mathrm{C}}2(c_{\mathrm{s}}^{2} + v_{\mathrm{a}}^{2})}$ can also be neglected in prominence conditions. Therefore, once these terms have been neglected, there is no influence of Cowling’s resistivity on slow waves, and its frequency can be written as

$$\begin{aligned} \omega = \pm \frac{c_{\mathrm{s}} v_{\mathrm{a}}}{\sqrt{(c_{\mathrm{s}}^{2} + v_{\mathrm{a}}^{2})}} k_{{x}}, \end{aligned}$$

(83)

which for $c_{\mathrm{s}} \ll v_{\mathrm{a}}$, as in our case, becomes the frequency of adiabatic slow waves $\omega = c_{\mathrm{s}} k_{{x}} = c_{\mathrm{s}} k \cos \theta$, with $\theta$ the angle between the magnetic field and the wavevector $k$. In the above equation, $c_{\mathrm{s}}$ is given by Equation 76, which means that the frequency of adiabatic slow waves depends on the ionization degree [$i_{\textrm{d}}$] which, at the same time influences the value of $\gamma$, this being the only influence of partial ionization in oblique propagation. Therefore, keeping $k$ and $\theta$ constant, in the case of fully ionized plasma the frequency of slow waves would be greater than in the case of partial ionization, and this frequency decreases when the ionization degree is decreased since the adiabatic exponent also decreases.

This conclusion also applies to the slow-wave frequencies obtained from Equation 79 when terms involving Cowling’s resistivity are neglected, since, as stated above, they are only important in the case of low temperatures and large wavenumbers, and only terms related to the thermal characteristic times appear in the fifth-degree dispersion relation.

5 Summary and Conclusions

Because of phase speed and observed periods, some observations of small-amplitude oscillations in prominences suggest that slow magnetoacoustic waves are responsible for these oscillations. Besides, observations have also reported temporal damping of these oscillations as well as, in a few cases, amplification. In both cases, the ratio between damping/amplifying times and periods is small, suggesting strong damping/amplification. In interpreting these oscillations in terms of MHD waves, mechanisms for wave damping have been proposed to explain the attenuation of the oscillations, although it has been difficult to match the strong damping found in observational data as well as to explain the cases of temporal amplification. Therefore, considering a heating–cooling misbalance, we have tried to find the needed conditions for the temporal damping/amplification of oscillations. To this end, we have taken into account single-fluid MHD equations considering a non-adiabatic energy equation including radiation, thermal conduction, and a heating term that depends on powers of the density and temperature through exponents $a$ and $b$ which we consider as free parameters. By linearizing these equations, we have derived, in the case of parallel propagation, the dispersion relation for coupled slow and thermal waves when only radiation and heating are taken into account. Our theoretical results have been obtained by solving this dispersion relation, which provides us with the exact frequencies for slow and thermal waves; however, for a better understanding of the temporal behavior of these exact solutions, we have searched for approximate analytical solutions which, within the wavenumber interval considered, provide with an excellent guide to understanding the temporal behavior of the exact solutions. Using these approximate solutions, we were able to derive a set of inequalities that represent the conditions needed for the damping/amplification of slow and thermal waves. Once the parameters $a$ and $b$ have been fixed and a radiative function has been chosen, these inequalities allow us to know, in a simple way, the approximate temporal behavior of slow and thermal waves. In this study, we have kept the strength of the magnetic field and density as constants, while we have modified the considered wavenumber and the prominence-plasma temperatures. Starting from a fully ionized prominence plasma, and a fixed wavenumber, when plasma temperature is decreased, the ionization degree is also decreased and, consequently, the adiabatic exponent is also decreased, and the results indicate that the damping or amplification is enhanced, which can be understood from the behavior of quality factors. Furthermore, it is also observed that the oscillatory periods become smaller when the ionization degree is increased. These two features can be understood by looking at the behavior of the real and imaginary parts of the non-adiabatic sound speed versus the ionization degree, both quantities decrease when the ionization degree decreases and, for a fixed wavenumber, they determine the behavior of the real and imaginary parts of the slow wave frequencies.

On the other hand, fixing the ionization degree, when the wavenumber is increased, the real and imaginary parts of the slow waves frequency are also increased, which indicates that the period decreases while damping or amplification are enhanced, but this increase is not linear but seems to be parabolic. This is due to the fact that the real and imaginary parts of the non-adiabatic sound speed are not constant, but depend on the wave frequency.

The modifications of the physical conditions of prominence plasma and wavenumber together with changes of radiative functions and heating terms, through exponents $a$ and $b$, give rise to significant changes in the temporal behavior of slow and thermal waves. Our results show that different rates of amplification and damping as well as almost constant amplitude, in the case of very weak damping or amplification, can be obtained, and the rates of amplification or damping of slow waves have been expressed in terms of a quality factor $Q$.

On the other hand, from the data about periods and damping/amplifying times in the observed small-amplitude oscillations by Molowny-Horas et al. (1999), which have been interpreted in terms of slow waves, observational quality factors can be derived. These observational quality factors are positive, except for one which is negative, and they can be compared with our theoretical results. This comparison shows that with a suitable combination of radiative functions and $a$ and $b$ parameters, it is possible to obtain theoretical quality factors closely matching the different observational quality factors. Besides, as one attempt to apply our theoretical results, we have tried to reproduce two observational curves from Molowny-Horas et al. (1999) representing the temporal behavior of the Doppler velocity, one showing attenuation and the other displaying amplification. Finally, oblique propagation has also been considered, however, although in this case Cowling’s resistivity appears in the corresponding dispersion relation, the effect on slow and thermal waves is negligible, and only would affect fast waves, which are not considered in this study.

The main conclusion that we can draw from this study is that the effect of considering thermal misbalance with heating mechanism, which depends on powers of density and temperature through the free parameters $a$ and $b$, together with typical wavenumbers of prominence oscillations and the effect of partial ionization, allows us to obtain a variety of different temporal behaviors for the slow magnetoacoustic waves, representing all types of damping/amplification, which can be used to match observational data. Therefore, under the assumption that slow magnetoacoustic waves are responsible for the oscillations, our theoretical results can be useful to explain the strong temporal damping/amplification reported in prominence small-amplitude oscillations.

Appendix

The exact solutions to Equation 65 are given by

$$\begin{aligned} & \omega _{1} = \left [- \left ( \left ( 2^{1/3} \left ( -3 c_{\textrm{s}}^{2} k_{x}^{2} + \frac{1}{\tau _{\textrm{r}2}^{2}} \right )\right )/\right . \right . \\ & \left ( 3 \left ( - \frac{27\ \mathrm{i} \ c_{\textrm{s}}^{2}k_{x}^{2}}{\tau _{\mathrm{r}1}} + \sqrt{{4} \left ( - 3\ c_{\textrm{s}}^{2} k_{x}^{2} + \frac{1}{\tau _{\mathrm{r}2}^{2}}\right )^{3} + \left ( - \frac{27 \ \mathrm{i} \ c_{\textrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}1}}- \frac{2 \mathrm{{i} }}{\tau _{\mathrm{r}2}^{3}}+ \frac{9\ \mathrm{i}\ c_{\textrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}2}}\right )^{2}} - \right . \right . \\ & \left . \left .\left .\frac{2 \mathrm{{i}}}{\tau _{\mathrm{r}2}^{3}} + \frac{9 \mathrm{i} c_{\textrm{s}}^{2} k_{x}^{2} }{\tau _{\mathrm{r}2}} \right )^{1/3} \right )\right ) + \\ & \frac{ \left ( - \frac{27 \mathrm{i} c_{\textrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}1}}+ \sqrt{4 (-3 c_{\textrm{s}}^{2} k_{x}^{2} + \frac{1}{\tau _{\mathrm{r}2}^{2}})^{3} + (- \frac{27 \mathrm{i} c_{\textrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}1} 2^{3}} + \frac{9 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}2}})^{2}} - \frac{2 \mathrm{i}}{\tau _{\mathrm{r}2}^{3}}+ \frac{9 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau ^{2}} \right )^{1/3}}{3 \times 2^{1/3}} \\ & \left . + \frac{\mathrm{i}}{3 \tau _{\mathrm{r}2}} \right ], \end{aligned}$$

(84)

$$\begin{aligned} & \omega _{2} =\left [ \left ( (1 + \mathrm{i} \sqrt{3}) \left ( - 3 \ c_{\mathrm{s}}^{2} k_{x}^{2} + \frac{1}{\tau _{\mathrm{r}2}^{2}} \right )\right ) \right . / \left ( 3 \times 2^{2/3} \right . \\ & \left ( - \frac{27\mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2} }{\tau _{\mathrm{r}1}} + \sqrt{4 \left (-3 c_{\mathrm{s}}^{2} k_{x}^{2} + \frac{1}{\tau _{\mathrm{r}2}^{2}} \right )^{3} + \left ( - \frac{27 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}1}}- \frac{2 \mathrm{i}}{\tau _{\mathrm{r}2}^{3}}+ \frac{9 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}2}}\right )^{2} - } \right . \\ & \left . \left .\frac{2 \mathrm{i}}{\tau _{\mathrm{r}2}^{3}} + \frac{9 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}2}} \right )^{1/3} \right ) - \frac{1}{6 \times 2^{1/3}} \left (1 - \mathrm{i} \sqrt{3} \right ) \\ & \left ( - \frac{27 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}1}} + \sqrt{4 \left (-3 c_{\mathrm{s}}^{2} k_{x}^{2} + \frac{1}{\tau _{\mathrm{r}2}^{2}} \right )^{3} + \left ( - \frac{27 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}1}}- \frac{2 \mathrm{i}}{\tau _{\mathrm{r2}}^{3}}+ \frac{9 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}2}}\right )^{2} - } \right . \\ & \left . \left . \frac{2 \mathrm{i}}{\tau _{\mathrm{r}2}^{3}} + \frac{9 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}2}} \right )^{1/3} + \frac{1}{3 \tau_{\mathrm{r}2} ^{2}} \right ] , \end{aligned}$$

(85)

$$\begin{aligned} & \omega _{3} = \left [ \left ( (1 - \mathrm{i} \sqrt{3}) \left (- 3 c_{\mathrm{s}}^{2} k_{x}^{2} + \frac{1}{\tau _{\mathrm{r}2}^{2}}\right )\right ) / \left ( 3 \times 2^{2/3} \right .\right . \\ & \left ( - \frac{27\mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2} }{\tau _{\mathrm{r}1}} + \sqrt{4 \left (-3 c_{\mathrm{s}}^{2} k_{x}^{2} + \frac{1}{\tau _{\mathrm{r}2}^{2}} \right )^{3} + \left ( - \frac{27 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}1}}- \frac{2 \mathrm{i}}{\tau _{\mathrm{r}2}^{3}}+ \frac{9 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}2}}\right )^{2} - } \right . \\ & \left . \left . \frac{2 \mathrm{i}}{\tau _{\mathrm{r}2}^{3}} + \frac{9 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}2}} \right )^{1/3} \right ) - \frac{1}{6 \times 2^{1/3} }(1 + \mathrm{i} \sqrt{3} ) \\ & \left ( - \frac{27\mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2} }{\tau _{\mathrm{r}1}} + \sqrt{4 \left (-3 c_{\mathrm{s}}^{2} k_{x}^{2} + \frac{1}{\tau _{\mathrm{r}2}^{2}} \right )^{3} + \left ( - \frac{27 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}1}}- \frac{2 \mathrm{i}}{\tau _{\mathrm{r}2}^{3}}+ \frac{9 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}2}}\right )^{2} - } \right . \\ & \left . \left . \frac{2 \mathrm{i}}{\tau _{\mathrm{r}2}^{3}} + \frac{9 \mathrm{i} c_{\mathrm{s}}^{2} k_{x}^{2}}{\tau _{\mathrm{r}2}} \right )^{1/3} + \frac{1}{3 \tau_{\mathrm{r}2} ^{2}} \right ] \end{aligned}$$

(86)