Correlations in $n$-local scenario

Abstract

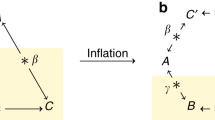
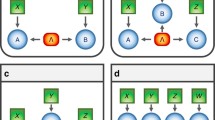
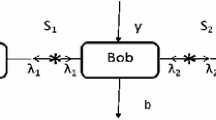
Recently Bell-type inequalities were introduced in Branciard et al. (Phys Rev A 85:032119, 2012) to analyze the correlations emerging in an entanglement swapping scenario characterized by independence of the two sources shared between three parties. The corresponding scenario was referred to as bilocal scenario. Here, we derive Bell-type inequalities in $n+1$ party scenario, i.e., in $n$-local scenario. Considering the two different cases with several number of inputs and outputs, we derive local and $n$-local bounds. The $n$-local inequality studied for two cases are proved to be tight. Replacing the sources by maximally entangled states for two binary inputs and two binary outputs and also for the fixed input and four outputs, we observe quantum violations of $n$-local bounds. But the resistance offered to noise cannot be increased as compared to the bilocal scenario. Thus increasing the number of parties in a linear fashion in source-independent scenario does not contribute in lowering down the requirements of revealing quantumness in a network in contrast to the star configuration (Tavakoli et al. in Phys Rev A 90:062109, 2014) of $n+1$ parties.

Appendices

Appendix 1: representation of the $4$-local correlation in terms of $q_{\bar{\alpha }_{1}\bar{ \alpha }_{2} \bar{\alpha }_{3}\bar{\alpha }_{4}}$

We note here that $\bar{\alpha }_{1}$ is specified by $\lambda _1$; $\bar{ \alpha }_{2}$ is specified by $\lambda _1, \lambda _2$; $\bar{\alpha }_{3}$ is specified by $\lambda _2, \lambda _3$; and $\bar{\alpha }_{4}$ is specified by $\lambda _3$. Then $\bigcup _{\bar{ \alpha }_{2}}\Lambda ^{123}_{\bar{\alpha }_{1}\bar{ \alpha }_{2} \bar{\alpha }_{3}\bar{\alpha }_{4}} = \Lambda ^{123}_{\bar{\alpha }_{1}\bar{\alpha }_{3}\bar{\alpha }_{4}} = \Lambda ^{1}_{\bar{\alpha }_{1}} \times {\Lambda ^{23}_{\bar{\alpha }_{3}\bar{\alpha }_{4}}}$, $\bigcup _{\bar{\alpha }_{3}}\Lambda ^{123}_{\bar{\alpha }_{1}\bar{ \alpha }_{2} \bar{\alpha }_{3}\bar{\alpha }_{4}} = \Lambda ^{123}_{\bar{\alpha }_{1}\bar{\alpha }_{2}\bar{\alpha }_{4}} = \Lambda ^{12}_{\bar{\alpha }_{1}\bar{\alpha }_{2}} \times {\Lambda ^{3}_{\bar{\alpha }_{4}}}$ and

$$\begin{aligned} q_{\bar{\alpha }_{1}\bar{\alpha }_{3}\bar{\alpha }_{4}}= & {} \sum _{\bar{\alpha }_{2}}q_{\bar{\alpha }_{1}\bar{ \alpha }_{2} \bar{\alpha }_{3}\bar{\alpha }_{4}}= \int \!\!\int \!\!\int _{\Lambda ^{1}_{\bar{\alpha }_{1}} \times {\Lambda ^{23}_{\bar{\alpha }_{3}\bar{\alpha }_{4}}}} d\lambda _1d\lambda _2d\lambda _3\rho (\lambda _1, \lambda _2, \lambda _3). \end{aligned}$$

(35)

$$\begin{aligned} q_{\bar{\alpha }_{1}\bar{\alpha }_{2}\bar{\alpha }_{4}}= & {} \sum _{\bar{\alpha }_{3}}q_{\bar{\alpha }_{1}\bar{ \alpha }_{2} \bar{\alpha }_{3}\bar{\alpha }_{4}}= \int \!\!\int \!\!\int _{\Lambda ^{12}_{\bar{\alpha }_{1}\bar{\alpha }_{2}} \times {\Lambda ^{3}_{\bar{\alpha }_{4}}}} d\lambda _1d\lambda _2d\lambda _3\rho (\lambda _1, \lambda _2, \lambda _3). \end{aligned}$$

(36)

$$\begin{aligned} q_{\bar{\alpha }_{1}\bar{\alpha }_{4}}= & {} \sum _{\bar{\alpha }_{3}}q_{\bar{\alpha }_{1}\bar{\alpha }_{3}\bar{\alpha }_{4}} = \int \!\!\int \!\!\int _{\Lambda ^{1}_{\bar{\alpha }_{1}} \times {\Lambda ^{2} \times \Lambda ^{3}_{\bar{\alpha }_{4}}}} d\lambda _1d\lambda _2d\lambda _3\rho (\lambda _1, \lambda _2, \lambda _3). \end{aligned}$$

(37)

$$\begin{aligned} q_{\bar{\alpha }_{1}\bar{\alpha }_{2}}= & {} \sum _{\bar{\alpha }_{4}}q_{\bar{\alpha }_{1}\bar{\alpha }_{2}\bar{\alpha }_{4}} = \int \!\!\int \!\!\int _{\Lambda ^{12}_{\bar{\alpha }_{1}\bar{\alpha }_{2}} \times {\Lambda ^{3}}} d\lambda _1d\lambda _2d\lambda _3\rho (\lambda _1, \lambda _2, \lambda _3). \end{aligned}$$

(38)

$$\begin{aligned} q_{\bar{\alpha }_{3}\bar{\alpha }_{4}}= & {} \sum _{\bar{\alpha }_{1}}q_{\bar{\alpha }_{1} \bar{\alpha }_{3}\bar{\alpha }_{4}} = \int \!\!\int \!\!\int _{\Lambda ^{1} \times {\Lambda ^{23}_{\bar{\alpha }_{3}\bar{\alpha }_{4}}}} d\lambda _1d\lambda _2d\lambda _3\rho (\lambda _1, \lambda _2, \lambda _3). \end{aligned}$$

(39)

$$\begin{aligned} q_{\bar{\alpha }_{1}}= & {} \sum _{\bar{\alpha }_{4}}q_{\bar{\alpha }_{1}\bar{\alpha }_{4}} = \int \!\!\int \!\!\int _{\Lambda ^{1}_{\bar{\alpha }_{1}} \times \Lambda ^{2} \times \Lambda ^{3}} d\lambda _1d\lambda _2d\lambda _3\rho (\lambda _1, \lambda _2, \lambda _3). \end{aligned}$$

(40)

$$\begin{aligned} q_{\bar{\alpha }_{4}}= & {} \sum _{\bar{\alpha }_{1}}q_{\bar{\alpha }_{1}\bar{\alpha }_{4}} = \int \!\!\int \!\!\int _{\Lambda ^{1} \times \Lambda ^{2} \times \Lambda ^{3}_{\bar{\alpha }_{4}}} d\lambda _1d\lambda _2d\lambda _3\rho (\lambda _1, \lambda _2, \lambda _3). \end{aligned}$$

(41)

where $\Lambda ^1 = \bigcup _{\bar{\alpha }_{1}}\Lambda ^{1}_{\bar{\alpha }_{1}}$ , $\Lambda ^2 =\bigcup _{\bar{\alpha }_{2}\bar{\alpha }_{3}}\Lambda ^{2}_{\bar{\alpha }_{2}\bar{\alpha }_{3}}$ and $\Lambda ^3 = \bigcup _{\bar{\alpha }_{4}}\Lambda ^{3}_{\bar{\alpha }_{4}}$ are the corresponding state spaces of the variables $\lambda _1, \lambda _2$ and $\lambda _3$, respectively.

Now if we now consider $P$ as $4$-local, then the independence condition (7) implies [Eq. (42) is obtained from (35), (39), (40) and Eq. (43) is obtained from (36), (38), (41)] for all $\bar{\alpha }_{1}, \bar{\alpha }_{3}$ and $\bar{\alpha }_{4}$,

$$\begin{aligned} q_{\bar{\alpha }_{1}\bar{\alpha }_{3}\bar{\alpha }_{4}}= & {} q_{\bar{\alpha }_{1}}q_{\bar{\alpha }_{3}\bar{\alpha }_{4}}\quad \forall \bar{\alpha }_{1}, \bar{\alpha }_{3}, \bar{\alpha }_{4}. \end{aligned}$$

(42)

$$\begin{aligned} q_{\bar{\alpha }_{1}\bar{\alpha }_{2}\bar{\alpha }_{4}}= & {} q_{\bar{\alpha }_{1}\bar{\alpha }_{2}} q_{\bar{\alpha }_{4}}\quad \forall \bar{\alpha }_{1}, \bar{\alpha }_{2}, \bar{\alpha }_{4}. \end{aligned}$$

(43)

The above result can be easily extended to $n$-local scenario.

Appendix 2: Proof of Eq. (16)

We define,

$$\begin{aligned} \langle A_{1,x_1}\rangle _{\lambda _{1}}= & {} \sum _{a_1}(-1)^{a_1}P^{22}(a_1|x_1,\lambda _{1}) \end{aligned}$$

(44)

$$\begin{aligned} \langle A_{i,x_i}\rangle _{\lambda _{i}}= & {} \sum _{a_i}(-1)^{a_i}P^{22}(a_i|x_i,\lambda _{i},\lambda _{i+1}),\quad i=2,\ldots ,n \end{aligned}$$

(45)

$$\begin{aligned} \langle A_{n+1,x_{n+1}}\rangle _{\lambda _{n}}= & {} \sum _{a_{n+1}}(-1)^{a_{n+1}}P^{22}(a_{n+1}|x_{n+1},\lambda _{n}). \end{aligned}$$

(46)

Since by assumption $P^{22}$ is $n$-local, it has a $n$-local decomposition of the form (6) and (7). So we get,

$$\begin{aligned} I^{22}_{A_2,\ldots A_n}= & {} \frac{1}{4}\int \int \ldots \int d\lambda _{1},\ldots d\lambda _{n} \Pi _{i=1}^n\rho _{i}(\lambda _{i})(\langle A_{1,0}+A_{1,1}\rangle _{\lambda _{1}})\nonumber \\&\times \,(\langle A_{n+1,0}+A_{n+1,1}\rangle _{\lambda _{n}}) (\langle A_{2,0}\ldots A_{n-1,0}\rangle )_{\lambda _{1}\ldots \lambda _{n}}. \end{aligned}$$

Now,

$$\begin{aligned} \mid \langle A_{2,0}\ldots A_{n-1,0}\rangle _{\lambda _{1}\ldots \lambda _{n}} \mid \le 1. \end{aligned}$$

(47)

Using the above relation, we have,

$$\begin{aligned} \mid I^{22}_{A_2,\ldots A_n}\mid\le & {} \frac{1}{4}\int \int d\lambda _{1} d\lambda _{n}\rho _{1}(\lambda _{1})\rho _{n}(\lambda _{n}) (\langle A_{1,0}+A_{1,1}\rangle _{\lambda _{1}})\nonumber \\&\times \,(\langle A_{n+1,0}+A_{n+1,1}\rangle _{\lambda _{n}})\int \ldots \int \Pi _{i=2}^{n-1}\rho _{i}(\lambda _{i})\\= & {} \int d\lambda _{1}\rho _{1}(\lambda _{1}) \frac{\mid (\langle A_{1,0}+A_{1,1}\rangle )_{\lambda _{1}}\mid }{2}\nonumber \\&\times \,\int d\lambda _{n}\rho _n(\lambda _{n}) \frac{\mid (\langle A_{n+1,0}+A_{n+1,1}\rangle )_{\lambda _{n}}\mid }{2}. \end{aligned}$$

Similarly for $J^{22}_{A_2,\ldots A_n}$ it can be shown that,

$$\begin{aligned} \mid J^{22}_{A_2,\ldots A_n}\mid\le & {} \int d\lambda _{1}\rho _{1}(\lambda _{1}) \frac{\mid (\langle A_{1,0}-A_{1,1}\rangle )_{\lambda _{1}}\mid }{2}\nonumber \\&\times \int d\lambda _{n}\rho _n(\lambda _{n}) \frac{\mid (\langle A_{n+1,0}-A_{n+1,1}\rangle )_{\lambda _{n}}\mid }{2}. \end{aligned}$$

Now, by using Holder’s inequality for $4$ positive quantities $\mid (\langle A_{1,0}+A_{1,1}\rangle )_{\lambda _{1}}\mid$, $\mid (\langle A_{1,0}-A_{1,1}\rangle )_{\lambda _{1}}\mid$, $\mid (\langle A_{n+1,0}+A_{n+1,1}\rangle )_{\lambda _{n}}\mid$, $\mid (\langle A_{n+1,0}-A_{n+1,1}\rangle )_{\lambda _{n}}\mid$ we get,

$$\begin{aligned}&\sqrt{\mid I^{22}_{A_2,\ldots A_n}\mid } + \sqrt{\mid J^{22}_{A_2,\ldots A_n}\mid }\\&\quad \le \sqrt{\int d\lambda _{1}\rho _{1}(\lambda _{1})\left( \frac{\mid (\langle A_{1,0}+A_{1,1}\rangle )_{\lambda _{1}}\mid }{2} +\frac{\mid (\langle A_{1,0}-A_{1,1}\rangle )_{\lambda _{1}}\mid }{2}\right) }\\&\quad \le \sqrt{\int d\lambda _{n}\rho _{n}(\lambda _{n})\left( \frac{\mid (\langle A_{n+1,0}+A_{n+1,1}\rangle )_{\lambda _{n}}\mid }{2}+\frac{\mid (\langle A_{n+1,0}-A_{n+1,1}\rangle )_{\lambda _{n}}\mid }{2}\right) }. \end{aligned}$$

Again, $\frac{\mid (\langle A_{1,0}+A_{1,1}\rangle )_{\lambda _{1}}\mid }{2}+\frac{\mid (\langle A_{1,0}-A_{1,1}\rangle )_{\lambda _{1}}\mid }{2}$ = $\max (\mid \langle A_{1,0}\rangle _{\lambda _{1}}\mid , \mid \langle A_{1,1}\rangle _{\lambda _{1}}\mid )\le 1$ and similarly $\max (\mid \langle A_{n+1,0}\rangle _{\lambda _{1}}\mid , \mid \langle A_{n+1,1}\rangle _{\lambda _{1}}\mid )\le 1$. Using these we get,

$$\begin{aligned} \sqrt{\mid I^{22}_{A_2,\ldots A_n}\mid }+ \sqrt{\mid J^{22}_{A_2,\ldots A_n}\mid } \le \int d\lambda _{1}\rho _1(\lambda _{1}). \int d\lambda _{n}\rho _n(\lambda _{n}) = 1. \end{aligned}$$

(48)

Hence the inequality (16) is satisfied. $\square$