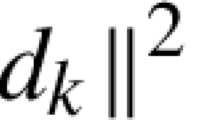Skip to main content
SpringerLink
Log in

A class of split-step balanced methods for stiff stochastic differential equations

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

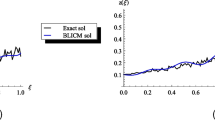
In this paper we design a class of general split-step balanced methods for solving Itô stochastic differential systems with m-dimensional multiplicative noise, in which the drift or deterministic increment function can be taken from any chosen one-step ODE solver. We then give an analysis of their order of strong convergence in a general setting, but for the mean-square stability analysis, we confine our investigation to a special case in which the drift increment function of the methods is replaced by the one from the well known Rosenbrock method. The resulting class of stochastic differential equation (SDE) solvers will have more appropriate and useful mean-square stability properties for SDEs with stiffness in their drift and diffusion parts, compared to some other already reported split-step balanced methods. Finally, numerical results show the effectiveness of these methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Alcock, J., Burrage, K.: A note on the Balanced Method. BIT 46(4), 689–710 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  2. Burrage, K., Burrage, P.M., Tian, T.: Numerical methods for strong solutions of stochastic differential equations: an overview. Roy. Soc. London Proc. Ser. A 460(2041), 373–402 (2004)

    MathSciNet  Google Scholar 

  3. Burrage, K., Tian, T.: The composite Euler method for stiff stochastic differential equations. J. Comput. Appl. Math. 131, 407–426 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  4. Dekker, K., Verwer, J.: Stability of Runge-Kutta Methods for Stiff Nonlinear Differential equations. Elsiver-North Holand, Amesterdam (1984)

    MATH  Google Scholar 

  5. Devroye, L., Karasözen, B., Kohler, M., Korn, R. (eds.): Recent Developments in Applied Probability and Statistics: Dedicated to the Memory of Jürgen Lehn. Berlin, Heidelberg, Springer (2010)

    MATH  Google Scholar 

  6. Fisher, P., Platen, E.: Application of balanced method to stochastic differential equations in filtering. Monte Carlo Methods Appl. 5, 19–38 (1999)

    Article  MathSciNet  Google Scholar 

  7. Haghighi, A., Hosseini, S.M.: On the stability of some second order numerical methods for weak approximation of Itô SDEs. Numer. Algorithms 57, 101–124 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  8. Hairer, E., Wanner, G.: Solving Ordinary Differential Equations. II, Stiff and Differential-Algebraic Problems. Springer-Verlag, Berlin (1996)

    MATH  Google Scholar 

  9. Higham, D.J.: Mean-square and asymptotic stability of the stochastic theta method. SIAM J. Numer. Anal. 38(3), 753–769 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  10. Higham, D.J.: An algorithmic introduction to numerical simulation of stochastic differential equations. SIAM Rev. Edu. Sect. 43, 525–546 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  11. Kahl, C., Schurz, H.: Balanced Milstein methods for ordinary SDEs. Monte Carlo Methods Appl. 12(2), 143–170 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  12. Kloeden, P.E., Platen, E.: A survey of numerical methods for stochastic differential equations. Stoch. Hydrol. Hydraul. 3, 155–178 (1989)

    Article  MATH  Google Scholar 

  13. Kloeden, P.E., Platen, E., Wright, W.: The approximation of multiple stochastic integrals. Stoch. Anal. Appl. 10, 431–441 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  14. Kloeden, P.E., Platen, E.: Numerical Solution of Stochastic Differential Equations. Applications of Mathematics, vol. 23. Springer-Verlag, Berlin (1999)

    Google Scholar 

  15. Milstein, G.N.: A theorem on the order of convergence of mean square approximations of solutions of systems of stochastic differential equations. Theory Probab. Appl. 32, 738–741 (1988)

    Article  Google Scholar 

  16. Milstein, G.N.: Numerical Integration of Stochastic Differential Equations. Kluwer, Dordrecht (1995)

    Google Scholar 

  17. Milstein, G.N., Platen, E., Schurz, H.: Balanced implicit methods for stiff stochastic systems. SIAM J. Numer. Anal. 35, 1010–1019 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  18. Petersen, W.P.: A general implicit splitting for stabilizing numerical simulations of Itô stochastic differential equations. SIAM J. Numer. Anal. 35, 1439–1451 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  19. Stoer, J., Bulirsch, R.: Introduction to Numerical Analysis, Second Edition. Springer-Verlag, Berlin (1991)

    Google Scholar 

  20. Tian, T.H., Burrage, K.: Implicit Taylor methods for stiff stochastic differential equations. Appl. Numer. Math. 38, 167–185 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  21. Wang, P., Liu, Z.: Split-step backward balanced Milstein methods for stiff stochastic systems. Appl. Numer. Math. 59, 1198–1213 (2009)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, Faculty of Mathematical Sciences, Tarbiat Modares University, P.O. Box 14115-175, Tehran, Iran

    Amir Haghighi & S. Mohammad Hosseini

Authors
  1. Amir Haghighi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. Mohammad Hosseini
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Amir Haghighi.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Haghighi, A., Hosseini, S.M. A class of split-step balanced methods for stiff stochastic differential equations. Numer Algor 61, 141–162 (2012). https://doi.org/10.1007/s11075-012-9534-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-012-9534-5

Keywords

Search

Navigation