Skip to main content
SpringerLink
Log in

The embracing Voronoi diagram and closest embracing number

  • Published:
Journal of Mathematical Sciences Aims and scope Submit manuscript

Abstract

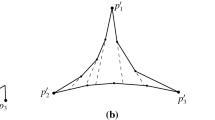
A point q is embraced by a set of points S if q is interior to the convex hull of S [8]. In some illumination applications where points of S are lights and q is a point to be illuminated, the embracing concept is related to a good illumination [4, 6], also known as the ∆-guarding [12] and the well-covering [10]. In this paper, we are not only interested in convex dependency (which is actually the embracing notion) but also in proximity. Suppose that the sites of S are lights or antennas with limited range; due to their limited power, they cover a disk of a given radius r centered at the sites of S. Only the points lying in such disks are illuminated. If we want to embrace the point q with the minimum range r, we need to know which is the closest light s q to q such that q lies in the convex hull formed by s q and the lights of S closer to q than s q . This subset of S related to point q is called the closest embracing set for q in relation to S and its cardinality is the closest embracing number of q. By assigning every point q in the convex hull of S to its closest embracing site s q , we obtain a partition of the convex hull that we call the embracing Voronoi diagram of S. This paper proves some properties of the embracing Voronoi diagrams and describes algorithms to compute such diagrams, as well as the levels in which the convex hull is decomposed regarding the closest embracing number.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. M. Abellanas, A. Bajuelos, G. Hernández, and I. Matos, “Good illumination with limited visibility,” In: Proc. of the International Conference of Numerical Analysis and Applied Mathematics, Wiley-VCH Verlag (2005), pp. 35–38.

  2. M. Abellanas, A. Bajuelos, G. Hernández, I. Matos, and B. Palop, “Minimum illumination range voronoi diagrams,” In: Proc. of the 2nd International Symposium on Voronoi Diagrams in Science and Engineering (2005), pp. 231–238.

  3. M. Abellanas, A. Bajuelos, and I. Matos, “Some problems related to good illumination,” In: O. Gervasi and M. Gavrilova (eds): Proc. of ICCSA 2007, LNCS 4705, Springer-Verlag (2007), pp. 1–14.

  4. M. Abellanas, S. Canales, and G. Hernández, Buena Iluminación, Actas de las IV Jornadas de Matematica Discreta y Algorítmica, Madrid 2004, pp. 236–246.

    Google Scholar 

  5. T. Asano, S. K. Ghosh, and T. C. Shermer, “Visibility in the plane,” In: J.-R. Sack and J. Urrutia (eds.): Handbook of Computational Geometry, Elsevier, Amsterdam (2000), pp. 829–876.

    Chapter  Google Scholar 

  6. S. Canales, “Métodos Heurísticos en problemas geométricos,” Visibilidad, Iluminación y Vigilancia, PhD Thesis, Universidad Politécnica de Madrid, (2004).

  7. M. Y. Chan, D. Chen, F. Y. L. Chin, and C. A. Wang, “Construction of the nearest neighbor embracing graph of a point set,” J. Comb. Optim., 11, No. 4, 435–443 (2006).

    Article  MATH  MathSciNet  Google Scholar 

  8. S. N. Chiu and I. S. Molchanov, “A new graph related to the directions of nearest neighbors in a point process,” Adv. Appl. Probab., 35, No. 1, 47–55 (2003).

    Article  MATH  MathSciNet  Google Scholar 

  9. H. Edelsbrunner, “Algorithms in combinatorial geometry,” Edited by W. Brauer, G. Rozenberg, and A. Salomaa, Springer-Verlag, New York Inc. (1987).

  10. A. Efrat, S. Har-Peled, and J. S. B. Mitchell, “Approximation algorithms for two optimal location problems in sensor networks,” In: Proc. of International Conference on Broadband Networks, Vol. 1 (2005), pp. 714–723.

    Google Scholar 

  11. S. K. Ghosh, Visibility Algorithms in the Plane, Cambridge University Press, Cambridge (2007).

    MATH  Google Scholar 

  12. J. Smith and W. Evans, “Triangle guarding,” In: Proc. of the 15th Canadian Conference on Computational Geometry (2003), pp. 76–80.

  13. J. Urrutia, “Art gallery and illumination problems,” In: Handbook of Computational Geometry, Edited by in J.-R. Sack and J. Urrutia, Elsevier (2000), pp. 973–1027.

Download references

Author information

Authors and Affiliations

  1. Departamento de Matemática Aplicada, Facultad de Informática, U.P.M., Madrid, Spain

    M. Abellanas & G. Hernández

  2. Departamento de Matemática & CEOC, Universidade de Aveiro, Aveiro, Portugal

    A. L. Bajuelos & I. Matos

  3. Escuela Universitaria Politécnica, Universidad de Valladolid, Valladolid, Spain

    B. Palop

Authors
  1. M. Abellanas
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. G. Hernández
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. A. L. Bajuelos
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. I. Matos
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. B. Palop
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to M. Abellanas.

Additional information

Translated from Sovremennaya Matematika i Ee Prilozheniya (Contemporary Mathematics and Its Applications), Vol. 63, Optimal Control, 2009.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Abellanas, M., Hernández, G., Bajuelos, A.L. et al. The embracing Voronoi diagram and closest embracing number. J Math Sci 161, 909–918 (2009). https://doi.org/10.1007/s10958-009-9610-0

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10958-009-9610-0

Keywords

Search

Navigation