BGK and Fokker-Planck Models of the Boltzmann Equation for Gases with Discrete Levels of Vibrational Energy

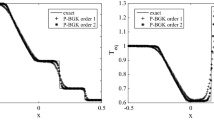
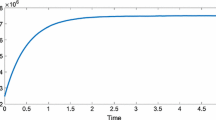
Abstract

We propose two models of the Boltzmann equation (BGK and Fokker-Planck models) for rarefied flows of diatomic gases in vibrational non-equilibrium. These models take into account the discrete repartition of vibration energy modes, which is required for high temperature flows, like for atmospheric re-entry problems. We prove that these models satisfy conservation and entropy properties (H-theorem), and we derive their corresponding compressible Navier–Stokes asymptotics.

Gaussian Integrals and Other Summation Formulas

In this section, we give some integrals and summation formula that are used in the paper.

First, we remind the definition of the absolute Maxwellian $M_0(V) = \frac{1}{(2\pi )^{\frac{3}{2}}}\exp (-\frac{|V|^2}{2})$. We denote by $\langle \phi \rangle = \int _{{\mathbb {R}}^3}\phi (V)\, dV$ for any function $\phi$. It is standard to derive the following integral relations (see [24], for instance), written with the Einstein notation:

$$\begin{aligned}&\langle M_0 \rangle _V = 1, \\&\langle V_iV_jM_0 \rangle _V = \delta _{ij}, \qquad \langle V_i^2M_0 \rangle _V = 1, \qquad \langle |V|^2M_0 \rangle _V = 3, \\&\langle V_iV_jV_kV_lM_0 \rangle _V = \delta _{ij}\delta _{kl} + \delta _{ik}\delta _{jl} + \delta _{il}\delta _{jk} , \qquad \langle V_i^2V_j^2M_0 \rangle _V = 1 + 2\, \delta _{ij} \\&\langle V_iV_j|V|^2M_0 \rangle _V = 5 \,\delta _{ij}, \qquad \langle |V|^4M_0 \rangle _V = 15, \\&\langle V_iV_j|V|^4M_0 \rangle _V = 35 \,\delta _{ij}, \qquad \langle |V|^6M_0 \rangle = 105, \end{aligned}$$

while all the integrals of odd power of V are zero. Note that the first relation of each line implies the other relations of the same line: these relations are given here to improve the readability of the paper. From the previous Gaussian integrals, it can be shown that for any $3\times 3$ matrix C, we have

$$\begin{aligned} \langle V_iV_jC_{kl}V_kV_lM_0 \rangle _V = C_{ij} + C_{ji} + C_{ii}\delta _{ij}. \end{aligned}$$

Finally, we have also used the following relations:

$$\begin{aligned} \int _{0}^{+\infty } J e^{-J} \, dJ = \int _{0}^{+\infty } e^{-J} \, dJ = 1, \end{aligned}$$

and also

$$\begin{aligned} \sum _{i=0}^{+\infty } e^{-iT_0/T} = \frac{1}{1-e^{-T_0/T}} \quad \text { and } \quad \sum _{i=0}^{+\infty } i e^{-iT_0/T} = \frac{e^{-T_0/T}}{(1-e^{-T_0/T})^2}. \end{aligned}$$