Abstract
I study a model of growth and income distribution in which workers and firms bargain à la Nash (Econometrica 18(2):155–162, 1950) over wages and productivity gains, taking into account the trade-offs faced by firms in choosing factor-augmenting technologies. The aggregate environment resulting from self-interested, objective function-maximizing decision rules on wages, productivity gains, savings and investment, is described by a two-dimensional dynamical system in the employment rate and output/capital ratio. The economy converges cyclically to a long-run equilibrium involving a Harrod-neutral profile of technical change, a constant rate of employment of labor, and constant input shares. The type of oscillations predicted by the model is qualitatively consistent with the available data on the United States (1963–2003), replicates the dynamics found in earlier models of growth cycles such as Goodwin (A growth cycle, in C.H. Feinstein (ed). Socialism, Capitalism and Economic Growth. Cambridge University Press, Cambridge 1967. Cambridge University Press, Cambridge, 1967); Shah and Desai (Econ J 91:1006–1010, 1981); van der Ploeg (J Macroecon 9:1–12, 1987); Flaschel (J Econ: Zeitschrift für Nationalökonomie 44:63–69, 1984) and Sportelli (J Econ: Zeitschrift für Nationalökonomie 61(1):35–64, 1995), and can be verified numerically in simulations. Institutional change, as captured by variations in workers’ bargaining power, has a positive effect on the long-run rate of growth of output per worker but a negative effect on long-run employment. Economic policy can also affect the growth and distribution pattern through changes in the unemployment compensation, which also have a positive long-run impact on labor productivity growth but a negative long-run impact on employment. In both cases, employment can overshoot its new equilibrium value along the transitional dynamics.









Similar content being viewed by others
Notes
Although the standard Neoclassical growth model can also account for the Kaldor facts, the fundamental difference is in that induced innovation links distributive shares with technical progress, while in the Neoclassical growth model income distribution is determined by capital/labor substitution—that is, by the shape of the aggregate production function.
The sensitivity of the original Goodwin model to small modifications in its basic assumptions, a problem known as ‘structural instability’, has captured the attention of many scholars working in this tradition, and the debate was particularly vibrant in this Journal (Velupillai 1979; Flaschel 1984). van der Ploeg (1987) and Sportelli (1995) provide conditions to overcome the structurally unstable outcome of the Goodwin model with stable limit cycles which, differently from induced technical change (or factor substitution) retain the perpetuity of the distributional conflict at the heart of the Goodwin growth cycle.
I also show in Sect. 4.4 that the main conclusions reached survive the introduction of instantaneous capital/labor substitution through a Neoclassical production function. A deeper reason to focus on Leontief production functions builds on a view about production which starts from the consideration that at each moment in time production takes place with fixed input/output coefficients. Over time, however, profit-maximizing decisions about the direction of technical change are responsible for capital deepening, and will make the aggregate production function look Neoclassical, with diminishing returns to capital per worker in standard fashion. The role of capital deepening over time resulting from biased technical change as opposed to instantaneous factor substitution has been emphasized by Michl (1999) as well as Foley and Michl (1999, p. 127).
A microfoundation for the IPF resulting from a general CES production technology is provided in Funk (2002).
Using Nash bargaining as a wage-setting mechanism is pretty standard in the labor market literature, whether or not workers are assumed to be unionized. Examples include Pissarides (2001) in the matching literature, and Oswald (1985), Blanchflower and Oswald (1990) regarding labor unions. The advantage of Nash bargaining is that it focuses on the outcome of the bargaining problem. Also, the bargaining mechanism can be justified strategically as in Binmore et al. (1986). Finally, bargaining between workers and firms in this paper occurs on wages and productivity only. If workers were unionized, this would amount to a simple right-to-manage model: workers will accept the equilibrium employment rate that is compatible with the bargaining wage evaluated at a market equilibrium appearing in (12). The results of the paper would change if, in efficient bargaining fashion, the level of employment entered the maximization program below.
A traditional proxy for \(\eta \) is the rate of unionization, but one can also think about different aspects of labor legislation that increase the relative weight of workers in wage negotiations. Further, recent literature has suggested to look at the percentage of rents produced by firms and captured by workers. See Appendix E.
Since the choice variables are \(w, \beta \), it makes no difference if we consider profits, \({ rK}\), or the profit rate, to appear in the firms’ gain.
These claims are substantiated in Appendix A.2.
We will relax this assumption in Sect. 4.1.
Problems of this kind are not new in contemporary treatments of the labor market (see Pissarides 2001, Chapter 1.7). Considering only a ‘rational expectations’ solution is clearly a limitation of the present analysis, which however makes room for future research.
For those who remain skeptic, the solution above can be alternatively found using \(\exp \left\{ -\int r(s)ds \right\} \) as an integrating factor for the transition equation on capital stock, and exploiting the transversality condition. In fact, the solution of the Euler equation for consumption will be \(C(t)=C(0)\exp \left\{ -\int (r(s)-\rho )ds\right\} \). Using \(\exp \left\{ -\int r(s)ds \right\} \) as integrating factor, we can solve the transition equation for capital up to a constant of integration. Because of the transversality condition, such constant turns out to be zero. Therefore, it is easy to determine \(C(0)=\rho K(0)\), from which the solution for \(K(t)\) follows immediately. A similar derivation, which produces the same result as here once the consumption good is assumed to exchange at the same price as the capital good, can be found in Acemoglu (2009, p. 667). In that context, too, what drives the result is the iso-elastic form of the utility function.
It is worthwhile to stress once again the assumptions about saving decisions we made in this paper. It is assumed that saving and investment choices take place given the outcome of negotiation about wages and productivity gains. The reason why this assumption is made is not only its intuitive plausibility, but the fact that the Nash bargaining structure (3), although allowing bargainers to choose control variables taking advantage on information on both production and innovation environments, already gives the workplace a strongly cooperative character, because it involves the joint maximization of utility gains. The inclusion of investment decisions into a grand, single optimization problem would configure the firm as a cooperative, and describing such an environment is outside the focus of this paper.
The role of the direction of technical progress in distributional conflict is discussed, among others, in Bowles and Kendrick (1970).
This is a key result in this paper, established formally in Proposition 8.
The software used for the simulations is Mathematica 7, and the code is available from the author upon request.
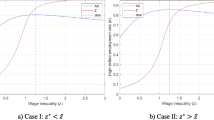
Note that, because of the fixed-coefficients assumption on output technology, effective inputs are complements in production, and therefore any increase in the labor-augmenting parameter \(A\) shifts the demand for labor inwards. Even if we assumed a smooth production function, however, the result of Proposition 8 would not substantially change with an elasticity of substitution smaller than one, which is also supported by most of the empirical evidence on economic growth, as summarized in Acemoglu (2003). An increase in labor-augmenting technologies would determine an increase in the demand for labor only with an elasticity of substitution greater than one.
In this paper, I do not address in depth the problem of financing unemployment benefits. As Pissarides (2001, Chapter 9) shows, modeling the funding for labor market policies through taxation and introducing the government sector produces dramatic analytical complications in frameworks with equilibrium unemployment. To circumvent problems like these, Shapiro and Stiglitz (1984) originally assumed that it is firms offering unemployment packages as well as wages to workers. Adopting such a strategy here would amount to reduce the area of the bargaining set, and it would be equivalent to imposing a shut-down cost for firms. With a lump-sum tax rate on capital outlays \(\tau \), and a balanced government budget, bargaining would determine workers’ wage as \(w =\rho V_U+\eta (A-\rho V_U-\tau )\). The tax rate would obviously appear in the determination of the profit rate, but the narrative would not change. We can then keep \(b\) as a simple shifting parameter, with the understanding that the profit rate in this section should be seen as net of taxes, and leave a deeper analysis of financing considerations for future research.
The proof of this proposition goes along the lines of Appendix B.2, and it is left as an exercise.
For those trained in PK economics, there is no independent investment function in the Goodwin (1967) model. Hence, savings and investment are always equal along any point of the Goodwin trajectories, leaving no room for short-run adjustments in capacity utilization in order to correct disequilibrium situations in the goods market.
Such rate is set equal to 1 in the Goodwin model, but nothing would change if a certain fraction, say 80 % was chosen. It would be sufficient to rescale the output/capital ratio \(B\) by multiplying it by such desired rate of utilization.
I thank an anonymous referee for suggesting to work out both specifications of workers’ savings. For those familiar with history of thought, the analysis in this section hints at the Pasinetti vs anti-Pasinetti debate of the 1960s, although in the simplified case without government. A list of references to that debate is provided in Faria and Araujo (2004).
It is easy to verify that, when the elasticity of substitution equals zero, that is in the Leontief case, imposing \(x=1\) also implies \(f(1)=1\), so that \(r=B[1-w/A]\).
Thus, the equation describing the firm-level direction of technical change in this section reads \(-g_\beta =\frac{1-\frac{xf^{\prime }(x)}{f(x)}}{\frac{xf^{\prime }(x)}{f(x)}}=\frac{\mathrm{Profit \, Share}}{\mathrm{Labor\, Share}}\). Further, it is somewhat tedious, but definitely not hard to show that, once the marginal product of labor is equated to the bargaining wage, Eq. (14) holds.
As a consistency check, observe that in the Leontief case \(x=1=f(x)\), so that \(B_{ss}\) reduces to the sum \(\alpha (\cdot )+n+\rho \) as in Sect. 4.3.
A concise exposition of these findings appears in Bowles (2004, Chapter 5).
Goodwin (1967) and followers would phrase this sentence by saying that the role of the equilibrium unemployment is to put the distributional conflict between capital and labor to rest. In this interpretation, equilibrium unemployment closely resembles a notion of ‘reserve army’ of labor, that shrinks or expands in response to the interaction between accumulation and technical change. See also Bowles (1985).
References
Abowd J, Lemieux T (1993) The effect of product market competition on collective bargaining agreements: the case of foreign competition in Canada. Q J Econ 108:983–1004
Acemoglu D (2003) Labor and capital augmenting technical change. J Eur Econ Assoc 1:1–37
Acemoglu D (2009) Introduction to modern economic growth. Princeton University Press, Princeton
Arrow K (1970) The technology factor in international trade. National Bureau of Economic Research, New York
Barbosa-Filho NH, Taylor Lance (2007) Distributive and demand cycles in the US economy—a structuralist Goodwin model. Metroeconomica 57:389–411
Binmore K, Rubinstein A, Wolinsky A (1986) The Nash bargaining solution in economic modelling. Rand J Econ 17:176–188
Blanchflower D, Oswald A (1990) The wage curve. Scand J Econ 92(2):215–235
Blanchflower D, Oswald A, Sanfrey P (1996) Wages, profits and rent sharing. Q J Econ 111 1:227–251
Bowles S (1985) The production process in a competitive economy: Walrasian, Neo-Hobbesian, and Marxian models. Am Econ Rev 75(1):16–36
Bowles S (2004) Microeconomics. Behavior, institutions, and evolution. Princeton University Press, Princeton
Bowles S, Kendrick D (1970) Notes and problems in microeconomic theory. Markham Publishing Company, Chicago
Bureau of Labor Statistics (2009a) Unemployment Rate—Civilian Labor Force—LNS14000000. http://www.bls.gov/data
Bureau of Labor Statistics (2009b) Economics new release—Union Members Summary. http://www.bls.gov/news.release/union2.nr0.htm
Davis SJ, Haltiwanger JC, Schuh S (1997) Job creation and job destruction. MIT Press, Cambridge
Drandakis EM, Phelps E (1965) A model of induced invention, growth and distribution. Econ J 76(304):823–840
Dutt AK (1997) Equilibrium, path dependence and hysteresis in post-Keynesian models. In: Arestis P, Palma G, Sawyer M (eds) Capital controversy, post-Keynesian economics and the history of economic thought: essays in honour of Geoff Harcourt. Routledge, London
Extended Penn World Tables (2008). v. 3.0. http://homepage.newschool.edu/foleyd/
Faria JR, Araujo RA (2004) An intertemporal pasinettian model with government sector. Int J Business Econ 3(3):257–268
Flaschel P (1984) Some stability properties of Goodwin’s growth cycle: a critical elaboration. J Econ—Zeitschrift für Nationalökonomie 44:63–69
Flaschel P, Groh G (1995) The classical growth cycle: reformulation, simulation and some facts. Econ Notes 24:293–326
Foley DK (2003) Endogenous technical change with externalities in a classical growth model. J Econ Behav Organ 52:167–189
Foley DK, Michl TR (1999) Growth and distribution. Harvard University Press, Cambridge
Michl TR, Foley DK (2004) Social security in a classical growth model. Camb J Econ 28(1):1–20
Funk P (2002) Induced innovation revisited. Economica 68:155–171
Goodwin R (1967) A growth cycle. In: Feinstein C (ed) Socialism, capitalism, and economic growth. Cambridge University Press, Cambridge
Harvie D (2000) Testing Goodwin: growth cycles in ten OECD countries. Camb J Econ 24:349–376
Julius AJ (2005) Steady-state growth and distribution with an endogenous direction of technical change. Metroeconomica 56:1
Julius AJ (2009) The Wage-wage-..-wage-profit relation in a multisector bargaining economy. Metroeconomica 60(3):537–559
Kaldor N (1961) Capital accumulation and economic growth. In: Lutz FA, Hague DC (eds) The theory of capital. St. Martins Press, New York, p 222
Kamien M, Schwartz N (1969) Induced factor augmenting technological change from a microeconomic viewpoint. Econometrica 37(4):668–684
Kauermann G, Teuber T, Flaschel P (2008) Estimating loops and cycles using penalized splines. CEM working paper, Bielefeld
Kennedy C (1964) Induced bias in innovation and the theory of distribution. Econ J 74:541–47
Lavoie M (1995) The Kaleckian model of growth and distribution and its neo-Ricardian and neo-Marxian critiques. Camb J Econ 19(6):789–818
Lavoie M (1996) Traverse, hysteresis and normal growth rates of capacity utilization in Kaleckian models of growth and distribution. Rev Radic Polit Econ 28(4):113–147
Lavoie M, Rodríguez G, Seccareccia M (2004) Similitudes and discrepancies in post-Keynesian and Marxist theories of investment: a theoretical and empirical investigation. Int Rev Appl Econ 18(2):127–149
Marglin S (1984) Growth, distribution and prices. Harvard University Press, Cambridge
Michl TR (1999) Biased technical change and the aggregate production function. Int Rev Appl Econ 13
Mohun S, Veneziani R (2006) Structural stability and Goodwins growth cycle. Struct Chang Econ Dyn 17:437–451
Nash J (1950) The bargaining problem. Econometrica 18(2):155–162
Nordhaus WD (1967) Essays on the theory of optimal economic growth. MIT Press, Cambridge
Oswald AJ (1985) The economic theory of trade unions: an introductory survey. Scand J Econ 87(2):160–193
Piketty T, Saez E (2003) Income inequality in the United States, 1913–1998. Q J Econ 143:1–39
Pissarides C (2001) Equilibrium unemployment theory. MIT Press, Cambridge
Romer P (1986) Increasing returns and long run growth. J Polit Econ 98, part II, 1002–1037
Rubinstein A (1982) Perfect equilibrium in a bargaining model. Econometrica 50(1):97–109
Shah A, Desai M (1981) Growth cycles with induced technical change. Econ J 91:1006–1010
Shapiro C, Stiglitz J (1984) Equilibrium unemployment as a worker discipline device. Am Econ Rev 74(3):433–444
Skott P (2010a) Growth, instability and cycles: Harrodian and Kaleckian models of accumulation and income distribution. In: Setterfield M (ed) Handbook of alternative theories of economic growth. Edward Elgar
Skott P (2010) Theoretical and empirical shortcomings of the Kaleckian investment function. Metroeconomica. doi:10.1111/j.1467-999X.2010.04111.x
Spence M (1977) Entry, capacity, investment and oligopolistic pricing. Bell J Econ The RAND Corporation 8(2):534–544
Sportelli MC (1995) A Kolmogoroff generalized predator–prey model of Goodwin’s growth cycle. J Econ—Zeitschrift für Nationalökonomie 61(1):35–64
Taylor L (2004) Reconstructing macroeconomics. Harvard University Press, Cambridge
van der Ploeg F (1987) Growth cycles, induced technical change, and perpetual conflict over the distribution of income. J Macroecon 9:1–12
van Reenen J (1996) The creation and capture of economic rents: wages and innovation in a panel of UK companies. Q J Econ 111(1):195–226
Velupillai K (1979) Some stability properties of Goodwin’s growth cycle. J Econ—Zeitschrift für Nationalökonomie 39:245–257
Acknowledgments
I thank Rudi von Arnim, Guido Cozzi, Peter Flaschel, Michalis Nikiforos, Christian Proaño, Codrina Rada, Rick van der Ploeg, Peter Skott, Luca Zamparelli, participants to the 2010 Eastern Economic Association Meetings, and two anonymous referees for extremely valuable comments and suggestions. I am most indebted to Duncan Foley for his guidance and constructive criticism.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix A: Solution of the bargaining problem
1.1 A.1 Proof of Proposition 1
Form the current-value Hamiltonian:
and let harmlessly \(\gamma _1 \equiv p_1 e^{-\beta ^* t}, \gamma _2 \equiv p_1 e^{-g(\beta ^*)t}\), \(\beta ^*\) being the solution value for \(\beta \) to be determined. Necessary conditions for maximization are:
Solving (23) for \(w\) gives (4). Also, a necessary condition for optimality of the solution is the existence of continuous function \(p_1(t), p_2(t)\) satisfying:
where the LHS of the two equations are equal to the familiar \(\rho \gamma _i-\dot{\gamma }_i, i=1,2\), and \(\omega \equiv w/A\) is the share of wages in firm’s output. Also, the following transversality conditions must hold at an optimal control path:
Because of concavity of the objective function and convexity of the constraint set, conditions (23)–(26) are also sufficient for a maximum of problem (3).
A stationary solution for (25) and (26) where \(\dot{p}_1=\dot{p}_2=0\) satisfies:
Divide the first equation by the second and use (24) to get (5).
1.2 A.2 Behavior of rates of factor-augmentations at the firm level
In order to get started in studying the behavior of factor-augmentations, rewrite Eq. (5) as:
Such an equation yields an implicit function \(\beta (\omega , \rho )\) whose partial derivatives fulfill standard properties. We have that:
Clearly,
Therefore,
so that \(\beta _\omega <0\). Our assumption about the IPF imply \(\alpha _\omega >0\).
Appendix B: Proofs of various propositions
1.1 B.1 Proof of Proposition 2
We have:
Furthermore,
Finally,
1.2 B.2 Proof of Proposition 3
Using the same approach as in Appendix A.2, we can construct a function \(\Gamma (\beta , v; \rho , \lambda , \eta )=0\) such that:
Since
we have that:
and our assumptions about the IPF ensure that \(\alpha _v>0\). Also,
Hence,
and \(\alpha _\eta >0\). Further,
so that
Therefore, \(\alpha _\lambda <0\). Finally,
so that
so that \(\alpha _\rho <0\).
1.3 B.3 Proof of Proposition 4
Existence and uniqueness of a long-run equilibrium are easy to check after a glance at Fig. 3. A look at Eqs. (17) and (18) reveals that the long-run equilibrium is of the purely Harrod-neutral type, with zero capital-productivity growth and a growth rate of labor-augmenting technologies equal to \(\alpha _{ss}=g(0; \cdot )\). The equilibrium employment rate is constant and equal to \(\beta ^{-1}(0; \cdot )\). Finally, once the long-run employment rate of the model is achieved, the wage share will be constant, and given by \(\eta \frac{\rho +\lambda +q(v_{ss})}{\rho +\lambda +\eta q(v_{ss})}\).
1.4 B.4 Proof of Proposition 5
Linearize the above system around its non-trivial rest point to obtain the Jacobian matrix:
This matrix has a negative trace, as \(\beta _v<0,-q_v<0, -\alpha _v< 0\), and a positive determinant. Therefore, its two eigenvalues have real parts that are of the same sign and sum up to a negative number, and this proves the claim.
1.5 B.5 Proof of Proposition 7
We have that:
Further,
Also,
Finally,
1.6 B.6 Proof of Proposition 8
Since \(\beta (v_{ss}; \eta , \lambda , \rho )=0\), we must have that \(\beta _{v_{ss}}dv_{ss}+\beta _\eta d\eta =0\), from which:
As both \(\beta _\eta , \beta _{v_{ss}}<0\), the claim is proved.
1.7 B.7 Proof of Proposition 9
We have:
1.8 B.8 Proof of Proposition 11
Since \(\beta _{v_{ss}}dv_{ss}+\beta _b db=0\),
As both \(\beta _b, \beta _{v_{ss}}<0\), the claim is proved.
Appendix C: Bargaining in the model with substitution
The statement of the bargaining problem with substitution is similar to the non-substitution case, and therefore omitted. The current-value Hamiltonian \(H_S\) associated with such problem is:
where \(\gamma _1, \gamma _2\) are defined as in Appendix A. Setting \(\partial H_S/\partial w=0\) yields:
and setting \(\partial H_S/\partial \beta =0\) gives (24). Proceeding as in the non-substitution case, a stationary solution in which the adjoint variables \(\gamma _1, \gamma _2\) don’t change over time satisfies:
Dividing both sides of (29) by (30) and using (24), one obtains \(-g_\beta =\frac{f(x)-xf^{\prime }(x)}{xf^{\prime }(x)}\).
Once a bargaining deal is struck, profit-maximizing firms will demand labor up to the point where its marginal product \(Af^{\prime }(x)\) is equal to the wage. The wage share in firm’s output is then \(wL/Y=xf^{\prime }(x)/f(x)\), so that the firm-level optimal direction of technical change satisfies (5). Once the outside option \(V_U\) is determined through (8), we can define a market equilibrium as in Sect. 2.5, at which real wage and profit rate are:
Appendix D: Stability analysis in the model with substitution
The Jacobian matrix evaluated at the steady state is:
which has a negative trace and positive determinant if and only if \(\sigma <1/\epsilon \), thus yielding the same results of the non-substitution case if this restriction on the elasticity of substitution is satisfied.
Appendix E: Numerical simulations
For this exercise, we assume the following parameter calibration: \(n=.02\), \(\alpha (v_{ss}, \cdot )=.02\), which match first moments in population growth and labor productivity growth in the US. As far as rates of job creation and job destruction are concerned, Davis et al. (1997) suggest to calibrate \(\lambda =.113, q(v_{ss})=.092\). The plot in Fig. 2 points an average employment rate around 94 %, which we take as our benchmark value for \(v_{ss}\). If we assume a linear specification for \(q(\cdot )\), then we can internally calibrate the first derivative \(q_v\). Finally, we need to set a value for the parameter describgin workers’ bargaining power. On the one hand, we could use the national US average for the unionization rate. The current value is 12.1 %, although this figure has been steadily decreasing in recent decades from 33 % in the 1960s (BLS 2009b). On the other hand, we could follow several authors (Abowd and Lemieux 1993; Blanchflower et al. 1996; van Reenen 1996) who estimated the percentage of rents produced by firms and captured by wages. These estimates provide values for \(\eta \) ranging from 0.08 to 0.3. In line with these findings, I run simulations corresponding to several values of \(\eta \), respectively \(\eta =\{0.1, 0.2, 0.3\}\). The following table summarizes the parameter values used for the simulation runs displayed in Figs. 4, 5 and 6.
Rights and permissions
About this article
Cite this article
Tavani, D. Bargaining over productivity and wages when technical change is induced: implications for growth, distribution, and employment. J Econ 109, 207–244 (2013). https://doi.org/10.1007/s00712-012-0287-3
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00712-012-0287-3