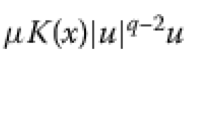Abstract
In this paper we will study the equation
with \(N=3,\) where \( S_2(D^2u)(x)=\sum _{1\le i<j\le {N}}\lambda _i(x)\lambda _j(x)\), being \(\lambda _i,\) the solutions to the equation
\(i=1,\dots ,N,\) and \(\Omega \) is a bounded domain with smooth boundary. We deal with several boundary conditions looking for the appropriate framework to get existence and multiplicity of nontrivial solutions. This kind of equation is related to some models of growth, and for this reason it is natural to study the effect of zero order local reaction terms of the type \(F_{\lambda }(x,u)=\lambda |u|^{p-1}u\), with \(\lambda \in \mathbb {R}\), \(\lambda >0\), and \(0<p<\infty \), and also the solvability of the boundary problems with a source term \(f\) satisfying some integrability hypotheses.
Similar content being viewed by others
References
Agmon, S., Douglis, A., Nirenberg, L.: Estimates near the boundary for solutions of elliptic partial differential equations satisfying general boundary conditions. I. Comm. Pure Appl. Math. 12, 623–727 (1959)
Agmon, S., Douglis, A., Nirenberg, L.: Estimates near the boundary for solutions of elliptic partial differential equations satisfying general boundary conditions. II. Comm. Pure Appl. Math. 17, 35–92 (1964)
Ambrosetti, A., Malchiodi, A.: Nonlinear analysis and semilinear elliptic problems. In: Cambridge Studies in Advanced Mathematics, vol. 104, pp 60–65 (2006)
Ambrosetti, A., Rabinowitz, P.H.: Dual variational methods in critical point theory and applications. J. Funct Anal. 14, 349–381 (1973)
Calderón, A.P., Zygmund, A.: On singular integrals. Am. J. Math. 78, 289–309 (1956)
Coifman, R., Lions, P.L., Meyer, Y., Semmes, S.: Compensated compactness and Hardy spaces. J. Math. Pures Appl. 72, 247–286 (1993)
Coffman, C.V., Duffin, R.J., Shaffer, D.H.: Constructive Approaches to Mathematical Models, pp. 267–277. Academic Press, New York (1979)
Ekeland, I.: On the variational principle. J. Math. Anal. Appl. 47, 324–353 (1974)
Ehrenpreis, L.: Solution of some problems of division. I. Division by a polynomial of derivation. Am. J. Math. 76(4), 883–903 (1954)
Ehrenpreis, L.: Solution of some problems of division. II. Division by a punctual distribution. Am. J. Math. 77(2), 286–292 (1955)
Escudero, C.: Geometric principles of surface growth. Phys. Rev. Lett. 101, 196102 (2008)
Escudero, C., Peral, I.: Some fourth order nonlinear elliptic problems related to epitaxial growth. J. Differ. Equ. 254, 2515–2531 (2013)
Ferrari, F.: Ground state solutions for \(k-\)th Hessian operators. Boll. Un. Mat. Ital. B (7) 9, 553–586 (1995)
Ferrari, F., Franchi, B., Verbitsky, I.E.: Hessian inequalities and the fractional Laplacian. J. Reine Angew. Math. 667, 133–148 (2012)
Ferrari, F., Verbitsky, I.E.: Radial fractional Laplace operators and Hessian inequalities. J. Differ. Equ. 253(1), 244–272 (2012)
García Azorero, J., Peral, I.: Multiplicity of solutions for elliptics problems with critical exponents or with a non-symmetric term. Trans. Am. Math. Soc. 323(2), 877–895 (1991)
Gazzola, F., Grunau, H., Sweers, G.: Polyharmonic boundary value problems. Positivity preserving and nonlinear higher order elliptic equations in bounded domains. In: Lecture Notes in Mathematics, 1991. Springer, Berlin (2010)
Malgrange, B.: Existence et approximation des solutions des quations aux drives partielles et des quations de convolution. Ann. Inst. Fourier 6, 271–355 (1955–56)
Marsili, M., Maritan, A., Toigo, F., Banavar, J.R.: Stochastic growth equations and reparametrization invariance. Rev. Mod. Phys. 68, 963–983 (1996)
Ortega, J.H., Zuazua, E.: Generic simplicity of the spectrum and stabilization for a plate equation. Siam J. Control Optim. 39(5), 1585–1614 (2001)
Rabinowitz, P.H.: Some global results for nonlinear eigenvalue problems. J. Funct. Anal. 7, 487–513 (1971)
Stein, E.M.: Singular integrals and differentiability properties of functions. In: Princeton Mathematical Series, No. 30. Princeton University Press, Princeton, N.J. (1970)
Stein, E.M.: Harmonic Analysis: Real-Variable Methods, Orthogonality and Oscillatory Integrals. Princeton University Press, Princeton (1993)
Stein, E.M., Weiss, G.: On the theory of harmonic functions of several variables. I. The theory of \(H^{p}\) -spaces. Acta Math. 103, 25–62 (1960)
Tartar, L.: Nonlinear Analysis and Mechanics. Heriott-Watt Symposium, IV. Pitman, London, 1979
Tartar, L.: Systems of Nonlinear Partial Differential Equations. Reidel, Dordrecht (1983)
Tso, K.: Remarks on critical exponents for hessian operators. Annales Inst. H. Poincaré Anal. Non Linéaire 7, 113–122 (1990)
Acknowledgments
F. Ferrari and I. Peral wish to thank the Department of Mathematics of the Universidad Autónoma de Madrid and to the department of Mathematics of the Universitá di Bologna, respectively, for its kind hospitality.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by P. Rabinowitz.
F. Ferrari was partially supported by the GNAMPA project: “Equazioni non lineari su varietà: proprietà qualitative e classificazione delle soluzioni” and FP7-IDEAS-ERC Starting Grant 2011 #277749 (EPSILON). M. Medina and I. Peral are supported by project MTM2010-18218 of MICINN, Spain.
Rights and permissions
About this article
Cite this article
Ferrari, F., Medina, M. & Peral, I. Biharmonic elliptic problems involving the 2nd Hessian operator. Calc. Var. 51, 867–886 (2014). https://doi.org/10.1007/s00526-013-0698-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00526-013-0698-1