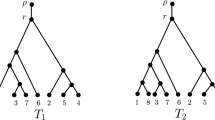
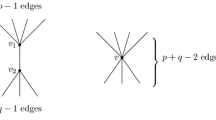Abstract
Studying the shape of phylogenetic trees under different random models is an important issue in evolutionary biology. In this paper, we propose a general framework for deriving detailed statistical results for patterns in phylogenetic trees under the Yule–Harding model and the uniform model, two of the most fundamental random models considered in phylogenetics. Our framework will unify several recent studies which were mainly concerned with the mean value and the variance. Moreover, refined statistical results such as central limit theorems, Berry–Esseen bounds, local limit theorems, etc., are obtainable with our approach as well. A key contribution of the current study is that our results are applicable to the whole range of possible sizes of the pattern.
Similar content being viewed by others
References
Aldous DJ (1991) The continuum random tree II: an overview. In: Barlow NT, Bingham NH (eds) Stochastic analysis. Cambridge University Press, Cambridge, pp 23–70
Baron G, Drmota M, Mutafchiev L (1996) Predecessors in random mappings. Comb Prob Comput 5: 317–335
Blum M, François O (2005) External branch length and minimal clade size under the neutral coalescent. Adv Appl Prob 37: 647–662
Blum M, Bortolussi N, Durand E, François O (2006a) APTreeshape: statistical analysis of phylogenetic tree shape. Bioinformatics 22: 363–364
Blum M, François O, Janson S (2006b) The mean, variance and limiting distributions of two statistics sensitive to phylogenetic tree balance. Ann Appl Prob 16: 2195–2214
Chern H-H, Fuchs M, Hwang H-K (2007) Phase changes in random point quadtrees. ACM Trans Alg 3:51
Darwin C (1859) The origin of species, reprinted by Penguin Books. London
Drmota M, Hwang H-K (2005) Profile of random trees: correlation and width of random recursive trees and binary search trees. Adv Appl Prob 37: 321–341
Drmota M, Janson S, Neininger R (2008a) A functional limit theorem for the profile of search trees. Ann Appl Prob 18: 288–333
Drmota M, Gittenberger B, Panholzer A, Prodinger H, Ward MD (2008b) On the shape of the fringe of various types of random trees. Math Math Appl Sci (in press)
Feng Q, Mahmoud H, Panholzer A (2008) Phase changes in subtree varieties in random recursive trees and binary search trees. SIAM J Discrete Math 22: 160–184
Fill JA, Kapur N (2004) Limiting distributions for additive functionals on Catalan trees. Theor Comp Sci 326: 69–102
Flajolet P, Sedgewick R (2009) Analytic combinatorics. Cambridge University Press, Cambridge
Flajolet P, Gourdon X, Martinez C (1997) Patterns in random binary search trees. Random Struct Alg 11: 223–224
Flajolet P, Sedgewick R (1995) An introduction to the analysis of algorithms. Addison-Wesley Professional, Reading
Fuchs M (2008) Subtree sizes in recursive trees and binary search trees: Berry–Esseen bound and Poisson approximation. Comb Prob Comput 17: 661–680
Fuchs M, Hwang H-K, Neininger R (2007) Profiles of random trees: limit theorems for random recursive trees and binary search trees. Algorithmica 46: 367–407
Harding EF (1971) The probabilities of rooted tree-shapes generated by random bifurcation. Adv Appl Prob 3: 44–77
Hwang H-K (2003) Second phase changes in random m-ary search trees and generalized quicksort: convergence rates. Ann Prob 31: 609–629
Hwang H-K (2007) Profiles of random trees: plane-oriented recursive trees. Random Struct Alg 30: 380–413
Hwang H-K, Neininger R (2002) Phase change of limit laws in the quicksort recurrences under varying toll functions. SIAM J Comput 31: 1687–1722
Hwang H-K, Nicodème P, Park G, Szpankowski W (2008) Profiles of tries. SIAM J Comput 38: 1821–1880
Knuth DE (1997) The art of computer programming, vol 1, 3rd edn. In: Fundamental algorithms. Addison-Wesley, Reading
Knuth DE (1998) The art of computer programming, vol 2, 3rd edn. In: Seminumerical algorithms. Addison-Wesley, Reading
Knuth DE (1998) The art of computer programming, vol. 3, 2nd edn. In: Sorting and searching. Addison-Wesley, Reading
Loève M (1977) Probability theory. I, 4th edn. Springer, New York
McKenzie A, Steel M (2000) Distribution of cherries for two models of trees. Math Biosci 164: 81–92
McKenzie A, Steel M (2001) Properties of phylogenetic trees generated by Yule-type specification models. Math Biosci 170: 91–112
Mooers AO, Heard SB (1997) Inferring evolutionary process from phylogenetic tree shape. Q Rev Biol 72: 31–54
Mooers AO, Heard SB (2002) Using tree shape. Syst Biol 51: 833–834
Pemantle R (2000) Generating functions with high-order poles are nearly polynomial. In: Mathematics and computer science (Versailles, 2000). Birkhäuser, Baesl, pp 305–321
Pemantle R, Wilson MC (2002) Asymptotics of multivariate sequences I: smooth points of the singular variety. J Comb Theory Ser A 97: 129–161
Pemantle R, Wilson MC (2004) Asymptotics of multivariate sequences, Part II: multiple points of the singular variety. Comb Prob Comp 13: 735–761
Pemantle R, Wilson MC (2008) Twenty combinatorial examples of asymptotics derived from multivariate generating functions. SIAM Rev 20: 199–272
Petrov VV (1975) Sums of indepedent random variables, Ergebnisse der Mathematik und ihrer Grenzgebiete, Band 82. Springer, New York
Rosenberg NA (2006) The mean and variance of the numbers of r-pronged nodes and r-caterpillars in Yule-generated genealogical trees. Ann Comb 10: 129–146
Semple C, Steel M (2003) Phylogenetics, Oxford University Press, Oxford
Stanley RP (1997) Enumerative combinatorics, vol 1. Cambridge University Press, Cambridge
Stanley RP (1999) Enumerative combinatorics, vol 2. Cambridge University Press, Cambridge
Szpankowski W (2001) Average-case analysis of algorithms on sequences. Wiley, New York
Yule GU (1924) A mathematical theory of evolution, based on the conclusions of Dr. J. C. Willies. Philos Trans R Soc London B 213: 21–87
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Chang, H., Fuchs, M. Limit theorems for patterns in phylogenetic trees. J. Math. Biol. 60, 481–512 (2010). https://doi.org/10.1007/s00285-009-0275-6
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00285-009-0275-6