Skip to main content

Advertisement

SpringerLink
Log in

Dynamics of one-dimensional spiking neuron models

  • Published:
Journal of Mathematical Biology Aims and scope Submit manuscript

Abstract.

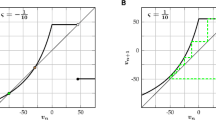
In this paper we make a rigorous mathematical analysis of one-dimensional spiking neuron models in a unified framework. We find that, under conditions satisfied in particular by the periodically and aperiodically driven leaky integrator as well as some of its variants, the spike map is increasing on its range, which leaves no room for chaotic behavior. A rigorous expression of the Lyapunov exponent is derived. Finally, we analyse the periodically driven perfect integrator and show that the restriction of the phase map to its range is always conjugated to a rotation, and we provide an explicit expression of the invariant measure.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Alstrom, P., Christiansen, B., Levinsen, M.T.: Nonchaotic transition from quasiperiodicity to complete phase locking. Phys. Rev. Lett. 61, 1679–1682 (1988)

    Article  MathSciNet  Google Scholar 

  2. Artun, O., Shouval, H., Cooper, L.: The effect of dynamic synapses on spatiotemporal receptive fields in visual cortex. PNAS 95, 11999–12003 (1998)

    Article  Google Scholar 

  3. Ascoli, C., Barbi, M., Chillemi, S., Petracchi, D.: Phase-locked responses in the Limulus lateral eye. Theoretical and experimental investigation. Biophys. J. 19, 219–40 (1977)

    Google Scholar 

  4. Brette, R.: Rotation numbers of discontinuous orientation-preserving circle maps. Set-Valued Anal. 2003, in press

  5. Brette, R., Guigon, E.: Reliability of spike timing is a general property of spiking model neurons. Neural Comput. 15, 279–308 (2003)

    Article  Google Scholar 

  6. Coombes, S.: Liapunov exponents and mode-locked solutions for integrate-and-fire dynamical systems. Phys. Lett. A, (1999)

  7. Cornfeld, I., Fomin, S., Sinai, Y.: Ergodic theory, no. 245 in Grundlehren der mathematischen Wissenschaften, Springer-Verlag, 1981

  8. de~Melo, W., van Strien, S.: One-dimensional dynamics. Berlin: Springer-Verlag, 1993

  9. Denjoy, A.: Sur les courbes définies par les équations différentielles à la surface du tore. J. Math. Pures Appl. 9, 333–375 (1932)

    Google Scholar 

  10. Ermentrout, B.: Type i membranes, phase resetting curves, and synchrony. Neural Comput. 8, 979–1001 (1996)

    Google Scholar 

  11. Ermentrout, B., Kopell, N.: Parabolic bursting in an excitable system coupled with a slow oscillation. SIAM J. Appl. Math. 46, 233–253 (1986)

    MathSciNet  MATH  Google Scholar 

  12. Glass, L., Bélair, J.: Continuation of Arnold tongues in mathematical models of periodically forced biological oscillators. In: Nonlinear oscillations in Biology and Chemistry, H. Othmer, (ed.), no. 66 in Lecture Notes in Biomathematics. Berlin: Springer-Verlag, 1986, pp. 232–243

  13. Glass, L., Perez, R.: Fine structure of phase locking. Phys. Rev. Lett. 48, 1772–1775 (1982)

    Article  MathSciNet  Google Scholar 

  14. Guttman, R., Feldman, L., Jakobsson, E.: Frequency entrainment of squid axon membrane. J. Membrane Biol. 56, 9–18 (1980)

    Google Scholar 

  15. Herman, M.: Mesure de Lebesgue et nombre de rotation. Lecture Notes in Math, Vol. 597, Berlin: Springer, 1977, pp. 271–293

  16. Hodgkin, A., Huxley, A.: A quantitative description of membrane current and its application to conduction and excitation in nerve. J. Physiol. (Lond.) 117, 500–544 (1952)

    Google Scholar 

  17. Jensen, M., Bak, P., Bohr, T.: Complete devil’s staircase, fractal dimension and universality of mode-locking structure in the circle map. Phys. Rev. Lett. 50, 1637–1639 (1983)

    Article  Google Scholar 

  18. Keener, J., Hoppensteadt, F., Rinzel, J.: Integrate-and-fire models of nerve membrane response to oscillatory input. SIAM J. Appl. Math. 41, 503–517 (1981)

    MathSciNet  MATH  Google Scholar 

  19. Keener, J.P.: Chaotic behavior in piecewise continuous difference equations. Trans. Amer. Math. Soc. 261, 589–604 (1980)

    MathSciNet  MATH  Google Scholar 

  20. Knight, B.W.: Dynamics of encoding in a population of neurons. J. Gen. Physiol. 59, 734–66 (1972)

    Google Scholar 

  21. Koppl, C.: Phase locking to high frequencies in the auditory nerve and cochlear nucleus magnocellularis of the barn owl. Tyto alba. J. Neurosci. 17, 3312–21 (1997)

    Google Scholar 

  22. Kwapisz, J.: Poincare rotation number for maps of the real line with almost periodic displacement. Nonlinearity 13, 1841–1854 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  23. Lapicque, L.: Recherches quantitatives sur l’excitation électrique des nerfs traitée comme une polarisation. J. Physiol. Pathol. Gen. 9, 620–635 (1907)

    Google Scholar 

  24. Latham, P., Richmond, B., Nelson, P., Nirenberg, S.: Intrinsic dynamics in neuronal networks. i. theory. J. Neurophysiol. 83, 808–27 (2000)

    Google Scholar 

  25. Poincaré, H.: Sur les courbes définies par les équations différentielles. J. Math. Pures. App. I, 167, (1885)

  26. Rescigno, A., Stein, R., Purple, R., Poppele, R.: A neuronal model for the discharge patterns produced by cyclic inputs. Bull Math. Biophys. 32, 337–353 (1970)

    MATH  Google Scholar 

  27. Rhodes, F., Thompson, C.: Rotation numbers for monotone functions on the circle. J. London Math. Soc. 2, 360–368 (1986)

    MathSciNet  Google Scholar 

  28. Rhodes, F., Thompson, C.: Topologies and rotation numbers for families of monotone functions on the circle. J. London Math. Soc. 2, 156–170 (1991)

    MathSciNet  Google Scholar 

  29. Somers, D., Nelson, S., Mriganka, S.: An emergent model of orientation selectivity in cat visual cortical simple cells. J. Neurosci. 15, 5448–5465 (1995)

    Google Scholar 

  30. Song, S., Abbot, L.: Cortical development and remapping through spike timing-dependent plasticity. Neuron. 32, 339–350 (2001)

    Google Scholar 

  31. Song, S., Miller, K., Abbott, L.: Competitive Hebbian learning through spike-timing-dependent synaptic plasticity. Nature neurosci. 3, 919–926 (2000)

    Article  Google Scholar 

  32. Swiatek, G.: Rational rotation numbers for maps of the circle. Commun. Math. Phys. 119, 109–128 (1988)

    MathSciNet  MATH  Google Scholar 

  33. Tass, P., Rosenblum, M., Weule, J., Kurths, J., Pikovsky, A., Volkmann, J., Schnitzler, A., Freund, H.: Detection of n:m phase locking from noisy data: application to magnetoencephalography. Phys. Rev. Lett. 81, 3291–3294 (1998)

    Article  Google Scholar 

  34. Tiesinga, P.: Precision and reliability of periodically and quasiperiodically driven integrate-and-fire neurons. Phys. Rev. E, 65, (2002)

  35. Troyer, T., Miller, K.: Physiological gain leads to high ISI variability in a simple model of a cortical regular spiking cell. Neural Comput. 9, 971–83 (1997)

    Google Scholar 

  36. Veerman, J.J.P.: Irrational rotation numbers. Nonlinearity 2, 419–428 (1989)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Centre de Mathématiques et de Leurs Applications, Ecole Normale Supérieure de Cachan, 61, avenue du Président Wilson, 94230, Cachan, France

    Romain Brette

  2. INSERM U483, Université Pierre et Marie Curie, 9, quai Saint-Bernard, 75005, PARIS, France

    Romain Brette

Authors
  1. Romain Brette
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Romain Brette.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Brette, R. Dynamics of one-dimensional spiking neuron models. J. Math. Biol. 48, 38–56 (2004). https://doi.org/10.1007/s00285-003-0223-9

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00285-003-0223-9

Keywords

Search

Navigation