Evaluation of the quantum time-correlation functions employing the Hamilton–Jacobi dynamics framework

Abstract

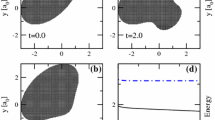
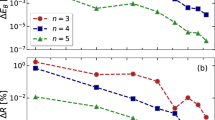
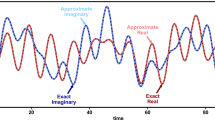
The quantum Hamilton–Jacobi equation (QHJE) is formally equivalent to the time-dependent Schrödinger equation, and the solutions to the QHJE can be easily interpreted in terms of trajectories providing a link between classical and quantum mechanics. The trajectory-based approaches to quantum molecular dynamics are, generally, appealing because they circumvent exponential scaling associated with exact quantum methods with the system size, and because, unlike classical molecular dynamics, such methods incorporate dominant quantum effects due to delocalization of wavefunctions describing the nuclei. We explore the utility of the QHJE framework for calculations of the time-correlation functions (TCFs) involving quantum evolution defined by the Boltzmann density operator and by the Hamiltonian time-evolution operator. The implementation is based on solutions to the imaginary-time counterpart to the QHJE, which yield approximations to the ground state wavefunction. The resulting nodeless wavefunction is used to generate a basis in coordinate space, which is efficient for evaluation of the low-lying excited states and of the quantum TCFs, including the Kubo-transformed TCFs, at low temperature. The QHJE/basis approach is illustrated on several model systems in and out of thermal equilibrium, i.e., the ${\rm H}_2$ dimer and bound anharmonic potentials. If a system exhibits large amplitude motion, e.g., in case of the nonequilibrium dynamics, then the real-time trajectory propagation provides an alternative to the basis representation, as demonstrated on a model describing the inversion mode of the ammonia molecule and ion.

Appendix: Variational approximation to the quantum potential

An analytically form of the approximate quantum potential ${\widetilde{U}}$ is essential for efficiency and accuracy of the quantum force computation. One way to achieve such ${\tilde{u}}$ is to represent the nonclassical component, r(x, t), of the QM momentum operator, defined by Eq. (7), as a superposition of basis functions in coordinate space. Approximation to this single object within a basis $\vec {f}(x)$,

$$\begin{aligned} r(x,t)\approx {\tilde{r}}(x,t)=\vec {c}(t)\cdot \vec {f}(x), \end{aligned}$$

(A1)

gives analytically approximate quantum potential,

$$\begin{aligned} {\widetilde{U}}=-\frac{1}{2m}({\tilde{r}}^2+\nabla {\tilde{r}}), \end{aligned}$$

(A2)

and analytically quantum force $F^{q}=-\nabla {\widetilde{U}}$. A detailed derivation for multidimensional systems is given in Ref. [28].

Vector $\vec {c}$ contains the time-dependent basis expansion coefficients, which are found by minimizing the average deviation between r(x, t) and ${\tilde{r}}(x,t)$

$$\begin{aligned} I=\langle (r-{\tilde{r}})^2\rangle _t= I_0+ \int \left( \nabla {\tilde{r}}(x,t)+{\tilde{r}}^2(x,t)\right) \rho (x,t)\mathrm{d}x. \end{aligned}$$

(A3)

Equation (A3) is obtained from integration by parts assuming $\rho =0$ at the limits of integration; $I_0$ denotes the term which does not depend on $\vec {c}$. By changing the integration variable to $x_t$, using Eq. (16), and replacing the integration with summation over discrete trajectories, one obtains

$$\begin{aligned} I=I_0+\int \left( \nabla {\tilde{r}}(x_t)+ {\tilde{r}}^2(x_t)\right) \rho (x_t)\mathrm{d}x_t=I_0+\sum _i \left( \nabla {\tilde{r}}(x_t^{(i)})+{\tilde{r}}^2(x_t^{(i)})\right) w^{(i)}. \end{aligned}$$

(A4)

The fact that neither $\rho (x,t)$ nor its derivatives appear in Eq. (A4) is of central importance for efficient implementation. The algorithm scales linearly with respect to the number of trajectories, since a single summation over the trajectories gives all the necessary matrix and vector elements.

Minimization of I with respect to the expansion coefficients yields a system of linear equations,

$$\begin{aligned} \nabla _c I=2 {\varvec{S}} \vec {c}+\vec {b}=0. \end{aligned}$$

(A5)

Here ${\varvec{S}}$ is the time-dependent matrix of the basis function overlaps; $\vec {b}$ is the vector of averages of the spatial derivatives of the basis functions,

$$\begin{aligned} S_{nm}=\langle f_n|f_m\rangle =\sum _i w^{(i)} f_nf_m\left| _{x=x_t^{(i)}}\right. , \quad b_n= \langle \frac{\mathrm{d} f_n}{\mathrm{d}x}\rangle =\sum _i w^{(i)} \frac{\mathrm{d} f_n}{\mathrm{d}x}\left| _{x=x_t^{(i)}}\right. \end{aligned}$$

The optimal coefficients are the elements of the vector,

$$\begin{aligned} \vec {c}=-\frac{1}{2}{\varvec{S}}^{-1}\vec {b}. \end{aligned}$$

(A6)

As shown in Ref. [26], ${\widetilde{U}}$ evaluated at the optimal values of $\vec {c}$, $\nabla _c I=0$, conserves the total energy of a wavefunction for time-independent classical potentials,

$$\begin{aligned} \frac{\mathrm{d}E}{\mathrm{d}t}=\langle \frac{p}{m}\frac{\mathrm{d}p}{\mathrm{d}t}+\nabla (V+{\widetilde{U}})\cdot \frac{\mathrm{d}x}{\mathrm{d}t}+\frac{\partial {\widetilde{U}}}{\partial t}\rangle =\langle \nabla _c {\widetilde{U}}\rangle \cdot \frac{\mathrm{d}\vec {c}}{\mathrm{d}t}=0. \end{aligned}$$

(A7)

The energy is conserved because ${\widetilde{U}}$ is proportional to $I-I_0$. Thus, minimization of I is equivalent to the variational determination of ${\widetilde{U}}$.

The smallest physically meaningful basis $\vec {f}(x)$ consists of just two functions $\vec {f}=(1,x)$, and the approximation can be written in a particularly transparent form,

$$\begin{aligned} {\tilde{r}}=-\frac{x-\langle x\rangle }{2(\langle x^2\rangle -\langle x\rangle ^2)}. \end{aligned}$$

(A8)

The wavefunction is normalized to 1, and the nonclassical momentum is approximated with a linear function centered at the average position of the wavepacket and inversely proportional to its variance, $\sigma =\langle x^2\rangle -\langle x\rangle ^2$. This functional form of ${\tilde{r}}$ generates a quadratic ${\widetilde{U}}$ and, consequently, a linear quantum force. Hence, the approximation is termed the linearized quantum force (LQF). Note that $F^q$ is inversely proportional to $\sigma ^2$; therefore, $F^{q}$ quickly vanishes for delocalized wavepackets.

From the physical point of view, the linear nonclassical momentum exactly corresponds to a Gaussian wavepacket evolving in time in a locally quadratic potential. For general potentials, it can describe the dominant quantum effects in semiclassical systems, such as wavepacket bifurcation, wavepacket spread in energy, and moderate tunneling. The zero-point energy effects are often described often for a few vibrational periods, depending on the anharmonicity of a system.