Skip to main content
SpringerLink
Log in

Near-Optimal Encoding for Sigma-Delta Quantization of Finite Frame Expansions

  • Published:
Journal of Fourier Analysis and Applications Aims and scope Submit manuscript

Abstract

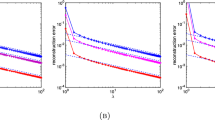
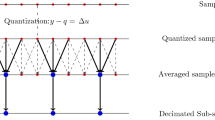
In this paper we investigate encoding the bit-stream resulting from coarse Sigma-Delta quantization of finite frame expansions (i.e., overdetermined representations) of vectors. We show that for a wide range of finite-frames, including random frames and piecewise smooth frames, there exists a simple encoding algorithm—acting only on the Sigma-Delta bit stream—and an associated decoding algorithm that together yield an approximation error which decays exponentially in the number of bits used. The encoding strategy consists of applying a discrete random operator to the Sigma-Delta bit stream and assigning a binary codeword to the result. The reconstruction procedure is essentially linear and equivalent to solving a least squares minimization problem.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Notes

  1. Moreover, there exists a covering with no more than \((\frac{3}{\epsilon} )^{d}\) elements (see, e.g., [24]).

  2. So that \(\mathbb{P} [{\bf t}_{j} \in\mathcal{S} ] = \mu (\mathcal{S} )\) for all measurable \(\mathcal{S} \subseteq\mathcal {D}\) and j∈[m].

  3. The specific form of the lower bound used for m below is taken from Theorem 12.12 of [12].

References

  1. Achlioptas, D.: Database-friendly random projections. In: Proceedings of the Twentieth ACM SIGMOD-SIGACT-SIGART Symposium on Principles of Database Systems, pp. 274–281. ACM, New York (2001)

    Chapter  Google Scholar 

  2. Ayaz, U.: Sigma-delta quantization and sturmian words. Master’s thesis, University of British Columbia (2009)

  3. Baraniuk, R., Davenport, M., DeVore, R., Wakin, M.: A simple proof of the restricted isometry property for random matrices. Constr. Approx. 28(3), 253–263 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  4. Benedetto, J., Powell, A., Yılmaz, Ö.: Sigma-delta (ΣΔ) quantization and finite frames. IEEE Trans. Inf. Theory 52(5), 1990–2005 (2006)

    Article  Google Scholar 

  5. Blum, J., Lammers, M., Powell, A., Yılmaz, Ö.: Sobolev duals in frame theory and sigma-delta quantization. J. Fourier Anal. Appl. 16(3), 365–381 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  6. Brady, D.J.: Multiplex sensors and the constant radiance theorem. Opt. Lett. 27(1), 16–18 (2002)

    Article  Google Scholar 

  7. Dasgupta, S., Gupta, A.: An elementary proof of a theorem of Johnson and Lindenstrauss. Random Struct. Algorithms 22(1), 60–65 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  8. Daubechies, I., DeVore, R.: Approximating a bandlimited function using very coarsely quantized data: a family of stable sigma-delta modulators of arbitrary order. Ann. Math. 158(2), 679–710 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  9. Deift, P., Krahmer, F., Güntürk, C.: An optimal family of exponentially accurate one-bit sigma-delta quantization schemes. Commun. Pure Appl. Math. 64(7), 883–919 (2011)

    Article  MATH  Google Scholar 

  10. Duarte, M.F., Davenport, M.A., Takhar, D., Laska, J.N., Sun, T., Kelly, K.F., Baraniuk, R.G.: Single-pixel imaging via compressive sampling. IEEE Signal Process. Mag. 25(2), 83–91 (2008)

    Article  Google Scholar 

  11. Fickus, M., Massar, M.L., Mixon, D.G.: Finite frames and filter banks. In: Finite Frames, pp. 337–379. Springer, Berlin (2013)

    Chapter  Google Scholar 

  12. Foucart, S., Rauhut, H.: A Mathematical Introduction to Compressive Sensing. Springer, Berlin (2013). ISBN 978-0-8176-4947-0

    Book  MATH  Google Scholar 

  13. Frankl, P., Maehara, H.: The Johnson-Lindenstrauss lemma and the sphericity of some graphs. J. Comb. Theory, Ser. B 44(3), 355–362 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  14. Güntürk, C.: One-bit sigma-delta quantization with exponential accuracy. Commun. Pure Appl. Math. 56(11), 1608–1630 (2003)

    Article  MATH  Google Scholar 

  15. Güntürk, C., Lagarias, J., Vaishampayan, V.: On the robustness of single-loop sigma-delta modulation. IEEE Trans. Inf. Theory 47(5), 1735–1744 (2001)

    Article  MATH  Google Scholar 

  16. Güntürk, C., Lammers, M., Powell, A., Saab, R., Yılmaz, Ö.: Sobolev duals for random frames and sigma-delta quantization of compressed sensing measurements. Found. Comput. Math. 13, 1–36 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  17. Hein, S., Ibraham, K., Zakhor, A.: New properties of sigma-delta modulators with dc inputs. IEEE Trans. Commun. 40(8), 1375–1387 (1992)

    Article  MATH  Google Scholar 

  18. Inose, H., Yasuda, Y.: A unity bit coding method by negative feedback. Proc. IEEE 51(11), 1524–1535 (1963)

    Article  Google Scholar 

  19. Johnson, W., Lindenstrauss, J.: Extensions of Lipschitz mappings into a Hilbert space. Contemp. Math. 26, 189–206 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  20. Kohman, T.P.: Coded-aperture x- or γ-ray telescope with least-squares image reconstruction. I. Design considerations. Rev. Sci. Instrum. 60(11), 3396–3409 (1989)

    Article  Google Scholar 

  21. Krahmer, F., Saab, R., Ward, R.: Root-exponential accuracy for coarse quantization of finite frame expansions. IEEE Trans. Inf. Theory 58(2), 1069–1079 (2012)

    Article  MathSciNet  Google Scholar 

  22. Krahmer, F., Saab, R., Yılmaz, Ö.: Sigma-delta quantization of sub-Gaussian frame expansions and its application to compressed sensing (2013). Preprint arXiv:1306.4549

  23. Krahmer, F., Ward, R.: New and improved Johnson-Lindenstrauss embeddings via the restricted isometry property. SIAM J. Math. Anal. 43(3), 1269–1281 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  24. Lorentz, G., von Golitschek, M., Makovoz, Y.: Constructive Approximation: Advanced Problems. Grundlehren der Mathematischen Wissenschaften. Springer, Berlin (1996)

    Book  MATH  Google Scholar 

  25. Norsworthy, S., Schreier, R., Temes, G., et al.: Delta-Sigma Data Converters: Theory, Design, and Simulation, vol. 97. IEEE Press, New York (1997)

    Google Scholar 

  26. Powell, A., Saab, R., Yılmaz, Ö.: Quantization and finite frames. In: Casazza, P., Kutinyok, G. (eds.) Finite Frames: Theory and Applications, pp. 305–328. Birkhauser, Basel (2012)

    Google Scholar 

  27. Von Neumann, J.: Distribution of the ratio of the mean square successive difference to the variance. Ann. Math. Stat. 12(4), 367–395 (1941)

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Michigan State University, East Lansing, USA

    Mark Iwen

  2. Department of Electrical and Computer Engineering, Michigan State University, East Lansing, USA

    Mark Iwen

  3. Department of Mathematics, University of California, San Diego, San Diego, USA

    Rayan Saab

Authors
  1. Mark Iwen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Rayan Saab
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mark Iwen.

Additional information

Communicated by Joel Tropp.

M.I. was supported in part by NSA grant H98230-13-1-0275. R.S. was supported in part by a Banting Postdoctoral Fellowship, administered by the Natural Sciences and Engineering Research Council of Canada (NSERC). The majority of the work reported on herein was completed while the authors were visiting assistant professors at Duke University.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Iwen, M., Saab, R. Near-Optimal Encoding for Sigma-Delta Quantization of Finite Frame Expansions. J Fourier Anal Appl 19, 1255–1273 (2013). https://doi.org/10.1007/s00041-013-9295-0

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00041-013-9295-0

Keywords

AMS Subject Classification

Search

Navigation