Skip to main content
SpringerLink
Log in

Wave speeds for the FKPP equation with enhancements of the reaction function

  • Published:
Zeitschrift für angewandte Mathematik und Physik Aims and scope Submit manuscript

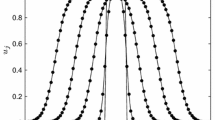
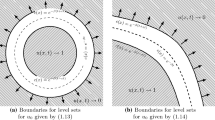
Abstract

In classes of N-particle systems and lattice models, the speed of front propagation is approximated by that of the corresponding continuum model, and for many such systems, the rate of convergence to the continuum speed is known to be slow as N → ∞. This slow convergence has been captured by including a cutoff function on the reaction terms in the continuum models. For example, the Fisher–Kolmogorov–Petrowskii–Piscounov (FKPP) equation with a cutoff has fronts that travel at the speed \({c \sim c_{\rm FKPP} - \frac{\pi^2}{(\ln(N))^2}}\) , which agrees well with data from numerical simulations of the corresponding N-particle systems, where c FKPP is the linear spreading speed. In Panja and van Saarloos (Phys Rev E 66:015206, 2002), an example is presented in which a small enhancement of the reaction function causes the propagation speeds of fronts to be larger than c FKPP. Such front speeds are also observed in stochastic lattice models where the growth rates in the regime of few particles are modified. In this article, we analyze the dynamics of traveling fronts in the FKPP equation with the constant enhancement function employed by Panja and van Saarloos. We present formulas for the wave speeds, develop the criteria on the parameters for which the front speeds are larger than the linear spreading speed even in the limit in which the size of the cutoff domain vanishes, study the rate of approach as N → ∞, and identify the mechanisms in phase space by which the constant enhancement of the reaction function makes possible the larger than linear wave speeds. In addition, we extend these results to the FKPP equation with two other enhancement functions, which are also of interest for continuum level modeling of lattice models and many-particle systems in the regimes of small numbers of particles, namely a linear enhancement function and an enhancement that is uniform above the linearized reaction function. We also derive explicit formulas for the parameters in these problems. The mathematical techniques used herein are geometric singular perturbation theory, geometric desingularization, invariant manifold theory, and normal form theory, all from dynamical systems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Abramowitz, M., Stegun, I.A. (Eds.): Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, National Bureau of Standards, Applied Mathematics Series 55. Dover Publications, New York (1972)

  2. Allen S.M., Cahn J.W.: A macroscopic theory for antiphase boundary motion and its application to antiphase domain coarsening. Acta Metal. Mater. 27, 1085–1095 (1979)

    Article  Google Scholar 

  3. Aronson, D.G., Weinberger, H.F.: Nonlinear diffusion in population genetics, combustion, and nerve pulse propagation. In: Partial Differential Equations and Related Topics, Lecture Notes in Mathematics, vol. 446, pp. 5–49. Springer, Berlin (1975)

  4. Aronson D.G., Weinberger H.F.: Multidimensional nonlinear diffusion arising in population genetics. Adv. Math. 30, 33–76 (1978)

    Article  MATH  MathSciNet  Google Scholar 

  5. Benguria R.D., Depassier M.C.: Speed of pulled fronts with cutoff. Phys. Rev. E 75, 051106 (2007)

    Article  Google Scholar 

  6. Benguria R.D., Depassier M.C., Haikala V.: Effect of a cutoff on pushed and bistable fronts of the reaction–diffusion equation. Phys. Rev. E 76, 051101 (2007)

    Article  Google Scholar 

  7. Bonckaert P.: Partially hyperbolic fixed points with constraints. Trans. Am. Math. Soc. 348, 997–1011 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  8. Bramson M., Calderoni P., DeMasi A., Ferrari P., Lebowitz J., Schonmann R.H.: Microscopic selection principle for a reaction–diffusion equation. J. Stat. Phys. 45, 905–920 (1986)

    Article  MATH  MathSciNet  Google Scholar 

  9. Breuer H.P., Huber W., Petruccione F.: Fluctuation effects on wave propagation in a reaction–diffusion process. Phys. D 73, 259–273 (1994)

    Article  MATH  Google Scholar 

  10. Breuer H.P., Huber W., Petruccione F.: The Macroscopic limit in a stochastic reaction–diffusion process. Europhys. Lett. 30, 69 (1995)

    Article  Google Scholar 

  11. Britton N.F.: Reaction–Diffusion Equations and Their Applications to Biology. Academic Press Inc., London (1986)

    MATH  Google Scholar 

  12. Brunet E., Derrida B.: Shift in the velocity of a front due to a cutoff. Phys. Rev. E 56(3), 2597–2604 (1997)

    Article  MathSciNet  Google Scholar 

  13. Chow S.-N., Li C., Wang D.: Normal Forms and Bifurcation of Planar Vector Fields. Cambridge University Press, Cambridge (1994)

    Book  MATH  Google Scholar 

  14. Cook J., Derrida B.: Lyapunov exponents of large, sparse random matrices and the problem of directed polymers with complex random weights. J. Stat. Phys. 61, 961–986 (1990)

    Article  MATH  MathSciNet  Google Scholar 

  15. Derrida B., Spohn H.: Polymers on disordered trees, spin glasses, and traveling waves. J. Stat. Phys. 51, 817–840 (1988)

    Article  MATH  MathSciNet  Google Scholar 

  16. Dumortier, F.: Techniques in the theory of local bifurcations: blow-up, normal forms, nilpotent bifurcations, singular perturbations. In: Schlomiuk, D. (ed.), Bifurcations and Periodic Orbits of Vector Fields, NATO ASI Series C, vol. 408, pp. 19–73, Kluwer Acad. Publ., Dordrecht (1993)

  17. Dumortier F.: Sharp upperbounds for the number of large amplitude limit cycles in polynomial Lienard systems. Discrete Contin. Dyn. Syst. 32, 1465–1479 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  18. Dumortier, F., De Maesschalck, P.: Topics in singularities and bifurcations of vector fields. In: Ilyashenko, Y., Rousseau, C., Sabidussi, G. (eds.) Normal Forms, Bifurcations, and Finiteness Problems in Differential Equations, NATO Sci. Ser. II Math. Phys. Chem., vol. 137, pp. 33–86. Kluwer Acad. Publ., Dordrecht (2004)

  19. Dumortier F., Kaper T.J.: Wave speeds for pushed fronts in scalar reaction–diffusion equations with cut-off. RIMS Kokyuroku Bessatsu B31, 117–134 (2012)

    MATH  MathSciNet  Google Scholar 

  20. Dumortier F., Popović N., Kaper T.J.: The asymptotic critical wave speed in a family of scalar reaction–diffusion equations. J. Math. Anal. Appl. 326, 1007–1023 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  21. Dumortier F., Popović N., Kaper T.J.: The critical wave speed for the FKPP equation with cut-off. Nonlinearity 20, 855–877 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  22. Dumortier F., Popović N., Kaper T.J.: A geometric approach to bistable front propagation in scalar reaction–diffusion equations with cut-off. Phys. D 239, 1984–1999 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  23. Dumortier F., Roussarie R.: Canard cycles and center manifolds. Mem. A.M.S. 121(577), 1–100 (1996)

    MathSciNet  Google Scholar 

  24. Dumortier, F., Roussarie, R.: Geometric singular perturbation theory beyond normal hyperbolicity. In: Jones, C.K.R.T., Khibnik, A. (eds.) Multiple-Time-Scale Dynamical Systems, IMA Vol. Math. Appl., vol. 122, pp. 29–63. Springer, New York (2001)

  25. Dumortier F., Roussarie R., Sotomayor J.: Bifurcations of cuspidal loops. Nonlinearity 10, 1369–1408 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  26. Fisher R.A.: The wave of advance of advantageous genes. Ann. Eugenics 7, 355–369 (1937)

    Article  Google Scholar 

  27. Guckenheimer J., Holmes P.: Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields, Applied Mathematics Sciences Series, vol. 42. Springer, New York (1983)

    Book  Google Scholar 

  28. Keener J., Sneyd J.: Mathematical Physiology, Interdisciplinary Applied Mathematics vol. 8. Springer, New York (1998)

    Google Scholar 

  29. Kerstein A.R.: Computational study of propagating fronts in a lattice-gas model. J. Stat. Phys. 45, 921–931 (1986)

    Article  Google Scholar 

  30. Kessler D.A., Ner Z., Sander L.M.: Front propagation: precursors, cutoffs, and structural stability. Phys. Rev. E 58(1), 107–114 (1998)

    Article  Google Scholar 

  31. Kolmogorov A.N., Petrowskii I.G., Piscounov N.: Etude de l’équation de la diffusion avec croissance de la quantité de matiére et son application à un problème biologique. Moscow Univ. Math. Bull. 1, 1–25 (1937)

    Google Scholar 

  32. Krupa M., Szmolyan P.: Extending geometric singular perturbation theory to nonhyperbolic points–fold and canard points in two dimensions. SIAM J. Math. Anal. 33, 286–314 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  33. Krupa M., Szmolyan P.: Relaxation oscillation and canard explosion. J. Differ. Equ. 174, 312–368 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  34. Mai J., Sokolov I.M., Blumen A.: Front propagation in one-dimensional autocatalytic reactions: The breakdown of the classical picture at small particle concentrations. Phys. Rev. E 62, 141–145 (2000)

    Article  Google Scholar 

  35. Méndez V., Campos D., Zemskov E.P.: Variational principles and the shift in the front speed due to a cutoff. Phys. Rev. E 72(5), 056113 (2005)

    Article  MathSciNet  Google Scholar 

  36. Panja D., van Saarloos W.: Fronts with a growth cut-off but with speed higher than the linear spreading speed. Phys. Rev. E, 66, 015206 (2002)

    Article  Google Scholar 

  37. Popović, N.: Front speeds, cut-offs, and desingularization: a brief case study in fluids and waves, Contemp. Math., vol. 440, pp. 187–195. Amer. Math. Soc., Providence, RI (2007)

  38. Popović N.: A geometric analysis of front propagation in a family of degenerate reaction–diffusion equations with cut-off. Z. Angew. Math. Phys. 62, 405–437 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  39. Popović N.: A geometric analysis of front propagation in an integrable Nagumo equation with a linear cut-off. Phys. D 241, 1976–1984 (2012)

    Article  MathSciNet  Google Scholar 

  40. Popović N., Szmolyan P.: A geometric analysis of the Lagerstrom model problem. J. Differ. Equ. 199, 290–325 (2004)

    Article  MATH  Google Scholar 

  41. Sternberg S.: On the structure of local homeomorphisms of Euclidean n-space II. Am. J. Math. 80, 623–631 (1958)

    Article  MATH  MathSciNet  Google Scholar 

  42. van Saarloos W.: Front propagation into unstable states. Phys. Rep. 386, 29–222 (2003)

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Universiteit Hasselt, Campus Diepenbeek, Agoralaan Gebouw D, 3590, Diepenbeek, Belgium

    Freddy Dumortier

  2. Department of Mathematics and Statistics, Boston University, 111 Cummington Mall, Boston, MA, 02215, USA

    Tasso J. Kaper

Authors
  1. Freddy Dumortier
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Tasso J. Kaper
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Tasso J. Kaper.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Dumortier, F., Kaper, T.J. Wave speeds for the FKPP equation with enhancements of the reaction function. Z. Angew. Math. Phys. 66, 607–629 (2015). https://doi.org/10.1007/s00033-014-0422-9

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00033-014-0422-9

Mathematics Subject Classification (1991)

Keywords

Search

Navigation