8.1 Introduction

In nature, there are four different fundamental forces that explain interactions of objects: electromagnetic, strong, weak, and gravitational forces. The gravitational and electromagnetic forces produce long-range interactions, and the strong and weak forces produce interactions at subatomic scales. Understanding the fundamental forces of nature has long been a profound goal in physics research. Each of the fundamental forces is characterized by its strength, effective range, and the nature of the particles that mediate the interaction. The Standard Model of particle physics is a theory describing three of the four known fundamental interactions and all elementary particles; the quantum description of the role of gravity in the Standard Model is incomplete. Although the Standard Model has had great success in describing most interactions and particles, there is a considerable piece of the puzzle missing: the nature of the dark matter. Recently, new theories have postulated the existence of new “exotic” interactions that may explain various anomalous phenomena that remain unexplained by the Standard Model [1]. In these theories, exotic interactions with very weak strength have ranges from sub-mm scales all the way to cosmological scales. The search for such exotic interactions has been motivated in recent decades by the cosmological dilemmas of dark matter and dark energy [2, 3].

8.1.1 Dark Matter and New Spin-Dependent Interactions

Over the last few decades, astrophysical observations have indicated that most of mass-energy density of our universe is in the form of non-baryonic components belonging to what is known as the “dark sector.” Recent measurements show that ordinary baryonic matter contributes only about 4% of the energy content in the universe. The remaining 96% of the energy content belongs to the dark sector with two sub-components called “dark energy” and “dark matter.” The dark energy is believed to accelerate the expansion of the universe and contributes about 68% of the energy content in our universe. Although the accelerating expansion of our universe has been verified by astronomical observations, the existence of dark energy is still under debate [4, 5].

On the other hand, the reality of dark matter is becoming more evident based on the observation of the abundance of light elements and the measurement of the cosmic microwave background (CMB), as well as from the measured galactic rotation curves, galactic cluster dynamics, and gravitational lensing studies (see Chap. 1). Dark matter is considered to be responsible for approximately 85% of the matter density and about 25% of total energy density in the universe.

The fact that dark matter cannot be explained in the framework of the Standard Model of particle physics creates the need for a new theory that extends beyond the Standard Model. There are two main categories of beyond-Standard-Model theories to explain dark matter: one class of theories suggest that dark matter is composed of neutralinos or other weakly interacting massive particles (WIMPs) and the other suggests that dark matter is made of axions or other weakly interacting sub-eV particles (WISPs), another term for the ultralight bosons described throughout this text. WIMPs have been a promising solution for the dark matter problem since they were first introduced [6]. However, non-observation of WIMPs at the Large Hadron Collider (LHC) and in other direct-detection dark matter experiments over past few decades gives rise to strong motivation to look for WISPs. A wide variety of theories beyond the Standard Model have predicted the existence of such weakly coupled scalar, pseudoscalar, vector, and axial-vector bosons with very light mass as dark matter candidates [2].

These ultralight bosons arise from spontaneous symmetry breaking of global \(\mathbb {U}(1)\) symmetries at a scale of f and their effective couplings to standard model particles are suppressed by a factor on the scale f [7,8,9,10], as discussed in Chap. 2. Examples include majorons which result from breaking of the global \(\mathbb {U}(1)\) B −L symmetry where B is the baryon number and L is the lepton number. Majorons could explain the small neutrino mass \(m_{\nu }\sim m_{l}^{2}/f_{L}\) where ml is the mass of the associated charged lepton and fL is the symmetry-breaking scale for lepton number [11, 12]. Familons are WISPs that arise from breaking of family symmetry which normally refers to various discrete, global, or local symmetries between quark-lepton families or generations. Familions couple to a divergence of current changing flavor quantum number and therefore can be emitted in flavor changing decays [13].

These ultralight bosons could be an answer not only for the dark matter problem, but also for many other fundamental questions in physics, such as the CP problem in QCD [7, 14, 15]. One well-known example of such an ultralight boson is the axion. The axion remains the most prominent solution of the strong CP problem many decades after its prediction and is also a very well-motivated dark matter candidate, as discussed in Chaps. 1 and 2 and elsewhere throughout this book. Now the question is if these ultralight bosons could also be linked to new interactions that are yet to be observed? If such an exotic long-range interaction mediated by an ultralight boson is discovered, it would have a profound impact on our understanding of nature.

8.1.2 New Spin-Dependent Interactions

Weakly coupled, long-range interactions are a generic consequence of a spontaneously broken continuous symmetry as shown by Goldstone’s theorem. Goldstone’s theorem states that if a system that is invariant under a continuous, global symmetry has this symmetry broken so that the ground (vacuum) state is not invariant with respect to the global symmetry (referred to as a spontaneously broken symmetry—see Chap. 2), there must be a state in the spectrum of excitations of the system with zero energy (which can be created from the ground state by performing a spacetime-dependent symmetry transformation). This state is called a Goldstone mode or Goldstone boson. Since the Goldstone mode is “gapless” so that its energy vanishes when its momentum vanishes, i.e., as ω → 0 then k → 0 (see Fig. 2.1 and surrounding discussion), the Goldstone mode oscillates with infinite wavelength (λ →∞) to minimize the energy of the wave by reducing the momentum. Thus the Goldstone mode corresponds to massless particles traveling with the speed of light. Therefore, when the underlying symmetry is exact up to an energy scale f, the process always produces a massless Goldstone boson [16].

However, when the symmetry is explicitly broken, meaning that the symmetry becomes an approximate symmetry instead of an exact symmetry, the Goldstone boson acquires a very small mass rather than being exactly massless. Such particles are referred as to “pseudo-Goldstone bosons” (again, see Chap. 2). The pseudo-Goldstone bosons acquire a small mass of order mb ∼ Λ2∕f that depends on the symmetry-breaking scale Λ of the continuous Lagrangian [17]. If such bosons have sufficiently small mass they have a macroscopic Compton wavelength and can mediate macroscopic interactions [18].

The ultralight pseudo-Goldstone bosons can couple to fundamental fermions through scalar and pseudoscalar vertices for spin-0 bosons, vector, axial-vector, tensor, and pseudotensor vertices for spin-1 bosons. The interactions can be classified in terms of a multipole expansion: well-known examples of possible allowed interactions are monopole-monopole, monopole-dipole, and dipole-dipole. Monopole-dipole interactions include a scalar coupling, gs, and a pseudoscalar coupling, gp, thereby violating P and T symmetry. This can be seen in the nonrelativistic limit, where this interaction is proportional to gsgpσ ⋅r where σ is the spin of one particle, and r is the distance between two particles: the spin σ is P-even and T-odd, whereas the position vector r is P-odd and T-even, making their scalar product, σ ⋅r, P- and T-odd. Dipole-dipole interactions are dependent on the spins of two particles, σ1 and σ2, and can be either velocity-independent or velocity-dependent. Spin-dependent but velocity-independent dipole-dipole interactions are proportional to \(g_{p}^{2} \boldsymbol {\sigma }_{1}\cdot \boldsymbol {\sigma }_{2}\) [19,20,21]. Depending on the model, the monopole and dipole couplings can occur for electrons and/or nuclei.

An interesting point is that, although the couplings can be extremely feeble for the interaction between single particles, a macroscopic object composed of many particles, e.g., on the order of ∼1022–1023, would produce a coherent field, thus enhancing the signal as compared to a single particle and potentially making it detectable with a sufficiently sensitive laboratory experiment [18]. Many of the experimental tests of such interactions have been done with polarized gases [22]. Torsion balance experiments also have recently set new limits on both monopole-dipole interactions and dipole-dipole interactions. But laboratory constraints on possible new interactions in the mesoscopic range, which is roughly defined in a scale of between ∼μm to ∼mm, have not yet been well developed [23]. In many cases, laboratory measurements combined with astrophysical data have produced the most stringent constraints on the products of the coupling constants gs and gp. These coupling strengths are constrained by experiments or astrophysical observations. For the QCD axion, gs and gp are related to the axion mass as they are fixed by the axion decay constant fa (see discussion in Chap. 2):

$$\displaystyle \begin{aligned} 6\times 10^{-27} \left( { \frac{10^{9}\mathrm{GeV}}{f_{a}}}\right) < g_{s} < 10^{-21} \left( { \frac{10^{9}\mathrm{GeV}}{f_{a}}}\right)~, \end{aligned} $$
(8.1)

and

$$\displaystyle \begin{aligned} g_{p}=\frac{C_{f}m_{f}} {f_{a}}=C_{f}\times 10^{-9} \left( { \frac{m_{f}}{1\mathrm{GeV}}}\right) \left( { \frac{10^{9}\mathrm{GeV}}{f_{a}}}\right)~, \end{aligned} $$
(8.2)

where Cf is a dimensionless coupling constant for the particular fermion considered.

8.2 Spin-Dependent Interactions Mediated by Light Bosons: Classification

Spin-dependent interactions mediated by ultralight bosons were first described by Moody and Wilczek along with some suggestions for experimental tests in Ref. [18]. They proposed experimental tests to detect axions via the macroscopic forces mediated by axion exchange. A phenomenological theory was also developed by Dobrescu and Mocioiu [1]. They listed all possible spin-dependent interactions satisfying rotational invariance and standard assumptions of quantum field theory. Fadeev et al. later revisited and derived nonrelativistic potentials mediated by spin-0 and spin-1 bosons [24]. They have updated the Dobrescu and Mocioiu’s work with more details and several corrections, for example, including contact-terms in the coordinate-space nonrelativistic potentials, which can affect atomic- and molecular-scale experiments, in particular [25].

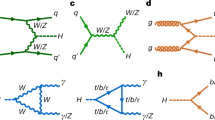
Exotic spin-dependent interactions that are mediated by spin-0 and spin-1 bosons between two fermions with masses m1 and m2, and spins σ1 and σ2 can be derived from the elastic scattering of two fermions in the nonrelativistic limit as shown in Fig. 8.1. The scattering is mediated by a boson of mass mb and the four-momentum q is transferred from fermion 2 to fermion 1.

Fig. 8.1
figure 1

The Feynman diagram of elastic scattering between two fermions with masses m1 and m2 mediated by ultralight bosons with mass mb with four-momentum transferred from vertex 2 to vertex 1

Full size image

The Lagrangian describing the interaction between fermions ψ mediated by a spin-0 boson ϕ is given as:

$$\displaystyle \begin{aligned} {\mathcal{L}}_{\psi}=\phi\sum_{ \psi }\bar{\psi }\left( { g_{s,\psi}+i \gamma_{5} g_{p,\psi}} \right)\psi~, {} \end{aligned} $$
(8.3)

where γ5 = −iγ0γ1γ2γ3 are Dirac matrices. The interaction constants gs,ψ and gp,ψ parametrize the scalar and pseudoscalar interaction strengths, respectively.

The Lagrangian describing the interaction mediated by exchange of a massive spin-1 boson (denoted Z′) can be separated from that describing the interaction mediated by a massless spin-1 boson (denoted γ′) due to the presence of a longitudinal polarization appearing for Z′ bosons. In the case of a massive spin-1 Z′ boson, the Lagrangian becomes

$$\displaystyle \begin{aligned} {\mathcal{L}}_{Z^{\prime}}=Z^{\prime}_{\mu}\sum_{ \psi }\bar{\psi }\left( { g_{V,\psi}+ \gamma_{5} g_{A,\psi}} \right)\psi~, {} \end{aligned} $$
(8.4)

where gV,ψ and gA,ψ are the vector and axial-vector interaction strengths, respectively. The interaction Lagrangian describing the exchange of a massless spin-1 γ′ boson is:

$$\displaystyle \begin{aligned} \mathcal{L}_{\gamma'}=\frac{v_{h}}{\Lambda^{2}}P_{\mu\nu}\sum_{\psi}\bar{\psi}\sigma^{\mu\nu}\left(\mathrm{Re}(C_{\psi})+i\gamma_{5}\mathrm{Im}(C_{\psi})\right)\psi~, {} \end{aligned} $$
(8.5)

where ψ denotes the fermion field, vh is the Higgs vacuum expectation value, Λ is the ultraviolet energy cut-off scale of the Lagrangian in Eq. (8.5),

$$\displaystyle \begin{aligned} P_{\mu\nu}=\partial_{\mu}A_{\nu}-\partial_{\nu}A_{\mu} \end{aligned} $$
(8.6)

is the field strength tensor of the massless spin-1 boson γ′,

$$\displaystyle \begin{aligned} \sigma^{\mu\nu}=\frac{i}{2}[\gamma^{\mu},\gamma^{\nu}]~, \end{aligned} $$
(8.7)

and Re(Cψ) and Im(Cψ) denote the respective interaction strengths. The details of these cases can be found in Ref. [24].

The nonrelativistic momentum-space potential V (q) can be estimated from the scattering matrix M(q) in the leading order as

$$\displaystyle \begin{aligned} V(q)\approx-\frac{M(q)}{4m_{1}m_{2}}~. {} \end{aligned} $$
(8.8)

The nonrelativistic coordinate-space potential can be obtained by applying the three-dimensional Fourier transformation to Eq. (8.8):

$$\displaystyle \begin{aligned} V(r)=\int\frac{d^{3}q}{(2\pi)^{3}}V(q)e^{i\boldsymbol{q}\cdot\boldsymbol{r}}=\int\frac{d^{3}q}{(2\pi)^{3}}\frac{-M(q)}{4m_{1}m_{2}}e^{i\boldsymbol{q}\cdot\boldsymbol{r}}. {} \end{aligned} $$
(8.9)

8.2.1 Interactions Mediated by Massive Spin-0 Bosons

Interactions mediated by a massive spin-0 boson can be derived from the Lagrangian in Eq. (8.3). They can be classified by three different type of interactions: scalar-scalar, pseudoscalar-scalar, and pseudoscalar-pseudoscalar interactions.

8.2.1.1 Scalar-Scalar Interaction

The scattering matrix of the interaction between two fermions via the exchange of a spin-0 boson at the tree level can be calculated from the Feynman rules in momentum space,

$$\displaystyle \begin{aligned} iM(q)=[i\bar{u}(p_{f,1})g_{s,1}u(p_{i,1})][i\bar{u}(p_{f,2})g_{s,2}u(p_{i,2})]\frac{i}{q^{2}-m_b^{2}}~, {} \end{aligned} $$
(8.10)

where mb is the mass of the boson which mediates the interaction and u(p) is the spinor. The four-momentum of the mediating boson is defined as

$$\displaystyle \begin{aligned} \boldsymbol{q}=\boldsymbol{p}_{1,f}-\boldsymbol{p}_{1,i}=\boldsymbol{p}_{2,i}-\boldsymbol{p}_{2,f}~, \end{aligned} $$
(8.11)

and the average momenta of each fermion are defined as

$$\displaystyle \begin{aligned} \boldsymbol{p}_{1}=\frac{\boldsymbol{p}_{1,i}+\boldsymbol{p}_{1,f}}{2}~, \end{aligned} $$
(8.12)
$$\displaystyle \begin{aligned} \boldsymbol{p}_{2}=\frac{\boldsymbol{p}_{2,i}+\boldsymbol{p}_{2,f}}{2}~, \end{aligned} $$
(8.13)

where the labels 1, 2 denote the fermions and i, f denote the initial and final state of the fermions as defined from Fig. 8.1. In the nonrelativistic limit (|p|≪ m), the momentum-space Dirac spinor u(p) can be expanded up to the first order in p as

$$\displaystyle \begin{aligned} u(p) \approx \sqrt{m} \begin{pmatrix} \displaystyle{\left(1-\frac{\boldsymbol{\sigma}\cdot\boldsymbol{p}}{2m}\right)}\xi \\[0.3cm] \displaystyle{\left(1+\frac{\boldsymbol{\sigma}\cdot\boldsymbol{p}}{2m}\right)}\xi \end{pmatrix}~, {} \end{aligned} $$
(8.14)

where ξ is a 2 × 1 matrix with normalization ξ†ξ = 1 (ξ is often also called a spinor in the literature).

In the nonrelativistic limit, by using Eqs. (8.10) and (8.14) in the expression for the coordinate-space potential (8.9), the potential of the scalar-scalar interaction becomes

$$\displaystyle \begin{aligned} V_{ss}(r)= -g_{s,1}g_{s,2}\mathcal{V}_{ss}=-g_{s,1}g_{s,2}\int\frac{d^{3}q}{(2\pi)^{3}}\frac{1}{|\boldsymbol{q}|{}^2+m_b^{2}}e^{i\boldsymbol{q}\cdot\boldsymbol{r}}~, {} \end{aligned} $$
(8.15)

where we note that in the nonrelativistic limit the spacelike component of the momentum dominates, \(q^2 = q_0^2 - |\boldsymbol {q}|{ }^2 \approx -|\boldsymbol {q}|{ }^2\). The integration in spherical coordinates results in the potential Vss(r):

$$\displaystyle \begin{aligned} V_{ss}(r)=-\frac{g_{s,1}g_{s,2}}{4\pi}\frac{e^{-m_b r}}{r}~, {} \end{aligned} $$
(8.16)

which includes the well-known Yukawa-type factor.

8.2.1.2 Pseudoscalar-Scalar Interaction

In the case of a pseudoscalar-scalar interaction between two fermions, the scattering matrix can be obtained from the Feynman diagram as follows:

$$\displaystyle \begin{aligned} iM(q)=[i^{2}\bar{u}(p_{f,1})g_{p,1}\gamma_{5}u(p_{i,1})][i\bar{u}(p_{f,2})g_{s,2}u(p_{i,2})]\frac{i}{q^{2}-m_b^{2}}, {} \end{aligned} $$
(8.17)

where we assumed a pseudoscalar coupling on vertex 1 (gp,1) and a scalar coupling on vertex 2 (gs,2), respectively.

In the nonrelativistic limit, the spinor products in the scattering amplitude become

$$\displaystyle \begin{aligned} &\bar{u}(p_{f})u(p_{i})\approx 2m,\\ &\bar{u}(p_{f})\gamma^{5}u(p_{i})\approx \mp \boldsymbol{\sigma}\cdot\boldsymbol{q}, \end{aligned} $$
(8.18)

where the sign of the pseudoscalar vertex depends on the direction of momentum transfer.

This scalar-pseudoscalar interaction is normally called a monopole-dipole interaction in the sense of the multipole expansion. From Eqs. (8.18) and (8.17), the potential describing the monopole (gs) and dipole (igpσ ⋅q∕2m) interaction between two fermions can be expressed based on Eq. (8.9) as,

$$\displaystyle \begin{aligned} {V}_{ps}(r)=-\frac{g_{p,1}g_{s,2}}{2im_{1}}\mathcal{V}_{ps}(r)=-\frac{g_{p,1}g_{s,2}}{2im_{1}}\int\frac{d^{3}q}{(2\pi)^{3}}\frac{\boldsymbol{\sigma}_1\cdot \boldsymbol{q}}{|\boldsymbol{q}|{}^2+m_b^{2}}e^{i\boldsymbol{q}\cdot\boldsymbol{r}}, \end{aligned} $$
(8.19)

where the integration function \(\mathcal {V}_{ps}(r)\) can be obtained by taking the inner product between the spin vector and the gradient of \(\mathcal {V}_{ss}(r)\):

$$\displaystyle \begin{aligned} \mathcal{V}_{ps}(r)=-i\boldsymbol{\sigma}_1\cdot\nabla\mathcal{V}_{ss}(r). {} \end{aligned} $$
(8.20)

With the calculation of \(\mathcal {V}_{ps}(r)\), the interaction potential becomes

$$\displaystyle \begin{aligned} V_{ps}(r)=-\frac{g_{p,1}g_{s,2}}{8\pi m_{1}}\left(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}}\right)\left(\frac{m_b}{r}+\frac{1}{r^{2}}\right)e^{-m_br}. {} \end{aligned} $$
(8.21)

8.2.1.3 Pseudoscalar-Pseudoscalar Interaction

The scattering matrix describing a pseudoscalar-pseudoscalar interaction is given by

$$\displaystyle \begin{aligned} iM(q)=[i^{2}\bar{u}(p_{f,1})g_{p,1}\gamma_{5}u(p_{i,1})][i^{2}\bar{u}(p_{f,2})g_{p,2}\gamma_{5}u(p_{i,2})]\frac{i}{q^{2}-m_b^{2}}~. \end{aligned} $$
(8.22)

In this matrix, the momentum is transferred from vertex two to vertex one. From Eq. (8.18), the scattering matrix in the nonrelativistic limit becomes,

$$\displaystyle \begin{aligned} iM(q)=-(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{q})(\boldsymbol{\sigma}_{2}\cdot\boldsymbol{q})\frac{-i}{|\boldsymbol{q}|{}^2+m_b^{2}}~. \end{aligned} $$
(8.23)

From Eq. (8.9), the potential for the pseudoscalar-pseudoscalar interaction between two fermions is

$$\displaystyle \begin{aligned} V_{pp}(r)=\frac{g_{p,1}g_{p,2}}{4m_{1}m_{2}} \mathcal{V}_{pp}(r)=\frac{g_{p,1}g_{p,2}}{4m_{1}m_{2}} \int\frac{d^{3}q}{(2\pi)^{3}}\frac{(\boldsymbol{\sigma}_{1} \cdot \boldsymbol{q})(\boldsymbol{\sigma}_{2}\cdot \boldsymbol{q})}{|\boldsymbol{q}|{}^2+m_b^{2}}e^{i\boldsymbol{q}\cdot\boldsymbol{r}}~, {} \end{aligned} $$
(8.24)

where the labels 1 and 2 indicate each fermion with mass m1 and m2, respectively. The spin product in the integral part in Eq. (8.24) can be expressed via the summation of each spin state a, b = 1, 2, 3:

$$\displaystyle \begin{aligned} \mathcal{V}_{pp}(r)=\sum_{a}\sum_{b}\int\frac{d^{3}q}{(2\pi)^{3}}\frac{\sigma_{1,a}\sigma_{2,b}q_{a}q_{b}}{|\boldsymbol{q}|{}^2+m_b^{2}}e^{i\boldsymbol{q}\cdot\boldsymbol{r}}~. \end{aligned} $$
(8.25)

Analogously to the method used in Eq. (8.20), \(\mathcal {V}_{pp}(r)\) can be derived from \(\mathcal {V}_{ss}(r)\) in the following way:

$$\displaystyle \begin{aligned} \mathcal{V}_{pp}(r)=-\sum_{a}\sum_{b}\sigma_{1,a}\sigma_{2,b}\partial_{a}\partial_{b}\mathcal{V}_{ss}. {} \end{aligned} $$
(8.26)

The evaluation of partial derivatives in Eq. (8.26) yields

$$\displaystyle \begin{aligned} 4\pi\partial_{a}\partial_{b}\mathcal{V}_{ss}=\left(\partial_{a}\partial_{b}e^{-m_br}\right)\frac{1}{r}+2\left(\partial_{a}e^{-m_br}\right)\left(\partial_{b}\frac{1}{r}\right)+e^{-m_br}\left(\partial_{a}\partial_{b}\frac{1}{r}\right). {} \end{aligned} $$
(8.27)

By completing the calculation of Eq. (8.27), the potential for the pseudoscalar-pseudoscalar interaction becomes

(8.28)

8.2.2 Interactions Mediated by Massive Spin-1 Bosons

Interactions between two fermions mediated by a massive spin-1 boson (Z′) can be described using the Lagrangian in Eq. (8.4). In this case, the terms describing the possible vertex interactions are given by

$$\displaystyle \begin{aligned} &g_{V}\bar{u}(p_{f})\gamma^{\mu}u(p_{i})~,\\ &g_{A}\bar{u}(p_{f})\gamma^{\mu}\gamma^{5}u(p_{i})~. \end{aligned} $$
(8.29)

Using approximate nonrelativistic solutions, the spinor products for each vertex can be simplified as

$$\displaystyle \begin{aligned} &\bar{u}(p_{f})\gamma^{0}u(p_{i})\approx 2m,\\ &\bar{u}(p_{f})\boldsymbol{\gamma}u(p_{i})\approx 2\boldsymbol{p}\pm i\boldsymbol{q}\times\boldsymbol{\sigma},\\ &\bar{u}(p_{f})\gamma^{0}\gamma^{5}u(p_{i})\approx 2\boldsymbol{\sigma}\cdot\boldsymbol{p},\\ &\bar{u}(p_{f})\boldsymbol{\gamma}\gamma^{5}u(p_{i})\approx 2m\boldsymbol{\sigma}, \end{aligned} $$
(8.30)

where ± in the vector component is the direction of vector vertex.

8.2.2.1 Vector-Vector Interaction

The scattering matrix element of the vector-vector interaction is given by

$$\displaystyle \begin{aligned} iM(q)=g_{V,1}g_{V,2}[i\bar{u}(p_{f,1})\gamma^{\mu}u(p_{i,1})][i\bar{u}(p_{f,2})\gamma^{\nu}u(p_{i,2})]i\frac{g_{\mu\nu}-q_{\mu}q_{\nu}/m_b^{2}}{m_b^{2}-q^{2}}. \end{aligned} $$
(8.31)

Since the vector current is conserved in the interaction, the qμqν term in the propagator can be neglected. Therefore the scattering matrix can be simplified in the nonrelativistic limit as

$$\displaystyle \begin{aligned} iM(q)\approx -ig_{V,1}g_{V,2}\frac{4m_{1}m_{2}-(2\boldsymbol{p}_{1}-i\boldsymbol{q}\times\boldsymbol{\sigma}_{1})\cdot(2\boldsymbol{p}_{2}+i\boldsymbol{q}\times\boldsymbol{\sigma}_{2})}{|\boldsymbol{q}|{}^2+m_b^{2}}. \end{aligned} $$
(8.32)

The potential describing the vector-vector interaction in momentum space is approximately

$$\displaystyle \begin{aligned} V(q)\approx &\frac{g_{V,1}g_{V,2}}{m_b^{2}+|\boldsymbol{q}|{}^2} \\ &\times\left(1-\frac{(\boldsymbol{q}\times\boldsymbol{\sigma}_{ 1})\cdot(\boldsymbol{q}\times\boldsymbol{\sigma}_{2})}{4m_{1}m_{2}}-\frac{\boldsymbol{p}_{1}\cdot\boldsymbol{p}_{2}}{m_{1}m_{2}}-i\frac{\boldsymbol{p}_{1}\cdot(\boldsymbol{q}\times\boldsymbol{\sigma}_{2})-\boldsymbol{p}_{2}\cdot(\boldsymbol{q}\times\boldsymbol{\sigma}_{1})}{2m_{1}m_{2}}\right). \end{aligned} $$
(8.33)

In the nonrelativistic limit, the above expression can be simplified as

$$\displaystyle \begin{aligned} V(q)\approx\frac{g_{V,1}g_{V,2}}{m_b^{2}+|\boldsymbol{q}|{}^2}\left(1-\frac{(\boldsymbol{q}\times\boldsymbol{\sigma}_{ 1})\cdot(\boldsymbol{q}\times\boldsymbol{\sigma}_{2})}{4m_{1}m_{2}}\right). \end{aligned} $$
(8.34)

The dot product of the cross products between spin and momentum of each fermion can be decomposed into

$$\displaystyle \begin{aligned} (\boldsymbol{q}\times\boldsymbol{\sigma}_{ 1})\cdot(\boldsymbol{q}\times\boldsymbol{\sigma}_{2})=|\boldsymbol{q}|{}^{2}(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{\sigma}_{2})-(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{1})(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{2})~. \end{aligned} $$
(8.35)

The coordinate-space potential can be obtained as

$$\displaystyle \begin{aligned} V_{VV}(r) = g_{V,1}g_{V,2} \int \frac{d^{3}q}{(2\pi)^{3}} \frac{e^{i\boldsymbol{q} \cdot \boldsymbol{r}}}{m_b^{2}+|\boldsymbol{q}|{}^2} \left(1-\frac{|\boldsymbol{q}|{}^2(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{\sigma}_{2})-(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{1})(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{2})}{4m_{1}m_{2}}\right). {} \end{aligned} $$
(8.36)

The first term of Eq. (8.36) is simply the Yukawa potential which we already studied in the case of scalar-scalar interaction, Eq. (8.16).

$$\displaystyle \begin{aligned} \int\frac{d^{3}q}{(2\pi)^{3}}\frac{e^{i\boldsymbol{q}\cdot\boldsymbol{r}}}{m_b^{2}+|\boldsymbol{q}|{}^2}=\frac{e^{-m_br}}{4\pi r}. \end{aligned} $$
(8.37)

The second and third terms of Eq. (8.36) can be evaluated by using the results from our analysis of the pseudoscalar-pseudoscalar interaction,

$$\displaystyle \begin{aligned} \int\frac{d^{3}q}{(2\pi)^{3}}\frac{q^2 e^{i\boldsymbol{q}\cdot\boldsymbol{r}}}{m_b^{2}+|\boldsymbol{q}|{}^2}=-\nabla^{2}{\left(\frac{e^{-m_br}}{4\pi r}\right)} = \left(\delta(\boldsymbol{r})-\frac{m_b^{2}}{4\pi r}\right)e^{-m_br}~, \end{aligned} $$
(8.38)

and

$$\displaystyle \begin{aligned} \int\frac{d^{3}q}{(2\pi)^{3}}\frac{(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{1})(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{2})e^{i\boldsymbol{q}\cdot\boldsymbol{r}}}{m_b^{2}+|\boldsymbol{q}|{}^2} & = \frac{\boldsymbol{\sigma}_{1}\cdot\boldsymbol{\sigma}_{2}}{4\pi}\left(\frac{1}{r^{3}}+\frac{m_b}{r^{2}}+\frac{4\pi}{3}\delta(\boldsymbol{r})\right)e^{-m_br} \\ &\quad -\frac{(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}})(\boldsymbol{\sigma}_{2}\cdot\hat{\boldsymbol{r}})}{4\pi}\left(\frac{3}{r^{3}}+\frac{3m_b}{r^{2}}+\frac{m_b^{2}}{r}\right)~. \end{aligned} $$
(8.39)

From these relationships, the interaction potential of the vector-vector interaction becomes

$$\displaystyle \begin{aligned} V_{VV}(r)&=g_{V,1}g_{V,2}\frac{e^{-m_br}}{4\pi r}\\&\quad +\frac{g_{V,1}g_{V,2}}{16\pi m_{1}m_{2}} \left(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{\sigma}_{2}\right)\left(\frac{1}{r^{3}}+\frac{m_b}{r^{2}}+\frac{m_b^{2}}{r}-\frac{8\pi}{3}\delta(\boldsymbol{r})\right)e^{-m_br} \\ &\quad -\frac{g_{V,1}g_{V,2}}{16\pi m_{1}m_{2}}(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}})(\boldsymbol{\sigma}_{2}\cdot\hat{\boldsymbol{r}})\left(\frac{3}{r^{3}}+\frac{3m_b}{r^{2}}+\frac{m_b^{2}}{r}\right)e^{-m_br}~. {} \end{aligned} $$
(8.40)

8.2.2.2 Axial-Vector-Vector Interaction

The potential describing the axial-vector-vector interaction can be calculated by assuming an axial-vector coupling in the first vertex and a vector coupling in the second vertex. In this case, the scattering matrix is given by

$$\displaystyle \begin{aligned} M(q)=-g_{A,1}g_{V,2}i[i\bar{u}(p_{f,1})\gamma^{\mu}\gamma_{5}u(p_{i,1})][i\bar{u}(p_{f,2})\gamma^{\nu}u(p_{i,2})]i\frac{g_{\mu\nu}-q_{\mu}q_{\nu}/m_b^{2}}{m_b^{2}-q^{2}}~. \end{aligned} $$
(8.41)

Since the vector current is conserved, qμJμ = 0 imposes the condition that the qμqν term vanishes. In the nonrelativistic limit, the momentum-space potential is

$$\displaystyle \begin{aligned} V(q) \approx \frac{g_{A,1}g_{V,2}}{4m_{1}m_{2}(m_b^{2}+|\boldsymbol{q}|{}^2)}\left[4m_{2}\boldsymbol{\sigma}_{1}\cdot\boldsymbol{p}_{1}-2m_{1}\boldsymbol{\sigma}_{1}\cdot(2\boldsymbol{p}_{2}+i\boldsymbol{q}\times\boldsymbol{\sigma}_{2})\right]~. \end{aligned} $$
(8.42)

It can be simplified as

$$\displaystyle \begin{aligned} V(q) \approx \frac{g_{A,1}g_{V,2}}{m_b^{2}+|\boldsymbol{q}|{}^2}\left[\boldsymbol{\sigma}_{1}\cdot\left(\frac{\boldsymbol{p}}{m_{1}}-\frac{\boldsymbol{p}_{2}}{m_{2}}\right)+i\frac{\boldsymbol{q}\cdot(\boldsymbol{\sigma}_{1}\times\boldsymbol{\sigma}_{2})}{2m_{2}}\right]. \end{aligned} $$
(8.43)

The coordinate-space potential can thus be obtained by applying a Fourier transform:

$$\displaystyle \begin{aligned} V_{AV}(r)&=\frac{g_{A,1}g_{V,2}}{4\pi}\boldsymbol{\sigma}_{1}\cdot\left(\frac{\boldsymbol{p}}{m_{1}}-\frac{\boldsymbol{p}_{2}}{m_{2}}\right)\frac{e^{-m_br}}{r}\\&\quad -\frac{g_{A,1}g_{V,2}}{8\pi m_{2}}(\boldsymbol{\sigma}_{1}\times\boldsymbol{\sigma}_{2})\cdot\hat{\boldsymbol{r}}\left(\frac{m_b}{r}+\frac{1}{r^{2}}\right)\frac{e^{-m_br}}{r}~. \end{aligned} $$
(8.44)

8.2.2.3 Axial-Vector-Axial-Vector Interaction

The main difference between an axial-vector-axial-vector interaction and a vector-vector interaction is that the axial current of each fermion is not conserved whereas the vector current is conserved. Therefore, in the case of the axial-vector-axial-vector interaction, it is necessary to consider the qμqν term in the propagator. The scattering matrix becomes

$$\displaystyle \begin{aligned} M(q)=-g_{A,1}g_{A,2}i[i\bar{u}(p_{f,1})\gamma^{\mu}\gamma_{5}u(p_{i,1})][i\bar{u}(p_{f,2})\gamma^{\nu}\gamma_{5}u(p_{i,2})]i\frac{g_{\mu\nu}-q_{\mu}q_{\nu}/m_b^{2}}{m_b^{2}-q^{2}}~,\end{aligned} $$
(8.45)

and so consequently the momentum-space potential can be written as

$$\displaystyle \begin{aligned} V(q) = \frac{g_{A,1}g_{A,2}}{m_b^{2}+|\boldsymbol{q}|{}^2}\left(\frac{\boldsymbol{\sigma}_{1}\cdot\boldsymbol{p}_{1}}{m_{1}}\frac{\boldsymbol{\sigma}_{2}\cdot\boldsymbol{p}_{2}}{m_{2}}-\boldsymbol{\sigma}_{1}\cdot\boldsymbol{\sigma}_{2}+\frac{(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{1})(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{2})}{m_b^{2}}\right)~. {} \end{aligned} $$
(8.46)

The first term in Eq. (8.46) can be neglected because pi∕mi ≪ 1 in the nonrelativistic limit. The coordinate-space potential then becomes

(8.47)

8.2.3 Interactions Mediated by Massless Spin-1 Bosons

For massless spin-1 bosons, the interaction Lagrangian is described as

$$\displaystyle \begin{aligned} \mathcal{L}_{\gamma'}=\frac{v_{h}}{\Lambda^{2}}P_{\mu\nu}\bar{\psi}\sigma^{\mu\nu}\left[\mathrm{Re}(C_{\psi})+i\gamma_{5}\mathrm{Im}(C_{\psi})\right]\psi~, \end{aligned} $$
(8.48)

where the Pμν is the field strength tensor of the massless gauge boson γ′, and \(\sigma ^{\mu \nu }=\frac {i}{2}[\gamma ^{\mu },\gamma ^{\nu }]\). Using anti-symmetric properties of the tensors, the Lagrangian can be written as

$$\displaystyle \begin{aligned} \mathcal{L}_{\gamma'}=2i\frac{v_{h}}{\Lambda^{2}}\partial_{\mu}A_{\nu}\bar{\psi}\gamma^{\mu}\gamma^{\nu}\left[\mathrm{Re}(C_{\psi})+i\gamma_{5}\mathrm{Im}(C_{\psi})\right]\psi~. \end{aligned} $$
(8.49)

Using ∂μ = iqμ for the operator, then

$$\displaystyle \begin{aligned} \mathcal{L}_{\gamma'}=-2\frac{v_{h}}{\Lambda^{2}}q_{\mu}A_{\nu}\bar{\psi}\gamma^{\mu}\gamma^{\nu}\left[\mathrm{Re}(C_{\psi})+i\gamma_{5}\mathrm{Im}(C_{\psi})\right]\psi~. \end{aligned} $$
(8.50)

The matrix elements \(\bar {u}(p_{f})\gamma ^{\mu }\gamma ^{\nu }u(p_{i})\) and \(\bar {u}(p_{f})\gamma ^{\mu }\gamma ^{\nu }\gamma _{5}u(p_{i})\) in the scattering matrix need to be estimated. In the nonrelativistic limit, the qμ can be approximated to only have a spacelike component. Therefore, the spinor products in the leading order become (± corresponds to the particle 1 and 2 cases, respectively):

$$\displaystyle \begin{aligned} q_{i}\bar{u}(p_{f})\gamma^{i}\gamma^{0}u(p_{i}) &= mq_{i} \begin{pmatrix} {\left(1+\frac{\boldsymbol{\sigma}\cdot\boldsymbol{p}}{2m}\right)}\xi^{\dagger}, & {\left(1-\frac{\boldsymbol{\sigma}\cdot\boldsymbol{p}}{2m}\right)}\xi^{\dagger} \end{pmatrix}\\&\quad \times \begin{pmatrix} 0&\sigma^{i}\\ -\sigma^{i}&0 \end{pmatrix} \begin{pmatrix} 0&1\\ 1&0 \end{pmatrix} \begin{pmatrix} \displaystyle {\left(1-\frac{\boldsymbol{\sigma}\cdot\boldsymbol{p}}{2m}\right)}\xi \\[0.3cm] \displaystyle {\left(1+\frac{\boldsymbol{\sigma}\cdot\boldsymbol{p}}{2m}\right)}\xi \end{pmatrix}\\ &=2i\boldsymbol{p}_{1,2}\cdot(\boldsymbol{q}\times\boldsymbol{\sigma})\pm(\boldsymbol{\sigma}\cdot\boldsymbol{q})^{2}, \end{aligned} $$
(8.51)

and

$$\displaystyle \begin{aligned} q_{i}\bar{u}(p_{f})\gamma^{i}\gamma^{j}u(p_{i})&=mq_{i}\begin{pmatrix} (\xi^{\dagger}, & \xi^{\dagger} \end{pmatrix} \begin{pmatrix} 0&\sigma^{i}\\ -\sigma^{i}&0 \end{pmatrix} \begin{pmatrix} 0&\sigma^{j}\\ -\sigma^{j}&0 \end{pmatrix} \begin{pmatrix} \xi\\ \xi \end{pmatrix}\\ &=-2m(-i(\boldsymbol{q}\times\boldsymbol{\sigma})_{j}). \end{aligned} $$
(8.52)

In the case of i ≠ j, the spinor products become

$$\displaystyle \begin{aligned} q_{i}\bar{u}(p_{f})\gamma^{i}\gamma^{0}\gamma_{5}u(p_{i})&=mq_{i}\begin{pmatrix} \xi^{\dagger}, & \xi^{\dagger} \end{pmatrix} \begin{pmatrix} 0&\sigma^{i}\\ -\sigma^{i}&0 \end{pmatrix} \begin{pmatrix} 0&1\\ 1&0 \end{pmatrix} \begin{pmatrix} -1&0\\ 0&1 \end{pmatrix} \begin{pmatrix} \xi\\ \xi \end{pmatrix}\\ &=-2m(\boldsymbol{\sigma}\cdot\boldsymbol{q}), \end{aligned} $$
(8.53)

and

$$\displaystyle \begin{aligned} q_{i}\bar{u}(p_{f})\gamma^{i}\gamma^{j}\gamma_{5}u(p_{i})&=mq_{i}\begin{pmatrix} (1+\frac{\boldsymbol{\sigma}\cdot\boldsymbol{p}}{2m})\xi^{\dagger}, & (1-\frac{\boldsymbol{\sigma}\cdot\boldsymbol{p}}{2m})\xi^{\dagger} \end{pmatrix}\\ &\quad \times \begin{pmatrix} -\sigma^{i}\sigma^{j}&0\\ 0&-\sigma^{i}\sigma^{j} \end{pmatrix} \begin{pmatrix} -1&0\\ 0&1 \end{pmatrix} \begin{pmatrix} \displaystyle {\left(1-\frac{\boldsymbol{\sigma}\cdot\boldsymbol{p}}{2m}\right)}\xi\\[0.3cm] \displaystyle {\left(1+\frac{\boldsymbol{\sigma}\cdot\boldsymbol{p}}{2m}\right)}\xi \end{pmatrix}\\ &=-2(\boldsymbol{q}\times(\boldsymbol{p}\times\boldsymbol{\sigma}))_{j}~. \end{aligned} $$
(8.54)

There are three different types of possible interactions mediated by massless spin-1 bosons: tensor-tensor, pseudotensor-tensor, and pseudotensor-pseudotensor. Details of concerning these interactions can be found in Ref. [24].

8.2.3.1 Tensor-Tensor Interaction

For the tensor-tensor interaction, the scattering matrix element is

$$\displaystyle \begin{aligned} iM(q)&=\frac{4v^{2}_{h}\mathrm{Re}(C_{1})\mathrm{Re}(C_{2})}{\Lambda^{4}}[iq_{i}\bar{u}(p_{f,1})\gamma^{i}\gamma^{j}u(p_{i,1})]\\&\quad \times[iq_{l}\bar{u}(p_{f,2})\gamma^{l}\gamma^{m}u(p_{i,2})]\frac{-ig^{jm}}{q^{2}}. \end{aligned} $$
(8.55)

In the nonrelativistic limit, the scattering matrix becomes

$$\displaystyle \begin{aligned} iM(q)=\frac{4v^{2}_{h}\mathrm{Re}(C_{1})\mathrm{Re}(C_{2})}{\Lambda^{4}}[-2m_{1}(\boldsymbol{q}\times\boldsymbol{\sigma}_{1})]_{j}[-2m_{2}(\boldsymbol{q}\times\boldsymbol{\sigma}_{2})]_{m}\frac{ig^{jm}}{|\boldsymbol{q}|{}^{2}}~. \end{aligned} $$
(8.56)

Then the momentum-space potential is given by

$$\displaystyle \begin{aligned} V(q)=\frac{4v_{h}^{2}\mathrm{Re}(C_{1})\mathrm{Re}(C_{2})}{\Lambda^{4}}\frac{e^{i\boldsymbol{q}\cdot\boldsymbol{r}}}{|\boldsymbol{q}|{}^{2}}(-(\boldsymbol{q}\times\boldsymbol{\sigma}_{1})\cdot(\boldsymbol{q}\times\boldsymbol{\sigma}_{2}))~. \end{aligned} $$
(8.57)

After the Fourier transform, the coordinate-space potential becomes

$$\displaystyle \begin{aligned} V_{TT}(r)=\frac{4v_{h}^{2}\mathrm{Re}(C_{1})\mathrm{Re}(C_{2})}{4\pi\Lambda^{4}}\left[(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{\sigma}_{2})\left(\frac{1}{r^{3}}-\frac{8\pi}{3}\delta(\boldsymbol{r})\right)-(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}})(\boldsymbol{\sigma}_{2}\cdot\hat{\boldsymbol{r}})\frac{3}{r^{3}}\right]~. \end{aligned} $$
(8.58)

8.2.3.2 Pseudotensor-Pseudotensor Interaction

For the pseudotensor-pseudotensor interaction, the leading order contribution comes from the timelike components. The corresponding scattering matrix is

$$\displaystyle \begin{aligned} iM(q)&=\frac{4v^{2}_{h}\mathrm{Im}(C_{1})\mathrm{Im}(C_{2})}{\Lambda^{4}}[i^{2}q_{i}\bar{u}(p_{f,1})\gamma^{i}\gamma^{0}\gamma_5u(p_{i,1})]\\&\quad \times[i^{2}q_{l}\bar{u}(p_{f,2})\gamma^{l}\gamma^{0}\gamma_5u(p_{i,2})]\frac{-ig^{00}}{q^{2}}~, \end{aligned} $$
(8.59)

In the nonrelativistic limit, it becomes

$$\displaystyle \begin{aligned} iM(q)=\frac{4v^{2}_{h}\mathrm{Im}(C_{1})\mathrm{Im}(C_{2})}{\Lambda^{4}}[i^{2}(-2m_{1})(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{q})][i^{2}(-2m_{2})(\boldsymbol{\sigma}_{2}\cdot\boldsymbol{q})]\frac{ig^{00}}{|\boldsymbol{q}|{}^{2}}~. \end{aligned} $$
(8.60)

Then the momentum-space potential is given by

$$\displaystyle \begin{aligned} V(q)=\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Im}(C_{2})}{\Lambda^{4}}\frac{e^{i\boldsymbol{q}\cdot\boldsymbol{r}}}{|\boldsymbol{q}|{}^{2}}(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{1})(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{2})~, \end{aligned} $$
(8.61)

yielding the coordinate-space potential:

$$\displaystyle \begin{aligned} V_{\tilde{T}\tilde{T}}(r)=\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Im}(C_{2})}{4\pi\Lambda^{4}}\left[(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{\sigma}_{2})\left(\frac{1}{r^{3}}+\frac{4\pi}{3}\delta(\boldsymbol{r})\right)-(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}})(\boldsymbol{\sigma}_{2}\cdot\hat{\boldsymbol{r}})\frac{3}{r^{3}}\right]. \end{aligned} $$
(8.62)

8.2.3.3 Pseudotensor-Tensor Interaction

Unlike the tensor-tensor or pseudotensor-pseudotensor interaction, the leading order of the fermion current for the pseudotensor-tensor interaction depends on the fermion momenta. In the following calculation, we assume that the first vertex involves the pseudotensor coupling and the second vertex involves the tensor coupling. The pseudotensor term appears in leading order with the timelike component of the gamma matrix, while the tensor term appears in leading order with the spacelike component of the gamma matrix and is thus associated with momentum. Therefore, it is necessary to evaluate both the timelike and spacelike components in order to estimate the scattering amplitude.

Timelike Component:

The timelike component of the scattering matrix is given by

$$\displaystyle \begin{aligned} M(q)_{00}&=-i\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})}{\Lambda^{4}}[i^2q_{i}\bar{u}(p_{f,1})\gamma^{i}\gamma^{0}\gamma_{5}u(p_{i,1})]\\&\quad \times[iq_{i}\bar{u}(p_{f,2})\gamma^{i}\gamma^{0}u(p_{i,2})]\frac{ig_{00}}{|q|{}^{2}} \end{aligned} $$
(8.63)

In the nonrelativistic limit, it becomes

$$\displaystyle \begin{aligned} M(q)_{00}\rightarrow -i\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})}{\Lambda^{4}}\frac{[-2m_{1}(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{q})][2i\boldsymbol{p}_{2}\cdot(\boldsymbol{q}\times\boldsymbol{\sigma}_{2})-(\boldsymbol{\sigma}_{2}\cdot\boldsymbol{q})^{2}]}{|q|{}^{2}}. {} \end{aligned} $$
(8.64)

Let us evaluate the above expression term by term. Note that the following calculations employ the path integral formalism, making use of the integration measure \(\int \mathcal {D}q\) to carry out the functional integral over all possible trajectories (see, for example, discussion in Refs. [26, 27]). First,

$$\displaystyle \begin{aligned} &\int \mathcal{D}q e^{iqr}\frac{1}{|q|{}^{2}}(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{q})\boldsymbol{p}_{2}\cdot(\boldsymbol{q}\times\boldsymbol{\sigma}_{2})=\sum_{i,l}p_{2}^{i}\epsilon^{ijk}\sigma_{2,k}\sigma_{1,l}\int \mathcal{D}q e^{iqr}\frac{q_{j}q_{l}}{|q|{}^{2}}~,\\ &=\frac{1}{4\pi}\sum_{i,l}p_{2}^{i}\epsilon^{ijk}\sigma_{2,k}\sigma_{1,l}\left[\frac{\delta_{jl}}{r^{3}}-\frac{3r_{j}r_{l}}{r^{5}}+\frac{4\pi}{3}\delta_{jl}\delta(\boldsymbol{r})\right]~,\\ &=\frac{1}{4\pi}\left[\frac{\boldsymbol{\sigma}_{1}\cdot(\boldsymbol{\sigma}_{2}\times \boldsymbol{p}_{2})}{r^{3}}-3\frac{\boldsymbol{p}_{2}\cdot(\hat{\boldsymbol{r}}\times\boldsymbol{\sigma}_{2})(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}})}{r^{3}}+\frac{4\pi}{3}\boldsymbol{\sigma}_{1}\cdot(\boldsymbol{\sigma}_{2}\times \boldsymbol{p}_{2})\delta(\boldsymbol{r})\right]~,\\ &=\frac{1}{4\pi}\left[\boldsymbol{p}_{2}\cdot(\boldsymbol{\sigma}_{1}\times\boldsymbol{\sigma}_{2})\left(\frac{1}{r^{3}}+\frac{4\pi}{3}\delta(\boldsymbol{r})\right)+3\frac{\boldsymbol{p}_{2}\cdot(\boldsymbol{\sigma}_{2}\times\hat{\boldsymbol{r}})(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}})}{r^{3}}\right]~. \end{aligned} $$
(8.65)

Second,

$$\displaystyle \begin{aligned} &\int \mathcal{D}q e^{iqr}\frac{1}{|q|{}^{2}}(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{q})(\boldsymbol{\sigma}_{2}\cdot\boldsymbol{q})^{2}=\int \mathcal{D}q e^{iqr}\frac{1}{|q|{}^{2}}|q|{}^{2}(\boldsymbol{\sigma}_{1}\cdot\boldsymbol{q})\\ &=-i\boldsymbol{\sigma}_1\cdot\nabla\delta(\boldsymbol{r}). \end{aligned} $$
(8.66)

Inserting the expressions (8.65) and (8.66) into Eq. (8.64), we obtain

$$\displaystyle \begin{aligned} M(r)_{00}=&-\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})m_1}{\pi\Lambda^{4}}\boldsymbol{p}_{2}\cdot(\boldsymbol{\sigma}_{1}\times\boldsymbol{\sigma}_{2})\left(\frac{1}{r^{3}}+\frac{4\pi}{3}\delta(\boldsymbol{r})\right)\\ &-\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})m_1}{\pi\Lambda^{4}}\frac{3\boldsymbol{p}_{2}\cdot(\boldsymbol{\sigma}_{2}\times\hat{\boldsymbol{r}})(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}})}{r^{3}}\\ &-\frac{8v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})m_1}{\Lambda^{4}}\boldsymbol{\sigma}_1\cdot\nabla\delta(\boldsymbol{r})~. \end{aligned} $$
(8.67)

This timelike component of the pseudotensor-tensor potential can then be written as

$$\displaystyle \begin{aligned} V_{00}(r)=&\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})}{8\pi\Lambda^{4}}(\boldsymbol{\sigma}_{1}\times\boldsymbol{\sigma}_{2})\left\{-\frac{\boldsymbol{p}_{2}}{m_{2}},\left(\frac{1}{r^{3}}+\frac{4\pi}{3}\delta(\boldsymbol{r})\right)\right\}\\ &+\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})}{8\pi\Lambda^{4}}\left\{-\frac{\boldsymbol{p}_{2,i}}{m_{2}},\frac{3(\boldsymbol{\sigma}_{2}\times\hat{\boldsymbol{r}})_{i}(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}})}{r^{3}}\right\}\\ &-\frac{2v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})}{\Lambda^{4}}\frac{\boldsymbol{\sigma}_1\cdot\nabla\delta(\boldsymbol{r})}{m_{2}}. \end{aligned} $$
(8.68)

Spacelike Component:

The spacelike component of the scattering matrix is

$$\displaystyle \begin{aligned} M(q)_{lm}&=-i\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})}{\Lambda^{4}}\\&\quad \times[i^2q_{i}\bar{u}(p_{f,1})\gamma^{i}\gamma^{l}\gamma_{5}u(p_{i,1})][iq_{i}\bar{u}(p_{f,2})\gamma^{i}\gamma^{m}u(p_{i,2})]\frac{ig_{lm}}{|q|{}^{2}}~. \end{aligned} $$

In the nonrelativistic limit

$$\displaystyle \begin{aligned} M(q)_{jj}\rightarrow &-i\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})}{\Lambda^{4}}\frac{[2m_{2}i(\boldsymbol{q}\times\boldsymbol{\sigma}_{2})_{j}][-2(\boldsymbol{q}\times(\boldsymbol{p}_{1}\times\boldsymbol{\sigma}_{1}))_{j}]}{|q|{}^{2}}\\ &=-\frac{16v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})m_2}{\Lambda^{4}}\frac{[(\boldsymbol{q}\times\boldsymbol{\sigma}_{2})_{j}][(\boldsymbol{q}\times(\boldsymbol{p}_{1}\times\boldsymbol{\sigma}_{1}))_{j}]}{|q|{}^{2}}~. \end{aligned} $$
(8.69)

Summing over the j index gives the inner product of the two vectors and yields

$$\displaystyle \begin{aligned} (\boldsymbol{q}\times\boldsymbol{\sigma}_{2})\cdot(\boldsymbol{q}\times(\boldsymbol{p}_{1}\times\boldsymbol{\sigma}_{1}))=(\boldsymbol{q}\cdot\boldsymbol{\sigma}_{1})\boldsymbol{q}\cdot(\boldsymbol{\sigma}_{2}\times\boldsymbol{p}_{1})-(\boldsymbol{q}\cdot\boldsymbol{p}_{1})(\boldsymbol{q}\cdot(\boldsymbol{\sigma}_{2}\times\boldsymbol{\sigma}_{1}))~. {} \end{aligned} $$
(8.70)

Taking the Fourier transform with respect to the three-momentum q, the first term gives

$$\displaystyle \begin{aligned} \boldsymbol{p}_{1}\cdot(\boldsymbol{\sigma}_{1}\times\boldsymbol{\sigma}_{2})\left(\frac{1}{r^{3}}-\frac{4\pi}{3}\delta(\boldsymbol{r})\right)+\frac{3(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}})\boldsymbol{p}_{1}\cdot(\boldsymbol{\sigma}_{2}\times\hat{\boldsymbol{r}})}{r^{3}}~. \end{aligned} $$
(8.71)

From the conservation of energy-momentum, q ⋅p = 0, the second term in Eq. (8.70) vanishes. Thus combining the timelike and spacelike components together, the potential for the pseudotensor-tensor interaction is given by

$$\displaystyle \begin{aligned} V_{\tilde{T}T}(r)=&\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})}{8\pi\Lambda^{4}}(\boldsymbol{\sigma}_{1}\times\boldsymbol{\sigma}_{2})\left\{\frac{\boldsymbol{p}_{1}}{m_{1}}-\frac{\boldsymbol{p}_{2}}{m_{2}},\left(\frac{1}{r^{3}}+\frac{4\pi}{3}\delta(\boldsymbol{r})\right)\right\}\\ &+\frac{4v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})}{8\pi\Lambda^{4}}\left\{\frac{\boldsymbol{p}_{1}}{m_{1}}-\frac{\boldsymbol{p}_{2,i}}{m_{2}},\frac{3(\boldsymbol{\sigma}_{2}\times\hat{\boldsymbol{r}})_{i}(\boldsymbol{\sigma}_{1}\cdot\hat{\boldsymbol{r}})}{r^{3}}\right\}\\ &-\frac{2v_{h}^{2}\mathrm{Im}(C_{1})\mathrm{Re}(C_{2})}{\Lambda^{4}}\frac{\boldsymbol{\sigma}_1\cdot\nabla\delta(\boldsymbol{r})}{m_{2}}. \end{aligned} $$
(8.72)

8.3 Searches for New Interactions Between Polarized Electrons and Unpolarized Nucleons

Several experiments have searched for the monopole-dipole interaction between polarized electrons and unpolarized nucleons mediated by axions or ALPs, described by the monopole-dipole interaction potential [Eq. (8.21)]

$$\displaystyle \begin{aligned} V_{\mathrm{sp}}(\boldsymbol{r})=\frac{\hbar^{2}g_{s}^{\mathrm{N}}g_{p}^{\mathrm{e}}}{8\pi m_{e}}\left( {\frac{1}{\lambda r} + \frac{1}{r^{2}} }\right) e^{-r/\lambda} \boldsymbol{\sigma}\cdot \hat{\boldsymbol{r}}~, {} \end{aligned} $$
(8.73)

where r is the displacement vector between electron and nucleon, \(g_{s}^{\mathrm {N}}\) and \(g_{p}^{\mathrm {e}}\) are the scalar and pseudoscalar coupling constants of axions to the nucleon and to the electron, respectively, me is mass of electron, λ is the axion Compton wavelength, and σ is the spin unit vector. For example, Ni et al. tried to measure an induced magnetization in a paramagnetic salt located near a heavy copper mass [28]. Hammond et al. [29] observed the motion of three copper cylinders located between a source of polarized electrons consisting of two split toroidal electromagnets inside a magnetic shield system that enabled high spin polarization with negligible external magnetic field [30]. Youdin et al. searched for monopole-dipole couplings between a nearby lead mass and the spins of 133Cs and 199Hg atoms using co-located atomic magnetometers [31]. Torsion balances have been a successful method used for searches at the millimeter scale [32] as well as at Earth and solar system scales [33]. In this section we describe the working principles of three example experiments which have produced recent world-leading constraints: a torsion pendulum experiment, an electron-spin-resonance experiment, and a spectroscopy experiment using trapped ions [34].

8.3.1 Torsion Pendulum Experiments

The most stringent experimental constraint at distance scales below ≈1 mm on an axion-mediated interaction has been made with a torsion pendulum experiment by Hoedl et al. [32], which is a characteristic example of the sort of experimental method used to search for exotic interactions mediated by new bosons. The torsion pendulum apparatus consists of two parts: a split toroidal electromagnet and a planar torsion pendulum suspended between two magnet halves. The magnet halves are fixed in the apparatus (see Fig. 8.2). The pendulum is free to twist about the axis of the torsion fiber. The twist angle of the pendulum is optically monitored with an autocollimator [32]. The autocollimator measures small angles by comparing the position of a collimated laser beam that is reflected from the surface of the pendulum to the position of a reference laser beam. An axion-mediated force between the polarized electrons in the electromagnet and the unpolarized silicon atoms in the pendulum would generate a torque on the pendulum which is given by \(g_{s}^{N}g_{p}^{e} G(x, \lambda _{\mathrm {a}})\), where G(x, λa) is a geometrical factor and x is the distance between the pendulum and the symmetry plane between the magnet halves. This interaction acts like an effective “magnetic field” (pseudo-magnetic field) to generate torque on the pendulum, thereby behaving as a torsion spring. When the effective magnetic field is switched from one direction to the other direction by changing the polarization of the electron spins in the electromagnet, if there is a new macroscopic interaction in the form of Eq. (8.73), the pendulum experiences a torque. Thus a spin-dependent interaction could be detected by measuring the change in the equilibrium twist angle of the torsion pendulum. The strength of the interaction depends on the distance between pole and pendulum. Figure 8.3 shows the exclusion limit based on this torsion balance experiment (red dashed line labeled Hoedl et al. [32]), as well as constraints from other experiments [35].

Fig. 8.2
figure 2

Schematic diagram of a torsion pendulum experiment designed to search for a monopole-dipole coupling between electron spins and nucleons. Two toroidal electromagnet halves source polarized electrons. A planar torsion pendulum suspended between the two magnet halves sources unpolarized nucleons. The pendulum is freely suspended by a tungsten wire. Adapted from Ref. [32]

Full size image
Fig. 8.3
figure 3

Constraints on monopole-dipole couplings between nucleons and electrons \(|g_s^N g_p^e|/(\hbar c)\) from laboratory experiments and astrophysical observations, adapted and updated from Ref. [35]. Constraints from experiments discussed in this chapter include those from Ref. [32], shown by the red dashed line labeled Hoedl et al. [32], Ref. [36], shown by the red dotted line labeled Crescini et al. (2017) [36], Ref. [34], shown by the black short-dashed line labeled Wineland et al. [19], and Ref. [37], shown by the dashed blue line labeled Lee et al. [37]. Further discussion of other constraints can be found in Ref. [35]

Full size image

Although \(g_{s}^{N}g_{p}^{e}\) would be very small for QCD axions due to the fact that \(g_{s}^{N} \propto \theta {{ }_{\mbox{ QCD}}}\), where θQCD is the CP-violating phase appearing in the Lagrangian describing the strong interaction (see discussion in Chap. 2), this experiment has the advantage compared to axion haloscope searches (as discussed in, for example, Chaps. 4 and 6) that axions are sourced directly from the local object. Thus the effect would exist even if the axion is not the dominant component of dark matter, and means that the signal is not subject to the myriad uncertainties affecting the interpretation of limits from haloscope experiments due to the unknown local distribution of dark matter (see, for example, discussion in Chaps. 3 and 10). Because the axion-mediated interaction is locally sourced in this experiment, and, in fact, all the experiments considered in this chapter, the axion-induced signal can be purposefully modulated in a controlled fashion, making it potentially easier to distinguish from noise.

8.3.2 Electron-Spin Based Magnetometer Searches

Recent magnetometry experiments have provided the best constraints on monopole-dipole couplings of electron spins at distances of order 1–10 cm [36]. The QUAX-gsgp experiment described in Ref. [36] is an adaptation of the QUAX experiment (QUest for AXions) to search for monopole-dipole interactions between an unpolarized source mass and the electron spins in a paramagnetic gadolinium oxy-orthosilicate Gd2SiO5 crystal (GSO) crystal. The GSO crystal is cooled down to 4K in a liquid helium cryostat. Figure 8.4 shows the diagram of the setup; the derived constraints on electron-spin couplings are shown in Fig. 8.3 with the red dotted line labeled as Crescini et al. (2017) [36]. An unpolarized lead source mass is spun a few cm away from the GSO crystal and the change in magnetization is read out with a dc superconducting quantum interference device (SQUID) magnetometer. The setup includes concentric superconducting shields placed within an outermost μ-metal shield in order to reduce the flux trapped in the inner superconducting shields. The overall rejection factor of magnetic shield system is expected to be ∼1012, significantly reducing environmental magnetic disturbances. The distance between the center of mass of each lead source and the GSO crystal is modulated in time by mounting the masses on a rotating 70 cm diameter aluminum disk that rotates at a constant angular velocity. The minimum distance between each source and detector is 3.7 cm.

Fig. 8.4
figure 4

Schematic diagram of the setup for the short-range spin-dependent force search of the QUAX-gsgp experiment. An unpolarized lead source mass wheel is spun in proximity to a paramagnetic GSO crystal, and the resulting change in its polarization is read out with a SQUID magnetometer. Figure from Ref. [36]

Full size image

8.3.3 Spectroscopic Constraints with Trapped Ions

Constraints on novel electron monopole-dipole interactions have also been obtained using hyperfine spectroscopy of trapped and cooled 9Be+ ions in experiments performed by Wineland et al. [34]. Here by reversing the magnetic field along the direction of the Earth’s gravitational field, novel spin-dependent frequency shifts can be constrained. Here the source of the interaction was assumed to be nucleons in the Earth and the resulting frequency shift between two Zeeman sublevels within the electronic ground state hyperfine manifold was measured. In the absence of novel spin-dependent interactions, the field reversal should result in no frequency shift. The frequency shift was determined to be less than ∼13.4 μHz, resulting in the limits shown by the black short-dashed line in Fig. 8.3.

8.4 Monopole-Dipole Searches with Polarized Nuclear Spins and Unpolarized Nucleons

Several experimental techniques have been employed to search for novel spin-dependent monopole-dipole interactions Vsp between nucleons. Some experiments have used ultra-cold neutrons (UCNs) and 3He to test spin-dependent interactions between polarized and unpolarized nucleons. Baessler et al. tried to find a deviation from the expected energy levels of UCNs in the Earth’s gravitational field [38]. Serebrov et al. searched for a change in the UCN precession frequency due to a spin-dependent interaction [39]. Pethkhov et al. looked for a change in the spin relaxation time of 3He induced by exotic spin-dependent interactions [40]. Fu et al. also set a constraint on the monopole-dipole interaction [41] by reanalyzing existing data on the spin relaxation times of polarized 3He in the context of exotic spin-dependent interactions [42]. Here we describe in more detail a few examples based on magnetometry and nuclear magnetic resonance (NMR), including experiments under development.

8.4.1 Axion Searches with Comagnetometers

A powerful technique to search for axion-mediated interactions is to measure variations in the nuclear Larmor precession frequency as a source mass is brought near a polarized atomic vapor. There have been many successful approaches, for example, relying on comagnetometry with multiple nuclear spin species [43,44,45,46], nuclear spins along with alkali gases [37], and single-species liquid comagnetometers based on NMR measurements of different nuclei in identical molecules [47].

8.4.1.1 Noble Gas Comagnetometer

A method to search for non-magnetic, spin-dependent interactions is to use a sensitive low-field comagnetometer based on detection of free spin precession of gaseous, nuclear polarized samples [45]. The idea is to measure spin precession of two species, 3He and 129Xe gas, in the same volume. The Larmor frequencies of 3He and 129Xe in a guiding magnetic field B are given by ωL,He(Xe) = γHe(Xe)B, with γHe(Xe) being the gyromagnetic ratios of the respective gas species with γHe∕γXe = 2.75408159. The goal of employing a comagnetometer is to separate out background magnetic fields and drifts from any anomalous spin-dependent interactions. One seeks to establish a signal which will vanish for ordinary magnetic fields but be sensitive to new physics. In practice perfect subtraction of ordinary backgrounds is challenging for several reasons, but the technique is quite powerful and has produced constraints on monopole-dipole interactions between nuclei at a variety of laboratory scale distances.

The influence of the ambient magnetic field and its temporal fluctuations cancels in the difference of measured Larmor frequencies of the co-located spin samples

$$\displaystyle \begin{aligned} \Delta\omega=\omega_{\mathrm{He}}-\frac{\gamma_{\mathrm{He}} }{\gamma_{\mathrm{Xe}} } \omega_{\mathrm{Xe}}~. {} \end{aligned} $$
(8.74)

This frequency shift in Eq. (8.74) can be separated into three parts [45]:

$$\displaystyle \begin{aligned} \Delta\omega(t)=\Delta\omega_{\mathrm{lin}}+\epsilon_{\mathrm{He}}A_{\mathrm{He}}e^{-t/T^{*}_{2, \mathrm{He}}}-\epsilon_{\mathrm{Xe}}A_{\mathrm{Xe}}e^{-t/T^{*}_{2, \mathrm{Xe}}}~, {} \end{aligned} $$
(8.75)

where 𝜖He(Xe) are the respective geometry-dependent factors describing self-field effects, \(T^{*}_{2, \mathrm {He(Xe)}}\) are the respective effective spin coherence relaxation times, and AHe(Xe) are the constants describing the amplitude of the spin polarization of the respective species. The first part is a constant frequency shift, Δωlin, due to Earth’s rotation which is not compensated by comagnetometry. This effect is commonly referred to as the gyro-compass effect and is nicely demonstrated and well-described in Ref. [48]. The other two parts are related to the generalized Ramsey-Bloch-Siegert shift [49,50,51] arising from the self-fields caused by the precessing 3He/129Xe nuclear spins appearing in nonspherical vapor cells [52]. In the same manner, the weighted accumulated phase difference acquired during precession, Δ Φ(t) = ΦHe(t) − (γHe∕γXe) ΦXe, can also be measured. Any anomalous frequency shift generated by non-magnetic spin interactions, such as the monopole-dipole interaction described in Eq. (8.21), could be analyzed by monitoring Δω(t) and Δ Φ(t), respectively (Fig. 8.5).

Fig. 8.5
figure 5

Schematic diagram of the experimental setup from Ref. [45] used to search for anomalous monopole-dipole interactions. The 3He/129Xe cell is located at the center. The BGO crystal is placed on right (or left) side with respect to the sample. The crystal travels back and forth toward the cell with a certain frequency. The SQUID module located on the top of the cell monitors the precession frequency of each species. Adapted from Ref. [45]

Full size image

The experiment was done inside the magnetically shielded room (MSR) at the Physikalisch-Technische Bundesanstalt Berlin (PTB). A homogeneous magnetic guide field of ∼350nT was provided in the MSR. The detection of spin precession was done with multi-channel low Tc dc SQUID device. The SQUID sensor detects a sinusoidal magnetic flux change due to the nuclear spin precession of the gas. The spin precession frequency shift due to any monopole-dipole interaction is induced by an unpolarized mass with high nucleon density. In this experiment, a cylindrical BGO crystal (Bi4Ge3O12), which is non-conductive and non-magnetic (χmag ≈ 0), with nucleon density (ρ = 7.13 g∕cm3) was used. The BGO crystal source mass is alternately moved to the left and right side of the 3He∕Xe cell. If a sufficiently strong monopole-dipole interaction exists, the movement of the BGO crystal would produce a frequency shift correlated with the motion of the source mass. In the case of a non-zero spin-dependent interaction, a shift \(\Delta \omega ^{w}_{\mathrm {sp}}\) in the weighted frequency difference described by Eq. (8.74) can be extracted from respective frequency measurements in the “close” and “distant” BGO positions given by

$$\displaystyle \begin{aligned} \Delta \omega^{w}_{\mathrm{sp}}=\frac{2V^{c}_{\Sigma}}{\hbar}\left( { 1-\frac{\gamma_{\mathrm{He}}}{\gamma_{\mathrm{Xe}}}} \right)~. {} \end{aligned} $$
(8.76)

Here the average potential in the “close” BGO position, \(V^c_{\Sigma }\), is obtained by integration of the monopole-dipole interaction potential Vsp over the volume of the massive unpolarized sample averaged over the volume of the polarized spin sample, each having a cylindrical shape [45]. The estimation of \(V^c_{\Sigma }\) assumes that the He and Xe nuclei can be described by the single-particle Schmidt model, see, for example, discussion in Ref. [53]. The constraints derived from this experiment are shown by the black dotted line labeled Tullney et al. [45] in Fig. 8.6.

Fig. 8.6
figure 6

Constraints on monopole-dipole couplings between nucleons and neutrons \(|g_s^N g_p^n|/(\hbar c)\) from laboratory experiments and astrophysical observations, adapted and updated from Ref. [35]. Constraints from experiments discussed in this chapter include those from Ref. [45], shown by the black dotted line labeled Tullney et al. [45], Ref. [46], shown by the red dashed line labeled Bulatowicz et al. [46], Ref. [37], shown by the dashed blue line labeled Lee et al. [37], and Ref. [54], shown by the long-dashed red line labeled Chu et al. [54]. Further discussion of other constraints can be found in Ref. [35]

Full size image

A different experiment was performed with a dual-species comagnetometer employing 129Xe and 131Xe and using Rb as an optical magnetometer for readout of the nuclear spin precession [46]. The Rb atoms are optically spin polarized and, through spin-exchange collisions, polarize the Xe nuclei parallel to a dc magnetic field. The Rb atoms serve as a magnetometer that detects the Xe precession since the transverse magnetic fields of the polarized Xe produce an oscillating transverse spin polarization of the Rb atoms. This is detected optically as a rotation of the polarization of a linearly polarized sense laser [46]. The setup is shown in Fig. 8.7 and limits on scalar-pseudoscalar interactions are derived in the distance range of approximately 1 mm. NMR frequency shifts in polarized 129Xe and 131Xe could arise due to a monopole-dipole interaction when a zirconia rod was moved back and forth near the NMR cell. By comparing the simultaneous frequencies of the two Xe isotopes, magnetic field changes are distinguished from frequency shifts due to the monopole-dipole coupling using the same principle of comagnetometry discussed in the previous paragraphs. Using prior calculations of the neutron spin contribution to the nuclear angular momentum in 129Xe and 131Xe, a new upper bound on the product \(g_s^Ng^n_p\) for was obtained for ranges at the millimeter scale as shown by the red dashed line labeled Bulatowicz et al. [46] in Fig. 8.6.

Fig. 8.7
figure 7

The setup for the dual-species free-induction decay (FID) comagnetometer used to search for mm-scale monopole-dipole interactions. Sample FID data, where Xe spin precession was optically detected via laser light probing Rb spins co-located with the Xe spins, are shown (FID oscillations are frequency down-converted to ≈0.3 Hz from 45 Hz or 152 Hz). The unpolarized source is the movable zirconia rod, which can induce frequency shifts of the Xe spin precession if a monopole-dipole interaction of the form given by Eq. (8.21) exists. Figure from Ref. [46]

Full size image

8.4.1.2 Noble Gas: Alkali Comagnetometer Searches

Searches for new spin-dependent interactions have also been performed with K-3He comagnetometers [37, 55]. Recent work was done with a moveable unpolarized Pb source mass at a distance of approximately 15 cm from the K-3He comagnetometer in order to search for both electron- and nuclear-spin-coupled interactions that arise when there are both pseudoscalar and scalar couplings [37]. The experiment employed overlapping ensembles of spin-polarized K and 3He, which are strongly coupled via Fermi-contact interactions during spin-exchange collisions when the resonant frequencies of K and 3He are matched. This allows effective cancellation of magnetic fields and fast transient response [37]. In particular, the cancellation is for fields transverse to the external field due to the adiabatic following of the 3He spins. (The system is insensitive to fields along the applied external field, because those do not create torques on the spins initially aligned along the leading field.)

Rubidium is used for spin-exchange optical pumping (SEOP), which polarizes the K by collisions, and Rb and K-3He spin-exchange collisions polarize 3He to approximately 2%. The comagnetometer signal is proportional to the difference of the anomalous magnetic-like field couplings to the nuclear spin in 3He and the electron spin in K. The He-3 adiabatically follows the magnetic field and the leading field is tuned so that its effect on the K spins exactly balances that of the He-3 magnetization, making the comagnetometer insensitive to regular magnetic fields to first order. The rotation rate of the apparatus about the y axis represents an example of a non-magnetic coupling to spin that does not cancel in the comagnetometer. A challenge in this experiment was that the motion of the Pb source masses produced a subtle mechanical effect due to temperature changes correlated with the positions of the masses [37]. Constraints derived from this experiment are shown in both Figs. 8.3 and 8.6 by the dashed blue line labeled Lee et al. [37].

8.4.2 NMR-Based Spin-Dependent Searches

A sub-mm-range search was performed using room temperature polarized 3He gas in a cell with a 250 μm thick window and unpolarized source mass [54]. The experimental diagram is shown in Fig. 8.8. The cylindrical 3He cell is located in a uniform magnetic field. Correction coils compensate for residual leading field gradients. 3He is polarized using spin-exchange optical pumping in the spherical pumping chamber, and polarized 3He atoms diffuse into the lower 40-cm long cylindrical chamber, which has two hemispherical glass windows at both ends. Two pick-up coils are used: pick-up coil A is mounted below the window to measure the precession frequency shift of the polarized 3He nuclei due to spin-dependent short-range interactions with the unpolarized mass. Pick-up coil B is positioned farther away to be insensitive to short-range interactions, and its signal is used to monitor the leading field drift and background fields. The frequencies measured in both coils are subtracted for each measurement. The 3He cell position is adjusted to optimize the transverse spin relaxation time measured from coils A and B. The leading field is tuned to produce a 3He Larmor frequency near 23.8 kHz and the authors apply a 24 kHz radiofrequency (RF) pulse to tip the spins by a small angle with negligible polarization loss. The precessing polarized 3He nuclei induce an electromotive forces (EMF) in the pick-up coils which is recorded. Two source masses for the experiment are used having differing nucleon densities and low magnetic impurities: a ceramic mass block and a liquid mixture of 1.02% MnCl2 in pure water. Constraints derived from this experiment are shown in Fig. 8.6 by the long-dashed red line labeled Chu et al. [54].

Fig. 8.8
figure 8

Experimental setup for the NMR measurement with a 3He sample cell, polarizing cell (spherical), polarizing laser, Helmholtz coils, and source mass. Precession of the polarized 3He nuclei is measured by the induced EMF in the pick-up coils. Figure from Ref. [54]

Full size image

8.4.3 Resonant NMR-Based Spin-Dependent Interaction Search: ARIADNE

The Axion Resonant InterAction DetectioN Experiment (ARIADNE) aims to detect axion-mediated spin-dependent interactions between an unpolarized source mass and a spin-polarized 3He low-temperature gas [56]. As previously noted in the discussion around Eq. (8.21), the axion can mediate an interaction between fermions (e.g., nucleons) with a potential given by

$$\displaystyle \begin{aligned} V_{sp}(r)=\frac{\hbar^2 g_s^N g_p^N}{8 \pi m_f}\left( \frac{1}{r \lambda_a}+\frac{1}{r^2}\right) e^{-\frac{r}{\lambda_a}} \left(\boldsymbol{\sigma} \cdot \hat{\boldsymbol{r}} \right), {} \end{aligned} $$
(8.77)

where mf is their mass, σ is the Pauli spin matrix, r is the vector between them, and λa = h∕(mac) is the axion Compton wavelength. For the QCD axion the scalar and dipole coupling constants \(g_s^N\) and \(g_p^N\) are related to the axion mass. Since the axion couples to σ, which is proportional to the nuclear magnetic moment, the axion coupling can be treated as an effective “magnetic field” Beff (i.e., a pseudo-magnetic field). This effective field is used to resonantly drive spin precession in a laser-polarized cold 3He gas. This is accomplished by spinning an unpolarized tungsten mass sprocket near the 3He vessel. As the teeth of the sprocket pass by the sample at the nuclear Larmor precession frequency, the magnetization in the longitudinally polarized He gas begins to precess about the axis of an applied field. This precessing transverse magnetization is detected with a SQUID. The 3He sample acts as an amplifier to transduce the small effective magnetic field into a larger real magnetic field detectable by the SQUID.

Integrating over the source mass, via Eq. (8.77), an axion with λa < R will generate a potential a distance r from the surface of the source mass

$$\displaystyle \begin{aligned} V_a (r) \approx \frac{g_s^N g_p^N}{2 m_N} \lambda_a^2 n_N e^{- \frac{r}{\lambda_a}}~, \end{aligned} $$
(8.78)

where mN and nN are the nucleon mass and density of the material, respectively. Here we assume the NMR sample thickness is of order λa and the source mass surface is effectively flat. A spin-polarized nucleus near this rotating sprocket will feel an effective magnetic field of approximately

$$\displaystyle \begin{aligned} \boldsymbol{B}_{\mathrm{eff}} \approx \frac{1}{\hbar \gamma_N} \nabla V_a(r) {\left(1+\cos{}(n \omega_{\mathrm{rot}} t)\right)}~, \end{aligned} $$
(8.79)

where γN is the nuclear gyromagnetic ratio and n is the number of segments, for a sample thickness of order λa. Beff is parallel to the radius of the sprocket.

The NMR sample with net polarization Mz parallel to the axis of the sprocket (and a Larmor frequency 2μN ⋅Bext∕ħ = ω determined by an axial field Bext) will develop a time-varying perpendicular magnetization Mx in response to the resonant effective axion field Beff given by

$$\displaystyle \begin{aligned} M_x(t)\approx \frac{1}{2} n_s p \mu_N \gamma_N B_{\mathrm{eff}} T_2 {\left(e^{-t/T_1}-e^{-t/T_2}\right)} \cos{}( \omega t)~, {} \end{aligned} $$
(8.80)

where p is the polarization fraction, ns is the spin density in the sample, and μN is the nuclear magnetic moment. Mx(t) grows approximately linearly with time until t ∼ T2, the transverse relaxation time, and then decays at the longer longitudinal relaxation time T1. Mx(t) can be detected by a SQUID with its pick-up coil axis oriented radially. Note the SQUID detects the changing magnetization of the sample, not the axion field itself (which is not a “real” magnetic field and thus does not affect the SQUID reading directly).

Superconducting shielding is needed around the sample to screen it from ordinary magnetic field noise which would otherwise limit the sensitivity of the measurement. The ultimate limit is set by spin-projection noise (SPN) in the sample itself [56], given as

$$\displaystyle \begin{aligned} \sqrt{M_N^2} = \sqrt{\frac{\hbar \gamma n_s \mu_{\mathrm{He}}T_2 }{2V}} \end{aligned} $$
(8.81)

and the minimum transverse magnetic resonant field detectable with this setup is given by:

$$\displaystyle \begin{aligned} \begin{array}{rcl} {} B_{\mathrm{min}}& \approx&\displaystyle p^{-1}\sqrt{\frac{2\hbar b}{n_s \mu_{\mathrm{He}} \gamma V T_2}} =3 \times 10^{-19} ~{\mathrm{T}} \\ & &\displaystyle \times \left( \frac{1}{p} \right) \sqrt{ \left(\frac{b}{1\,\mathrm{Hz}}\right) \left( \frac{1\,\mbox{mm}^3}{V}\right) \left( \frac{10^{21}~\mbox{cm}^{-3}}{n_s}\right) \left( \frac{1000~\mbox{s}}{T_2}\right)} . \end{array} \end{aligned} $$
(8.82)

Here V  is the sample volume, γ is the gyromagnetic ratio for 3He = (2π) × 32.4 MHz/T, b is the measurement bandwidth, and μHe = −2.12 × μn is the 3He nuclear moment, where μn is the nuclear Bohr magneton. The estimated SQUID magnetometer limited sensitivity is shown in Fig. 8.9.

Fig. 8.9
figure 9

(left) Setup: a sprocket-shaped source mass is rotated so its “teeth” pass near an NMR sample at its resonant frequency. (right) Projected reach for monopole-dipole axion-mediated interactions. The band bounded by the red (dark) solid line and dashed line denotes the limit set by transverse magnetization noise, depending on achieved T2. Constraints and expectations for the QCD axion also are shown, adapted from Refs. [37, 56]

Full size image

The experiment sources the axion in the lab, and can explore all mass ranges in our sensitivity band simultaneously, unlike experiments which must scan over the allowed axion oscillation frequencies (masses) by tuning a cavity (e.g., as described in Chap. 4) or magnetic field (e.g., as described in Chap. 6). Distinct from other magnetometry experiments [37, 46, 54], the experiment uses a resonant enhancement technique. Assuming sources of systematic error and noise can be mitigated, the approach is expected to be spin-projection noise limited, and in principle allows several orders of magnitude improvement, yielding sufficient sensitivity to detect the QCD axion (Fig. 8.9). In principle, an experiment like ARIADNE could be adapted to use a polarized source mass, in order to search for anomalous dipole-dipole interactions [56]. Using a polarized source mass, however, increases the need for the screening of ordinary magnetic interactions.

8.5 Spectroscopic Measurements of Spin-Spin Coupled Interactions

Comparison of precision spectroscopy of atoms [57] and molecules [58] with theoretical expectations allows one to place stringent constraints on new exotic interactions at atomic scales. As an example, we consider here a recent experiment that has resulted in orders of magnitude improvement for constraints on the existence of anomalous dipole-dipole forces on angstrom length scales [58]. Constraints were obtained by comparison of NMR measurements and theoretical calculations of J-coupling in deuterated molecular hydrogen (HD). Such couplings have the form JI ⋅S (here, I and S are nuclear spin operators) and arise due to a second-order hyperfine interaction. Exotic spin-spin interactions mediated by new bosons, described, for example, by Eqs. (8.28), (8.40), and (8.47), also contain terms proportional to I ⋅S that can lead to a shift ΔJ of the J-coupling. Experimentally measured J-coupling is in good agreement with theoretical calculations [58], ruling out novel angstrom-range anomalous spin-dependent forces at a level several orders of magnitude better than prior constraints from molecular beam measurements [59] (Fig. 8.10).

Fig. 8.10
figure 10

Limits on dipole-dipole couplings between protons and nucleons \(|g_p^N g_p^p|/(\hbar c)\), described by Eq. (8.28), derived from comparison of NMR measurements and theory for J-coupling in deuterated molecular hydrogen [58], shown by the light gray shaded region, along with limits from molecular beam experiments [59], shown by the dark gray shaded region. The prediction for dipole-dipole couplings mediated by the QCD axion in the Kim-Shifman-Vainshtein-Zakharov (KSVZ) model [18] is also shown for comparison. Figure adapted from Ref. [58]

Full size image

8.6 Outlook

Future prospects for improvements in the search for novel spin-dependent interactions are promising with new cryogenic and quantum technologies. Cryogenic torsion balance technology could provide substantial gains beyond the thermal noise limit for spin-dependent torque experiments. Spin squeezing or coherent collective modes could offer prospects for improved sensitivity beyond the standard quantum limit of spin-projection noise in experiments such as ARIADNE, potentially allowing sensitivity all the way down to the SQUID-limited sensitivity (dashed-dotted line in Fig. 8.9). This would allow one to rule out the axion over a wide range of masses, and when combined with other promising techniques [60,61,62], and existing experiments [63, 64] already sensitive to QCD axions, could, in principle, allow the QCD axion to be searched for over its entire allowed mass range.